Lamar has 27 shirts to fold. Let n be the number of shirts he would have left to fold after folding f of them. Write an equation relating n to f. Then use the equation to find the number of shirts he would have left to fold after folding 11 of them.
Answers
Step-by-step explanation:
Number of shirts she didn't fold= n
Number of shirts she folded = f
So = total number of shirts - number of shirts folded = shirts that aren't folded
= equation = 27 - f = n
= so if she folded 11, f = 11
So n = 27 - 11
So n = 16
So, 16 shirts are left to fold
Related Questions
1. Lucy monitors the position of a large fish in the ocean with a depth finder. The device records the depth of the fish. Initially, the fish swims at a depth of 13.52 feet below sea level. Suddenly the fish rises 7.8 feet.
(a) What expression represents the position of the fish relative to sea level after it rises 7.8 feet?
(b) What is the position of the fish after it rises 7.8 feet relative to sea level? Show your work. Answer in a complete sentence.
Answers
(a) The expression that represents the position of the fish relative to sea level after it rises 7.8 feet is 13.52-7.8 = 5.72 , and
(b) The position of fish after rising 7.8 feet will be 5.72 feet below the sea level .
In the question ,
it is given that
fish swims 13.52 below the sea level ,
original depth = 13.52 feet
fish rises by 7.8 feet relative to the sea level
Part(a)
Since the fish has risen 7.8 feet ,
so , the risen height 7.8 feet will be subtracted from original depth 13.52 feet
the expression becomes 13.52 - 7.8 = 5.72 .
Part(b)
The position of fish after it rises 7.8 feet is calculated using
= 13.52 - 7.8
Subtracting 7.8 from 13.52 we get
= 5.72
Therefore , (a) The expression that represents the position of the fish relative to sea level after it rises 7.8 feet is 13.52-7.8 = 5.72 , and
(b) The position of fish after rising 7.8 feet will be 5.72 feet below the sea level .
Learn more about Equation here
https://brainly.com/question/18109354
#SPJ1
\(3 {}^{2} + ( - 4 {}^{2} ) + 5 {}^{2} \)I'm confused on that can you help me please?
![[tex]3 {}^{2} + ( - 4 {}^{2} ) + 5 {}^{2} [/tex]I'm confused on that can you help me please?](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/mzIhnFCA5ANcSNxMzmHyC3SNR139rZS9.jpeg)
Answers
We have to replace the values in the equation
\(x^2+y^2+z^2=(3)^2+(-4)^2+(5)^2=9+16+25=50\)Since the square of a negative number is a positive number, (-4)^2 = +16
"Although Jhon was alive once more that doesn't mean that he was happy. he had fun with his dead mom and a gas truck. " which book is this from
harry poter
emile and the pengins
bob the builder
my wii is broke I need help
all and the panda of fireeeeeeee
cred we pop
can I see ur pe pe
thanks bro
also jk plz don't
Ngithanda iglu kuyinto enhle enamathelayo ngiyabonga bantu
Answers
Answer:
um i think all the panda of fireeeeeeee
Step-by-step explanation:
:D
Which of these characteristics is necessary for the Central Limit Theorem to hold?
a. Each individual measurement must be Normally distributed.
b. Each individual measurement must be Identically distributed
c.Each individual measurement must be Independent of every other measurement
d.Both A and C are necessary for the Central Limit Theorem to hold.
e.Both B and C are necessary for the Central Limit Theorem to hold.
f. All three are necessary for the Central Limit Theorem to hold.
Answers
In the given question both the options D and E are necessary for the Central Limit Theorem to hold.
The Central Limit Theorem (CLT) is a fundamental concept in statistics that describes the behavior of sample means when the sample size is large. According to the CLT, the distribution of sample means approaches a normal distribution regardless of the shape of the original population distribution, given certain conditions.
Option A states that each individual measurement must be normally distributed. This is not a necessary condition for the CLT to hold. The original population distribution does not have to be normal; it can be any distribution shape.
Option B states that each individual measurement must be identically distributed. This is not a necessary condition for the CLT to hold. The measurements can have different distributions, as long as they satisfy the other conditions.
Option C states that each individual measurement must be independent of every other measurement. This is a necessary condition for the CLT to hold. The independence of measurements ensures that each observation contributes to the overall sample mean independently, without being influenced by other observations.
Therefore, options D and E are the correct choices. Both the independence of measurements (option C) and a sufficient sample size (option B) are necessary conditions for the Central Limit Theorem to hold.
Learn more about Central Limit Theorem (CLT) here:
https://brainly.com/question/14267593
#SPJ11
Suppose that Leni and Thom are lawyers and each has a taxable income of $230,000. They can't decide if they should be married in December or in January. If they marry in December, then they are considered married for the entire tax year and could file a joint return. If they get married in January of the next year, they would file a separate return each as a single taxpayer. Examine Schedules X and Y-1. Which filing status would yield the lower tax? If so, by how much? is there really a marriage penalty?
Answers
Answer:
1. Neither would yield the lower tax.
2. (not sure yet about how much)
3. and there is not really a marriage penalty.
6. An angle is equal to two-seventh of its supplement. Find the angle.
Answers
The angle that is equal to two-seventh of its supplement is 40°.
Two angles A and B are supplementary to each other, if and only if m ∠A + m ∠B = 180°.
In the question, we are asked to find the angle that is two-seventh of its supplement.
We assume the angle to be x°.
The supplement of this angle will be valued as (180 - x)°.
Now, we are told that the required angle is two-seventh of its supplement, that is, x = (2/7)(180 - x), which makes an equation in the variable x.
We can solve this equation as follows:
x = (2/7)(180 - x),
or, 7x = 360 - 2x,
or, 7x + 2x = 360,
or, 9x = 360,
or, x = 40.
Thus, the angle that is equal to two-seventh of its supplement is 40°.
Learn more about supplementary angles at
https://brainly.com/question/2046046
#SPJ9
How much the statistic varies from one sample to another is known as the ____ _____ of a statistic.
Answers
How much a statistic varies from one sample to another is known as the sampling variability of a statistic.
Testing variability arises due to the reality that exceptional samples of the same size can produce special values of a statistic, although the samples are described from the same populace. the quantum of slice variability depends on the scale of the sample, the variety of the populace, and the unique statistic being calculated.
The end of statistical conclusion is to apply the data attained from a sample to make consequences about the population, while counting for the slice variability of the statistic. ways similar as thesis checking out and tone belief intervals do not forget the sampling variability of a statistic to make redundant accurate consequences about the populace.
Learn more about sampling variability:-
https://brainly.com/question/30530844
#SPJ4
Keisha is saving money to buy a game. So far she has saved $12, which is three-fourths of the total cost of the game. How much does the game cost?
Answers
if you divide 12 by three to get the three parts you get 4. each fourth of the price is $4. you then multiply that by 4 to get $16
Consider the following. x = e^t, y = e^(−3t)
(a) Eliminate the parameter to find a Cartesian equation of the curve.
(b) Sketch the curve and indicate with an arrow the direction in which the curve is traced as the parameter increases.
Answers
To indicate the direction in which the curve is traced as the parameter increases, we can draw an arrow that points from lower left to upper right, since the curve moves from the point (1, 1) at t = 0 to the right and upward as t increases.
To eliminate the parameter, we can use the fact that x = e^t and y = e^(-3t) to solve for t in terms of x and y. Taking the natural logarithm of both sides of x = e^t, we get ln(x) = t. Similarly, taking the natural logarithm of both sides of y = e^(-3t), we get ln(y) = -3t, or t = (-1/3)ln(y). Substituting this expression for t into the equation we found for ln(x), we get ln(x) = (-1/3)ln(y), which simplifies to ln(x^3) = ln(y^(-1)), or x^3 = 1/y. This is the Cartesian equation of the curve.
To sketch the curve, we can start by noting that both x and y are positive for all values of t, since e^t and e^(-3t) are always positive. As t increases, x and y both increase, but y increases much more slowly than x since e^(-3t) decreases rapidly as t increases. This means that the curve starts out very steep (since the slope of the tangent line at t = 0 is dx/dt = 1 and dy/dt = -3), but becomes flatter and flatter as t increases. The curve approaches the x-axis as t approaches infinity, but never touches it.
Know more about logarithm here:
https://brainly.com/question/30085872
#SPJ11
ASKING FOR A LONG TIME PLS HELP GONNA MAKE BRAINLIEST all i know is 20 2/3 is not right. TYSM
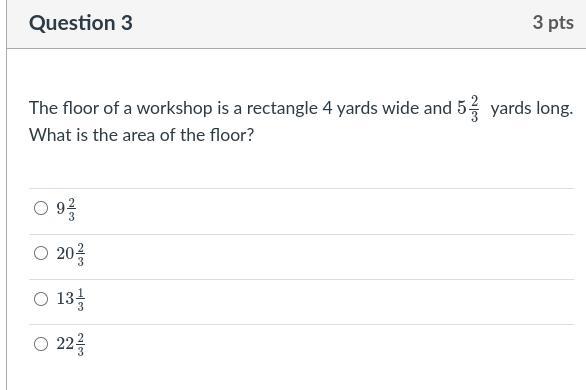
Answers
Answer:
it is 20 2/3
Step-by-step explanation:
because the area/formula of a rectangle is l x w so multiply the 4 and 5 2/3 and you get B...
Length: (5 2/3) 5.66 x width: 4 = 22.666 or 22 2/3
Help me if you can. don't give me the wrong answer

Answers
Answer:
72
The angles in a triangle adds to 180 so 180-62-44=72
Please thank and rate
and possibly brainlest
Have a great day

Cine mark is having a summer special. If you get a membership, the one-time cost is $ 12.50 plus $2.00 per movie that you watch. Write a linear equation that will show the cost of membership and each movie seen.
Slope:
Y-intercept:
Equation:
Answers
Answer:
Y= 12.50 x + 2.00
Finding the sum of the measures of the interior angles of a convex nonagon
Answers
Answer:
7 pie
Step-by-step explanation:
the sum of the interior angles of a nonagon is 1260 degrees.
Now The sum of interior angles of a convex N-sided polygon equals to π(N−2) radians.
In case N=9 (nanogon)
this sum is
π(9−2)=7π (radians)
The sum of the measures of the interior angles of a convex nonagon is given by the equation A = 1260°
What is the sum of the interior angles of a polygon?
The sum of the interior angles of a polygon is given by the formula
Sum of Interior angles of a polygon with n sides is
nθ = 180 ( n - 2 )
where n is the number of sides
θ = angle in degrees
Given data ,
Let the sum of the interior angles of a convex nonagon be A
Now , the equation will be
Let the number of sides of the polygon be n = 9 sides
The sum of the interior angles of a polygon is calculated by the equation ,
nθ = 180 ( n - 2 )
Substituting the values in the equation , we get
nθ = 180 ( 9 - 2 )
nθ = 180 x 7
nθ = 1260°
Therefore , the value of A is 1260°
Hence , the sum of angles is 1260°
To learn more about sum of interior angles click :
https://brainly.com/question/14611583
#SPJ5
Hot Spot is a California lottery game. Players pick 1 to 10 Spots (sets of numbers, each from 1 to 80) that they want to play per draw. For example, if you select a 4 Spot, you play four numbers. The lottery draws 20 numbers, each from 1 to 80. Your prize is based on how many of the numbers you picked 27% match one of those selected by the lottery. The odds of winning depend on the number of Spots you choose to play. For example, the overall odds of winning some prize in 4 Spot is approximately 0.256.
You decide to play the 4 Spot game and buy 5 tickets. Let X be the number of tickets that win some prize. 6452 Location 18277 of 68468 32°F Mostly cloudy 6:10 3/14 the location of the mean on your histogram.
a. Xhas a binomial distribution. What are n and p?
b. What are the possible values that x can take?
c, Find the probability of each value of X. Draw a probability histogram for the distribution of X. (See Figure 14.2 on page 331 for an example of a probability histogram.)
d. What are the mean and standard deviation of this distribution? Mark the location of the mean on your histogram
Answers
Based on the information provided, a) if X has a binomial distribution, n = 5 and p = 0.256. b) X can take values in the range of 0 to 5. c) The values of P(x) for x = 0, 1, 2, 3, 4 , 5 are 0.228, 0.392, 0.269, 0.093, 0.016, and 0.011 respectively. d) mean = 1.28 and standard deviation = 0.995.
a) If X has a binomial distribution n represents the number of tickets bought which is 5 and p represents the probability of winning a prize after taking a single ticket which is 0.256.
b) X can take values in the range of 0 to 5, which indicates the possible number of won tickets. Hence, x = 0, 1, 2, 3, 4, 5. c)
Using the binomial distribution formula, P (x) = nCx*p^x*(1 – p)^(n-x). Hence,
P(0) = 5C0*(0.256)^0*(1 – 0.256)^)(5-0) = 0.228
P(1) = 5C1*(0.256)^1*(1 – 0.256)^)(5-1) = 0.392
P(2) = 5C2*(0.256)^2*(1 – 0.256)^)(5-2) = 0.269
P(3) = 5C3*(0.256)^3*(1 – 0.256)^)(5-3) = 0.093
P(4) = 5C4*(0.256)^4*(1 – 0.256)^)(5-4) = 0.016
P(5) = 5C5*(0.256)^5*(1 – 0.256)^)(5-5) = 0.011
d) The mean and standard deviation of the distribution is given by:
mean = n*p = 5*0.256 = 1.28
standard deviation = √np(1 – p) = √5(0.256)(1 – 0.256) = 0.995
Learn more about Binomial distribution:
https://brainly.com/question/24756209
#SPJ4
The following formula gives the volume
�
VV of a pyramid, where
�
AA is the area of the base and
ℎ
hh is the height:
�
=
1
3
�
ℎ
V=
3
1
AhV, equals, start fraction, 1, divided by, 3, end fraction, A, h
Rearrange the formula to highlight the base area.
�
=
A=A, equals
Answers
The required steps are as follows:
Multiply both sides of the equation by 3.
Divide both sides of the equation by h.
To rearrange the formula to highlight the base area, we can first multiply both sides of the equation by 3 to get:
V = 3Ah
Then, we can divide both sides of the equation by h to get:
A = V/h
Therefore, the rearranged formula to highlight the base area is A = V/h.
Learn more about the rearrangement of the formula here:
https://brainly.com/question/11963448
#SPJ1
-4(w+1) = -24−4(w+1)=−24minus, 4, left parenthesis, w, plus, 1, right parenthesis, equals, minus, 24 w =w=w, equals
Answers
Answer:
W=5
Step-by-step explanation:
-4(w+1}=-24
-4w-4=-24
-4w=-20
w=5
Answer:
its 5
Step-by-step explanation:
i got i correct ;)
The residents of a city voted on whether to raise property taxes. The ratio of yes votes to no votes was 5 to 4. If there were 2348 no votes, what was the total
number of votes?

Answers
Answer:
2935
Step-by-step explanation:
if you divide the total of no votes (2348) to 4 you will get 587
587 x the number of yes votes (5)
would be 2935
Let A,B, and C be the matrices with sizes 2×2,2×3, and 3×2 respectively. Which of the following is/are true? (select all that apply) If the product AB=0 (zero matrix), then either A=0 or B=0. Both BC and CB are square matrices. The matrix A+BC is defined. A=Al2=I2 A, where I2 is the 2×2 identity matrix. BC=CB
Answers
The correct statements are:
1. If the product AB = 0 (zero matrix), then either A = 0 or B = 0.
4. BC = CB.
Explanation:
1. If the product of two matrices AB equals the zero matrix, it implies that at least one of the matrices A or B (or both) must be the zero matrix for the product to result in zero.
4. The statement BC = CB states that the order of multiplication of matrices B and C does not affect the resulting matrix. This property holds true for matrices of any size as long as the dimensions are compatible for matrix multiplication.
The other statements are not necessarily true in general:
2. Both BC and CB being square matrices is not guaranteed. The product of two matrices can result in a square matrix only if the number of columns of the first matrix is equal to the number of rows of the second matrix. In this case, B has 3 columns and C has 2 rows, so BC and CB are not square matrices.
3. The matrix A + BC is not defined since the addition of matrices requires them to have the same dimensions. In this case, A is a 2×2 matrix, while BC is a 2×3 matrix, so they cannot be added together.
5. The statement A = A12 = I2 implies that matrix A is equal to the 2×2 identity matrix I2. However, this is not necessarily true as the given information does not provide any details about the values or properties of matrix A.
Learn more about matrix here:-
https://brainly.com/question/29995229
#SPJ11
Show that the process X(t):=e t/2
cos(W(t)),0≤t≤T, is a martingale w.r.t. any filtration for Brownian motion and represent it as an Itô process on any time interval [0,T],T>0.
Answers
A stochastic process X(t) is called a martingale if the expected value of X(t) given all information available up to and including time s is equal to the value of X(s).
Thus, to show that the process X(t):=e^(t/2)cos(W(t)), 0 ≤ t ≤ T is a martingale w.r.t. any filtration for Brownian motion, we need to prove that E(X(t)|F_s) = X(s), where F_s is the sigma-algebra of all events up to time s.
As X(t) is of the form e^(t/2)cos(W(t)), we can use Itô's lemma to obtain the differential form:dX = e^(t/2)cos(W(t))dW - 1/2 e^(t/2)sin(W(t))dt
Taking the expectation on both sides of this equation gives:E(dX) = E(e^(t/2)cos(W(t))dW) - 1/2 E(e^(t/2)sin(W(t))dt)Now, as E(dW) = 0 and E(dW^2) = dt, the first term of the right-hand side vanishes.
For the second term, we can use the fact that sin(W(t)) is independent of F_s and therefore can be taken outside the conditional expectation:
E(dX) = - 1/2 E(e^(t/2)sin(W(t)))dt = 0Since dX is zero-mean, it follows that X(t) is a martingale w.r.t. any filtration for Brownian motion.
Now, let's represent X(t) as an Itô process on the interval [0,T]. Applying Itô's lemma to X(t) gives:
dX = e^(t/2)cos(W(t))dW - 1/2 e^(t/2)sin(W(t))dt= dM + 1/2 e^(t/2)sin(W(t))dt
where M is a martingale with M(0) = 0.
Thus, X(t) can be represented as an Itô process on [0,T] of the form:
X(t) = M(t) + ∫₀ᵗ 1/2 e^(s/2)sin(W(s))ds
Hence, we have shown that X(t) is a martingale w.r.t. any filtration for Brownian motion and represented it as an Itô process on any time interval [0,T], T > 0.
To know more about martingale visit:
brainly.com/question/32735198
#SPJ11
What is a linear function table?.
Answers
A linear function table is a table having a constant rate of change between its datapoints
A linear equation is is an equation that consists of each variable raised with a maximum of one degree. the linear equation in terms of variables x and y can be written in the form of y = mx + c, where m is the slope and x is the independent variable, and y is the dependent variable when the data points of the given table are potted in a graph, we get a straight line for a linear function table, a such function must have a constant slope in between its points
to know more about linear function table refer to the link https://brainly.com/question/20286983?referrer=searchResults.
#SPJ4
What is the predetermined overhead rate? \( \$ 10.00 / \mathrm{MH} \) \( \$ 17.50 / \mathrm{MH} \) \( \$ 20.00 \) / MH \( \$ 32.86 / \mathrm{MH} \)
Answers
The predetermined overhead rate is the estimated manufacturing overhead cost per unit of a specific allocation base.
In the options, there are four different rates:
1. $10.00 / MH (MH stands for machine hour): This means that the estimated manufacturing overhead cost per machine hour is $10.00.
2. $17.50 / MH: This indicates that the estimated manufacturing overhead cost per machine hour is $17.50.
3. $20.00 / MH: This implies that the estimated manufacturing overhead cost per machine hour is $20.00.
4. $32.86 / MH: This shows that the estimated manufacturing overhead cost per machine hour is $32.86.
Each rate represents the estimated cost of manufacturing overhead per unit of the allocation base (machine hour) and is used to allocate overhead costs to products or services based on their usage of the allocation base.
The specific rate chosen depends on the nature of the business, its cost structure, and the accuracy of the estimated overhead costs.
The correct question is ''What is the predetermined overhead rate?\(\( \$ 10.00 / \mathrm{MH} \) \( \$ 17.50 / \mathrm{MH} \) \( \$ 20.00 \) / MH \( \$ 32.86 / \mathrm{MH} \)\).''
To know more about overhead rate refer here:
https://brainly.com/question/31953044#
#SPJ11
D
Question 1
(02.03)
Read the following statement: Line segment CD is congruent to line segment XY.
Which of the following is an equivalent statement? (4 points)

Answers
Dorothy has a basket of apples. She weighs them and makes a table to show how many apples she has of each weight. Make a line plot to show the data. What is the total weight of Dorothy's apples? Dorothy groups apples that have the same weight in a basket. She has 5 baskets. Do any baskets have the weight?
Answers
The line plot shows that Dorothy has two baskets of 5 apples each, one basket of 3 apples, one basket of 2 apples, and one basket of 1 apple. The total weight of Dorothy's apples is 16.
Dorothy has a basket of apples. She decided to weigh the apples and make a table to show how many apples she has of each weight. She created a line plot to show the data. From the line plot, it can be seen that Dorothy has two baskets of 5 apples each, one basket of 3 apples, one basket of 2 apples, and one basket of 1 apple. The total weight of Dorothy's apples is 16. This means that Dorothy has a total of 16 apples. Since Dorothy has grouped apples that have the same weight in a basket, it can be seen that each basket has a different weight. The two baskets of 5 apples each have a total weight of 10, the basket of 3 apples has a total weight of 3, the basket of 2 apples has a total weight of 2, and the basket of 1 apple has a total weight of 1. Therefore, Dorothy has a total of 16 apples with a total weight of 16.
Learn more about weight here
https://brainly.com/question/28355526
#SPJ4
5. water is being poured into a cone-shaped cup at a rate of 60 m' per second. the height of the cup is h meter and the vertical angle is 60'. how fast is h 60° (a) the radius of the surface of the water increasing when the depth of the water is 10 m? [5 marks] (b) the water level increasing when the depth of the water is 15 m? (5 marks]
Answers
When the depth of the water is 10 m, the radius is increasing at a rate of 80 m/s, and when the depth is 15 m, the water level is increasing at the same rate of 80 m/s.
To solve the problem, we'll use related rates, which involves finding the rate at which one quantity changes with respect to another quantity. Let's address each part of the problem separately:
(a) To find the rate at which the radius of the surface of the water is increasing when the depth of the water is 10 m, we'll need to find the relationship between the radius and the depth.
Using the given information, we know that the vertical angle of the cone is 60°. This means the half-angle at the apex of the cone is 30°.
Now, let's denote the radius of the surface of the water as 'r' and the depth of the water as 'd'. We want to find dr/dt, the rate at which the radius is changing with respect to time.
By considering the similar triangles formed by the height of the cup, the radius, and the depth of the water, we can establish the relationship:
r/d = tan(30°)
To find dr/dt, we differentiate both sides of the equation with respect to time:
\((1/d) * (dr/dt) = sec^2(30°) * (d/dt)\)
Since the rate at which the water is being poured is given as 60 m^3 per second, d/dt = \(60 m^3/s.\)
Plugging in the values, we have:
\((1/10) * (dr/dt) = sec^2(30°) * 60\)
Simplifying, we find:
\((dr/dt) = (600 * sec^2(30°))/10\)
sec(30°) = 1 / cos(30°) = 1 / (√3/2) = 2/√3 = (2√3) / 3
Now, let's substitute this value into the equation and simplify further:
(1/10) * (dr/dt) = (sec^2(30°)) * 60
(1/10) * (dr/dt) = ((2√3) / 3)^2 * 60
(1/10) * (dr/dt) = (4/3) * 60
(1/10) * (dr/dt) = 80
Finally, we can solve for (dr/dt) by multiplying both sides of the equation by 10:
(dr/dt) = 80 * 10
(dr/dt) = 800
Finally, we substitute the value of \(sec^2(30°) = 4/3:\)
\((dr/dt) = (800/10) = 80 m/s\)
Therefore, when the depth of the water is 10 m, the radius of the surface of the water is increasing at a rate of 80 m/s.
(b) To find the rate at which the water level is increasing when the depth of the water is 15 m, we need to determine dh/dt, the rate at which the height is changing with respect to time.
Using the relationship between the height and the depth of the water in the cone, we have:
h = d * tan(30°)
Differentiating both sides of the equation with respect to time, we get:
Let's denote the derivative with respect to time as d/dt. The chain rule states that if y = f(u) and u = g(t), then dy/dt = dy/du * du/dt.
In this case, we have h = d * tan(30°), where d is a constant. Differentiating both sides with respect to time, we get:
d/dt (h) = d/dt (d * tan(30°))
The derivative of h with respect to time (dh/dt) is what we're looking for. The derivative of d (a constant) with respect to time is zero since it is not changing.
To differentiate tan(30°), we need to use the trigonometric identity:
d/dx (tan(x)) = sec^2(x)
Therefore:
d/dt (tan(30°)) = sec^2(30°)
Substituting these results back into the original equation, we have:
dh/dt = 0 + d/dt (d * tan(30°))
dh/dt = 0 + d * sec^2(30°)
Since \(sec^2(30°)\) is a constant, we can simplify further:
dh/dt = d * \(sec^2(30°)\)
dh/dt =\(sec^2(30°) * (d/dt)\)
Again, substituting d/dt =\(60 m^3/s\), we have:
dh/dt =\(sec^2(30°) * 60\)
Using the value of sec^2(30°) = 4/3:
dh/dt = (4/3) * 60
Simplifying, we find:
dh/dt = 80 m/s
Therefore, when the depth of the water is 15 m, the water level is increasing at a rate of 80 m/s.
Learn more about ”depth” here:
brainly.com/question/13804949
#SPJ11
solve the problem and then click on the correct answer choice. jane must select 3 different items for each dinner she will serve. the items are to be chosen from among 5 different vegetarian and 4 different meat selections. if at least one of the selections must be vegetarian, how many different dinners can jane create?
Answers
Jane can select 80 different types of dinner consisting of 3 different items from 5 different vegetarian items and 4 different meat items available.
For finding the various combination of dinners available which has atleast one vegetarian item we will apply combination formula with the given data i.e., \(^{n} C_{r} = \frac{n!}{(n-r)!.r!}\)
A combination is a way of selecting items from a collection where the order of selection does not matter.
We will have to apply the formula separately for vegetarian and meat items.
Combinations available
A. 1 vegetarian item and 2 meat items
\(^{5} C_{1} and ^{4} C_{2}\) (In combination "and" means to multiply)
= \(\frac{5!}{(5-1)!.1!} . \frac{4!}{(4-2)!.2!}\)
= \(\frac{5!}{(4)!.1!} . \frac{4!}{(2)!.2!}\)
= \(\frac{5.4.3.2.1}{(4.3.2.1).(1)}. \frac{4.3.2.1}{(2.1).(2.1)}\)
= 5.3.2
= 5.6
= 30
B. 2 vegetarian items and 1 meat item
\(^{5} C_{2} and ^{4} C_{1}\)
= \(\frac{5!}{(5-2)!.2!} . \frac{4!}{(4-1)!.1!}\)
= \(\frac{5!}{(3)!.2!} . \frac{4!}{(3)!.1!}\)
= \(\frac{5.4.3.2.1}{(3.2.1).(2.1)}. \frac{4.3.2.1}{(3.2.1).(1)}\)
= 5.2.4
= 10.4
= 40
C. 3 vegetarian items and no meat item
= \(^{5} C_{3} and ^{4} C_{0}\)
= \(\frac{5!}{(5-3)!.3!} . \frac{4!}{(4-0)!.0!}\)
= \(\frac{5.4.3.2.1}{(2.1).(3.2.1)}. 1\)
= 5.2
= 10
Now, we will add all the different combination
Total combinations = 30+40+10
= 80 different type of dinners
To know more about combination
https://brainly.com/question/13387529
#SPJ4
C. Find the equation of a line passing through (2, 2) and having inclination 30º.
Answers
The equation of line is (y-2) = 1/\(\sqrt{3}\)(x-2)
What is Equation of line?
The equation of a line is the algebraic representation of a line using cartesian coordinates.
The general form of the equation of a straight line is written as y = mx + cy=mx+c.
Where m is the gradient of the straight line
and c is the y-intercept of the straight line.
And
The equation of line passing through point (\(x_{1}\), \(y_{1}\)) is
(y-\(y_{1}\)) = m (x-\(x_{1}\))
Given line in the graph passes through (2,2).
We have to find the equation of the line.
Given, angle of inclination is 30.
m = tan (angle of inclination)
m = tan 30
m = 1/\(\sqrt{3}\)
Therefore after substituting, equation of line
(y-2) = 1/\(\sqrt{3}\)(x-2)
To know more about equation of line, visit:
https://brainly.com/question/11552995
#SPJ9
Help again please
Brainliest if correct

Answers
Answer:
it would be the first one i think
Step-by-step explanation:
Answer:
Option C: between 2.75 and 3.0
Step-by-step explanation:
Maybe this might help somewhat.

Does this triangle have a hypotenuse of 39 feet long

Answers
Answer:
Yes it does
Step-by-step explanation:
did it on edge
What are the measurements of the little 2 small cube things on each side, better yet what's the surface area? Thank you

Answers
Answer:
166 units²Step-by-step explanation:
We know that:
W = 7L = 5H = 4Surface area is the area of the faces of the cuboid.
Work:
=> 2(7 x 4) + 2(5 x 4) + 2(5 x 7)=> 2(28) + 2(20) + 2(35)=> 56 + 40 + 70=> 166 units²Hence, Option C is correct.
Hoped this helped.
\(BrainiacUser1357\)
Write the negation of the following statement: ABC and DEC are
complementary angles.
A)OZABC and ZDEC are not complementary angles.
B)ZABC and ZDEC are supplementary angles.
C)The sum of ABC and ZDEC is 180 degrees.
D)The sum of ABC and ZDEC is 90 degrees.
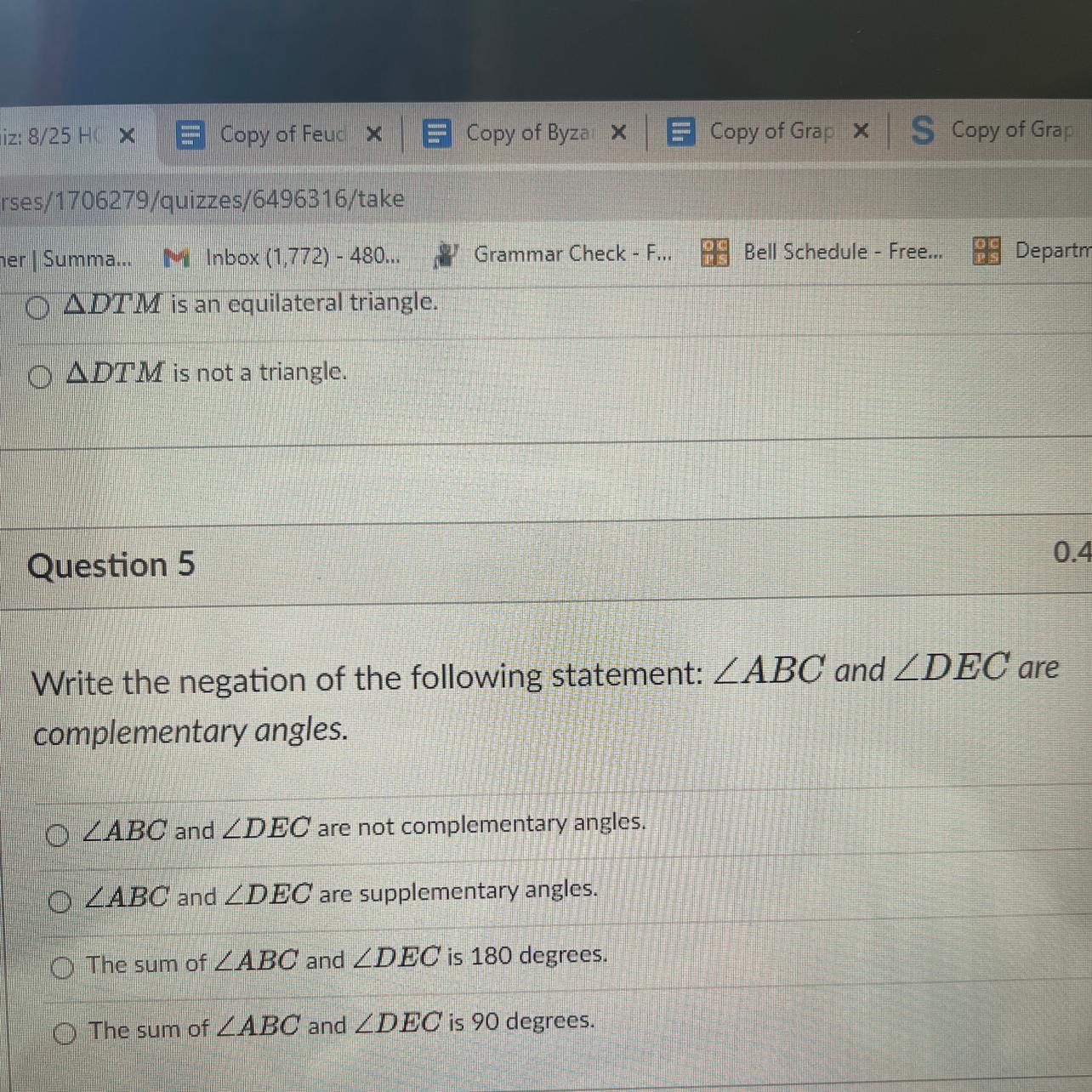
Answers
Why?
Complementary angles: add up to 90 degrees.
Supplementary angles: add up to 180
degrees.