multiply (2x – 3)(x2 – 5x + 3)
Answers
Answer:
(2x2- 10x2+ 6x -3x2 + 15x -9) simplified to (-12x2+21x-9)
Step-by-step explanation:
I could explain but you would just be confused because I did it in my head and I don't do it the way they teach us to if you want to make sure my answer is right you should be able to simplify my simplified version back into the original problem
Related Questions
HURRY PLS I NEED IT
What is the purpose of apportionment? (its ideal, value, or vision)
Answers
Answer:
An apportionment is the allocation of a loss between all of the insurance companies that insure a piece of property. This allocation is used to determine a percentage of liability for each insurer.
I'm new here! Sorry if this didn't help
What is the zero of (-8, -4) and (7, 8)?
Answers
Answer:
212
Step-by-step explanation:
Amir spent a total of $53.75 on breakfast sandwiches and bagels for his friends who are visiting from out of town. Each breakfast sandwich costs $4.25, and each bagel costs $2.00. He bought 5 more bagels than breakfast sandwiches.
Write a system of equations that could be used to solve for the number of breakfast sandwiches and bagels he bought.
And solve the system of equations to determine how many bagels and sandwiches Amir bought.
Answers
The system of equations is:
2b + 4.25s = 53.75
b - s = 5
The number of bagels bought is 12 and the number of sandwiches bought is 7.
How many bagels and sandwiches were bought?2b + 4.25s = 53.75 equation 1
b - s = 5 equation 2
Where:
n = number of bagels bought s = number of sandwiches boughtThe elimination method would be used to determine the required values.
Multiply equation 2 by 2
2b - 2s = 10 equation 3
Subtract equation 3 from equation 1 :
6.25s = 43.75
Divide both sides of the equation by 2.25
s = 43.75 / 6.25
s = 7
Substitute for s in equation 1:
2b + 4.25(7) = 53.75
2b + 29.75 = 53.75
2b = 53.75 - 29.75
2b = 24
b = 24 / 2
b = 12
To learn more about system of equations, please check: https://brainly.com/question/25875552
#SPJ1
A tank contains 60 kg of salt and 1000 L of water. Pure water enters a tank at the rate 6 L/min. The solution is mixed and drains from the tank at the rate 3 L/min. (a) What is the amount of salt in the tank initially? (b) Find the amount of salt in the tank after 4.5 hours. (c) Find the concentration of salt in the solution in the tank as time approaches infinity. (Assume your tank is large enough to hold all the solution.)
Answers
Initially, the tank contains 60 kg of salt, calculated by multiplying the salt concentration (0.06 kg/L) by the water volume (1000 L).
In the given scenario, the tank starts with a known salt concentration and water volume. By multiplying the concentration (0.06 kg/L) with the water volume (1000 L), we find that the initial amount of salt in the tank is 60 kg.
After 4.5 hours, considering the rate of water entering and leaving the tank, the net increase in solution volume is 810 L. Multiplying this by the initial concentration (0.06 kg/L), we determine that the amount of salt in the tank after 4.5 hours is 48.6 kg.
As time approaches infinity, with a constant inflow and outflow of solution, the concentration of salt in the tank stabilizes at the initial concentration of 0.06 kg/L.
Learn more about Multiplying click here :brainly.com/question/25834626
#SPJ11
guy pls help me!!!!!

Answers
STEP BY STEP EXPLANATION:
90-46=44
44-8=36
36/9= 4
Work out the shaded area

Answers
Answer:
193.28 cm^2
Step-by-step explanation:

Answer:
366 cm²Step-by-step explanation:
Refer to attached
The ares consists of half-circle and a triangle
Radius of circle:
22 cm/2 = 11 cmArea = 1/2*3.14*11² ≈ 190 cm²Sided of the triangle:
Base = 22 cm, height = 16 cmArea = 1/2*22*16 = 176 cm²Total area:
190 + 176 = 366 cm²
18. a. If B is any echelon form of A, then the pivot columns of B form a basis for the column space of A. b. Row operations preserve the linear dependence relations among the rows of A. C. The dimension of the null space of A is the number of Columns of A that are not pivot columns.
Answers
a. True. Pivot columns of an echelon form of A form a basis for the column space of A.
b. True. Row operations preserve linear dependence relations among the rows of A.
c. False. The dimension of the null space of A is the number of columns of A minus the number of pivot columns.
18. a. If B is any echelon form of A, then the pivot columns of B form a basis for the column space of A.
This statement is true. An echelon form of a matrix is obtained by performing row operations on the original matrix to transform it into a specific triangular form. In this form, the pivot columns correspond to the columns containing the leading entries in each row. The pivot columns of an echelon form of matrix A will also be pivot columns of matrix A itself.
The column space of a matrix is the span of its column vectors. Since the pivot columns of B are a subset of the column vectors of A, they will also span the column space of A. Therefore, the pivot columns of B form a basis for the column space of A.
b. Row operations preserve the linear dependence relations among the rows of A.
This statement is true. When we perform row operations on a matrix, such as multiplying a row by a scalar, adding rows together, or swapping rows, the resulting matrix will have the same row space as the original matrix. This means that the linear dependence relations among the rows of the original matrix will be preserved in the transformed matrix.
c. The dimension of the null space of A is the number of columns of A that are not pivot columns.
This statement is false. The dimension of the null space of A, also known as the nullity of A, is the number of free variables in the reduced row echelon form of A. It is equal to the number of columns of A minus the number of pivot columns. Therefore, the dimension of the null space of A is the number of columns of A minus the number of pivot columns, rather than the other way around.
To summarize:
a. True. Pivot columns of an echelon form of A form a basis for the column space of A.
b. True. Row operations preserve linear dependence relations among the rows of A.
c. False. The dimension of the null space of A is the number of columns of A minus the number of pivot columns.
Know more about Pivot columns here,
https://brainly.com/question/28583749
#SPJ11
The cells of a particular micro-organism increase logarithmically. If g represents cell growth and h represents time, in hours, which graph best represents the growth pattern of the cells of this organism?
Answers
Micro-organisms change large organic molecules to forms that can be absorbed and used inside the cell through the process of decomposition.
We have,
Different organic compounds decompose when plant residues are added back to the earth. Decomposition is a biological process in which complicated organic molecules found in dead matter are physically broken down and biochemically converted into less complex organic and inorganic molecules.
The biological activity and the process of cycling carbon in the soil are both aided by the ongoing increase of decomposing plant residues to the soil's surface. These processes are also aided by the decomposition of soil organic matter and the development and decay of roots. Carbon cycling is the ongoing transformation of organic and inorganic carbon compounds in the earth, plants, and the atmosphere by micro- and macro organisms.
The organic molecules in organic matter are broken down during decomposition into smaller organic molecules that need additional breakdown or into mineralized minerals. Different organic substances can be broken down by microorganisms in different ways. Amino acids and sugars are among the first organic substances to break down because they are simple to do so. Lignin, phenols, and waxes will last the longest in the earth, while cellulose will degrade more slowly.
In this question, the process that occurs when micro-organisms change large organic molecules to forms that can be absorbed and used inside the cell is decomposition.
To know more about Decomposition, visit,
brainly.com/question/8009068
#SPJ1
complete question:
How do micro-organisms change large
organic molecules to forms that can be
absorbed and used inside the cell
Who know how to do this ??♀️

Answers
Answer:
Not me sorry I can't help nut I hope you get the answer
Jason has 34 blueberry scones and 85 raspberry scones. There is 17 bags of scones each filled with an equal amount of blueberry scones and raspberry scones. How many blueberry scones and how many blackberry scones can go in each bag?
Answers
Answer:
2 blueberry and 5 raspberry scones go in each bag
Step-by-step explanation:
34+85=119
119/17=7
34/85= 2/5
so 2 blueberry and 5 raspberry scones go in each bag
hope that helps :)
Answer:
2 Blueberry scones and 5 Raspberry scones
Step-by-step explanation:
Given:
34 Blueberry scones
85 raspberry scones
17 Bags to fit scones
34 Blueberry so 34 divided by the Bag 17 (34 ÷ 17 = 2)
85 Raspberry scones so 85 divided by the Bag 17 ( 84 ÷ 17 = 5)
Therefore Jason can put 2 Blueberry scones and 5 Raspberry scones in each Bag
Select the correct description for the quadratic expression below

Answers
the product of x and a factor not depending on x
What is quadratic equation
it's a second-degree quadratic equation which is an algebraic equation in x. Ax2 + bx + c = 0, where a and b are the coefficients, x is the variable, and c is the constant term, is the quadratic equation in its standard form. A non-zero term (a 0) for the coefficient of x2 is a prerequisite for an equation to be a quadratic equation. The x2 term is written first, then the x term, and finally the constant term is written when constructing a quadratic equation in standard form. In most cases, the numerical values of letters a, b, and c are expressed as integral values rather than fractions or decimals.
The equation given 3x(m-6n)²
This expression contains two factors:
1 factor: 3x
2 factor: (m-6n)²
Hence the product of x and a factor not depending on x.
Learn more about quadratic equation , by the following link.
https://brainly.com/question/1214333
#SPJ1
Which of the following ordered pairs represents a solution to the equation below?
y = 3x−2
Answers
Answer:
(0,-2) (1,1) (2,4)
Step-by-step explanation:
X1,X2,...,XnX1,X2,...,Xn be a random sample of size n from the exponential distribution whose pdf isf(x:θ)=(1/θ)e−x/θ,0
Answers
To maximize the likelihood function, we take the derivative with respect to θ and set it equal to zero: d/dθ[L(θ|X1,X2,...,Xn)]=−n/θ+(X1+X2+⋯+Xn)/θ2=0.
The MLE for θ in the exponential distribution is simply the sample mean of the observed data.
The exponential distribution is a continuous probability distribution that describes the time between events in a Poisson point process. X1,X2,...,XnX1,X2,...,Xn is a random sample of size n from this distribution, which means that each XiXi is an independent and identically distributed random variable with the same exponential distribution.
The probability density function (pdf) of the exponential distribution is given by f(x:θ)=(1/θ)e−x/θ, where θ is the scale parameter. This means that the probability of observing a value x from the distribution is proportional to e−x/θ, with the constant of proportionality being 1/θ.
To estimate the value of θ based on the observed data, we can use the method of maximum likelihood estimation (MLE). The likelihood function for the sample X1,X2,...,XnX1,X2,...,Xn is given by L(θ|X1,X2,...,Xn)=∏i=1n(1/θ)e−Xi/θ=(1/θ)n e−(X1+X2+⋯+Xn)/θ.
Solving for θ, we get θ=(X1+X2+⋯+Xn)/n, which is the sample mean.
Know more about exponential distribution here:
https://brainly.com/question/31130457
#SPJ11
the value of “y” varies directly with “x”. if y= 56, then x= 4
Answers
write the equation in slope intercept-form for the line that passes through (0,-7) and (10,3)
Answers
Help me fast please!

Answers
Answer:
10^6
Step-by-step explanation:
The reason is because 2x5 = 10 and then you add the exponents when multiplying, making it 10^8
The price of an item has risen to $301 today. Yesterday it was $140 Find the percentage increase.
Answers
Answer: 115%
Step-by-step explanation: ur welcome
Answer:
53.2%
Step-by-step explanation:
301x53.2%= 160.132 = 160.13
301-160.13=140.87
About 53.2%
what is the slope of a line whose equation is 2x-2y=20
Answers
Answer:
The slope is 1.
Step-by-step explanation:
2x - 2y = 20
Move 2x to the other side by subtracting it.
-2y = -2x + 29
Divide by -2 on both sides
y = x - 13
Mark as Brainliest if you found it helpful!
Determine whether the following improper integral converges or diverges. If it converges, find its value. Hint: integrate by parts.
∫[infinity]17ln(x)x3dx
Use your answer above and the Integral Test to determine whether
[infinity]∑n=17ln(n)n3
is a convergent series.
Answers
The series \(\sum n=17^{[\infty]} ln(n)/n^3\) is a convergent series.
To determine whether the improper integral
\(\int [\infty,17] ln(x)/x^3 dx\)
converges or diverges, we can use the Limit Comparison Test.
Let's compare it to the convergent p-series \(\int [\infty] 1/x^2 dx:\)
lim x→∞ ln(x)/\((x^3 * 1/x^2)\) = lim x→∞ ln(x)/x = 0
Since the limit is finite and positive, and the integral ∫[infinity] \(1/x^2\) dx converges, by the Limit Comparison Test, we can conclude that the integral \(\int [\infty,17] ln(x)/x^3 dx\) converges.
To find its value, we can integrate by parts:
Let u = ln(x) and dv = 1/x^3 dx, then du = 1/x dx and v = -1/(2x^2)
Using the formula for integration by parts, we get:
\(\int [\infty,17] ln(x)/x^3 dx = [-ln(x)/(2x^2)] [\infty,17] + ∫[\infty,17] 1/(x^2 \times 2x) dx\)
The first term evaluates to:
-lim x→∞ \(ln(x)/(2x^2) + ln(17)/(217^2) = 0 + ln(17)/(217^2)\)
The second term simplifies to:
\(\int [\infty,17] 1/(x^3 \times 2) dx = [-1/(4x^2)] [\infty,17] = 1/(4\times 17^2)\)
Adding the two terms, we get:
\(\int [\infty,17] ln(x)/x^3 dx = ln(17)/(217^2) + 1/(417^2)\)
\(\int [\infty,17] ln(x)/x^3 dx \approx 0.000198\)
Now, we can use the Integral Test to determine whether the series
\(\sum n=17^{[\infty]} ln(n)/n^3\)
converges or diverges.
Since the function\(f(x) = ln(x)/x^3\) is continuous, positive, and decreasing for x > 17, we can apply the Integral Test:
\(\int [n,\infty] ln(x)/x^3 dx ≤ \sum k=n^{[\infty]} ln(k)/k^3 ≤ ln(n)/n^3 + \int [n,\infty] ln(x)/x^3 dx\)
By the comparison we have just shown, the improper integral \(\int [\infty,17] ln(x)/x^3 dx\) converges.
Thus, by the Integral Test, the series also converges.
For similar question on convergent.
https://brainly.com/question/31328203
#SPJ11
Using the Integral Test, we can now determine whether the series ∑(from n=1 to infinity) (ln(n)/n^3) converges. Since the improper integral of the same function converges and the function is positive, continuous, and decreasing, the series also converges.
To determine whether the improper integral ∫[infinity]17ln(x)x3dx converges or diverges, we can use the integral test. Let's first find the antiderivative of ln(x):
∫ln(x)dx = xln(x) - x + C
Now, we can use integration by parts with u = ln(x) and dv = x^3dx:
∫ln(x)x^3dx = x^3ln(x) - ∫x^2dx
= x^3ln(x) - (1/3)x^3 + C
Now, we can evaluate the improper integral:
∫[infinity]17ln(x)x^3dx = lim as b->infinity [∫b17ln(x)x^3dx]
= lim as b->infinity [(b^3ln(b) - (1/3)b^3) - (17^3ln(17) - (1/3)17^3)]
= infinity
Since the improper integral diverges, we can conclude that the series [infinity]∑n=17ln(n)n^3 also diverges by the integral test.
Therefore, the improper integral ∫[infinity]17ln(x)x^3dx diverges and the series [infinity]∑n=17ln(n)n^3 also diverges.
To determine whether the improper integral ∫(from 1 to infinity) (ln(x)/x^3) dx converges or diverges, we can use integration by parts. Let u = ln(x) and dv = 1/x^3 dx. Then, du = (1/x) dx and v = -1/(2x^2).
Now, integrate by parts:
∫(ln(x)/x^3) dx = uv - ∫(v*du)
= (-ln(x)/(2x^2)) - ∫(-1/(2x^3) dx)
= (-ln(x)/(2x^2)) + (1/(4x^2)) evaluated from 1 to infinity.
As x approaches infinity, both terms in the sum approach 0:
(-ln(x)/(2x^2)) -> 0 and (1/(4x^2)) -> 0.
Thus, the improper integral converges, and its value is:
((-ln(x)/(2x^2)) + (1/(4x^2))) evaluated from 1 to infinity
= (0 + 0) - ((-ln(1)/(2*1^2)) + (1/(4*1^2)))
= 1/4.
Using the Integral Test, we can now determine whether the series ∑(from n=1 to infinity) (ln(n)/n^3) converges. Since the improper integral of the same function converges and the function is positive, continuous, and decreasing, the series also converges.
Learn more about integral at: brainly.com/question/18125359
#SPJ11
(16-8x-7x^2+2x^3)/(x-4)
Answers
The division of 16 - 8x - 7x² + 2x³ by x - 4 will have a quotient of 2x² + x - 4 and a remainder of 0 using synthetic division.
Dividing with synthetic divisionThe procedure for synthetic division involves the following steps:
Divide.
Multiply.
Subtract.
Bring down the next term, and
Repeat the process to get zero or arrive at a remainder.
We shall rearrange 16 - 8x - 7x² + 2x³ to become 2x³ - 7x² - 8x + 16 and then divide by x - 4 as follows;
2x³ divided by x equals 2x²
x - 4 multiplied by 2x² equals 2x³ - 8x²
subtract 2x³ - 8x² from 2x³ - 7x² - 8x + 16 will give x² - 8x
x² divided by x equals x
x - 4 multiplied by x equals x² - 4x
subtract x² - 4x from x² - 8x will give us -4x + 16
-4x divided by x equals -4
x - 4 multiplied by -4 equals -4x + 16
subtract -4x + 16 from -4x + 16 will result to a remainder of 0
Therefore by synthetic division, 16 - 8x - 7x² + 2x³ divided by x - 4 is equal to the quotient of 2x² + x - 4 with a remainder of 0.
Learn more about synthetic division here: https://brainly.com/question/25289437
#SPJ1
Suppose the student population of Algebra City was 3,845, 721 in 2015. The population was predicted to increase 1.18% each year for the next 25
years. What will the student population of Algebra City be in the year 2025?
3,891, 342 students
4,324,386 students
5, 156, 376 students
4,537,950 students
Answers
Answer:
4,324,386 students
Step-by-step explanation:
Student population in 2015 = 3,845,721
Percentage of students population in 2015 = 100%
Percentage increase of students each year = 1.18%
What will the student population of Algebra City be in the year 2025?
2025 population = 3,845,721(100% + 1.18%)^t
Where
t = number of years
= 2025 - 2015
= 10 years
2025 population = 3,845,721(100% + 1.18%)^t
= 3,845,721(1 + 0.118)^10
= 3,845,721(1.0118)^10
= 3,845,721(1.1244670934955)
= 4,324,386.7152646077555
Approximately,
= 4,324,386 students
30 percent of what number is 15
Answers
Step-by-step explanation:
50
because 50*(30/100)=1500/100
then it gives 15
so the 30 percent of a number is 15 that number is 50.
The requried, 30 percent of 50 is 15, as of the given condition.
What is the percentage?The percentage is the ratio of the composition of matter to the overall composition of matter multiplied by 100.
We can use algebra to solve the problem.
Let's call the number we're looking for "x". Then we can translate the problem statement into an equation:
30% of x is 15:
0.3x = 15
To solve for x, we can divide both sides of the equation by 0.3:
x = 15 ÷ 0.3
x = 50
Therefore, 30 percent of 50 is 15.
Learn more about percentages here:
brainly.com/question/13450942
#SPJ2
3(x+2y+5z) ?????
??????????????????????????????????????????????????????????????????????
Answers
Answer:
3x + 6y + 15z
Step-by-step explanation:
Use distributive property:
(3*x) + (3*2y) + (3*5z)
(3x) + (6y) + (15z)
3x + 6y + 15z
Hope this helped :)
a direct mail company wishes to estimate the proportion of people on a large mailing list that will purchase a product. suppose the true proportion is 0.06. if 235 are sampled, what is the probability that the sample proportion will differ from the population proportion by more than 0.03? round your answer to four decimal places.
Answers
The probability that the sample proportion will differ from the population proportion by more than 0.03 is 0.9476
To determine the probability
The given parameters are:
True proportion and the mean (p) = 0.06
The samples size (n) = 235
Start by calculating the standard deviation using
\(\sigma = \sqrt{\frac{p(1-p)}{n} }\)
This gives
\(\sigma = \sqrt{\frac{0.06\;*\;(1-0.06)}{235} }\)
\(\sigma = \sqrt{\frac{0.06\;*\;(0.94)}{235} }\)
\(\sigma = \sqrt{\frac{0.0564}{235} }\)
\(\sigma = \sqrt{0.00024 }\)
\(\sigma = 0.01549\)
Next, we calculate the x values
\(x = x_{1} \pm x_{2}\)
where x₁ = 0.06 and x₂ = 0.03
x = x₁ + x₂ , x₁ - x₂
x = 0.06 + 0.03, 0.06 - 0.03
x = 0.09, 0.03
Calculate the z score for both x values
\(z = \frac{x\; - \; \mu}{\sigma}\)
\(z = \frac{0.09\; - \; 0.06}{0.01549}, \frac{0.03\; - \; 0.06}{0.01549}\)
\(z = \frac{0.03}{0.01549}, \frac{-0.03}{0.01549}\)
\(z = 1.94, -1.94\)
The p values at the z scores are:
p = 0.9738, 0.0262
Note: refer to z tables to find the p values
Calculate the difference
p = 0.9738 - 0.0262
p = 0.9476
Hence, the probability that the sample proportion will differ from the population proportion by less than 0.03 is 0.9476
Visit the link below to learn more about probabilities:
brainly.com/question/25870256
#SPJ4
Select the expression that has a value of 6.
2,400 ÷ 40
2,400 ÷ 4
240 ÷ 40
240 ÷ 4
Answers
Answer:
240÷40 is your answer :)
2400/40 = 60
2400/4 = 600
240/4 = 60
Use the chebyshev bound developed to prove the law of large numbers to bound the probability that the total numbe rof dots i ebtween 300 and 400
Answers
The Chebyshev bound can be used to provide an upper bound on the probability that the total number of dots lies between 300 and 400.
The Chebyshev bound states that for any random variable with a finite mean and variance, the probability that the random variable deviates from its mean by more than a certain number of standard deviations is bounded. In this case, we can use the Chebyshev bound to estimate the probability that the total number of dots deviates from its mean by more than a certain amount.
To apply the Chebyshev bound, we need to know the mean and variance of the total number of dots. Once we have these values, we can calculate the standard deviation and determine the number of standard deviations that correspond to the interval between 300 and 400.
By using the Chebyshev bound, we can provide an upper bound on the probability that the total number of dots lies between 300 and 400. The bound becomes tighter as the sample size increases, and it approaches the actual probability as the sample size approaches infinity. Therefore, the Law of Large Numbers is indirectly invoked in using the Chebyshev bound to estimate the probability.
Learn more about probability here:
https://brainly.com/question/32117953
#SPJ11
I wish to accumulate $100 000 over 20 years at 10% p.a. compounded annually. What should be the amount of my annual payments?
Answers
To accumulate $100,000 over 20 years at a 10% annual interest rate compound annually, you would need to make annual payments of $8,218.64.
You can use the formula for the future value of an annuity to accumulate $100,000 over 20 years at a 10% annual interest rate compound on a yearly basis:
\(FV = Pmt * [(1 + r)^n - 1] / r\)
Where:
FV is future value, which is $100,000 in that case
Pmt: annual payment
r: annual interest rate, which is 10%
n: number of payment periods, which is 20
By plugging in values:
\($100,000 = Pmt * [(1 + 0.1)^(20) - 1] / 0.1\)
Solving for Pmt, we get:
\(Pmt = $100,000 / [(1 + 0.1)^(20) - 1] / 0.1\\Pmt = $100,000 / 12.167\\Pmt = $8,218.64\)
Learn more about compound here:
https://brainly.com/question/24095823
#SPJ1
pls answer i'll mark u brainlist
a plane take 5/3 hours to cover a distance flying at 340 km per hour.find the time taken if the speed is increased to 850 km per hour
Answers
Answer: 2/3 hours
Step-by-step explanation:
okay so the speed now is 850, which is 850/340 = 2.5 times the original speed
Since It's 2.5 times the original speed,
2.5x = 5/3
so x = 5/3/2.5 = 2/3
Answer:
2/3 of an hour
Step-by-step explanation:
Here is the information that the question gives us.
A plane covers a distance in 5/3 of an hour at 340 km per hour. How long would it take for a plane to cover the same distance while traveling at 850 km per hour?
Remember, distance = speed * time. Using that formula, we can find the distance with this equation:
distance = 340 * 5/3
Multiply.
distance = 1700/3 km
Then, we can plug this distance in to find the time it would take for a plane going at 850 km/hour.
distance = speed * time
1700/3 = 850 * time
Divide both sides by 850
(1700/850) / 3 = time
time = 2/3 hour
Thus, the answer is 2/3 of an hour.
Just in case, let's check the answer. Because both the planes travel the same distance, then their speeds * time should both equal "distance". In other words, they should be equal.
340 * 5/3 = 2/3 * 850
Simplify.
1700/3 = 1700/3
So, the answer is correct.
I hope this helps! Feel free to ask any questions!
a little help pls this old i don't remember any of it

Answers
Answer:
Bowling ball
Step-by-step explanation:
A bowling ball has much more mass than all three of the other choices and the amount of kinetic energy is determined based on a object's mass and velocity, but in this case, all 4 balls are traveling at the same velocity. Therefore, we know that the bowling ball has much more mass so therefore it has the most kinetic energy.
PLEASE HELP ASAP!!!
A video screen is 16 in. by 12 in. tall. What is the width of the largest complete image possible for a photograph that is 2 in. wide by 3 in. tall?
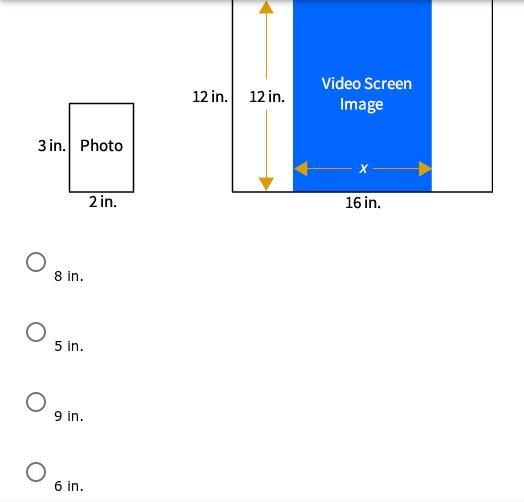
Answers
Answer:
8
Step-by-step explanation:
12 ÷ 3 = 4
4 × 2 = 8
:) hope this helps
Answer:
The answer is is 8 inches.