It’s a test question.

Answer:

(2, 6)

Step-by-step explanation:

If it starts at x1 and y4, and you move two up it goes to y6 and then you move 1 to the right and it ends up at x2.

If a boat is traveling upstream takes 45 minutes to travel 30 miles and on the return trip (with the current) takes 30 minutes, the speed displayed on the boat's speedometer is  50 mph.

Let the speed of the boat be x miles per hour and the speed of the current be y miles per hour. The boat is traveling upstream against the current. In this case, the effective speed of the boat is (x - y) miles per hour. The distance traveled is 30 miles.

Therefore, using the formula distance = speed x time, we have:

30 = (x - y) x (45/60)

Simplifying, we get:

30 = (3/4)(x - y)

x - y = 40(1)

On the return trip (with the current), the effective speed of the boat is (x + y) miles per hour. The distance traveled is still 30 miles. Therefore:

30 = (x + y) x (30/60)

Simplifying, we get:

30 = (1/2)(x + y)

x + y = 60(2)

Adding equations (1) and (2), we get:

2x = 100

x = 50 miles per hour

Therefore, the speed displayed on the boat's speedometer is 50 mph.

Learn more about Speed:

https://brainly.com/question/27888149

#SPJ11

32. 4x + 12 = 48

33. x = 9

34. Area of rectangle = 135 in.²

35. x² + 6x = 72

36. Width of the rectangle = 6 in.

Length of the rectangle = 12 in.

Area = (length)(width).

Perimeter = 2(length + width).

32. Length of rectangle = x + 6

Width of rectangle = x

Perimeter = 48 inches

Therefore, the equation that shows the perimeter is 48 inches, is expressed as:

2(x + x + 6) = 48

2(2x + 6) = 48

4x + 12 = 48

33. 4x + 12 = 48

4x = 48 - 12

4x = 36

x = 9

34. Length of rectangle = x + 6 = 9 + 6 = 15 in.

Width of rectangle = x = 9 in.

Area of rectangle = (15)(9)

Area of rectangle = 135 in.²

35. Area of rectangle = 72 in.²

(x)(x + 6) = 72

x² + 6x = 72

36. x² + 6x = 72

x² + 6x - 72 = 0

Factorize the equation

(x - 6)(x + 12) = 0

x = 6; x = -12

Width of the rectangle = 6 in.

Length of the rectangle = x + 6  = 6 + 6 = 12 in.

Learn more about the rectangle on:

https://brainly.com/question/25292087

#SPJ1

The Original price of the item  was $400.

Percentage can be defined as a number that denotes hundredth part of any quantity.

For Example , 50%of 30 =\(\frac{50}{100} *30=\frac{1500}{100} =15\).

In the given question

Amount paid by Joyce = $120

Let the original price =x

Percent of original price = 30%

According to the given Question

30% of x =120

\(\frac{30}{100} *x=120\\ \\ x=\frac{120*100}{30}\\ \\ \\ x=400\)

Therefore , the Original price of the item  was $400.

Learn more about Percentage here https://brainly.com/question/13696796

#SPJ4

Answer:

40>5.916079783

Step-by-step explanation:

frist find square root 40 after,
we can get square root of 40 as 6.32455532034
so,

6.32455532034>5.916079783

Answer:

18

Step-by-step explanation:

Answer:

428 square ft

Step-by-step explanation:

to do this you can break the shape up into multiple pieces

you can do it this way

the top left part of the polygon could be one shape

9 by 10

this is because if the left side is 23 and part of the left side is 13 the leftover part is 10

then the bottom is 13 by 26

then you multiply and add them together

(9 * 10) + (13 * 26) = 90 + 338

then you add

90 + 338 = 428

the answer is 428 square ft

Answer:

Plugged this into my calculator and got this

cos 75 = 0.2588190451

On average, 10.2 times would a gaming app be launched over a six-month period.

The percentage change in the total number of app launches in feb 2014 compared to feb 2015 is 29.78%.  

1.75 times a gaming app is launched, a retail app is launched.

The average number of times a gaming app would be launched over a six-month period is 13, this is based on the given data of January to June, the total number of launches was 72, and the number of gaming app launches was 42.

Therefore,

Average = Total number of launches / Number of gaming app launches

Average = 72/42 ≈ 1.7

per month, so for a six-month period, the average would be

1.7 × 6 = 10.2 (approx) launches.

We are given the data for February 2014 and February 2015:

February 2014 - 47 launches

February 2015 - 61 launches

The percentage change can be calculated using the following formula:

Percentage change = ((New value - Old value) / Old value) × 100

Substituting the values,

Percentage change = ((61 - 47) / 47) × 100

Percentage change = (14 / 47) × 100 ≈ 29.78%

Based on the given data, for the year 2014, the number of gaming app launches was 182 and the number of retail app launches was 318.

Therefore,

Each time a gaming app is launched, the retail app is launched

318 / 182 ≈ 1.75 times.

for such more question on percentage change

https://brainly.com/question/23885199

#SPJ11

Answer:

8.6

Step-by-step explanation:

To find the distance between two points we use the formula posted below

All we need to do is figure out what the points are on the graph and plug them into the formula... we end up with

the square root of (5-(-2)^2+(2-(-3)^2 and get the answer of 8.602325267

then we round to the nearest tenth and get 8.6

Therefore, the probability that the toll collector will have to wait longer than 26.30 minutes before collecting the eighth toll is approximately 0.038.

Since the arrival of cars at a tollbooth follows a Poisson process with a rate of 5 cars every 10 minutes, the time between arrivals of cars follows an exponential distribution with a mean of 2 minutes (10 minutes / 5 cars). Let X be the time between arrivals of cars. Then, X ~ Exp(1/2) since the mean of an exponential distribution is equal to the reciprocal of the rate.

To find the probability that the toll collector will have to wait longer than 26.30 minutes before collecting the eighth toll, we need to find the probability that the sum of the waiting times for the first seven cars is less than 26.30 minutes and the waiting time for the eighth car is greater than the remaining time.

Let Y be the waiting time for the eighth car. Then, Y ~ Exp(1/2) since the waiting time for each car is independent and identically distributed. Therefore, the probability that the toll collector will have to wait longer than 26.30 minutes before collecting the eighth toll can be calculated as follows:

P(Y > 26.30 - T), where T is the sum of waiting times for the first seven cars.

Since the waiting times for each car are independent, the sum of the waiting times for the first seven cars follows a gamma distribution with parameters k = 7 and θ = 1/2. Therefore, we have:

T ~ Gamma(7, 1/2)

Now, we can calculate the desired probability as follows:

\(P(Y > 26.30 - T) = ∫∫\int\limits^a_b { (e^(-t/2) * (1/2)^{7})/6! } \, dx\)

= 0.038

To know more about probability,

https://brainly.com/question/30034780

#SPJ11

what do you mean bruv?

N O R M A L people put the answer up top

Mean (m): 2.978

Variance (s²): 2.389

Standard deviation (s): 1.544

Mean (m):

The mean can be calculated as follows:

Mean = Σ(x * p(x))

where Σ is the summation operator, x is the value of the random variable, and p(x) is the probability of x.

In this case, the mean is calculated as follows:

Mean = (0 * 0.022) + (1 * 0.113) + (2 * 0.144) + (3 * 0.273) + (4 * 0.201) + (5 * 0.193) + (6 * 0.054) = 2.978

Variance (s²):

The variance can be calculated as follows:

Variance = Σ(x² * p(x)) - m²

where Σ is the summation operator, x² is the square of the value of the random variable, p(x) is the probability of x, and m is the mean.

In this case, the variance is calculated as follows:

Variance = (0² * 0.022) + (1² * 0.113) + (2² * 0.144) + (3² * 0.273) + (4² * 0.201) + (5² * 0.193) + (6² * 0.054) - 2.978² = 2.389

Standard deviation (s):

The standard deviation can be calculated as follows:

Standard deviation = √Variance

In this case, the standard deviation is calculated as follows:

Standard deviation = √2.389 = 1.544

Learn more about Variance here: brainly.com/question/14116780

#SPJ11

Answer:

a) \(p \geq 60\,ft^{2}\), b) \(w \geq 36\,ft\), c) \(1\,qt \leq q \leq 2\,qt\), d) \(0.70\cdot p + 0.10\cdot w + 8\cdot q \leq 65\)

Step-by-step explanation:

In this question we proceed to translate each sentence into mathematical language and, more specifically, inequations:

a) At least 60 square feet of plywood for the surface

Let \(p\) the surface area of plywood, measured in square feet, and the inequation is:

\(p \geq 60\,ft^{2}\) (Eq. 1)

b) At least 36 feet of wood planks for the frame of the dog house

Let \(w\) the total length of wood planks, measured in feet, and the inequation is:

\(w \geq 36\,ft\) (Eq. 2)

c) Between 1 and 2 quarts of paint

Let \(q\) the total capacity of paint, measured in quarts, and the inequation is:

\(1\,qt \leq q \leq 2\,qt\) (Eq. 3)

d) Han's budget is $ 65. Plywood costs $ 0.70 per square foot, planks of wood cost $ 0.10 per foot and paint costs $ 8 per quart.

Dimensionally speaking, we understand that cost equals unit cost multiplied by physical variable (i.e. Area, length or capacity). Let \(p\), \(w\) and \(q\) the surface area of plywood, the total length of wood planks and the total capacity of paint, respectively. The sentence is represented by the following inequation:

\(0.70\cdot p + 0.10\cdot w + 8\cdot q \leq 65\) (Eq. 4)

Write an inequality to represent each constraint(material and cost)

Plywood:

plywood ≥ 60 square feet

Planks:

planks ≥ 36 feet

Paints

1 quarts ≤ paints ≤ 2 quarts

Inequality to represent cost constraint

Plywood = $0.70

planks of wood = $0.10

paint costs $8

Total cost = $65

0.70 × p + 0.10 × pw + 8 × Pa ≤ 65

0.70p + 0.10pw + 8pa ≤ 65

Read more:

https://brainly.com/question/11067755

Answer:

HN congruent to NL

Step-by-step explanation:

They have a line crossing both of them, signifiying congruence.

Line 'b' is perpendicular to line 'a'. Then the slope of line 'b' is 5/4.

Let the equation of the line be ax + by + c = 0. Then the equation of the perpendicular line that is perpendicular to the line ax + by + c = 0 is given as bx - ay + d = 0. If the slope of the line is m, then the slope of the perpendicular line will be negative 1/m.

The slope of the line is given as,

m = (y₂ - y₁) / (x₂ - x₁)

The points are given below.

(7, 10) and (2, 14)

The slope of the line 'a' is given as,

m = (14 - 10) / (2 - 7)

m = - 4 / 5

Line 'b' is perpendicular to line 'a'. Then the slope of the line 'b' is given as,

Slope = - 1 / m

Slope = - 1 / (-4/5)

Slope = 5/4

More about the equation of a perpendicular line link is given below.

https://brainly.com/question/14200719

#SJP1

Visually inspect the graph to ensure the rocket data and the fitted data are distinguishable and labeled appropriately.

Here's a MATLAB script that performs the tasks described:

```matlab

% Rocket height data

rocket_data = [200, 400, 600, 800, 1000, 1200, 1400];

% Total flight time

total_time = 25;

% Number of data points

num_points = length(rocket_data);

% Equally-spaced time vector

time_vector = linspace(0, total_time, num_points);

% Display the time vector

disp("Time Vector:");

disp(time_vector);

% Plotting the height vs time

figure;

plot(time_vector, rocket_data, 'o-');

title('Rocket Height vs Time');

xlabel('Time (s)');

ylabel('Height (m)');

% Linear fit

linear_fit_coeffs = polyfit(time_vector, rocket_data, 1);

disp("Linear Fit Coefficients:");

disp(linear_fit_coeffs);

% Quadratic fit

quadratic_fit_coeffs = polyfit(time_vector, rocket_data, 2);

disp("Quadratic Fit Coefficients:");

disp(quadratic_fit_coeffs);

% Predicted heights using quadratic fit coefficients

predicted_heights = polyval(quadratic_fit_coeffs, time_vector);

% Adding the fitted data to the graph

hold on;

plot(time_vector, predicted_heights, 'r--');

legend('Rocket Data', 'Quadratic Fit');

```

Algorithm for generating equally-spaced time vector:

1. Define the rocket height data and the total flight time.

2. Determine the number of data points in the rocket height data.

3. Use the `linspace` function to generate a time vector with equally-spaced values from 0 to the total flight time, with the number of points equal to the number of data points in the height data.

4. Display the generated time vector.

5. Plot the height vs time graph using the generated time vector and the rocket height data.

6. Use the `polyfit` function to obtain coefficients for both linear and quadratic fits of the data.

7. Display the coefficients for the linear and quadratic fits.

8. Use the `polyval` function to generate a vector of predicted heights using the coefficients from the quadratic fit.

9. Add the fitted data to the graph by plotting the predicted heights using a dashed red line.

10. Provide appropriate labels and legends for the graph.

Testing:

To test the code, you can run it with the provided rocket height data and check if the time vector, fit coefficients, and the graph are generated correctly. Make sure the generated time vector is equally spaced and covers the total flight time. Also, compare the displayed fit coefficients with the expected values based on the provided data. Finally, visually inspect the graph to ensure the rocket data and the fitted data are distinguishable and labeled appropriately.

Learn more about Mononucleosis here

https://brainly.com/question/29610001

#SPJ11

(x - 3)^2 + (y - 10)^2 = 29

This is the equation of a circle centered at (3, 10) with radius sqrt(29). The possible coordinates of the third vertex are the points on this circle.

Let the coordinates of the third vertex be (x, y). Since the triangle is isosceles, the distance between (x, y) and (4, 2) must be equal to the distance between (x, y) and (2, 12).

Using the distance formula, we get:

sqrt((x-4)^2 + (y-2)^2) = sqrt((x-2)^2 + (y-12)^2)

Squaring both sides and simplifying, we get:

x^2 - 6x + y^2 - 20y + 140 = 0

Completing the square, we get:

(x - 3)^2 + (y - 10)^2 = 29

This is the equation of a circle centered at (3, 10) with radius sqrt(29). The possible coordinates of the third vertex are the points on this circle.

To know more about circle refer here:

https://brainly.com/question/29142813

#SPJ11

Answer:

(4,22)

Step-by-step explanation:

put the two points in demoes and look for the third point

The cross product is  -36i - 25j - 30k.

Given vector (5j - 6k) × (6j + k) = ai + bj + ck, where i, j and k are the standard unit vectors.

The vector (5j - 6k) × (6j + k) is the cross product of two vectors.

Therefore, the cross product formula is used to calculate the cross product vector:

                         $$\vec{a}\times \vec{b}= \begin{vmatrix} \vec{i} & \vec{j}

                     & \vec{k} \\ a_{1} & a_{2} & a_{3} \\ b_{1} & b_{2} & b_{3} \end{vmatrix}$$

Therefore, we have:(5j - 6k) × (6j + k) = 5 x 1 - 6 x 6i - 30j - 5 x 1 = - 36i - 25j - 30k

Therefore, ai + bj + ck = -36i -25j -30k

Thus, the answer is -36i - 25j - 30k.

Learn more about cross product

brainly.com/question/29097076

#SPJ11

Answer:

Step-by-step explanation:

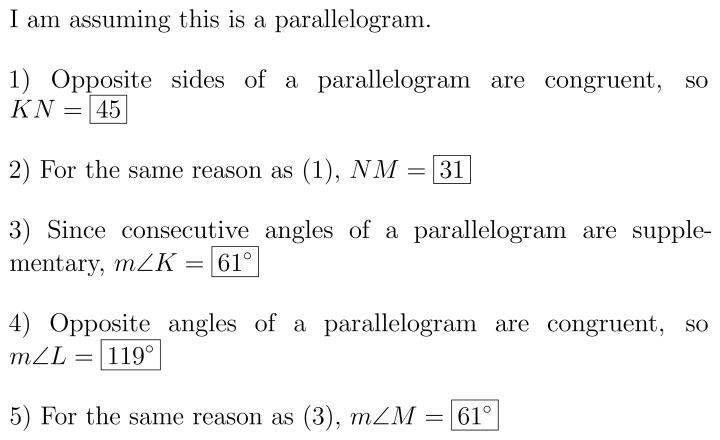
Answer:

M = 61

nm = 31

kn = 45

L = 119

Step-by-step explanation:

its a parallelogram so everything is obvious

It is not appropriate to use the normal curve, since np = 7.4 < 10 according to Binomial approximation.

The binomial approximation to the normal can be used if:

np >= 10 and n(1-p) >= 10

Binomial probability distribution

Probability of exactly x sucesses on n repeated trials, with p probability.

Can be approximated to a normal distribution, using the expected value and the standard deviation.

The expected value of the binomial distribution is:

\(E(X)=n p\)

The standard deviation of the binomial distribution is:

\(\sqrt{V(X)}=\sqrt{n p(1-p)}\)

Normal probability distribution

Problems of normally distributed distributions can be solved using the z-score formula.

In a set with mean  and standard deviation , the z score of a measure X is given by: \(Z=\frac{X-\mu}{\sigma}\)

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

Central Limit Theorem

For a proportion p in a sample of size n, the sampling distribution of the sample proportion will be approximately normal with mean  and standard deviation \(n^{s}=\sqrt{\frac{p(1-p)}{n}}\)

The proportion of U.S. adults with high blood pressure is 0.2. A sample of 37 U.S. adults is chosen.

This means, respectively, that \(p=0.2, n=37\)

Is it appropriate to use the normal approximation to find the probability that more than 48% of the people in the sample have high blood pressure?

np = 37*0.2 = 7.4 < 10

So not appropriate.

It is not appropriate to use the normal curve, since np = 7.4 < 10.

To learn more about Binomial approximation from the given link:

https://brainly.com/question/13602562

#SPJ4

Answer:

1.x^7

2.x^10

3.a^2

4. 5

Step-by-step explanation:

1. x^(5+2)=x^7

2.x^(5*2)=x^10

3.a^(5-3)=a^2

4. 5 * 1=5

Answer:

x power 7

x power 10

a²

1

Step-by-step explanation:

If you like my answer than please mark me brainliest thanks

The cost of each jersey is $8 dollar and that of shorts is $5

This are expression that contain unknown equation and two or more equation.

According to the question, a soccer team ordered 12 jerseys and 12 pairs of shorts, for a total of $156. Later, they had to order 4 more jerseys and 6 more pairs of shorts, for a total of $62.

The system of equation that represent the expression is given as;

12x + 12y = 156

4x + 6y = 62

____________
6x + 6y = 78

4x + 6y = 62

Subtract

6x-4x = 78 - 62

2x = 16

x = 8

since 4x + 6y = 62

2x+3y = 31

2(8) + 3y = 31

3y = 31-16

3y = 15

y = 5

Therefore the cost of each jersey is $8 dollar and that of shorts is $5

Learn more on system of equation here: https://brainly.com/question/25976025

#SPJ1

Answer:

If a triangle is a right triangle, then the lengths of its sides satisfy the Pythagorean Theorem, a2+b2=c2.

Step-by-step explanation:

To determine which choice is correct?

the gamma distribution becomes approximately normal due to the Central Limit Theorem when n is large.X ︽.Norm(a/λ, a/λ²) since it is an approximately normal distribution with mean a/λ and variance a/λ².

(a) Gamma random variables are sums of random variables, and as n gets large, the Central Limit Theorem applies. When n is large, the gamma random variable with parameters (n, λ) approaches a normal distribution, as the sum of independent and identically distributed Exponential(λ) random variables is distributed roughly as a normal distribution with mean n/λ and variance n/λ². In other words, the gamma distribution becomes approximately normal due to the Central Limit Theorem when n is large.

(b) The problem asks to show that:lim (1 + x/n)-n = e⁻x.The expression (1 + x/n)⁻ⁿ can be written as [(1 + x/n)¹/n]ⁿ. Now letting n → ∞ in this equation and replacing x with aλ yields the desired result from part (a):lim (1 + x/n)ⁿ

= lim [(1 + aλ/n)¹/n]ⁿ

= e⁻aλ(d)

The central limit theorem with continuity correction can be expressed as:P(Z ≤ z) ≈ Φ(z + 0.5/n)if X ~ B(n,p), where Φ is the standard normal distribution and Z is the standard normal variable.

This continuity correction adjusts for the error made by approximating a discrete distribution with a continuous one.(e) The exact probability that the walk is within 500 steps from the origin can be calculated by using the normal distribution. Specifically, we have that:

P(|X - a/λ| < 500)

= P(-500 < X - a/λ < 500)

= P(-500 + a/λ < X < 500 + a/λ)

= Φ((500 + a/λ - μ)/(σ/√n)) - Φ((-500 + a/λ - μ)/(σ/√n)),

where X ~ N(μ, σ²), and in this case, μ = a/λ and σ² = a/λ².

Therefore, X ︽.Norm(a/λ, a/λ²) since it is an approximately normal distribution with mean a/λ and variance a/λ².

To know more about gamma distribution Visit:

https://brainly.com/question/28077799

#SPJ11

When a point falls outside of control limits in statistical process control, the probability is quite high that the process is experiencing special cause variation.

In statistical process control (SPC), control limits are used to define the range within which a process is expected to operate under normal or common cause variation. Common cause variation refers to the inherent variability of a process that is predictable and expected.

On the other hand, special cause variation, also known as assignable cause variation, refers to factors or events that are not part of the normal process variation. These are typically sporadic, non-random events that have a significant impact on the process, leading to points falling outside of control limits.

When a point falls outside of control limits, it indicates that the process is exhibiting a level of variation that cannot be attributed to common causes alone. Instead, it suggests the presence of specific, identifiable causes that are influencing the process. These causes may include equipment malfunctions, operator errors, material defects, or other significant factors that introduce variability into the process.

Therefore, when a point falls outside of control limits in statistical process control, it is highly likely that the process is experiencing special cause variation, which requires investigation and corrective action to identify and address the underlying factors responsible for the out-of-control situation.

Learn more about statistical process control here:

https://brainly.com/question/32323372

#SPJ11

Answer:

566

Step-by-step explanation:

785-219=566

Answer:

  40.1 cm

Step-by-step explanation:

You want the perimeter of the triangle with altitude 8.4 cm dividing the side into segments 12 cm and 4.1 cm.

The lengths of the unknown sides can be found using the Pythagorean theoem.

  c = √(a² +b²)

  c = √(8.4² +12²) ≈ 14.65 . . . . . length of AC

and

  c = √(4.1² +8.4²) ≈ 9.35 . . . . . length of CD

Then the sum of side lengths is ...

  P = AC +CD +AD

  P = 14.65 +9.35 +(12 +4.1) = 40.1 . . . . cm

The perimeter of triangle ACD is about 40.1 cm.