Answers
Answer:
2x + 1y = 5
Step-by-step explanation:
You don't need to type the 1 because all ones are invisible in algebra.
Related Questions
Help help help please

Answers
Hey there! :)
Answer:
x = -7/12.
Step-by-step explanation:
Given:
\(x + \frac{5}{12} = -\frac{1}{6}\)
Create a common denominator for each term:
\(\frac{12x}{12} + \frac{5}{12} = -\frac{2}{12}\)
Disregard the denominator:
12x + 5 = -2
Subtract 5 from both sides:
12x = -7
Divide both sides by 12:
x = -7/12.
1. What is it called when the expenses and the revenue are equal, so there is no profit or loss ?
2. A company has determined that its expense and revenue functions are
E=- 1040p + 91,520 and R = -260? + 15,600p - 108,160.
At which price points can the company expect to break even?
Answers
2. To find the break-even point, we need to set the expense and revenue functions equal to each other and solve for p:
- 1040p + 91,520 = -260p + 15,600p - 108,160
- 1040p + 91,520 = 14,340p - 108,160
- 15,380p = -16,640
p = 1.08
Therefore, the company can expect to break even at a price point of $1.08 per unit.
Please answer correctly !!!!!! Will mark brainliest !!!!!!!!!!!

Answers
Answer:
I answered this already for you: https://brainly.com/question/16790720
for brainiest:):):):):):):)

Answers
Answer:
1. 16(pi)
2. 20.25(pi)
3. 30
4. 4
Step-by-step explanation:
1.
The formula for the are of a circle is (\((pi)(r)^2\)) where (pi) represents (3.1415), and (r) represents the radius or the distance from the center to the outer part of the circle. Substitute in the given values and solve,
\((pi)(4)^2\\\\16(pi)\)
2.
One can use a similar technique to find the area of the circle in the second problem. Only that the diameter of this circle is given. By its definition, the radius is always half of the diameter, thus one can substitute in the values and solve:
\((pi)(\frac{9}{2})^2\\\\(pi)(4.5)^2\\\\(pi)(20.25)\)
3.
When working with scaled representations versus their real counterpart, to go from the scaled representation to the real counterpart, one must multiply by the scale factor.
(6)(5)
= 30
4.
When one would like to go from the real subject to the scaled representation, one will divide by the given scale factor.
(20)/(5)
= 4
Please answer it now in two minutes

Answers
Answer:
59.0
Step-by-step explanation:
Given a right angled triangle, ∆XYZ, to know which trigonometric ratio formula to apply in finding the measure of angle X, note the following:
Opposite side to angle X = 6
Hypotenuse = 7
Therefore, we would apply the following trigonometric ratio formula to solve for m<X:
\( sin X = \frac{6}{7} \)
\( sin X = 0.8571 \)
\( X = sin^{-1}(0.8571) \)
\( X = 58.99 \)
\( m < X = 59.0 \) (rounded to nearest tenth)
If two box packers can pack two boxes in two minutes, how many packers will it take to pack 18 boxes in six minutes? Please provide answer in numeric form only. < Previous Next >
Answers
The number of Packers required to do the job in 18 minutes wouid be 3
Interpreting the questionIf two box packers can pack two boxes in two minutes, then each packer can pack one box per minute.
To pack 18 boxes in six minutes, we need 18/6=3 packers.
The problem can be expressed mathematically thus;
Number of packers = (Number of boxes / Time per box) / (Number of boxes per packer)
= (18 / 6) / 1
= 3
Therefore, the number of Packers required is 3
Learn more on word problems: https://brainly.com/question/25693822
#SPJ4
4. Which shows that these two rectangles are similar?

Answers
PLEASE HELP!!!! I don’t understand this topic please can someone help explain how to work out the attached question on similar area. Will mark brainliest!

Answers
Step-by-step explanation:
Look, you're taking these kinds of questions too serious and that's why you believe they're hard, now pay close attention:
the areas are defined in cm² but the radius is defined in cm, so you need find the positive root of cm².
\( \sqrt{ \frac{5500 {cm}^{2} }{220 {cm}^{2} } } = \frac{r}{5cm} = = > \\ 5 = \frac{r}{5} = = = > r = 25\)
and the question wants the base area:
\(s = \pi {r}^{2} = = = > s = 3.14 \times {(25cm)}^{2} = = > 1963.5 {cm}^{2} \)
Suppose that there are two assets that are available for investment and an investor has the following expected utility:
EU = E(Rp) − 0.5A(\sigma)2p
where expected return and standard deviation are expressed in decimals. For example, if expected return is 25%, standard deviation is 15%, and risk aversion is 5, expected utility is computed as:
EU =0.25−0.5*5*0.152 =0.1938
Now, assume that there is no other instrument (such as the risk-free security) available. Then, derive the analytical expressions for the optimal portfolio weights of the first and the second assets for this specific investor. (Hint: We are not talking about a numerical response here. Rather, you are asked to derive mathematically how you would compute for the optimal portfolio.)
Answers
To derive the analytical expressions for the optimal portfolio weights of the first and second assets, we need to maximize the expected utility function.
Let's assume the weights of the first and second assets are denoted by w1 and w2, respectively. Since there is no risk-free security available, the sum of weights must be equal to 1: w1 + w2 = 1.
To find the optimal portfolio weights, we need to maximize the expected utility function with respect to w1 and w2. This can be done using optimization techniques, such as Lagrange multipliers or calculus.
We can start by taking the derivative of the expected utility function with respect to w1 and set it equal to zero to find the critical points. Similarly, we take the derivative with respect to w2 and set it equal to zero.
Next, we solve these equations to find the values of w1 and w2 that satisfy the equations. These values will give us the optimal portfolio weights for the first and second assets.
Ihe analytical expressions for the optimal portfolio weights of the first and second assets can be derived by maximizing the expected utility function and solving the resulting equations.
To know more about weights , visit ;
https://brainly.in/question/9873207
#SPJ11
a host served two pies, one apple and one cherry. dinner guests ate twenty-five percent of the apple pie and sixty percent of the cherry pie. in all, what fraction of the total pies remained? a 23/20 b 3/4 c 23/40 d 3/5
Answers
The fraction of the total pies remained is option (A) 23/20 is the correct answer.
In this question,
A host served two pies, one apple and one cherry.
Dinner guests ate twenty-five percent of the apple pie and sixty percent of the cherry pie.
Apple pie = 25%
∴ Remaining = 100% - 25%
⇒ 75%
⇒ Fraction of apple pie remained is 3/4
Cherry pie = 60%
∴ Remaining = 100% - 60%
⇒ 40%
⇒ Fraction of cherry pie remained is 2/5
Thus fraction of the total pies remained is
⇒ 3/4 + 2/5
⇒ (15+8) / 20
⇒ 23/20
Hence we can conclude that the fraction of the total pies remained is option (A) 23/20 is the correct answer.
Learn more about fraction remained here
https://brainly.com/question/14671411
#SPJ4
Hurry
10 Superscript negative 3 Baseline times 10 times 10 Superscript k Baseline = 10 Superscript negative 3 Baseline = StartFraction 1 Over 10 cubed EndFraction
Negative 3
Negative 1
0
1
Answers
Answer:
-1
Step-by-step explanation:
10^-3 x 10 = 10^-2
But you need it to be 1/10^3
So 10^-2 x 10^-1 = -2 + -1
= 10^-3
In order to put it in simplest form, the power needs to be positive.
So = 1/10^3
Meaning the missing power is -1
-Hope this helps
can I get Brainliest?
Answer: -1
Step-by-step explanation:
Sue need to borrow $8000 to purchase a used car. The dealer arranges with a finance company to lend sue the money at 3.6% per year compounded monthly for 3 years.
What is sue’s monthly payment be?
Plz guys help me pass math
Question about financial application functions
Plz answer this last question on my test
Plz show all the working
God bless you brother or sister
Answer ASAP

Answers
Answer:
The monthly payment is $247.53
Step-by-step explanation:
We need to firstly calculate the total amount to be paid
We have this by using the compound interest formula;
A = I ( 1 + r/n)^nt
A is the total amount to be paid back
I is the amount borrowed which is $8,000
r is the interest rate = 3.6% = 0.036
n is the number of times in a year = 12 (monthly)
t is the number of years = 3
Substituting these values ;
A = 8000(1 + 0.036/12)^(12 * 3)
A = 8000(1 + 0.003)^36
A = 8000(1.003)^36
A = 8,910.94
The monthly payment is this amount divided by the number of months in 3 years which is 36
We have this as;
8,910.94/36 = $247.53
a steep mountain is inclined 80 degrees from the horizontal and rises 3400 vertical feet above the surrounding plain. a cable car is to be installed that will travel from a point 990 ft from the base of the mountain to the top. find the shortest length of cable needed.
Answers
Answer:
3753.20 ft
Step-by-step explanation:
tan 80° = 3400 ft/x
x = 599.51 ft = distance at ground level from point directly below mountain peek to edge of mountain.
599.51 ft + 990 ft = 1589.51 ft = horizontal distance at ground level from where cable is attached to the ground to the center of the mountain. This is a leg of a right triangle.
3400 ft is another leg of a right triangle.
(1589.51 ft)² + (3400 ft)² = y²
The hypotenuse, y, is the length of the cable.
y = 3753.20 ft
Find the area of the region bounded by the graphs of the equations f(x)=-x^(2)+4x and y=0
Answers
The Area of the region bounded by the graphs of the equations f(x) = -x^2 + 4x and y = 0 is 32/3 square units.
The area of the region bounded by the graphs of the equations f(x) = -x^2 + 4x and y = 0, we need to determine the x-values where the two curves intersect. These points will define the boundaries of the region.
Setting the two equations equal to each other, we have:
-x^2 + 4x = 0
Factoring out an x, we get:
x(-x + 4) = 0
This equation is satisfied when either x = 0 or -x + 4 = 0.
Solving -x + 4 = 0, we find:
x = 4
So, the two curves intersect at x = 0 and x = 4.
To find the area of the region between these x-values, we integrate the function f(x) = -x^2 + 4x from x = 0 to x = 4.
∫[-x^2 + 4x] dx from 0 to 4
Integrating, we get:
[-(x^3)/3 + 2x^2] from 0 to 4
Evaluating the definite integral, we have:
[-(4^3)/3 + 2(4^2)] - [-(0^3)/3 + 2(0^2)]
[-64/3 + 32] - [0]
(-64/3 + 32)
Simplifying, we get:
-64/3 + 96/3
32/3
So, the area of the region bounded by the graphs of the equations f(x) = -x^2 + 4x and y = 0 is 32/3 square units.
For more questions on Area .
https://brainly.com/question/2607596
#SPJ8
SVART We wish to compute 22+2 de 23 +3.2 - 92 - 27 0) We begin by factoring the denominator of the rational function. We get 23 +32 - 92 - 27- (2-a) (0 - 1) for ab. What area and b? FORMATTING: Make sure corresponds to the factor of the denominator that repeat we a b (1) Next, we express the fraction in the form 2+2 A B C + 23 +3.2 - 9. - 27 Give the exact values of A, B and C FORMATTING: Make sure A, B and C correspond to the appropriate denominators, as given in the above setup. A= 25/35 491 B= - 11/6 11/38 () Finally, we use this partial traction decomposition to compute the integral. Give its approximate value with 3 decimal places 2+2 dar -0.189 +3+32 - 9x - 27 La stion Mer
Answers
Given that 22+2 de 23+3.2-92-27We begin by factoring the denominator of the rational function: 23+32-92-27-(2-a)(0-1) for ab.
We can write the given function as:2 + 2/(x - 2) + 3/(x + 3) - 9/(x - 9) - 27/(x + 27)To find the values of A, B, and C, we need to take the common denominator which is (x - 2)(x + 3)(x - 9)(x + 27)A
= 2(x + 3)(x - 9)(x + 27) + 2(x - 2)(x - 9)(x + 27)
= 50x³ + 5350x² + 41325x + 52590B
= 3(x - 2)(x - 9)(x + 27) - 9(x + 3)(x - 2)(x + 27)
= - 44x³ - 2919x² - 405x - 972C
= (x - 2)(x + 3)(x - 9) + 3(x - 2)(x + 27) - 9(x - 9)(x + 27)
= - 6x³ - 27x² + 1107x + 972.
The partial fraction decomposition is given as:2 + 2/(x - 2) + 3/(x + 3) - 9/(x - 9) - 27/(x + 27) = (25/35)/(x - 2) + (-11/6)/(x + 3) + (11/38)/(x - 9) + (- 1/2)/(x + 27) Now, we can integrate it as follows: ∫(25/35)/(x - 2)dx + ∫(-11/6)/(x + 3)dx + ∫(11/38)/(x - 9)dx + ∫(-1/2)/(x + 27)dx= 25/35 ln│x - 2│ - 11/6 ln│x + 3│ + 11/38 ln│x - 9│ - 1/2 ln│x + 27│ + c
To know more about factoring visit:
https://brainly.com/question/14549998
#SPJ11
Point A is located at (0,4) and point B is located at (-2, -3). Find the x value for the point that is 1/4 the distance firm point A to point B. -1.5 -2 -0.5 -1
Answers
Answer:
.25
Step-by-step explanation:
distance between 0,4 is 1. The distance between _2 and _3 is minus 5. X equals.
.25
What would be the interest for Rs. 1000 in 2 years at the rate of Rs. 2 per month per hundred rupees? discribe
Answers
Answer:
Step-by-step explanation:
principle=1000
time= 2 years
rate = 2×12=24/100=0.24%
simple intrest= p×r×t
= 1000 × 0.24 × 2
=480 rupees
total amount will be 1000 + 480= 1480
Construction 1: To construct a line segment congruent to a given line segment Given: Line Segment AB To Construct: A line segment congruent to AB Construction: On a working line w, with any point C as a center and a radius equal to AB, construct an arc intersecting w at D. Then CD is the required line segment. Since AB = CD, AB = CD by definition of congruency
Answers
To construct a line segment congruent to AB, draw an arc with center C and radius AB on a working line w, intersecting w at D, resulting in CD being congruent to AB by having the same length.
To construct a line segment congruent to a given line segment AB:
Draw a working line w.
Use point C as the center and construct an arc with a radius equal to the length of AB.
Let the arc intersect line w at point D.
Line segment CD, connecting points C and D, is the required line segment.
By construction, CD is congruent to AB because they have the same length.
So, the correct statement should be: Since AB and CD have the same length, AB = CD, which demonstrates congruency between the line segments.
To know more line segment,
https://brainly.com/question/7988965
#SPJ11
Calculate the volume of the shaded space. Round to the nearest tenth, if necessary.

Answers
Answer:
Volume of the shaded space = 4480.0 yd³
Step-by-step explanation:
Volume of the shaded space = Volume of cuboid - Volume of Pyramid placed inside
Volume of cuboid = Length × Width × Height
= 28 × 12 × 20
= 6720 yd³
Volume of pyramid = \(\frac{1}{3}(\text{Area of the base})(\text{Height})\)
= \(\frac{1}{3}(28\times 12)(20)\)
= 2240 yd³
Volume of the shaded space = 6720 - 2240
= 4480.0 yd³
10
이
10
4
Which of the following are integers
Answers
Integers consists of positive whole numbers and their negative opposites and zero. |0| is equal to 0 which also makes it an integer.
Can someone help me with this one problem!!!!

Answers
Answer: No
Step-by-step explanation: No because y is not the same as x.
Picture attached below

Answers
Answer:
D. sin J = Cos L
Step-by-step explanation:
LK = √219² - 178² = 127.58
sin J = LK/LJ =127.58/219 = 0.58
Cos L = Lk/LJ = 0.58
sin L = 178/219 = 0.81
Cos J = 178/219 = 0.81
Tan J = 127.58/178 = 0.72
tan L = 178/127.58 = 1.40
The total cost of renovation depends on labor and materials. You decide to budget $25,000 towards labor. The amount, A, that each worker earns varies inversely to the number of workers on the job, n.
1) complete the table to indicate the amount earned for the given number of workers.
2) what do you notice about the product, A*n?
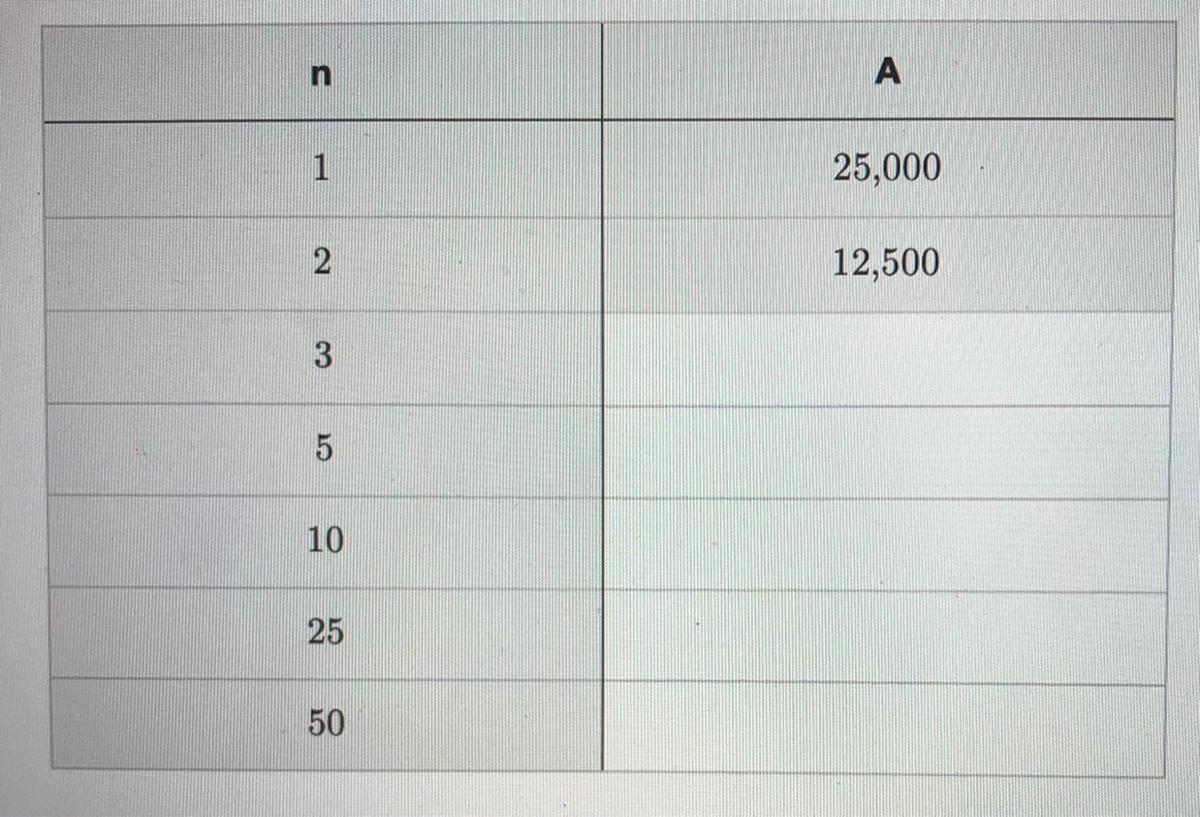
Answers
Answer:
see attachedA*n is the constant 25000Step-by-step explanation:
1)The amount A is said to vary inversely with n, so the equation relating the two is ...
A = k/n
The value of k can be found by multiplying this equation by n:
k = nA
Using values from the first line of the table, we find ...
k = (1)(25000) = 25000
So the relation we need to use to fill the table is ...
A = 25000/n
The filled table is attached.
__
2)As we saw in part 1, the product A*n is the constant of proportionality. It is a constant.

6. The graph of an exponential function is
shown. Write an equation for the function.
manu (3,13.5)
(2,4.5)
(1, 1.5)
olismolensando
Answers
On soloing the provided question, we can say that an equation from the graphs for the function. 3x + 13.5y = 4.5 and x+1.5y = 2
What is graphs?Mathematicians use graphs to logically represent or chart facts or values. Typically, a graph point will show the relationship between two or more objects. A graph is a non-linear data structure made up of nodes, or vertices, and edges. The nodes, also known as vertices, should be joined with glue. This graph's vertices are 1, 2, 3, and 5, while its edges are 1, 2, 1, 3, 2, 4, and 2.5. (3.5). (4.5). Graphical depictions of exponential growth in statistical charts (bar charts, pie charts, line charts, etc.). triangle-shaped logarithmic graph.
an equation for the function.
3x + 13.5y = 4.5
and x+1.5y = 2
To know more about graphs visit:
brainly.com/question/11950136
#SPJ1
Ana needs 40 mg of medicine twice a day. Each medicine tablet contains 15 mg, how many tablets will Ana need for 2 days?
Answers
Answer:
She will need 10 full tablets and two thirds of a tablet
Step-by-step explanation:
First we will find how many tablets ana needs for one day:
In each day ana needs 40x2 mg of medicine, they come in tablets of 15 mg. In one day, she needs 80 / 15 = 5 and 1/3 of a tablet.
In two days, she needs double that amount. (5 and 1/3) x 2 = 10 and 2/3
Ana need to take 10 and 2/3 tablets for 2 days.
What is an expression?An expression is a number, or a variable, or a combination of numbers and variables and operation symbols.
It is given that,
Ana need 40 mg tablet twice a day.
So, Total mg taken in a day = 40*2 mg = 80 mg
Since each tablet contains 15 mg
So, Total tablets taken in a day = Total mg taken / 15
⇒ Total tablets taken in a day = 80 / 15
⇒ Total tablets taken in a day = 5 and 1/3 tablets
Therefore Tablets needed in 2 days = 2*Total tablets taken in a day
⇒ Tablets needed in 2 days = 2*16/3
or, Tablets needed in 2 days = 32/3
or, Tablets needed in 2 days = 10 and 2/3 tablets
Thus,Ana need to take 10 and 2/3 tablets for 2 days.
To learn more about tablets:
https://brainly.com/question/15530165
#SPJ2
find the volume of the solid. PLEASE ANSWER Need help

Answers
Answer:
(5 × 7 × 13) + (6 × 7 × 3) = 455 + 126
= 581 cubic cm
28 liters in 2 minutes= ___ liters per minute
Answers
Answer:
14
Step-by-step explanation:
Write (8 x 10) + (6 x 1) +(2 x 0.1) + (7 x 0.001) with words.
Answers
Answer:
Eight times ten plus six times one plus two times one-tenth plus seven times one-thousandth.
Step-by-step explanation:
(8 x 10) = eight times ten
(6 x 1) = six times one
(2 x 0.1) = two times one-tenth
(7 x 0.001) = seven times one-thousandth
I am not always the best with the equations to words stuff, so please comment if I am wrong!
Determine all the unknown values of the figure below.

Answers
Answer:
F=10, S=26
Step-by-step explanation:
We know that the base is 24. Using that information, 10^2+24^2=26^2 which is a pythagorean triple
y varies jointly as x and z. If y = 12 when x = 4 and z = 3, find y when x = 9 and z = 8.
Answers
Answer:
y = 72
Step-by-step explanation:
Given y varies jointly as x and z then the equation relating them is
y = kxz ← k is the constant of variation
To find k use the condition y = 12 when x = 4 and z = 3
12 = k × 4 × 3 = 12k ( divide both sides by 12 )
1 = k
y = xz ← equation of variation
When x = 9 and z = 8 , then
y = 9 × 8 = 72
