Without using a calculator, compute the sine and cosine of 117 by using the reference angle. 6 What is the reference angle? radians. in what quadrant is this angle? (answer 1, 2, 3, or 4) 11л sin (167) COS 117 6 (Type sqrt(2) for V2 and sqrt(3) for 3.)
Answers
The reference angle is 27° and the angle of 117° is in the second quadrant. The sine of 117° is 1/2 and the cosine of 117° is -sqrt(3)/2.
To calculate the relation angle, we need to find the acute angle. To do this, we subtract 90 degrees from 117 degrees to get the reference angle.
reference angle = 117 degrees - 90 degrees = 27 degrees
117 degrees is in the second quadrant. we know that the sine of 117 degrees is positive and the cosine of 117 degrees is negative.
Using the reference angle of 27 degrees, the trigonometric angles are:
sin(117 degrees) = sin(180 degrees - 27 degrees)
sin(117 degrees) = sin(27 degrees)
sin(117 degrees) = 1/2
cos(117 degrees) = -cos(180 degrees - 27 degrees)
cos(117 degrees) = -cos(27 degrees)
cos(117 degrees) = -sqrt(3)/2
Therefore, we can conclude that the reference angle is 27 degrees and the angle of 117 degrees is in the second quadrant.
To learn more about reference angle
https://brainly.com/question/1603873
#SPJ4
Related Questions
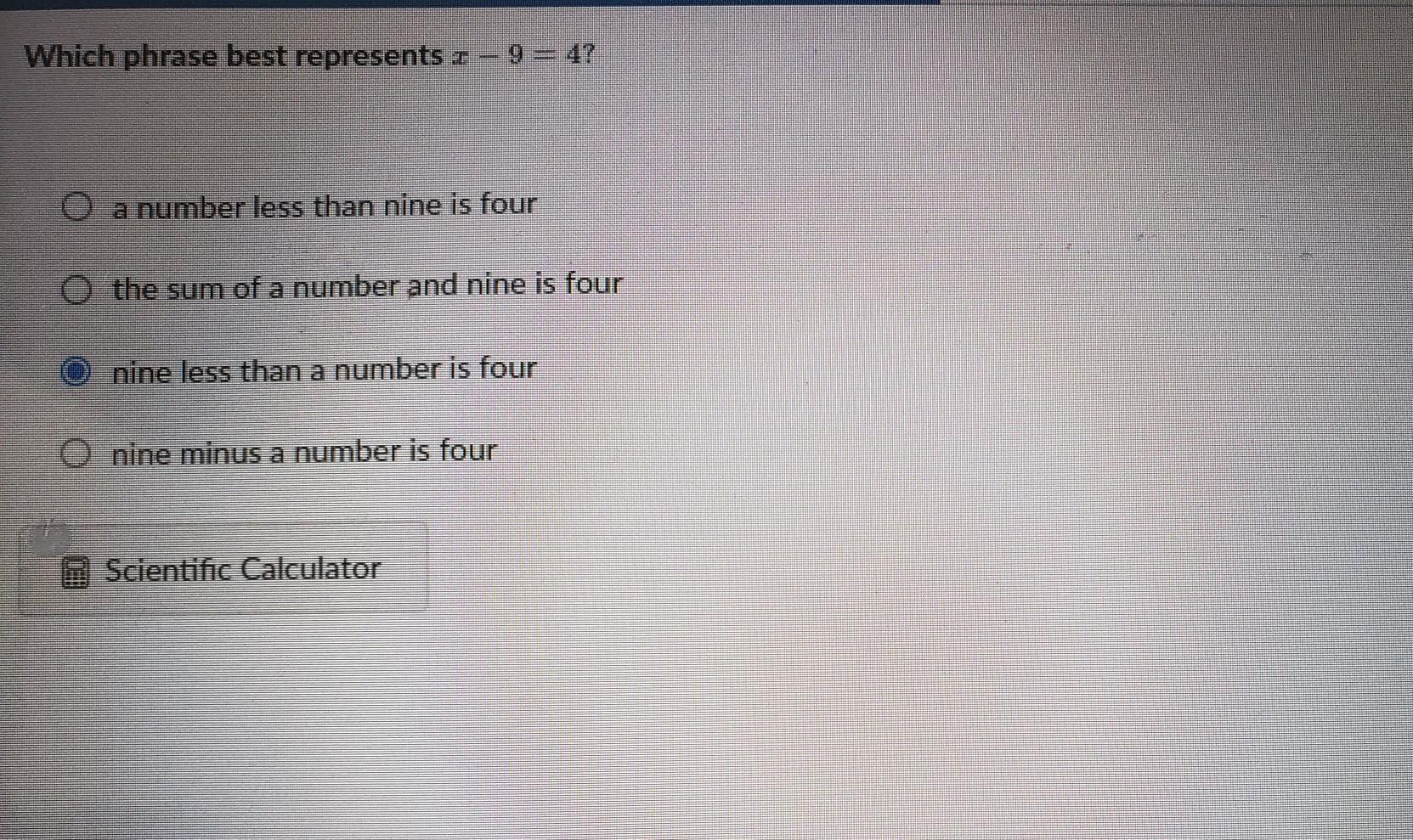
2/16 2 + x = 12
what is the answer to this equation?
Answers
Answer:
nomelase pero será chida la imagen que tienes
Please someone help with that

Answers
Answer:
a) -tan(π/4) = -1
b) -1/sin(π/3) = -(2/3)√3
Step-by-step explanation:
The given functions are reciprocals of the primary trig functions:
cot(x) = 1/tan(x)
csc(x) = 1/sin(x)
The tangent function has a period of π, and is equal to the cotangent of the complementary angle.
__
a)The given angle is an alias of -π/4, so we can write ...
cot(7π/4) = cot(-π/4) = -cot(π/4) = -tan(π/2 -π/4) = -tan(π/4)
-tan(π/4) = -1
__
b)csc(-π/3) = 1/sin(-π/3) = -1/sin(π/3) = -1/(√3/2) = -2/√3
-1/sin(π/3) = -2√3/3
Able has a younger sister and an older brother.
- Able’s age can be represented by x.
- Able’s sister is 4 years younger than him.
- Able’s older brother is twice as old as he is.
- The combined age of all three siblings is 32.
How old is Able’s younger sister?
9 years
13 years
5 years
3 years
Answers
Answer:
5 years
Step-by-step explanation:
1. if able's age is x, then his sister's is x-4, and his brother's is 2x
2. if these sum to 32, then we can formulate the equation x+(x-4)+2x=32
3. now solve for x, 4x-4=32, 4x=36, x=9
4. if able's sister is x-4 years old, she is 9-4, or 5 years old
For which set of values of x is the algebraic expressions x^2-16/x^2-4x-12 undefined
Answers
Answer:
x= -2 & x = 6
Step-by-step explanation:
To find undefined points, you need to know when the bottom of the fraction is equal to 0, because you can't divide by 0! (The numerator is irrelevant.) In order to do this, you can factor the denominator of x^2 - 4x - 12. What factors of -12 add up to -4? That would be -6 and 2, so you can factor it out to (x-6)(x+2). Because the coefficient of x in both of these cases is 1, you can take the shortcut of just taking the opposite sign of the two numbers being added to x in these factors, giving you 6 and -2 for your undefined values.
Let me know if you need a more thorough explanation, as I sort of skipped through some wordy things there to give a more concise answer.
Find the directional derivative of f at the given point in the direction indicated by the angle theta. f(x, y) = y cos(xy), (0, 1), theta = pi/3 D_u f(0, 1) =
Answers
Answer:
The directional derivative of f at (0, 1) in the direction of theta = pi/3 is sqrt(3)/2.
Step-by-step explanation:
To find the directional derivative of f at (0, 1) in the direction of theta = pi/3, we need to compute the dot product of the gradient of f at (0, 1) and the unit vector u in the direction of theta.
First, we need to find the gradient of f:
∇f(x, y) = <∂f/∂x, ∂f/∂y>
= <-y sin(xy), cos(xy) - xy sin(xy)>
Evaluating at (0, 1), we get:
∇f(0, 1) = <0, 1>
Next, we need to find the unit vector u in the direction of theta = pi/3:
u = <cos(pi/3), sin(pi/3)>
= <1/2, sqrt(3)/2>
Now we can compute the directional derivative:
D_u f(0, 1) = ∇f(0, 1) · u
= <0, 1> · <1/2, sqrt(3)/2>
= sqrt(3)/2
Therefore, The directional derivative of f at (0, 1) in the direction of theta = pi/3 is sqrt(3)/2.
of f at (0, 1) in the direction of theta = pi/3 is sqrt(3)/2.
To know more about directional derivative, refer here:
https://brainly.com/question/30365299
#SPJ11
what is the answer to number 2 at the top?

Answers
The equation of the transformed exponential function g(x) is g(x) = 2^-x - 1
Writing an exponential function for the graph of g(x)From the question, we have the following parameters that can be used in our computation:
Parent function: y = 2^x
The graph of the transformed exponential function g(x) passes through the points (-2,3), (-1,1), (0,0), (1,-0.5) and (2, -0.75)
So, we have the following transformation steps:
1st Transformation:
Reflect y = 2^x across the y-axis
So, we have
y = 2^-x
2nd Transformation:
Translate y = 2^-x down by 1 unit
So, we have
y = 2^-x - 1
This means that
g(x) = 2^-x - 1
Hence, the equation of the function g(x) is g(x) = 2^-x - 1
Read more about transformation at
brainly.com/question/1548871
#SPJ1
Expedia would like to test if the average round-trip airfare between Philadelphia and Dublin is less than $1,200. Which of the following hypothesis tests should be performed? A. Left-tailed B. Right-tailed C. Two-tailed D. There is not enough information to answer.
Answers
The subsequent hypothesis tests has to be run the alternative hypothesis Hₐ: μ < 1200, it is a left tail test.
Given that,
If the typical round-trip cost between Philadelphia and Dublin is less than $1,200, Expedia would want to investigate.
We have to find which of the subsequence hypothesis tests has to be run.
We know that,
The claim : If the average round-trip between Philedelphia and
Dublin is less than $1200.
So, claim is the alternate hypothesis.
Hₐ: μ < 1200
H₀: μ = 1200
Since the Hₐ: μ < 1200, it is a left tail test.
Therefore, the subsequent hypothesis tests has to be run the alternative hypothesis Hₐ: μ < 1200, it is a left tail test.
To learn more about hypothesis visit: https://brainly.com/question/29407794
#SPJ4
The dally wasginal revehish function assodated with selling x widgets is given ty AT(x)=+9x2+8x+10 (a) Doternine the revenue function, R(x), astociated with groduciog and aeiling s widgets. f(x)= (b) Determine the demand funclas reiating unf price, pet to to the couarsity demanded,
Answers
Given, the daily marginal revenue function associated with selling x widgets is given by AT(x) = +9x² + 8x + 10.
(a) Determine the revenue function, R(x), associated with producing and selling s widgets. f(x) =
(b) Determine the demand function relating the price, p, to the quantity demanded.
a) The revenue function, R(x), associated with producing and selling s widgets can be determined by integrating the daily marginal revenue function associated with selling x widgets. So, we have, R(x)
= ∫AT(x)dxFor this, let us integrate AT(x) by using integration formula, ∫x² dx
= (x³ / 3) + C∫(9x² + 8x + 10) dx
= (3x³ / 3) + (4x² / 2) + (10x / 1) + C
= 3x³ + 4x² + 10x + CTherefore, the required revenue function, R(x) is,R(x)
= 3x³ + 4x² + 10x + CWhere, C is a constant of integration.
b) The demand function relating the price, p, to the quantity demanded can be obtained by solving for the inverse of the demand function.The inverse of the demand function is given by, x = f⁻¹ (p).
AT(x) = 9x² + 8x + 10AT(x) + C
= 9x² + 8x + 10 + CNow, let us find the vertex of the parabolic function 9x² + 8x + 10.The x-coordinate of the vertex is given by, x = - b / (2a)
= - (8) / (2 x 9)
= - 4 / 9The y-coordinate of the vertex is given by, AT(- 4 / 9)
= 9(- 4 / 9)² + 8(- 4 / 9) + 10
= - 16 / 3 - 32 / 9 + 10
= - 38 / 9The vertex is, (- 4 / 9, - 38 / 9) Since the coefficient of the quadratic term is positive, the graph of the function opens upward. So, the demand function is a parabolic function with a vertex at (- 4 / 9, - 38 / 9).Therefore, the demand function relating the price, p, to the quantity demanded is given by,x = f⁻¹ (p) = (- 4 / 9) ± √(38 / 81 - AT(p)) / 9.
To know more about function, visit:
https://brainly.com/question/31062578
#SPJ11
PLEASE HELP WILL GIVE BRAINLIEST!!!!
Answers
Consider a continuous random variable x, which is uniformly distributed between 65 and 85. The probability of x taking on a value between 75 to 90 is ________. 0.50 0.075 0.75 1.00
Answers
The probability of x taking on a value between 75 to 90 is 0.25.
Given that x is a continuous random variable uniformly distributed between 65 and 85.To find the probability that x lies between 75 and 90, we need to find the area under the curve between the values 75 and 85, and add to that the area under the curve between 85 and 90.
The curve represents a rectangular shape, the height of which is the maximum probability. So, the height is given by the formula height of the curve = 1/ (b-a) = 1/ (85-65) = 1/20.Area under the curve between 75 and 85 is = (85-75) * (1/20) = (10/20) = 0.5Area under the curve between 85 and 90 is = (90-85) * (1/20) = (5/20) = 0.25.
To know more about variable visit:
https://brainly.com/question/15740935
#SPJ11
What is the solution to the equation below? Round your answer to two decimal places. log2 X= 1.7 A. x= 8.35 O x B. x= 13.60 O C. x = 10.56 = O D. X = 9.41
Answers
Solution
Given the question in the image, the following are the solution steps to answer the question.
STEP 1: Write the given logarithmic expression
\(\log_4x=1.7\)STEP 2: Find the value of x
State the rule of logarithm that applies
\(if\text{ }\log_ab=c,b=a^c\)Apply the rule
\(\begin{gathered} \log_4x=1.7 \\ \therefore4^{1.7}=x \\ by\text{ simplification,} \\ 10.55606329=x \\ x\approx10.56 \end{gathered}\)Hence, the value of x is approximately 10.56
using six rectangles to estimate this area or value of the definite integral is not very accurate (see the previous image). we either have an underestimate of the rectangles are below the curve, or we have an overestimate of the rectangles are above the curve. how could you improve on this estimate? suggest as many ways as you can think of to improve our accuracy for this area.
Answers
To improve the accuracy of estimating the area or value of a definite integral using rectangles, there are several strategies one can employ: 1. Increase the number of rectangles:
By subdividing the region into more rectangles, we can get a finer approximation of the area. The more rectangles we use, the closer the estimate will be to the actual value. 2. Use different types of rectangles: Instead of using only one type of rectangle (e.g., left endpoints or right endpoints), we can use a combination of left, right, and midpoint endpoints. This technique, known as the composite rule, can provide a more accurate estimation by minimizing the bias introduced by a single type of rectangle. 3. Use a more advanced numerical integration method: Instead of relying solely on the rectangle approximation (such as the Riemann sum), we can employ more sophisticated methods like the trapezoidal rule or Simpson's rule. These methods use a combination of rectangles and other shapes to provide a more accurate estimation.
4. Utilize adaptive methods: Adaptive methods adjust the size and placement of rectangles based on the behavior of the function being integrated. These methods dynamically allocate more rectangles to areas where the function exhibits significant variation, improving the accuracy of the estimate. 5. Use higher-order approximations: Higher-order approximations, such as higher-degree polynomial interpolations, can provide better accuracy by fitting the function more closely within each rectangle. By implementing these strategies, we can significantly enhance the accuracy of estimating the area or value of a definite integral using rectangles.
Learn more about six rectangles here: brainly.com/question/12963995
#SPJ11
According to the information presented in this video, all of the following activities are likely to occur in the information requirements determination (IRD) phase of systems analysis except _____.
A. reviews of paper-based forms and reports
B. meetings with the development team
C. beta testing
D. observation of system use
E. interviews with system users
Answers
According to the information presented in this video, all of the following activities are likely to occur in the information requirements determination (IRD) phase of systems analysis except beta testing.
According to the information presented in this video, all of the following activities are likely to occur in the information requirements determination (IRD) phase of systems analysis except beta testing. The IRD phase of the systems analysis process is used to determine information requirements for the system being developed. The IRD process identifies what information is needed to support business functions and operations.
The activities that are carried out during the IRD phase of the systems analysis process include: Reviews of paper-based forms and reports Interviews with system users Observation of system use Meetings with the development team Therefore, the answer is option C. Beta testing is not a part of the IRD phase of systems analysis.
To know more about information visit:
brainly.com/question/28259519
#SPJ11
a bag contains 2 red, 7 black, and 8 white balls. three balls are drawn, without replacement. what is the probability that one of each color is drawn? (enter your probability as a fraction.)
Answers
14/85 is the probability that one of each color is drawn.
How does probability explain work?
How likely something is to occur is known as its probability.We can discuss the probabilities of various outcomes, or how likely they are, whenever we are unsure of how an event will turn out.Statistics is the study of events subject to probability.Red balls = 2
black balls = 7
white balls = 8
total balls = 2 + 7 + 8 = 17
p( one ball from each color = ²C₁ * ⁷C₁ * ⁸C₁/ ¹⁷C₃
= 2 * 7 * 8//680
= 14/85
Learn more about probability
brainly.com/question/11234923
#SPJ4
Giving brainlist to who answeres this!

Answers
Answer:
Pretty sure its 20
Step-by-step explanation:
I just did the math
Answer:
5 i think
Step-by-step explanation:
§(* ̄▽ ̄*)§§(* ̄▽ ̄*)§§(* ̄▽ ̄*)§§(* ̄▽ ̄*)§§(* ̄▽ ̄*)§(∪.∪ )...zzz(∪.∪ )...zzz(∪.∪ )...zzz(∪.∪ )...zzz
Solve for n.Plse/thank you

Answers
Answer:
n= 36.87°
Step-by-step explanation:
opp/hyp = sin(n)
12/20 = sin(n)
sin^-1 (12/20) = n
n= 36.87°
Answer:
36.9°
Step-by-step explanation:
12 is the side OPPOSITE from the n° angle we're looking for. It is not touching the angle in any way; its completely way over on the OPPOSITE side of the right triangle. The 20 unit long side is the HYPOTENUSE of the right triangle (the longest side)
The trig function that puts together OPP and HYP is the sine function.
sin n° = OPP/HYP
sin n° = 12/20
sin n° = 0.6
Now you need a calculator to find the angle when you know the ratio (you have the ratio--its 12/20 or .6)
Probably (hopefully) above
the sin key on the calculator is sin^-1 or arcsin. You should use a shift key to use this function to find the angle. sin^-1 has nothing to do with -1 exponent, it is just the way to write "find the angle if you know the ratio"
sin ^-1 (0.6) = 36.87°
Some calculators even abbreviate arcsin even shorter to asin...(lame, bc too many different abbreviations!)
arcsin (0.6) = 36.87°
OR
asin (0.6) = 36.87°
Whatever the button says, it should come out the same. Round to the nearest degree 37° or round to the nearest tenth degree 36.9° or round to the nearest hundredth degree 36.87°
On a test that has a normal distribution, a score of 54 falls two standard deviations
above the mean, and a score of 42 falls one standard deviation below the mean.
Determine the mean of this test.
Answers
Let μ be the mean of the distribution and let σ be its standard deviation.
We know that 54 falls two standard deviations above the mean, this can be express as:
\(\mu+2\sigma=54\)We also know that 42 falls one standard deviation below the mean, this can be express as:
\(\mu-\sigma=42\)Hence, we have the system of equations:
\(\begin{gathered} \mu+2\sigma=54 \\ \mu-\sigma=42 \end{gathered}\)To find the mean we solve the second equation for the standard deviation:
\(\sigma=\mu-42\)Now we plug this value in the first equation:
\(\begin{gathered} \mu+2(\mu-42)=54 \\ \mu+2\mu-84=54 \\ 3\mu=138 \\ \mu=\frac{138}{3} \\ \mu=46 \end{gathered}\)Therefore, the mean of the distribution is 46
suppose that p is an int* variable. write several lines of code that will make p point to an array of 100 integers, place the numbers 0 through 99 into the array components, and return the array to the heap. do not use malloc.
Answers
The array can be created by declaring it and then assigning it to a pointer using the array name. Here is the code for making p point to an array of 100 integers, placing the numbers 0 through 99 into the array components, and returning the array to the heap without using malloc.```
int myArray[100];
int *p = myArray;
for (int i = 0; i < 100; i++) {
myArray[i] = i;
}
In the first line of code, an array of 100 integers is created by declaring it. The second line creates a pointer variable p that is set to the beginning of the array. Since the first element of an array can be accessed with a pointer to that array, p is equivalent to &myArray[0].The for loop fills the array with numbers from 0 to 99. The line myArray[i] = i; assigns the i-th element of the array the value of i.In the end, the array is returned to the heap.
Learn more about Array
brainly.com/question/30757831
#SPJ11
is the sequence geometric if so identify the common ratio -2 -4 -16
Answers
Yes, the sequence is geometric as it follows a pattern where each term is multiplied by a common ratio to get the next term. In this case, we can find the common ratio by dividing any term by its preceding term.
Let's choose the second and first terms:Common ratio = (second term) / (first term)= (-4) / (-2)= 2Now that we know the common ratio is 2, we can use it to find any term in the sequence. For example, to find the fourth term, we can multiply the third term (-16) by the common ratio:Fourth term = (third term) × (common ratio)= (-16) × (2)= -32Therefore, the fourth term of the sequence is -32. We can continue this pattern to find any other term in the sequence.
To know more about pattern visit :-
https://brainly.com/question/30571451
#SPJ11
Georgie buys a pack of chocolate which has 18 small chocolate bars. She ate three bars in the morning, Later in the
day, Georgie's brother ate three fifth of the chocolates bar that were left, what fraction of the chocolate bars is left in
the packet?
Answers
So if Georgie’s brother ate 3/5 you would figure out how many candy bars he ate by:
15 multiplied by the 3/5 = 9
So out of the 18 candy bars Georgie ate 3 and Georgie’s brother ate 9.
Henry drives at a speed of 80miles per hour how long will it take to drive 400miles
Answers
Step-by-step explanation:
6-4 practice properties of rhombuses rectangles and squares
Answers
Quadrilaterals, rectangles, and squares are all quadrilaterals, which means they have four sides. However, there are some differences in their properties. Here are the 6 properties of rhombuses, rectangles, and squares:
Properties of Rhombuses:
A rhombus has four congruent sides.Opposite angles of a rhombus are congruent.Diagonals of a rhombus bisect each other at right angles.The diagonals of a rhombus are perpendicular.The area of a rhombus can be calculated as A = ½ (d1 x d2), where d1 and d2 are the lengths of the diagonals.The perimeter of a rhombus is equal to four times the length of one side.Properties of Rectangles:
A rectangle has four right angles.Opposite sides of a rectangle are congruent.The diagonals of a rectangle are congruent.The area of a rectangle can be calculated as A = l x w, where l is the length and w is the width.The perimeter of a rectangle can be calculated as P = 2(l + w), where l is the length and w is the width.The diagonals of a rectangle bisect each other.Properties of Squares:
A square has all four sides congruent.All four angles of a square are right angles.The diagonals of a square are congruent and bisect each other at right angles.The area of a square can be calculated as A = s^2, where s is the length of one side.The perimeter of a square can be calculated as P = 4s, where s is the length of one side.The diagonals of a square divide it into four congruent right triangles.For more details about quadrilaterals click here:
https://brainly.com/question/29934440#
#SPJ11
If the length of the game room is 12 feet, what is the total square footage of the two rooms?
Answers
Answer:
Step-by-step explanation:
Well, all we have to do is square 12.
= \(12^{2}\)
= 12 x 12
= 144
The total square footage of the room is 144 feet.
I am not sure if this answer is correct, so please tell me if I am wrong. I hope this answer helped you! :)
If P(B)=0.3,P(A∣B)=0.5,P(B ′ )=0.7, and P(A∣B ′ )=0.8, find P(B∣A).
Answers
If P(B)=0.3, P(A|B)=0.5, P(B')=0.7and P(A|B')=0.8, then the value of the probability P(B|A)= 0.2113
To find the value of P(B|A), follow these steps:
The probability of B given A can be given by the product of the probability of A given B and the probability of B, divided by the total probability of B. So, the formula for P(B|A) = P(A|B) * P(B) / [P(A|B)*P(B)+P(A|B')*P(B')]. Substituting the values, we get P(B|A) = (0.5) (0.3) / [(0.5) (0.3) + (0.8) (0.7)] ⇒P(B|A) = 0.15 / [0.15 + 0.56] ⇒P(B|A) = 0.15 / 0.71 ⇒P(B|A) = 0.2113. Therefore, P(B|A) = 0.2113.Learn more about probability:
brainly.com/question/13604758
#SPJ11
A valid multiple regression analysis assumes or requires that Select one: a. The dependent variable is measured using an ordinal, interval, or ratio scale b. The residuals follow an F distribution c. The independent variables and the dependent variable have a linear relationship d. The observations are autocorrelated
Answers
The answer is option c. The independent variables and the dependent variable have a linear relationship.
The dependent variable and the independent variables must have a linear connection in order for a multiple regression analysis to be valid. This indicates that a straight line can be used to model the connection that exists between the variables that are independent and the dependent variable. Additionally, the dependent variable should be measured using an ordinal, interval, or ratio scale, as the regression model assumes that the dependent variable is continuous.
The residuals of the regression model should also follow a normal distribution, rather than an F distribution, in order to ensure that the model is accurately capturing the relationship between the independent variables and the dependent variable. Finally, the observations should not be autocorrelated, as this can lead to biased estimates and invalid inferences. Overall, adherence to these assumptions is critical for producing accurate and meaningful results from a multiple regression analysis.
To know more about regression analysis visit:
https://brainly.com/question/30011167
#SPJ4
Complete question
A valid multiple regression analysis assumes or requires that Select one:
a. The dependent variable is measured using an ordinal, interval, or ratio scale
b. The residuals follow an F distribution
c. The independent variables and the dependent variable have a linear relationship
d. The observations are autocorrelated
A probability experiment consists of rolling a fair 12 sided die. Find the probability of the event below rolling an 11
Answers
Answer:
the chance is 1/12 or 8.333...%
hope this helps
Step-by-step explanation:
State the following key features of the quadratic function below AND determine its equation. Thank you so much for your time I appreciate it loads!

Answers
Answer:
vertex: (4, -18)
domain: all real numbers
range: y ≥ -18
axis of symmetry: x = 4
x-intercepts: {-2, 10}
y-intercept: -10
min/max value: -18
equation: y= x^2 - 8x - 20
Step-by-step explanation:
- for the vertex, look at the graph. it should be the maxi/minimum point.
- the domain is all real numbers because this quadratic function has no restrictions. as you can see, there are arrows on both ends.
- the range can be -18 or greater than -18 as shown by the graph.
- the axis of symmetry is x = 4. it's like the mirror line.
- the x-intercept is when the function touches the x axis when y is equal to 0.
- the y-intercept is when x = 0 on the y axis.
- the min/max value is basically the y coordinate of the vertex. you can also look at the graph for it.
- find the quadratic equation using the roots
y = (x+2)(x-10)
y = x^2 -8x - 20
What I am really in need of help. If anyone answers this I will award 60 points to anyone!

Answers
You have torn a tendon and is facing surgery to repair it. The surgeon explains the risks to you: infection occurs in 2% of such operations, the repair fails in 13%, and both infection and failure occur together in 1%. What percentage of these operations succeed and are free from infection?
Answers
Out of the given statistics, the percentage of operations that succeed and are free from infection can be calculated as 84%.
To determine this, we need to subtract the combined occurrence of infection and failure (1%) from the total failure rate (13%). This yields the percentage of operations that fail but do not have an infection, which is 12%. Subtracting this from 100% gives us the percentage of operations that succeed and are free from infection, which is 88%.
However, since the question specifically asks for the percentage of operations that succeed and are free from infection, we need to consider that the 2% infection rate is included in the total failure rate. This means that out of the total failure rate of 13%, 2% accounts for the operations that have both infection and failure. Therefore, we subtract this 2% from the total failure rate of 13%, resulting in a failure rate of 11% without infection.
By subtracting this failure rate without infection from 100%, we obtain the percentage of operations that succeed and are free from infection, which is 89% - 11% = 84%. Thus, approximately 84% of these operations are expected to succeed and be free from infection.
Learn more about percentage here:
https://brainly.com/question/28960256
#SPJ11
The angle measurements in the diagram are represented by the following expressions.

Answers
Answer:
since those 2 lines are parrelel that means the angles are the same so
8x+6=4x+38
minus 6
8x=4x+32
minus 4x
4x=32
divide by 4
x=8
Hope This Helps!!!
The value of the x is 11 and the measure of the angle B is 82 degrees.
What are vertically opposite angles?It is defined as the angles when two lines intersect each other and at the intersecting point, some pair of angles are formed which we call vertically opposite angles, as the name describes that they have vertically opposite angles.
We have:
The angle measurements in the diagram are represented by the following expressions:
Angle A = 8x - 6
Angle B = 4x + 38
By using the vertically opposite angle property in angle B
As we know the alternate exterior angle are equal
8x - 6 = 4x + 38
4x = 44
x =11
Measure of angle B = 4(11) + 38 = 82 degrees
Thus, the value of the x is 11 and the measure of the angle B is 82 degrees.
Learn more about the vertically opposite angles here:
brainly.com/question/24287162
#SPJ5