Which of the following is the best buy?
12 pens for $1.56
15 pens for $1.65
9 pens for $1.35
18 pens for $2.52

Answers
Answer:
B
Step-by-step explanation:
It cannot be D, because it costs way more, it cannot be C because there are other options that provide better value. We can finally determine that it is B because for 3 pens more it is only 9 cents more
Related Questions
Find the principal. Annual rate of interest = 11 \%=11%equals, 11, percent Period = 3=3equals, 3 years Total interest = 825=825equals, 825 rupees Principal ==equals rupees
Answers
Answer:
Principal = 2,500 rupees
Step-by-step explanation:
The interest or simple interest is the extra money gained on n invested amount of money yielding a certain percentage interest at a time (t) in years
The formula for simple interest is given as follows:
I = P × R × T
where:
I = interest = 825 rupees
P = principal
R = interest rate = 11% = 11/100 = 0.11
T = time = 3 years
∴ 825 = P × 0.11 × 3
825 = P × 0.33
P = 825 ÷ 0.33
P = 2,500 rupees
This means that 2,500 rupees was invested to saved to yield an interest of 825 rupees after 3 years at a rate of 11% per year
how to do this question plz

Answers
Answer:
86.6 feet (3 s.f.)
Step-by-step explanation:
Please see attached picture.
tan60°= height of tower/ 50
Height of tower
= 50tan60°
= 50√3
= 86.6 ft (3 s.f.)
tanѲ= opp/ adj

this is due in less than 30 mins pls help

Answers
At a certain location a river is 12 meters wide. At this location the depth of the river in meters has been measured at 3 meter intervals.The cross-section is shown below. 3 3 3 3 0.5 2.3 12.9 2.1 13.8 (a) Use Simpson's rule with the five depth measurements to calculate the approximate area of the cross-section. 11 marks) (b) The river flows at 0.4 meters per second Compute the approximate volume of water flowing through the cross-section in 10 seconds
Answers
According to the information we can infer that the approximate area of the cross-section is 35.5 square meters. Additionally, the approximate volume of water flowing through the cross-section in 10 seconds is 142 cubic meters.
How to calculate the approximate area of the cross-section using Simpson's rule?To calculate the approximate area of the cross-section using Simpson's rule, we divide the width of the river (12 meters) into intervals and consider the depth measurements at each interval. Given the depth measurements:
3, 3, 3, 3, 0.5, 2.3, 12.9, 2.1, 13.8We use Simpson's rule to estimate the area by applying the formula:
Area ≈ (Width/3) * [f(x₀) + 4f(x₁) + 2f(x₂) + 4f(x₃) + ... + 2f(xₙ₋₂) + 4f(xₙ₋₁) + f(xₙ)]Using the given depth measurements, we substitute them into the formula and calculate:
Area ≈ (12/3) * [3 + 4(3) + 2(0.5) + 4(2.3) + 2(12.9) + 4(2.1) + 13.8]Area ≈ 35.5 square metersAccording to the information, the approximate area of the cross-section is 35.5 square meters.
How to calculate the approximante volume of water flowing through the cross-section?To calculate the approximate volume of water flowing through the cross-section in 10 seconds, we multiply the area of the cross-section by the velocity of the river.
Given the velocity of the river is 0.4 meters per second, and the time is 10 seconds:
Volume ≈ Area * Velocity * TimeVolume ≈ 35.5 * 0.4 * 10Volume ≈ 142 cubic metersAccording to the information, the approximate volume of water flowing through the cross-section in 10 seconds is 142 cubic meters.
Learn more about Simpson's rule in: https://brainly.com/question/32151972
#SPJ1
28 randomly selected students took the calculus final. If the sample mean was 87 and the standard deviation was 11.7, find the margin of error for a 92% confidence interval for the mean score of all students. a. 4.15 b. 5.14 c. 5.41 d. 4.51
Answers
The margin of error for a 92% confidence interval is approximately 3.87.
To find the margin of error for a 92% confidence interval, we can use the formula:
Margin of Error = Z * (Standard Deviation / sqrt(n))
Where Z is the z-score corresponding to the desired confidence level, Standard Deviation is the standard deviation of the sample, and n is the sample size.
First, we need to find the z-score for a 92% confidence level. Since the confidence level is 92%, the alpha level (α) is 1 - 0.92 = 0.08. We divide this by 2 to get the tail area on each side, which is 0.04. Looking up this value in the standard normal distribution table, we find the z-score to be approximately 1.75.
Now, substituting the values into the formula:
Margin of Error = 1.75 * (11.7 / sqrt(28))
≈ 1.75 * (11.7 / 5.29)
≈ 1.75 * 2.21
≈ 3.87
Therefore, the margin of error for a 92% confidence interval is approximately 3.87.
None of the given answer options (a, b, c, d) match the calculated value of 3.87.
To more on statistics:
https://brainly.com/question/24298037
#SPJ8
Fatima is taking a test that is 45 minutes long.
She finishes the test at 10:50 a.m.
What time did she start the test?
Answers
Answer:10:05
Step-by-step explanation:
subtract 45 from 50!
Answer:
She started the test at 10:05 am
Step-by-step explanation:
Take the end time and subtract the time of the test
10:50-45 = 10:05
She started the test at 10:05 am
Reflect the figure over the line y = 1. Plot all of the points of the reflected figure. You may click a plotted point to delete it.
Answers
Answer:
Step-by-step explanation:
Isabella went to taco stand. She got 2 tacos and 4 drinks for $11.60. Lucas went to the same stand and got 3 tacos and 1 drink for $8.40.
1. Write an equation on how you can found out how much 1 taco and 1 drink is.
2. Solve
Answers
2x + 4y = 11.60
x represents the price of tacos and y represents the price of drinks
For Lucas:
3x + 1y = 8.40.
This is a systems of equation and can be solved by three methods. I’ll use the substitution method.
y = 8.40 - 3x … plug that into the top equation and solve for..
2x + 4(8.40-3x) = 11.60
.. simplify;
2x + 33.6 - 12x = 11.60
.. combine like terms;
-10x + 33.60 = 11.60
.. solve;
-10x = -19
.. solve;
divide by -10 on both sides of the equation..
-19/-10 = 1.9.. x = 1.9 ; this means that tacos cost $1.90. Let’s plug this into our original equations to figure out the price of each drink.
3(1.9)+y=8.40
.. simplify;
5.7 + y = 8.40
.. solve;
y = $2.70.. this means each drink costs $2.70.
We can work even further to double check our answers by plugging both numbers in the equations. Remember;
y = 2.70 (drinks)
x = 1.90 (tacos)
Imagine you have two sets of 8 blocks, each measuring 1 cm along each edge. You arrange one set into a large cube, 2 blocks × 2 blocks × 2 blocks. You arrange the other set into a straight beam, 1 block × 8 blocks. Compared with the beam, the large cube has.
Answers
Compared with the beam, the large cube has the same volume but a smaller surface area.
What is a rectangle?It is defined as two-dimensional geometry in which the angle between the adjacent sides is 90 degrees. It is a type of quadrilateral. The area occupied by the rectangle in two-dimensional planner geometry.
The area of a rectangle can be calculated using the following formula:
Rectangle area = length x width
Given that, there are two sets of eight blocks, each with an edge measurement of 1 cm. Two blocks are placed on top of two other blocks to form a big cube. You assemble the second set into a 1-by-8-block straight beam.
Since the volume is the same.
The surface area of a cube,
=6a²
=6(2)²
=6×4
=24 cm²
The surface area of a beam,
=1 × 8
=8 cm²
Thus, compared with the beam, the large cube has the same volume but a smaller surface area.
Learn more about the rectangle here:
https://brainly.com/question/15019502
#SPJ1
write 2x X(5y-y)/2 in a simplified form
Answers
Answer:
I'm not sure if you included an extra x so I'm including two answers with the work.
The answers are 4 x^ 2 y and 4 x y.
Step-by-step explanation:


Answer:
The answer is 4xy
Step-by-step explanation:
Rewrite the division as a fraction.
\(\frac{2x⋅(5y-y) }{2}\)
Cancel the common factor of 2.
x ⋅ (5y - y)
Subtract y from 5y.
x ⋅ (4y)
Rewrite using the commutative property of multiplication.
4xy
I hope this helps.
IM FAILING PLEASE HELP. Independent versus dependent variables You are walking directly away from your house You are 5 miles away from your house when you start walking, so you can determine your distance from your house by adding 5 to the number of miles you have walked. In the equation below. I represents the number of miles you have walked, and y represents your distance from home in miles The relationship between these two variables can be expressed by the following equation: y = + 5 Identify the dependent and independent variables. Dependent variable Independent variable Your distance from home Number of miles you walk
Answers
Answer:
Independent: I
Dependent: y
Step-by-step explanation:
The reason y is dependent is because it's depending on how many miles you have walked so far
Solve: | x-3 | + 2 = −3
Answers
|x−3|+2=−3|x-3|+2=-3
Move all terms not containing |x−3||x-3| to the right side of the equation.
|x−3|=−5
Remove the absolute value term. This creates a ±± on the right side of the equation because |x|=±x|x|=±x.
x−3=±(−5)
Set up the positive portion of the ± solution.
x−3=−5
Move all terms not containing x to the right side of the equation.
x= −2
Set up the negative portion of the ± solution.
x−3 =5
Move all terms not containing x to the right side of the equation.
x=8
The solution to the equation includes both the positive and negative portions of the solution.
x=−2, 8
for the linear equation y = 2x 4, if x increases by 1 point, how much will y increase? (hint: think about the definition of the slope) group of answer choices 2 points 3 points 1 points 4 points
Answers
For the linear equation y = 2x 4, if x increases by 1 pointThe value of y will increase by 2 points(A).
The equation y = 2x + 4 represents a linear relationship, where the coefficient of x, which is 2, represents the slope of the line. The slope indicates how much y changes when x increases by 1. In this case, the slope is 2, meaning that for every increase of 1 in x, y will increase by 2.
Therefore, For the linear equation y = 2x 4, if x increases by 1 point,the value of y will increase by 2 points(A). This means that correct option is A.
For more questions like Equation click the link below:
https://brainly.com/question/16663279
#SPJ11
Regenerate response
What is the slope of the line?
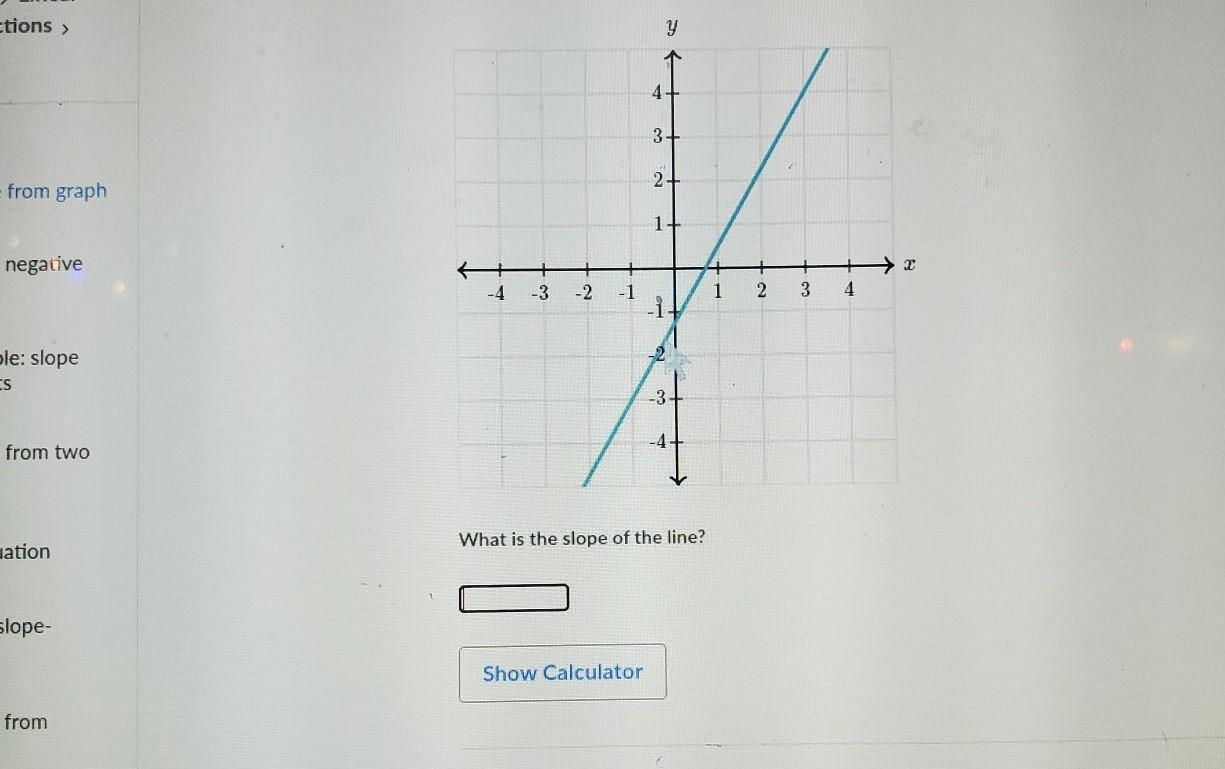
Answers
to get the slope of any straight line, we simply need two points off of it, let's use those two in the picture below.
\((\stackrel{x_1}{-1}~,~\stackrel{y_1}{-3})\qquad (\stackrel{x_2}{3}~,~\stackrel{y_2}{4}) ~\hfill \stackrel{slope}{m}\implies \cfrac{\stackrel{rise} {\stackrel{y_2}{4}-\stackrel{y1}{(-3)}}}{\underset{run} {\underset{x_2}{3}-\underset{x_1}{(-1)}}} \implies \cfrac{4 +3}{3 +1} \implies {\Large \begin{array}{llll} \cfrac{7 }{ 4 } \end{array}}\)

Question: What are all the values that a correlation r can possibly take? A. 0 ≤ r ≤ 1 B. r ≥0 C. -1 ≤ r ≤ 1 D. None of the above.
Answers
The correct answer is C. -1 ≤ r ≤ 1. The correlation coefficient, r, is a measure of the strength and direction of a linear relationship between two variables. It can take on any value between -1 and 1, inclusive.
A correlation coefficient of -1 indicates a perfect negative correlation, where as one variable increases, the other variable decreases in a perfectly linear fashion. A correlation coefficient of 0 indicates no linear relationship between the two variables. A correlation coefficient of 1 indicates a perfect positive correlation, where as one variable increases, the other variable increases in a perfectly linear fashion.
Therefore, statement A is not correct as it only includes the positive values of r. Statement B is not correct as it does not include negative values of r. Statement C is correct as it includes all possible values of r.
Learn more about correlation coefficient here brainly.com/question/15577278
#SPJ4
2- What is the cost to build a 3-on-3 court with an area of 165 square metres? The hoops and the paint will still be needed in the same quantities as the 5-on-5 court. However, the quantity of asphalt will be less. Complete your calculations and circle the final answer.
Answers
The cost to build a 3-on-3 court with an area of 165 square metres is $23,400.
What is circle ?
A circle is a two-dimensional closed shape in geometry that is defined as the set of all points in a plane that are equidistant from a fixed point called the center. The distance between the center and any point on the circle is called the radius.
To calculate the cost of building a 3-on-3 court with an area of 165 square meters, we need to consider the cost of the asphalt, hoops, and paint required for the court.
First, let's calculate the amount of asphalt needed. A 5-on-5 court typically requires around 200 square meters of asphalt. Since the 3-on-3 court is smaller, we can estimate that it will require approximately 75% of the asphalt needed for the 5-on-5 court:
75% x 200 square meters = 150 square meters of asphalt
Next, let's calculate the cost of the asphalt. The cost of asphalt varies depending on location, but we can estimate that it will cost around $150 per square meter:
150 square meters x $150 per square meter = $22,500 for asphalt
For the hoops and paint, we can assume that the quantities needed for the 3-on-3 court are the same as for the 5-on-5 court. The cost of a single basketball hoop is around $200, and the cost of paint for a basketball court is around $500.
So, the total cost to build a 3-on-3 court would be:
$22,500 for asphalt + $400 for hoops + $500 for paint = $23,400
Therefore, the cost to build a 3-on-3 court with an area of 165 square metres is $23,400.
To learn more about Circle from given link.
https://brainly.com/question/29142813
#SPJ1
Jessica plants a seed 3 1/2 inches below the ground. The plants grows from 15 3/4 inches . What is the height of the plant above ground?
Answers
Answer:
49/4 inches
Step-by-step explanation:
Inches below ground= 3 1/2 inches
Plant's full height=15 3/4 inches
Height above ground = 15 3/4 inches -3 1/2 inches
63/4 - 7/2
63-14/4
49/4 inches
the nutty professor sells cashews for $7.70 per pound and brazil nuts for $4.80 per pound. how much of each type should be used to make a 27 pound mixture that sells for $6.41 per pound?
Answers
The amount that each type would be 11.87 lbs of cashews and 15.13 lbs of brazil nuts
1. First, find the total cost of 27 lbs of the mixture: 27 lbs x $6.41/lb = $171.07.
2. Next, find the cost of cashews and brazil nuts in the mixture. Cashews cost $7.70/lb and brazil nuts cost $4.80/lb.
3. Subtract the cost of the brazil nuts from the total cost of the mixture: $171.07 - (27 lbs x $4.80/lb) = $105.27.
4. Divide the cost of the cashews ($105.27) by the cost of one pound of cashews ($7.70): $105.27/$7.70 = 13.66 lbs.
5. Subtract the number of pounds of cashews (13.66) from the total pounds of the mixture (27) to find the number of pounds of brazil nuts: 27 - 13.66 = 15.13 lbs.
6. Therefore, the mixture should contain 11.87 lbs of cashews and 15.13 lbs of brazil nuts.
See more about total cost at: https://brainly.com/question/5168855
#SPJ11
Point A=(7,9) is dilated from the origin by scale factor of r=11. what are the coordinates of point A
Answers
Answer:
42 and 54
Step-by-step explanation:
Express the area of the following shaded region in terms of (a) one or more integrals with respect to x, and (b) one or more integrals with respect to y
Answers
To express the area of the shaded region, set up one or more definite integrals using the given functions as limits of integration, either with respect to x or y.
To express the area of the shaded region in terms of one or more integrals, first identify the functions that bound the region. Let f(x) and g(x) be the functions bounding the region in the x direction, and h(y) and k(y) be the functions bounding it in the y direction.
(a) With respect to x: Area = ∫[g(x) - f(x)] dx, evaluated from a to b, where a and b are the limits of integration in the x direction.
(b) With respect to y: Area = ∫[k(y) - h(y)] dy, evaluated from c to d, where c and d are the limits of integration in the y direction.
To know more about integrals visit:
https://brainly.com/question/31433890
#SPJ11
If f(7) = 3, write a corresponding ordered-pair solution What is the corresponding ordered-pair?
Answers
An ordered-pair solution is a method of expressing the solution to an equation or system of equations using two numbers written in a specific order. The first number in the pair represents the input value, often denoted as x, and the second number represents the output value, often denoted as y.
The ordered pair is typically written as (x, y). This method is commonly used in algebra and graphing, where each ordered pair represents a point on a two-dimensional coordinate plane, and plotting multiple ordered pairs can help visualize a relationship or pattern between variables.
Since you're given that f(7) = 3, you can use this information to write a corresponding ordered-pair solution. An ordered pair consists of an input (x-value) and its corresponding output (y-value), written as (x, y).
In this case, the input is 7 and the output is 3, based on the given information f(7) = 3. Therefore, the corresponding ordered-pair solution is (7, 3).
The corresponding ordered-pair solution for f(7) = 3 is (7, 3).
To know more about ordered-pair solution visit:
https://brainly.com/question/23849657
#SPJ11
Please help I need help the question is in the picture

Answers
Answer:
z+x+y=180° because the angles in a triangle add up to 180.
For a recent paint job, Josh mixed red and white paint to make two different shades of pink. When the job was done, Josh ended up with leftover paint: 5 gallons of dark pink paint (80% red) and 4 gallons of light pink paint (30% red). Josh wants to make a medium pink color (50% red) to paint his daughter's bedroom. He will need 3 gallons to completely cover the walls. How much of each of the leftover paints should Josh mix to achieve his desired color?
? gallons of dark pink paint
? gallons of light pink paint
Answers
Josh should mix 1.2 gallons of dark pink paint and 1.8 gallons of light pink paint to achieve the desired medium pink color.
To find out how much of each leftover paint Josh should mix to achieve a medium pink color (50% red), we can set up a system of equations based on the percentages of red in the paints.
Let's assume that Josh needs x gallons of dark pink paint and y gallons of light pink paint to achieve the desired color.
The total amount of paint needed is 3 gallons, so we have the equation:
x + y = 3
The percentage of red in the dark pink paint is 80%, which means 80% of x gallons is red. Similarly, the percentage of red in the light pink paint is 30%, which means 30% of y gallons is red. Since Josh wants a 50% red mixture, we have the equation:
(80/100)x + (30/100)y = (50/100)(x + y)
Simplifying this equation, we get:
0.8x + 0.3y = 0.5(x + y)
Now, we can solve this system of equations to find the values of x and y.
Let's multiply both sides of the first equation by 0.3 to eliminate decimals:
0.3x + 0.3y = 0.3(3)
0.3x + 0.3y = 0.9
Now we can subtract the second equation from this equation:
(0.3x + 0.3y) - (0.8x + 0.3y) = 0.9 - 0.5(x + y)
-0.5x = 0.9 - 0.5x - 0.5y
Simplifying further, we have:
-0.5x = 0.9 - 0.5x - 0.5y
Now, rearrange the equation to isolate y:
0.5x - 0.5y = 0.9 - 0.5x
Next, divide through by -0.5:
x - y = -1.8 + x
Canceling out the x terms, we get:
-y = -1.8
Finally, solve for y:
y = 1.8
Substitute this value of y back into the first equation to solve for x:
x + 1.8 = 3
x = 3 - 1.8
x = 1.2
Therefore, Josh should mix 1.2 gallons of dark pink paint and 1.8 gallons of light pink paint to achieve the desired medium pink color.
for such more question on medium
https://brainly.com/question/14532771
#SPJ8
The radius of a circle is 2.2 feet. What is the length of an arc intercepted by an angle of pi/4 radians?
Answers
Answer: =2πr⋅(x360∘)
Step-by-step explanation:
PLS HELP ME I NEED THIS FASTTT ON SHOW ALL YOUR WORK
List the values for a, b and c in the following quadratic equation.
3x^2 + 6x = 7
Use your preferred method to solve the following quadratic equation for n. Please show ALL work to receive credit.
6n^2-18n -18=6
Use your preferred method to solve the following quadratic equation for n. Please show ALL work to receive credit.
x^2 + 8x = 10
Answers
Answer:
I tried my best so I hope this helps :)
(look at the screenshots for the work I don't feel like typing it out)
Step-by-step explanation:
1.) the solution is \(x=\frac{-3+\sqrt{30}}{3}\\\), \(x=-\frac{3+\sqrt{30}}{3}\)
2.) the solution is \(n=4,\:n=-1\)
3.) the solution is \(x=-4+\sqrt{26},\:x=-4-\sqrt{26}\)
Try using Symbolab, I use it all the time it gives the correct answer and it gives good explanations.



Dr. Garica is twice as old as his son. Twenty years ago he was 4 times as old as his son was then. How old are they now?
Answers
Answer:
Let's let g = Dr. Garcia's age and s = his son's age.
g = 2s, right?
20 years ago, Dr. Garcia was g - 20 years old. He was also 4(s - 20), 4 times his son's age 7 years ago.
g - 20 = 4(s - 20)
g - 20 = 4s - 80
In the first equation, we have g = 2s. We are going to substitute this g into the second equation.
2s - 20 = 4s - 80
60 = 2s
s = 30
So, his son is 30 and Mr. Garcia is 60. 30 x 2 = 60.
20 years ago, his son would have been 10 and Mr. Garcia would have been 40. 10 x 4 = 40.
Step-by-step explanation:
Brainliest?
Answer:
Dr. Garcia's age is 60, and his son's age is 30.
Step-by-step explanation:
Let x be Dr. Garcia's age, and y his son's age.
1. Dr. Garcia is twice as old as his son
x = 2 y
2. Twenty years ago, he was 4 times as old as his son was then
x - 20 = 4 (y - 20)
Substitute x in 2 and solve for y:
2 y - 20 = 4 (y - 20)
2 y - 20 = 4 y - 80
- 20 = 2 y - 80
60 = 2 y
y = 30
Put y back into 1:
x = 2 (30)
x = 60
So Dr. Garcia's age is 60, and his son's age is 30.
Use the Integral Test to determine the convergence or divergence of the following series, or state that the conditions of the test are not satisfied and, therefore, the test does not apply. k+ 7 Select the correct choice below and, if necessary, fill in the answer box to complete your choice. dx converges to the series also converges. Since the integral 7 e an exact answer.) OB. Since the integral dx diverges, the series also diverges. x 7 C. The Integral Test does not apply.
Answers
Using the Integral Test, the correct choice is B. Since the integral ∫(k+7)dx diverges, the series also diverges.
We are supposed to use Integral Test to determine the convergence or divergence of the series, ∑(k+7)dx.
We can use the Integral Test to test for the convergence of series if the function in the series is continuous, positive, and decreasing for all x greater than or equal to some value N.
The Integral Test states that a series converges if and only if the integral of the series term is convergent, i.e. if the integral of u(x)dx is convergent. Also, if the integral of u(x)dx diverges, the series is divergent.
So we need to check whether the function in the given series is continuous, positive, and decreasing for all x greater than or equal to some value N.
If we integrate the series, ∑(k+7)dx, we get:∫(k+7)dx= ∫k
dx + ∫7dx= (k²/2) + 7x+ C
where C is the constant of integration.
Since the value of C is not given, we cannot say anything about the exact value of the integral.
However, the value of the constant of integration does not matter in this case as we only need to determine whether the integral converges or diverges.
Therefore, we can use the Integral Test to determine the convergence or divergence of the series.
Using the Integral Test, the correct choice is B.
Since the integral ∫(k+7)dx diverges, the series also diverges.
Know more about Integral Test here:
https://brainly.com/question/31381575
#SPJ11
decreasing percent of change 78 to 24
Answers
The answer of the given question based on the percentage the answer is the percent of decrease from 78 to 24 is 69.23%.
What is Formula?A formula is mathematical expression that describes relationship between variables or values. It is used to calculate or derive specific result or value based on given inputs or conditions. Formulas can be simple or complex, depending on problem they are meant to solve
To find the percent of decrease from 78 to 24, we can use the formula:
percent decrease = [(original value - new value) / original value] x 100%
Plugging in the values we get:
percent decrease = [(78 - 24) / 78] x 100%
percent decrease = (54 / 78) x 100%
percent decrease = 0.6923 x 100%
percent decrease = 69.23%
Therefore, the percent of decrease from 78 to 24 is 69.23%.
To know more about Variable visit:
https://brainly.com/question/2466865
#SPJ1
How many solutions are there for the system of equations: -24x + 54y =153 and 16x - 36y = -102
Answers
The given system of equation has infinitely many solutions.
What is an equation?
An equation is a statement that shows that two expressions containing variables and/or numbers are equivalent. Equations are fundamentally questions, and the main motivations for the development of mathematics have been efforts to systematically find solutions to these problems.
We are given a system of equation as -24x + 54y = 153 and
16x - 36y = -102
On solving the first equation, we get
⇒ -24x + 54y = 153
⇒ -24x = 153 - 54y
⇒ x = \(\frac{9y}{4}\) - \(\frac{51}{8}\)
Now, on substituting this in the second equation, we get
⇒16 (\(\frac{9y}{4}\) - \(\frac{51}{8}\) ) - 36y = -102
⇒-102 = -102
⇒0 = 0
Hence, the given system of equation has infinitely many solutions.
Learn more about equation from the given link
https://brainly.com/question/25976025
#SPJ1
Unit 6 Final Test
Would appreciate some help as soon as its available (Has 3 parts) (ASAP)
1. The population of a town was 88 in 2016. The population quadruples every year. (a) Use the exponential growth model to write an equation that estimates the population t years after 2016. (b) Estimate the population of the town in 2023. Show your work. Answer:
2. Convert the following into a single log statement from the many log statements to 1. 7 Log x + 2log y – log 23 – 3 log z NOTE: You must show this in at least two steps. 1st line should be to convert the 2 the 3 and the 7 only. 2nd line can be the final answer. Answer:
3. A savings account is started with an initial deposit of $500. The account earns 7% interest compounded annually. (a) Write an equation to represent the amount of money in the account as a function of time in years. (b) Find the amount of time it takes for the account balance to reach 1 million. Show your work. Note: 1 million is a 1 with 6 zeros. Note2: you must use log functions to solve. Answer:
Answers
1. (a) An equation that estimates the population t years after 2016 is
P = \(88({4t)\).
b. The population of the town in 2023 is 2564.
2. log x + 2log y - log 23 - 3 log z is equal to log (x + y²)/log (23 + z³).
3. (a) An equation to represent the amount of money in the account as a function of time in years is A = 500(1 + 7/100)ⁿ.
(b) The amount of time it takes for the account balance to reach 1 million
is 112.5 years approximately.
What is the formula for exponential growth and exponential decaying function?The formula for exponential growth is \(y = y_0e^{(kt)}.\)
The formula for exponential decay is \(y = y_0e^{(-kt)}.\)
1. Given, The population of a town was 88 in 2016. The population quadruples every year.
Therefore, The exponential model of this situation is P = \(88({4t)\).
Now, From 2016 to 2023 it is 7 years.
Therefore, P = \(88({4\times7})\).
P = 2564.
2. Given, log x + 2log y - log 23 - 3 log z.
= log x + log y² - log 23 - log z³.
= log x + log y² - (log 23 + log z³).
= log (x + y²) - log (23 + z³).
= log (x + y²)/log (23 + z³).
3. Given, A savings account is started with an initial deposit of $500. The account earns 7% interest compounded annually.
We know the formula for compound interest is, A = P(1 + r/100)ⁿ.
a. A = 500(1 + 7/100)ⁿ.
b. 1000000 = 500(1 + 7/100)ⁿ.
2000 = (1 + 7/100)ⁿ.
2000 = 1.07ⁿ.
log_1.07 2000 = n.
n = 112.5 years approximately.
learn more about logarithms here :
https://brainly.com/question/30085872
#SPJ1