What is the value of the expression when n = 3?
-2 n(5 + n-8-3n)
12
24
42
ОО
54
Answers
Answer:
The value = 54
Step-by-step explanation:
–2n(5+n–8–3n) = –2(3)(5+3-8-3(3)) = –6(5+3-8-9)=–30–18+48+54=54
I hope I helped you^_^
Related Questions
Sarah Wiggum would like to make a single investment and have $1.7 million at the time of her retirement in 34 years. She has found a mutual fund that will earn 7 percent annually. How much will Sarah have to invest today
Answers
Answer:
Sarah has to invest $502,958.58 today.
Step-by-step explanation:
This is a simple interest problem.
The simple interest formula is given by:
\(E = P*I*t\)
In which E is the amount of interest earned, P is the principal(the initial amount of money), I is the interest rate(yearly, as a decimal) and t is the time.
After t years, the total amount of money is:
\(T = E + P\)
In this question:
\(t = 35, I = 0.07, T = 1700000\)
She has to invest P today.
\(T = E + P\)
\(1700000 = E + P\)
\(E = 1700000 - P\)
So
\(E = P*I*t\)
\(1700000 - P = P*0.07*34\)
\(3.38P = 1700000\)
\(P = \frac{1700000}{3.38}\)
\(P = 502958.58\)
Sarah has to invest $502,958.58 today.
Education: 3/2 t- 16 = 4/3 t - 6 How do I get that both variables stay at left side .
Answers
Answer:
60
Step-by-step explanation:
3/2t-16=4/3t-6
+16 +16
3/2t=4/3t+10
-4/3t
(t/6)6/1= 10(6/1)
t=60
Answer:
\(t=60\)
Step-by-step explanation:
To get both variables on one side, do the opposite of their current form and move them over (I'm not sure how else to explain this, so I'm just going to show you).
\(\frac{3}{2} t-16=\frac{4}{3} t-6\)
You see that the \(\frac{4}{3} t\) is positive, so you subtract it. The same goes for the -16 (do the opposite). You would add the -16 to the other side.
You need to do the opposite of them because only one number exists. If you move it, you need to cancel it out so that there is always only one of that number. (so when you add the -16 to the other side, the one on the left cancels out, but the same value (16) is still present on the right side and is added).
\(\frac{3}{2} t-\frac{4}{3} t=-6+16\)
Now you have this after moving like terms to their coinciding sides (you could do this any way, btw. It doesn't matter what order you add everything, so long as you get t on one side and by itself).
\(\frac{9}{6} t-\frac{8}{6}t =-6+16\\\frac{1}{6} t=10\\(\frac{6}{1} )(\frac{1}{6} t)=(\frac{6}{1} )(10)\\t=60\)
** Everything you do on one side, you need to do on the other side. This is a very important rule (and it goes hand in hand with the idea that there can only be one of each value, unless the exact same value appeared on both sides in the given equation).
What is the perimeter?
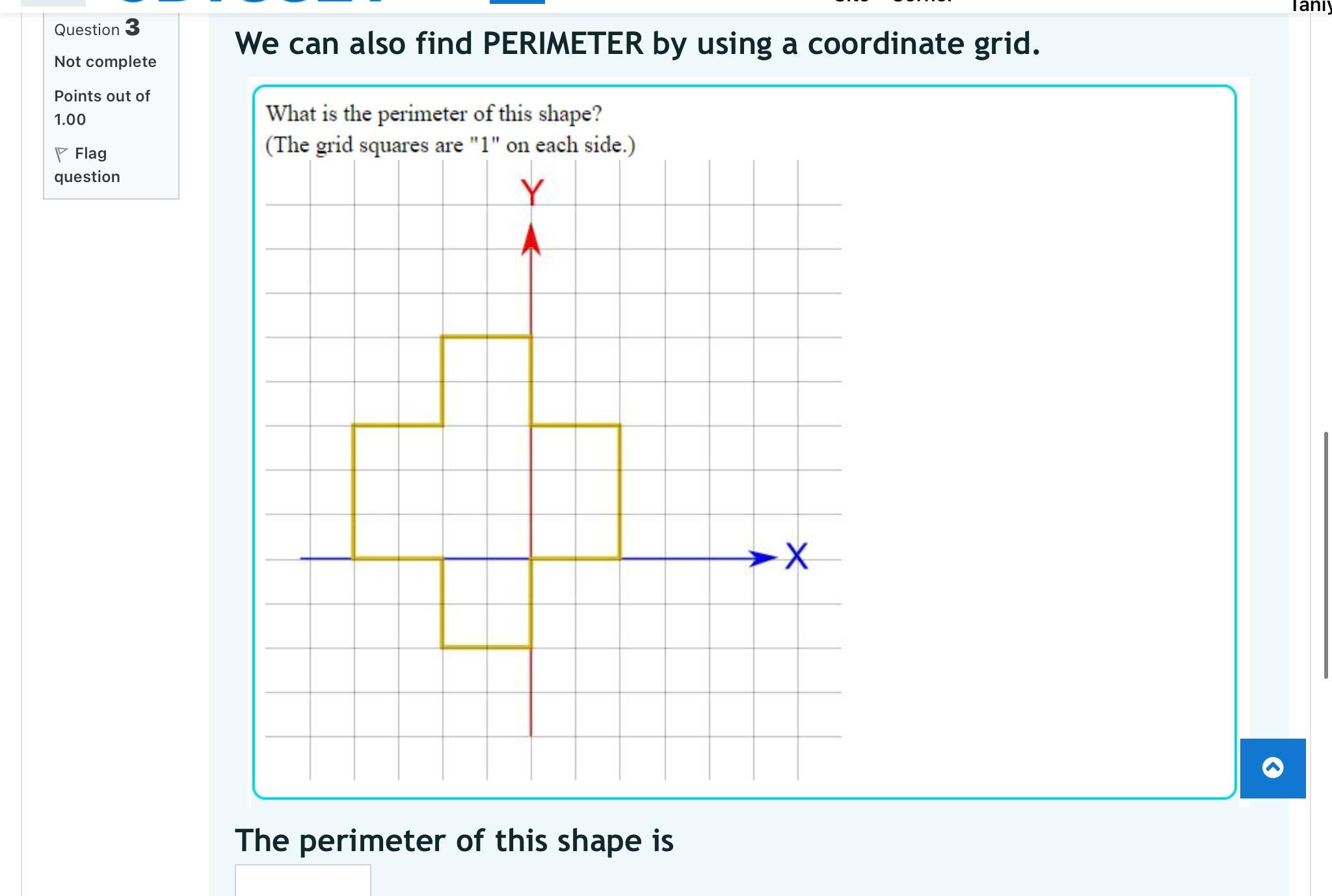
Answers
Answer:
26 (I think) :)
Step-by-step explanation:
i don’t know how to…
Answer:
26
Step-by-step explanation:
I think.
A squirrel is 1.1 feet tall. The squirrel shrinks to 1/10 of its height. How tall is the squirrel now? (This is an easy question for 10 points)
Answers
Answer:
the answer to the question is 0.11
yson does 75 sit-ups during his work out. He wants to increase his sit-ups by 15%. Which represents the new amount of sit-up
Answers
Answer:
he does 86 sit ups instead of 75 to increase by 15 percent
Step-by-step explanation:
Answer: The correct answer is 86.25 sit-ups (or round-up to 87 whole sit-ups).
Step-by-step explanation:
S = current amount of sit-ups (75)
S2 = Increased amount of sit-ups by 15%
The equation:
S2 = S + (S x .15)
S2 = 75 + (75 x .15)
S2 = 75 + 11.25
S2 = 86.25 sit-ups
Please answer this Calculus webwork question about differential equations:

Answers
Answer:
1.
\(p(t) = 2000({2}^{ \frac{t}{4} }) \)
2.
\(p(8) = 2000( {2}^{ \frac{8}{4} } ) = 2000( {2}^{2} ) = 2000(4) = 8000\)
3. p'(t) = 2,000(1/4)(2^(t/4))(ln 2)
= (500 ln 2)(2^(t/4))
p'(t) = 2,000 ln 2 cells/hour
= about 1,386 cells/hour
10: Write an equation for the relationship between time and distance for each horse. 11: At the given rates, how far would each horse run in 12 minutes?


Answers
We want to find the motion equation for the two horses and then use these equations to find how far would run each horse in 12 minutes.
The motion equations are:
For horse A: y = (1/4) mi/min*xFor horse B: y = (2/5) mi/min*xAnd in 12 minutes horse A moves 3 miles while horse B moves 4.8 miles
Finding the equations:
We can see that both equations pass through the point (0, 0), thus both equations are proportional equations of the form:
y = k*x
Then to get the equations we just need one point on each line, for example for horse A we can use the point (8 min, 2 mi)
Replacing that in the general equation we get:
2 mi = k*8min
Now we can solve that for k
(2 mi)/(8 min) = k
(1/4) mi/min = k
Notice that this is a speed.
For horse B we can use the point (5 min, 2 mi), in the same way than above we will get:
2 mi = k*5 min
(2mi/ 5 min) = k
(2/5) mi/min = k
Then the two equations are:
For horse A: y = (1/4) mi/min*xFor horse B: y = (2/5) mi/min*xEvaluating the equationsNow we want to see how far would each horse run in 12 minutes, we get this by evaluating bot equations in x = 12 min
For horse A we get:
y = (1/4) mi/min*12min = 3mi
For hose B we get:
y = (2/5) mi/min*12min = 4.8 mi
If you want to learn more about proportional relations, you can read:
https://brainly.com/question/12242745
Help me solve these two problems! Show the work

Answers
Answer:
see explanation
Step-by-step explanation:
2
4x² + 64 ← factor out the common factor of 4 from each term
= 4(x² + 16)
3
(a - 10)² = 121 ( take square root of both sides )
a - 10 = ± \(\sqrt{121}\) = ± 11 ( add 10 to both sides )
a = 10 ± 11
then
a = 10 - 11 = - 1
a = 10 + 11 = 21
In which quadrants is sine positive?
O A. I and IV
B. I and III
C. II and IV
D. I and II
Answers
Answer:
D. I and II
Step-by-step explanation:
In the first quadrant (I), all ratios are positive. In the second quadrant (II), sine (and cosec) are positive.
The solution is, sine is positive in, D. I and II. Option (D) is correct because, In the first quadrant (I), all ratios are positive. In the second quadrant (II), sine (and cosec) are positive.
What are trigonometric relations?Trigonometry is the study of the relationships between the angles and the lengths of the sides of triangles.
here, we have,
We are given to select the correct answer from the options provided.
The rule of signs for the trigonometric ratios in the four quadrants are as follows :
Quadrant I : All the three ratios, tangent, sine and cosine are positive.
Quadrant II : Only sine is positive, cosine and tangent are negative.
Quadrant III : Only tangent is positive, sine and cosine are negative.
Quadrant IV : Only cosine is positive, sine and tangent are negative.
so, we get,
In the first quadrant (I), all ratios are positive. In the second quadrant (II), sine (and cosec) are positive.
Therefore, using the above rules, we can say that the options (B), (C) and (A) are incorrect.
so, we have,
Option (D) is correct because, In the first quadrant (I), all ratios are positive. In the second quadrant (II), sine (and cosec) are positive.
To learn more about trigonometric relations click :
brainly.com/question/14450671
#SPJ7
Will Make Brianliest4−(2x+4)=5
Answers
Answer:
x=5 over 2 (it's a fraction)
Step-by-step explanation:
STEP 1 distribute : 4-1 (2x+4)=5
4-2x-4=5
STEP 2 subtract: 4-2x-4=5
-2x=5
STEP 3 divide both sides of the equation by the same term:
-2x=5
-2x 5
----- ------
-2 -2
STEP 4 simplify:
cancel the terms that are both the numerator and denominator
divide the numbers
x= - 5 over 2 (fraction)
I hope this helps
Sketch a graph of f(x)={- 5 if x < -2 2x-1 if-2 < x≤ 2 0 if x>2. (piecewise)
Answers
A graph of the given piecewise-defined function is shown in the image below.
What is a piecewise-defined function?In Mathematics and Geometry, a piecewise-defined function simply refers to a type of function that is defined by two (2) or more mathematical expressions over a specific domain.
Generally speaking, the domain of any piecewise-defined function simply refers to the union of all of its sub-domains. By critically observing the graph of this piecewise-defined function, we can reasonably infer and logically deduce that it is constant over several intervals or domains such as x > 2 and x < -2.
In conclusion, this piecewise-defined function has a removable discontinuity.
Read more on piecewise function here: brainly.com/question/18670055
#SPJ1

x + 2y > 15 solution or not solution
Answers
The solution for the equations is -5 and -10.
What is an equation?An equation contains one or more terms with variables connected by an equal sign and we can find the solutions using the equation or solving for the variables.
Example:
34x + 4y = 9 is an equation.
2x = 8 is an equation.
We have,
-x + 2y = -15 _____(1)
8x - 2y = -20 ______(2)
Using the substitution method.
From (1),
2y = -15 + x ______(3)
Substituting in (2),
8x - 2y = -20
8x - (-15 + x) = - 20
8x + 15 - x = - 20
7x + 15 = - 20
7x = -20 - 15
7x = -35
x = -5
And,
Substituting x = -5 in (3),
2y = -15 + x
2y = -15 - 5
2y = -20
y = -10
Thus,
The solution for the equations is -5 and -10.
Learn more about equations here:
https://brainly.com/question/17194269
#SPJ9
The complete question.
Find the solutions for the equations.
-x + 2y = -15
8x - 2y = -20
There are 800 pupils at Stoke College in Years 7, 8 & 9 in total. There are 27% of them
in Year 7,38% in Year 8. How many pupils are in Year 9?
Answers
Answer:
280 pupils
Step-by-step explanation:
27 + 38 + x = 100 Find what percentage is the Year 9 which is 35%
800 x 0.35 = 280 pupils
Use the rational zero theorem to list all possible rational zero's for the polynomial function: f(x)=2x^3+3x^2-8x+5 To earn full credit please share all work, calculations and thinking. If you prefer you can do the work by hand on a piece of paper, take a picture of that work and upload it.

Answers
The given polynomial function is:
\(f(x)=2x^3+3x^2-8x+5\)Since all the co-efficients are integers, we can apply the rational zero theorem.
The trailing co-efficient ( the co-efficient of the constant term) is 5.
Find its factors with the plus and minus sign; thus we have;
\(\begin{gathered} \text{Factors}=\pm1,\pm5 \\ \text{These are the possible values for p} \end{gathered}\)The leading co-efficient ( the co-efficient of the term with the highest degree) is 2.
Find its factors with the plus and minus sign; thus we have:
\(\begin{gathered} \text{Factors}=\pm1,\pm2 \\ \text{These are the }possible\text{ values for q} \end{gathered}\)Next, is finding all possible values for the rational expression p/q. Thus, we have:
\(\begin{gathered} \frac{p}{q}=\pm\frac{1}{1},\pm\frac{1}{2},\pm\frac{5}{1},\pm\frac{5}{2} \\ \frac{p}{q}=\pm1,\pm\frac{1}{2},\pm5,\pm\frac{5}{2} \end{gathered}\)Hence, the possible rational zeros for the polynomial function are:
\(\pm1,\pm\frac{1}{2},\pm5,\pm\frac{5}{2}\)what is the fraction if the denominator is 8
Answers
Step-by-step explanation:
1/8, 2/8, 3/8, 4/8, etc...
if i use 2 dozens of roses to make 5 bouquets, how many roses do i need to make 20 bouquets
Answers
Answer:
8
Step-by-step explanation:
2:5 dozens of roses : bouquets
x : 20
20/5 = 4
2 x 4 = 8. multiply the known dozens of roses (2) by the multiplier (4)
i nee d some help pls

Answers
Answer:
x = 7
Step-by-step explanation:
4x - 9 = 2x + 5
2x - 9 = 5
2x = 14
x = 7
IT IS 2!!!!!!!!!!!!!!!!
Which of the following is true for the function f(x)=2cos(x2)
a. The period is π
b. The period is π2
c. The period is 2π
d. The period is 4π
e. The period is 2
Answers
Answer:
c. The period is 2π
Step-by-step explanation:
The period of a function is the smallest value of $p$ for which\( f(x+p) = f(x)\) for all x.
For the function f(x) = 2cos(x^2), we can see that f(x+2π) = f(x) for all x.
This is because the cosine function has a period of 2π. Therefore, the period of f(x) = 2cos(x^2) is \(\boxed{2\pi}\)
PLEASE HELP WILL GIVE BRAINLIEST
The number of automobiles in a certain town was 1,890 in 2015, and it was 2,420 in 2020. If we were to make a linear model that gives the number of automobiles in this town as a function of the number of years since 2015, what would be the y-intercept?
Answers
The y-intercept of the linear model is 211,400, which represents the estimated number of automobiles in the town in the year 2015 (when the independent variable is zero).
To find the y-intercept of the linear model, we need to determine the value of the dependent variable (the number of automobiles) when the independent variable (the number of years since 2015) is equal to zero.
Let's first find the slope of the line, which represents the rate of change of the number of automobiles per year:
slope = (change in number of automobiles) / (change in number of years)
slope = (2420 - 1890) / (2020 - 2015) = 106 automobiles per year
Now we can use the point-slope form of a linear equation to find the y-intercept:
y - y1 = m(x - x1)
where y1 is the value of the dependent variable when the independent variable is x1. In this case, x1 = 2015, y1 = 1890, and m = 106 (the slope we just calculated).
y - 1890 = 106(x - 2015)
To find the y-intercept, we can set x = 0:
y - 1890 = 106(0 - 2015)
y - 1890 = -213,290
y = 211,400
for such more question on linear model
https://brainly.com/question/9753782
#SPJ11
When an object is weighed on a scale, the number displayed may vary from the object’s actual weight by at most 0.4 pounds. The scale says the object weighs 125.8 pounds. Part A: Write an absolute value inequality that describes the range of the actual weight of the object. Use the variable w to represent the actual weight of the object. Part B: Solve the absolute value inequality for w. Express your answer as a compound inequality.
Answers
The compound inequality that represents the range of the actual weight of the object is 125.4 ≤ w ≤ 126.2.
Part A: The absolute value inequality that describes the range of the actual weight of the object is:
|w - 125.8| ≤ 0.4
Part B: To solve the absolute value inequality, we can break it down into two separate inequalities:
w - 125.8 ≤ 0.4 and - (w - 125.8) ≤ 0.4
Solving the first inequality:
w - 125.8 ≤ 0.4
Add 125.8 to both sides:
w ≤ 126.2
Solving the second inequality:
-(w - 125.8) ≤ 0.4
Multiply by -1 and distribute the negative sign:
-w + 125.8 ≤ 0.4
Subtract 125.8 from both sides:
-w ≤ -125.4
Divide by -1 (note that the inequality direction flips):
w ≥ 125.4
Combining the solutions, we have:
125.4 ≤ w ≤ 126.2
The object is 125.4 ≤ w ≤ 126.2.
for more questions on compound
https://brainly.com/question/3989769
#SPJ8
Will mark brainliest
Worth 50 points I need help with this practice test.

Answers
Answer:
first question answer: \(\frac{1}{12}\)
second question answer: commutative property of multiplication
Answer:
\( \boxed{ \bold{ \boxed{ \sf{ \: 1. \: \: \: \: \: \frac{1}{12} }}}}\)
\( \boxed{ \bold{ \boxed{ \sf{2. \: \: \: \: associative \: property \: of \: multiplication}}}}\)
Step-by-step explanation:
1. \( \sf{ \sqrt{ \frac{1}{144} } }\)
⇒\( \sf{ \sqrt{ \frac{ {1}^{2} }{ {12}^{2} } } }\)
⇒\( \sf{ \sqrt{ { (\frac{1}{12}) }^{2} } }\)
⇒\( \sf{ \frac{1}{12} }\)
2. Associative property of multiplication
It states that the product of three or more integers remains unchanged even if they are grouped in different orders.
Hope i helped !
Best regards! :D
I don’t really understand this

Answers
A store is having a sale on jelly beans and almonds. For 3 pounds of jelly beans and 5 pounds of almonds, the total cost is $27. For 9 pounds of jelly beans and
7 pounds of almonds, the total cost is $51. Find the cost for each pound of jelly beans and each pound of almonds.
Cost for each pound of jelly beans:
Cost for each pound of almonds:
Answers
Answer:
Cost for each pound of jelly beans: $2.75
Cost for each pound of almonds: $3.75
Step-by-step explanation:
Let J be the cost of one pound of jelly beans.
Let A be the cost of one pound of almonds.
Using the given information, we can create a system of equations.
Given 3 pounds of jelly beans and 5 pounds of almonds cost $27:
\(\implies 3J + 5A = 27\)
Given 9 pounds of jelly beans and 7 pounds of almonds cost $51:
\(\implies 9J + 7A = 51\)
Therefore, the system of equations is:
\(\begin{cases}3J+5A=27\\9J+7A=51\end{cases}\)
To solve the system of equations, multiply the first equation by 3 to create a third equation:
\(3J \cdot 3+5A \cdot 3=27 \cdot 3\)
\(9J+15A=81\)
Subtract the second equation from the third equation to eliminate the J term.
\(\begin{array}{crcrcl}&9J & + & 15A & = & 81\\\vphantom{\dfrac12}- & (9J & + & 7A & = & 51)\\\cline{2-6}\vphantom{\dfrac12} &&&8A&=&30\end{array}\)
Solve the equation for A by dividing both sides by 8:
\(\dfrac{8A}{8}=\dfrac{30}{8}\)
\(A=3.75\)
Therefore, the cost of one pound of almonds is $3.75.
Now that we know the cost of one pound of almonds, we can substitute this value into one of the original equations to solve for J.
Using the first equation:
\(3J+5(3.75)=27\)
\(3J+18.75=27\)
\(3J+18.75-18/75=27-18.75\)
\(3J=8.25\)
\(\dfrac{3J}{3}=\dfrac{8.25}{3}\)
\(J=2.75\)
Therefore, the cost of one pound of jelly beans is $2.75.
A rose garden is formed by joining a rectangle and a semicircle, as shown below. The rectangle is 29ft long and 18ft wide. If the gardener wants to build a fence around the garden, how many feet of fence are required? Use the value 3.14 for, and do not round your answer. Be sure to include the correct unit in your answer
Answers
Answer:
The area of the garden is
797
f
t
2
.
Explanation:
First, let's find the area of the rectangle.
We know that the area of a rectangle is the length times width, or
20
⋅
32
in this example:
20
⋅
32
=
640
So the area of the rectangle is
640
f
t
2
.
You may not know the area of a semicircle, but that's fine
−
we know the area of a circle, or
π
r
2
.
Since the area of a semicircle is half the area of a circle, we just do the area of the circle divided by
2
:
So the area of a semicircle is
π
r
2
2
.
The question asks for
π
to just be
3.14
, so instead the equation is
A
=
3.14
r
2
2
The picture gives the diameter of the circle.
To find the radius, or
r
, we divide the diameter by
2
:
20
2
=
10
Now we can solve for the area of the semicircle:
3.14
(
10
)
2
2
3.14
(
100
)
2
314
2
157
f
t
2
Now that we now the areas of the rectangle and semicircle, we can add them up to find the area of the rose garden:
640
+
157
=
797
f
t
2
Find the equation of the line perpendicular to y=1/2x-1 and passes through the point (5,6)
Answers
The equation of the line perpendicular to y=1/2x-1 and passing through the point (5,6) is y = -2x + 16.
To find the equation of the line perpendicular to y=1/2x-1, we need to determine its slope. We can recall that the slope of a line in slope-intercept form, y = mx + b, is given by m, the coefficient of x. So in the given equation y=1/2x-1, the slope is 1/2.
The slope of a line perpendicular to this line will be the negative reciprocal of the slope, which means it will be -2. To see why this is true, remember that the product of the slopes of two perpendicular lines is always -1. So if the slope of the original line is m, the slope of the perpendicular line will be -1/m.
Now that we know the slope of the perpendicular line is -2, we can use the point-slope form of the equation of a line to find its equation. The point-slope form is:
y - y1 = m(x - x1)
where m is the slope and (x1, y1) is a point on the line.
We are given a point on the perpendicular line, (5, 6), so we can substitute that into the equation and also substitute in the slope, -2:
y - 6 = -2(x - 5)
y - 6 = -2x + 10
y = -2x + 16
Hence, the equation of the line perpendicular to y=1/2x-1 and passing through the point (5,6) is y = -2x + 16.
To learn more about Line of Perpendicular and Slope of a line, refer to the following answers:
https://brainly.com/question/25842670
https://brainly.com/question/30422569
PLS HELP! weights (in pounds) of catfish caught in the river: 4.8 3 2.7 4.4 4.8 9.9 What is the outlier? A) 3 lbs B) 4.8 lbs C) 9.9 lbs D) none
Answers
The correct answer is C) 9.9 lbs as it is above the upper boundary 6.4865, it is the outlier in this dataset.
What is an outlier?An outlier is an observation that is much higher or lower than the other observations in a dataset.
In this case, the weights of the catfish range from 2.7 to 4.8 lbs, with one observation that is much higher at 9.9 lbs.
Therefore, 9.9 lbs is the outlier in this dataset.
To find the outlier in this dataset, we can calculate the interquartile range (IQR).
This is done by first calculating the first quartile (Q1) and third quartile (Q3).
The Q1 for this dataset =3.75
and the Q3= 4.675.
IQR= Q3 - Q1
= 0.925.
We then calculate the lower boundary as Q1 - (1.5 x IQR) = 2.3625.
The upper boundary is Q3 + (1.5 x IQR)= 6.4865.
Since 9.9 lbs is above this upper boundary, it is the outlier in this dataset.
For more questions related to upper boundary
https://brainly.com/question/28725724
#SPJ1
Holly drew the parallelogram below to represent the design of her new garden. A parallelogram with base b and height h. She found that the area of the garden will be 127 and one-half square feet by using the equation Area = b h. If the height, h, of the parallelogram-shaped garden is 8 and one-half feet, what is the base, b, in feet? 1.5 7.5 15 75
Answers
Answer: i just took the quiz it is 15
Step-by-step explanation:
Answer:
The answer is 15
Step-by-step explanation:
She found that the area of the garden will be 127 and one-half square feet by using the equation Area = b h. If the height, h, of the parallelogram-shaped garden is 8 and one-half feet, what is the base, b, in feet? Hmm so it says that base times height equals 127 and one half square feet and the height is 8 and one half feet so you guessed it you have to divided 127 and one half square feet by 8 and one half feet which is 15.
Hope this helped for you understanding how to do this problem have a great day!
Calc II Question
The base of s is an elliptical region with boundary curve 9x^2 + 4y^2 = 36
Cross sections perpendicular to the x axis are isoscelees right triangle with hypotension in the base.
Correct answer is 24 but I don't know how they go that
Answers
Answer:
See below for explanation
Step-by-step explanation:
The area of an isosceles triangle is \(A=\frac{1}{2}bh\), so let's write the base as an equation of y:
\(\displaystyle 9x^2+4y^2=36\\\\4y^2=36-9x^2\\\\y^2=9-\frac{9}{4}x^2\\\\y=\pm\sqrt{9-\frac{9}{4}x^2\)
As you can see, our ellipse consists of two parts, so the hypotenuse of each cross-section will be \(\displaystyle 2\sqrt{9-\frac{9}{4}x^2\), and each height will be \(\displaystyle \sqrt{9-\frac{9}{4}x^2}\).
Hence, the area function for our cross-sections are:
\(\displaystyle A(x)=\frac{1}{2}bh=\frac{1}{2}\cdot2\sqrt{9-\frac{9}{4}x^2}\cdot\sqrt{9-\frac{9}{4}x^2}=9-\frac{9}{4}x^2\)
Since we'll be integrating with respect to x because the cross-sections are perpendicular to the x-axis, then our bounds will be from -2 to 2 to find the volume:
\(\displaystyle V=\int^2_{-2}\biggr(9-\frac{9}{4}x^2\biggr)\,dx\\\\V=9x-\frac{3}{4}x^3\biggr|^2_{-2}\\\\V=\biggr(9(2)-\frac{3}{4}(2)^3\biggr)-\biggr(9(-2)-\frac{3}{4}(-2)^3\biggr)\\\\V=\biggr(18-\frac{3}{4}(8)\biggr)-\biggr(-18-\frac{3}{4}(-8)\biggr)\\\\V=(18-6)-(-18+6)\\\\V=12-(-12)\\\\V=12+12\\\\V=24\)
Therefore, this explanation confirms that the correct volume is 24!
Which algebraic expression is equivalent to the expression below
5(4x + 5) - 17
A.
20x + 9
B.
5x + 9
C.
20x + 42
D.
20x + 8
Answers
Answer:
20x + 8
Step-by-step explanation:
5*4x + 5*5 -17
20x +25 -17
20x + 8
When the price ,p, charged for a boat tour was $25, the avg number of people per week ,n, was 500. when the price was reduced to $20, the avg numbers of customers per week increased to 650. >Find a formula for the demand curve, assuming that it is linear
Answers
For every 25 customers below 650, the price charged must be decreased by $1.
What is price?Price is the value of a product or service that an individual or business is willing to accept in exchange for a good or service. It is typically determined by the market forces of supply and demand. Prices are usually expressed in monetary terms, but can also be expressed in terms of labor time or other resources. Prices can vary widely depending on the availability of a good or service, the amount of competition, and other factors.
The demand curve can be found using the following equation:
p = (n-650)/25 + 20
Where p is the price charged for the boat tour and n is the average number of customers per week.
This equation can be interpreted as follows: for every 25 customers per week above 650 (the number of customers when the price was reduced to $20), the price charged for a boat tour must be increased by $1. Similarly, for every 25 customers below 650, the price charged must be decreased by $1.
To know more about price click-
https://brainly.com/question/19104371
#SPJ1