Answers
Answer:
12.5 %
Step-by-step explanation:
There are 8 total sections, and only one of them is yellow. So if we spun it eight times, the possibility of getting yellow is one time. As a result, 1/8 is 12.5%
Related Questions
Find the area of this figure.

Answers
Answer:
I think it's 16 tell me if I am wrong
Answer:
23.5 in²
Step-by-step explanation:
act like the whole triangle is there (9x7)
then us the triangle rule 1/2(b times h)
9x7=63/2=31.5
then subtract the missing part from the total area
31.5-(2x4)
31.5-8
23.5
hope this helps have a blessed day
What does the net decimal equivalent (NDE) represent?
Answers
Answer:
the product of the decimal equivalents of all discount in the series
I will mark brainliest!!
Answer choices are:
A. a 180 degrees clockwise rotation about the origin
B. a dilation with a scale factor of 6
C. a 90 degrees clockwise rotation about the origin
D. a reflection over the line x=6

Answers
Answer:
D
Step-by-step explanation:
My mom is a teacher lol
Which graph is defined by the function given below?
y= (x+3)(x +3)
-10
-10+
10-
A
B.
C
D.

Answers
Step-by-step explanation:
Since y = (x + 3)(x + 3), the root is x = -3.
Only Graph A touches the x-axis once.
Given: f(x) = 1/x+5 and g(x) = x - 2.
What are the restrictiorts of the domain of f(g(x))?
0 x4 -5
O X-3
OX= 2
There are no restrictions.
Answers
Answer:
The restriction of the domain of f(g(x)) is that x cannot be 3.
Step-by-step explanation:
The first step to solve this question is finding the function, replacing the values. So
\(f(x) = \frac{1}{x+5}\)
\(g(x) = x - 2\)
\(f(g(x)) = f(x - 2) = \frac{1}{x - 2 + 5} = \frac{1}{x - 3}\)
The function is a fraction, which means that the restriction is that the denominator cannot be zero. So
\(x - 3 \neq 0\)
\(x \neq 3\)
The restriction of the domain of f(g(x)) is that x cannot be 3.
Ravi says,” if 10 years are added to my age, then my age becomes 25.”if Ravi’s present age is x years,
then find the linear equation satisfying the given condition.
Answers
Answer:
The linear equation satisfying the given condition is,
\(x=t+15\)
where x is Ravi's age and t is the number of years
(after 10 years (t=10) his age becomes 25)
Step-by-step explanation:
Let x be Ravi's age
Now, his current age is unknown, but if you add 10 to it, then it becomes 25, so
x + 10 =25
so, his current age is x = 25 =10
x = 15
Now, we find the linear equation satisfying the given condition,
Let t be the number of years that have passed since the present time
so, if 0 years have passed, his age will be 15 years
after 1 year, his age will be 16 years
after 10 years, his age will be 25 years and so on
so
\(x = t + 15\)
this satisfies the given condition, since if we add ten years i.e t = 10,
then we get 25
The equation is:
⇨ x + 10 = 25Work/explanation:
Here's the linear equation for Ravi's age.
Ravi's age is x.
If you add 10 to x you get x + 10.
That gives 25.
So the linear equation is x + 10 = 25.
Which is the last operation preformed when evaluating (8-2x)²+4 for x=3
A addition
B multiplication
C subtraction
D applying the exponent
Please hurry
Answers
Answer: I think its c sorry if im whrong
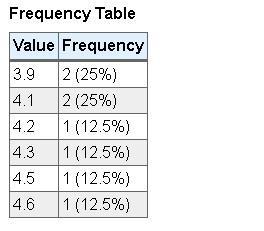
Please help withstandard deviation with measurements

Answers
Answer: Standard Deviation, σ: 0.23979157616564
Count, N: 8
Sum, Σx: 33.6
Mean, μ: 4.2
Variance, σ2: 0.0575
Steps
σ2 =
Σ(xi - μ)2
N
=
(4.5 - 4.2)2 + ... + (4.1 - 4.2)2
8
=
0.46
8
= 0.0575
σ = √0.0575
= 0.23979157616564
Step-by-step explanation: but here
it isnt letting me do the fraction or the other things so yeah it will look wrong but its write for the entire graph

mart math math math math

Answers
Explanation:
Segment AW bisects angle CAD.
This leads to the smaller pieces (angles CAW and DAW) to be equal to one another. Both are 20 degrees each. That totals to 20+20 = 40 degrees.
Therefore, angle CAD = 40 degrees.
The supplement of this is angle DAX
(angle CAD) + (angle DAX) = 180
angle DAX = 180 - (angle CAD)
angle DAX = 180 - 40
angle DAX = 140 degrees
A student graphed an ordered pair on a coordinate plane that was located on the y axis and was 5 units above the origin. Write the ordered pair that the student graphed with these characteristics.
Answers
If you want to graph a linear equation you have to have at least two points, but it's usually a good idea to use more than two points. When choosing your points try to include both positive and negative values as well as zero.
Example
Graft the function y = x + 2
Begin by choosing a couple of values for x e.g. -2, -1, 0, 1 and 2 and calculate the corresponding y values.
X Y = x + 2 Ordered pair
-2 -2 + 2 = 0 (-2, 0)
-1 -1 + 2 = 1 (-1, 1)
0 0 + 2 = 2 (0, 2)
1 1 + 2 = 3 (1, 3)
2 2 + 2 = 4 (2, 4)
Now you can just plot the five ordered pairs in the coordinate plane
picture13
At the moment this is an example of a discrete function. A discrete function consists of isolated points.
By drawing a line through all points and while extending the line in both directions we get the opposite of a discrete function, a continuous function, which has an unbroken graph.
picture14
If you only want to use two points to determine your line you can use the two points where the graph crosses the axes. The point in which the graph crosses the x-axis is called the x-intercept and the point in which the graph crosses the y-axis is called the y-intercept. The x-intercept is found by finding the value of x when y = 0, (x, 0), and the y-intercept is found by finding the value of y when x = 0, (0, y).
picture15
The standard form of a linear equation is
Ax+By=C,A,B≠0
Before you can graph a linear equation in its standard form you first have to solve the equation for y.
2y−4x=8
2y−4x+4x=8+4x
2y=4x+8
2y2=4x2+82
y=2x+4
From here you can graph the equation as we did in the example above.
The graph of y = a is a horizontal line where the line passes through the point (0, a)
picture16
Whereas the graph of x = a is a vertical line that passes through the point (a, 0)
picture17
I just need the coordinates and I'll draw the graphs.
Please answer this question it would mean so much to me.. I'm desperate.. .thank you so much... will give you brainliest!! 25 points !!

Answers
Answer:
Step-by-step explanation:


Answer:
A) The first equation has points of.... (0,5) and (5,-5)
B) The second equation has points of... (0,3) and (3, -1)
Step-by-step explanation:
If you need further explanation don't hesitate to ask :)
Please help with these math questions I really need it ! Don't just do it for points pls



Answers
Answer:
wouldn't that be 81 but i dont know
Answer:
1. d 2. 43.5 3. 817.5
Step-by-step explanation:
Simplify (w3)4•(w5)2
Answers
Answer:
\(w^{22}\)
Step-by-step explanation:
\((w^3)^4\cdot(w^5)^2=w^{3*4}\cdot w^{5*2}=w^{12}\cdot w^{10}=w^{12+10}=w^{22}\)
Mrs Loke had 2920 buttons. She packed them into packets of 8 buttons. (a) How many packets of 8 buttons did she pack? (b) If she were to pack all her buttons into packets of 5 buttons, how many fewer packets of 8 buttons than 5 buttons would she get?
Answers
Alex is planning to surround his pool ABCD with a single line of tiles. How many units of tile will he need to surround his pool? Round your answer to the nearest hundredth.
Answers
Answer:
18.97 units
Step-by-step explanation:
Assume that the pool looks like the diagram below.
The pool consists of two equal right triangles.
We know that AB = CD = 3.16.
1. Find the length of the pool
AD = BC, and we can use Pythagoras' Theorem to calculate AD.
AB² + AD² = BD²
3.16² + AD² = 7.07²
9.986 + AD² = 48.98
AD² = 48.98 - 9.986 = 40.00
AD = 6.324
2. Find the perimeter of the pool
P = AB + BC + CD + DA =3.16 + 6.324 + 3.16 + 6.324 = 18.97
Alex will need 18.97 units of tile to surround the pool.

Alex will need 13.42 units of tile to surround his pool.
A coordinate plane with quadrilateral ABCD at A (0,3), B (2,4), C (4,0), and D (2,-1).
How to find the boundary of the pool?To find the units of tiles that are needed to surround the pool ABCD with a single line of tiles is equal to the perimeter of the rectangle
Perimeter of the rectangle = 2 length+2 width
In the figure, the width is given as 2.24 units and the diagonal is 5 units.
So we can find the length using the Pythagorean theorem, which is c²=a²+b².
Now, 5²=(2.24)²+b²
b=4.47 units
Now, the perimeter of the pool will be 2 (4.47)+2 (2.24)
= 8.94 + 4.48
The perimeter of the rectangle = 13.42 units
Thus, Alex will need 13.42 units of tile to surround his pool.
To learn more about the perimeter of the rectangle visit:
https://brainly.com/question/15287805.
#SPJ5

1/4 times 2/2 PLS HURRY
Answers
Answer:
answer is 2/8 or simplified is still 1/4
Step-by-step explanation:
1/4
STEP BY STEP:
look at image
just remember that 2/8 can reduce to your answer, 1/4

A coin-operated machine sells plastic rings. It contains 5 white rings, 6 orange rings, 7 purple rings, and 15 pink rings. Constance puts a coin into the machine. Find the theoretical probability she gets a orange ring. Express your answer as a decimal. If necessary, round your answer to the nearest thousandth.
Answers
The theoretical probability of Constance getting an orange ring from the coin-operated machine is 0.182. This means that out of all the possible outcomes, there is an 18.2% chance that Constance will get an orange ring.
To find the theoretical probability of Constance getting an orange ring from the coin-operated machine, we need to first understand what theoretical probability means. Theoretical probability is the likelihood or chance of an event happening based on the number of possible outcomes. In this case, the possible outcomes are the different colored rings that the machine contains.
We are given that the machine contains 5 white rings, 6 orange rings, 7 purple rings, and 15 pink rings.
Therefore, the total number of rings in the machine is 5 + 6 + 7 + 15 = 33.
The probability of Constance getting an orange ring can be calculated by dividing the number of orange rings by the total number of rings in the machine.
Therefore, the theoretical probability of Constance getting an orange ring is:
6 / 33 = 0.181818...
To express this as a decimal, we can round it to the nearest thousandth, which is 0.182.
know more about theoretical probability here:
https://brainly.com/question/22962752
#SPJ11
Does anybody know this? Solve the system of equation

Answers
The solution to the given system of equations is x = -6, y = 5, and z = 3. That is, the solution is (-6, 5, 3)
Solving system of equationsFrom the question, we are to solve the given system of equations
The given system of equation is
x - 2y + 3z = -7 ------------ (1)
4x + 5y + z = 4 ------------ (2)
-x + y - 2z = 5 ------------ (3)
Add equations (1) and (3)
x - 2y + 3z = -7
-x + y - 2z = 5
--------------------------
-y + z = -2 ------------ (4)
Multiply equation (3) by 4 and add it to equation (2)
4 [-x + y - 2z = 5
-4x + 4y -8z = 20
+ (4x + 5y + z = 4
-------------------------
9y - 7z = 24 ------------- (5)
Solve equations (4) and (5) simultaneously
-y + z = -2
9y - 7z = 24
From equation (4)
-y + z = -2
z = -2 + y ---------- (6)
Substitute equation (6) into equation (5)
9y - 7z = 24
9y - 7(-2 +y) = 24
9y + 14 - 7y = 24
2y + 14 = 24
2y = 24 - 14
2y = 10
y = 10/2
y = 5
Substitute the value of y into equation (6)
z = -2 + y
z = -2 + 5
z = 3
Substitute the value of y and z into equation (1)
x - 2y + 3z = -7
x - 2(5) + 3(3) = -7
x - 10 + 9 = - 7
x - 1 = -7
x = -7 + 1
x = -6
Hence, the solution to the given system of equations is x = -6, y = 5, and z = 3. That is, the solution is (-6, 5, 3)
Learn more on Solving system of equations here: https://brainly.com/question/15352379
#SPJ1
The depth of water at a dock can be modeled as y=4 sin( pi 6 x)+9. At low tide , the water is 5 feet deep. What is the depth of water at high tide its maximum point )?

Answers
Answer:
your right the answer is A
have a good day (= <3 <3
Step-by-step explanation:
The depth of water at high tide at a maximum point is 13 feet because the maximum value of the sin function is 1 option (A) is correct.
What is trigonometry?Trigonometry is a branch of mathematics that deals with the relationship between sides and angles of a right-angle triangle.
We have a trigonometric function:
\(\rm y = 4 \ sin(\dfrac{\pi}{6}x)+9\)
As we know, the maximum value of a sin function varies [-1, 1]
At minimum value = -1
The depth of the water y = -4 + 9 = 5 feet
At maximum value, sin function = +1
y = 4 + 9
y = 13 feet
Thus, the depth of water at high tide at a maximum point is 13 feet because the maximum value of the sin function is 1 option (A) is correct.
Learn more about trigonometry here:
brainly.com/question/26719838
#SPJ2
100 POINTS!!!
What is the sum of 1/2 x-1/3 and 5x^2-2x+6? Write your answer in standard form
Answers
\(\\ \sf\Rrightarrow \left(\dfrac{1}{2}x-\dfrac{1}{3}\right)+5x^2-2x+6\)
\(\\ \sf\Rrightarrow \left(\dfrac{x}{2}-\dfrac{1}{3}\right)+5x^2-2x+6\)
\(\\ \sf\Rrightarrow \dfrac{3x-2}{6}+5x^2-2x+6\)
\(\\ \sf\Rrightarrow \dfrac{3x-2+30x^2-12x+36}{6}\)
\(\\ \sf\Rrightarrow \dfrac{30x^2+3x-12x-2+36}{6}\)
\(\\ \sf\Rrightarrow \dfrac{30x^2-9x+34}{6}\)
Triangle FGH, with vertices F(-5,-7), G(-2,-5), and H(-6,-2), is drawn inside a rectangle, as shown below.
Answers
The area of the triangle FGH is equal to 13√10 unit²
Given that the vertices;
F(-5,-7), G(-2,-5), and H(-6,-2)
We have to find the area of the triangle as;
Area of triangle = 1/2bh²
Here,
Area of triangle FGH = 1/2 (GH) (FG)²
Now,
Length of FG = √26
Length of GH = √10
Then,
Area of triangle FGH = 1/2 (GH) (FG)²
Area of triangle = 1/2 × √10 × √26²
Area of triangle = 1/2 × √10 × 26
Area of triangle FGH = 13√10
Therefore,
The area of the triangle FGH = 13√10 unit²
Learn more about triangles here;
brainly.com/question/12949215
#SPJ1
Please help I'll mark brainliest

Answers
Answer:
you would be correct in the second option, unknown
Step-by-step explanation:
anonymous is another word for unknown, i learned this in gamer talk ;P
Answer: Unknown.
Step-by-step explanation: When any author is anonymous whether they write myths, legends, short stories, etc, it means that their name is hidden or unknown. If you looked it up online, it would tell you this, "(of a person) not identified by name; of unknown name." Therefore, anonymous means an author not identified who is unknown.
Let me know if this is right! :)
Ayaan drank 11/4 bottles of water during a soccer game.what is this fraction as a mixed numeral
Answers
Ayaan drank 11/4 bottles of water during the soccer game, which can be expressed as a mixed numeral 2 and 3/4.
To convert the fraction 11/4 to a mixed numeral, we need to determine the whole number part and the fractional part.
Divide the numerator (11) by the denominator (4).
11 ÷ 4 = 2 remainder 3
The quotient 2 represents the whole number part, and the remainder 3 represents the fractional part.
Write the mixed numeral using the whole number part and the fractional part.
The mixed numeral for 11/4 is:
2 and 3/4
Therefore, Ayaan drank 11/4 bottles of water during the soccer game, which can be expressed as a mixed numeral 2 and 3/4.
for such more question on fraction
https://brainly.com/question/1622425
#SPJ8
Can someone help please
ASAP

Answers
Answer:
see explanation
Step-by-step explanation:
using the tangent ratio in the right triangle
tan A = \(\frac{opposite}{adjacent}\) = \(\frac{BC}{AB}\) = \(\frac{5}{11}\) , then
∠ A = \(tan^{-1}\) ( \(\frac{5}{11}\) ) ≈ 24.4° ( to 1 decimal place )
tan C = \(\frac{opposite}{adjacent}\) = \(\frac{AB}{BC}\) = \(\frac{11}{5}\) , then
∠ C = \(tan^{-1}\) ( \(\frac{11}{5}\) ) ≈ 65.6° ( to 1 decimal place )
using Pythagoras' identity in the right triangle
the square on the hypotenuse is equal to the sum of the squares on the other two sides, that is
AC² = AB² + BC² = 11² + 5² = 121 + 25 = 146 ( take square root of both sides )
AC = \(\sqrt{146}\) ≈ 12.08 ( to 2 decimal places )
For every part produced by a factory there are 5 ounces of scrap aluminum that can be recycled there are 16 ounces in 1 pound and 2,000 in 1 ton how many parts must the factory produce so that there is1 ton of scrap aluminum for recycling
Answers
The factory must produce 6,400 parts so that there is 1 ton of scrap aluminum for recycling.
It is given that For every part produced by a factory there are 5 ounces of scrap aluminum that can be recycled there are 16 ounces in 1 pound and 2,000 in 1 ton we need to find how many parts must the factory produce so that there is 1 ton of scrap aluminum for recycling
What is a Ratio?
A quantitative relation between two amounts showing the number of times one value contains in another
1 part produced ⇒ 5 ounces scrap aluminium recycled.
So, the ratio of parts produced : Scrap of aluminium = 1 : 5 .... (a)
Let us assume for m parts produced , 1 ton of scrap is recycled.
Also, 1 ton = 2, 000 pounds
and 1 pound = 16 ounces
⇒ 1 ton = 2000 x (16 ounces) = 32,000 ounces
So, for m parts made in the factory, 32,000 ounces scrap is recycled.
So, the ratio of parts produced : Scrap of aluminium = m : 32,000 .... (b)
From both the given ratio, we get
1 : 5 = m : 32,000
m=32000/5
m=6400
To learn more about ratios click here : https://brainly.com/question/18956877
#SPJ1
how many months are in y years and m months?
Answers
Answer:
Step-by-step explanation:
There are 12 months in one year, so there would be 12*y in y years.
Answer:
there 12 months in one 1 year
Step-by-step explanation:
sally and remy are each reading the same book. Sally read 1/4 of the book on monday and 2/4 of the book on tuesday. remy read 2/8 of the book on monday and 4/16 of the book on tuesday. Sally says that altogether she read more on monday and tuesday then remy. is sally correct, how do you know?
Answers
Answer: sally is correct, sally read 3/4 (0.75) of the book and rely read 0.5 (1/2) of the book
Step-by-step explanation:
Answer: She is correct
Step-by-step explanation: 1/4+2/4=3/4 of the book while 2/8=1/4 and 4/16=1/4 and 1/4+1/4=2/4 and 3/4>2/4 so Sally is correct. She read 1/4 more of the book Remy. By the way, if you are asking questions, please Capitalize names. Thank you.
*Please I need help quickly* Approximate the mean of the frequency distribution for the ages of the residents of a town.
The approximate mean age is years.
(Round to one decimal place as needed.)
Age
0-9
10-19
20-29
30-39
40-49
50-59
60-69
70-79
80-89
Frequency
30
32
12
20
25
53
49
13
8

Answers
Answer:
The mean age of the frequency distribution for the ages of the residents of a town is 43 years.
Step-by-step explanation:
We are given with the following frequency distribution below;
Age Frequency (f) X \(X \times f\)
0 - 9 30 4.5 135
10 - 19 32 14.5 464
20 - 29 12 24.5 294
30 - 39 20 34.5 690
40 - 49 25 44.5 1112.5
50 - 59 53 54.5 2888.5
60 - 69 49 64.5 3160.5
70 - 79 13 74.5 968.5
80 - 89 8 84.5 676
Total 242 10389
Now, the mean of the frequency distribution is given by the following formula;
Mean = \(\frac{\sum X \times f}{\sum f}\)
= \(\frac{10389}{242}\) = 42.9 ≈ 43 approx.
Hence, the mean age of the frequency distribution for the ages of the residents of a town is 43 years.
Answer:
The mean age of the frequency distribution for the ages of the residents of a town is 43 years.
Step-by-step explanation:
We are given with the following frequency distribution below;
Age Frequency (f) X
0 - 9 30 4.5 135
10 - 19 32 14.5 464
20 - 29 12 24.5 294
30 - 39 20 34.5 690
40 - 49 25 44.5 1112.5
50 - 59 53 54.5 2888.5
60 - 69 49 64.5 3160.5
70 - 79 13 74.5 968.5
80 - 89 8 84.5 676
Total 242 10389
Now, the mean of the frequency distribution is given by the following formula;
Mean =
= = 42.9 ≈ 43 approx.
Hence, the mean age of the frequency distribution for the ages of the residents of a town is 43 years
which two ratios form a proportion
A 1:2 and 4:2
b 1:2 6:3
c2:1 2:4
d 2:1 and 6 :3
Answers
Answer:c
Step-by-step explanation:
Did the test
Answer:
D. 2:1 & 6:3
Step-by-step explanation:
Proportion means equal so we need to see which ratios are equal.
To do that we need to make one fo the numbers in the ratio equal the corresponding number in the other ratio, then see if the other matches.
A:
1:2 & 4:2
The second number is already the same but the first isn't, therefore it is not proportional.
B:
1:2 & 6:3
To get 1 to equal 6 we must multiply it by 6, and what you do to one side you must do to the other so we have to multiply 2 by 6 as well
6:12 & 6:3
The first numbers are the same but the second isn't, therefore it is not proportional.
C:
2:1 & 2:4
The first numbers are the same but the second isn't, therefore it is not proportional
D:
2:1 & 6:3
to get 2 to equal 6 we need to multiply it by 3, which means we have to multiu 1 by 3 too
6:3 & 6:3
These are equal so this is proportional.
Write the exponential form of "(5)^-2 = 1/25" in logarithmic form *
Answers
The required logarithm form is -2log5
Given the exponential form of an equation expressed as;
\((5)^{-2} = 1/25\)
For us to change the exponential form into logarithmic equation, first we need to identify the base, move it to the other side of the expression and then take the logarithm
Take the logarithm of both sides
\(= log(5)^{-2} \\=-2log5\\\)
Hence the required logarithm form is -2log5
Learn more on logarithm here: https://brainly.com/question/17622052
![What Is The Probability Ofspinning A Yellow?[?]%](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/HSM7dPVeCFpwXYxAmy6pUUl1MEpRGa1T.png)