what is the measure of an angle if it is 660 less than 6 times its own supplement
Answers
Answer:
60
Step-by-step explanation:
Since this angle is unknown, let's just represent it with the variable "x". So the supplement angle of "x" is essentially, the value of the angle that we need to add to "x" to get 180. In other words let's just say that the supplement angle is "y". This means that: \(x+y=180\). If we subtract x from both sides we can represent "y" or the supplement angle as: \((180-x)\).
Since it's 660 less than 6 times it's own supplement let's first multiply the supplement by 6
\(6(180-x) = 1,080-6x\)
Now let's subtract 660 from it, since it's 660 less
\((1,080 - 6x) - (660) = 420 - 6x\)
This value should be equal to the angle, which is represented as "x". So let's set it equal to x
\(420-6x=x\)
Add 6x to both sides
\(420=7x\)
Divide both sides by 7
\(60 = x\)
Related Questions
this is due now!!!!!!!!!!!

Answers
Answer:
it's d
Step-by-step explanation:
x goes to the right 8 so it's +8
and y goes up u so it's +7
Answer:
D) (x, y) - ( x+8, y+ 7)
Step-by-step explanation:
A coordinates= (-5, -1)
Add -5+8=3
Add -1+7=6
Which gives you (3,6) which is A’
Do that to all the points and they all line up
Hope this helps :)
Read the paragraph. Replace each underlined noun by writing a pronoun above it.
Then, read the new paragraph.

Answers
Answer:
Step-by-step explanation:
She
She
Her
He
Their
They
They
Their
We
They
Him
A set of laptop prices are normally distributed with a mean of 750750750 dollars and a standard deviation of 606060 dollars. What proportion of laptop prices are between 624624624 dollars and 768768768 dollars?.
Answers
The proportion of the laptops is equal to 0.6 with the price range between 624 dollars to 768 dollars for the given mean and standard deviation.
As given in the question,
Given condition:
Price of of given laptops set are normally distributed:
Mean 'μ' is equal to 750 dollars
Standard deviation 'σ' is 60 dollars
let 'X' represents the price of laptops
Range of price is given by:
624 < X < 768
= (624 - μ)/σ < (X - μ)/σ < (768 - μ)/σ
= (624 - 750)/60 < (X - μ)/σ < (768 - 750)/60
= -126 /60 < (X - μ)/σ < 18 /60
= -2.1 < Z < 0.3
From the table , value of z-score is equal to
z-score for -2.1 = 0.01786
z-score for 0.3 = 0.6179
Required proportion is equal to
0.6179 - 0.01786
= 0.6179 - 0.0179
= 0.6
Therefore, the proportion of laptops with the price between 624 and 768 is equal to 0.6.
The complete question is :
A set of laptop prices are normally distributed with a mean of 750 dollars and a standard deviation of 60dollars.
What proportion of laptop prices are between 624 dollars and 768 dollars?!
You may round your answer to four decimal places.
Learn more about proportion here
brainly.com/question/15866190
#SPJ4
What is the perimeter of the base of the pyramid?

Answers
Answer:
18
Step-by-step explanation:
Length(L)=6
Breadth(B)=3
so...
P=2(L+B)
=2(6+3)
=2×9
=18
HURRY ASAP HELP
Store A charges $1,200 for repairs with a 6 percent sales tax. Store B charges $1,350 with a 7 percent sales tax. Explain how you would determine the amount of money saved by using Store A instead of Store B?
Answers
Step-by-step explanation:
Store A:
6% of 1,200 is 72
72 + 1,200 = 1,272
Thus, store A has a total of 1,272
Store B:
7% of 1,350 is 94.5
94.5 + 1,350 = 1444.5
Thus, Store B has a total of 1444.5
1444.5 - 1,272 = 172.5
Thus, Store A is cheaper.
Answer:
sales tax- rate×original amount
A- 6/100(1200)=72
B- 7/100(1350)=94.5
Which of the following statements must be true based on the diagram below? Select
all that apply. (Diagram is not to scale.)
L
K
O
M M
P
N
OP is a segment bisector.
OP is a perpendicular bisector.
OOP is an angle bisector.
O is the vertex of a pair of congruent angles in the diagram.
O is the vertex of a right angle.
Pis the midpoint of a segment in the diagram.

Answers
We have with a study the diagram of the diagram below and the explanations that follows the correct answers are
NONONOYESNOThe mid point of this segment can be derived by drawing diagonals across the 4 vertex LMNK.Generally
1)
OP is a segment bisector.
NO
OP is not a segment bisector because it those not divide segment LKMN in to two equal parts.
2)
OP is a perpendicular bisector.
NO
OP is not a perpendicular bisector because a perpendicular bisector is a bisector that cuts a segment into two equal parts and create an angle to the normal
3)
OP is an angle bisector
NO.
Because OP those not divide any angles into two equal parts
4)
O is the vertex of a pair of congruent angles in the diagram.
YES
Because O lines in between two angles of the same degree()
5)
O is the vertex of a right angle.
NO
Because O those not exist at a right angle
6)
The midpoint of a segment in the diagram
The mid point of this segment can be derived by drawing diagonals across the 4 vertex LMNK.
For more information on this visit
https://brainly.com/question/17581080
Consider a separation performed on a 35.0 mm long open tubular column with a 0.50 mm diameter, and a 2.0 μm thick stationary phase. Compound A eluted at 12.46 min, compound B eluted at 13.12 min, and the unretained solvent eluted at 1.265 min. What are the adjusted retention times, tr\', and retention factors, k, for compounds A and B?
What is the relative retention, alphar, for this separation?
The width at half-height, w1/2, of the peak for compounds A and B are 0.141 min and 0.272 min, respectively. Find the number of theoretical plates, N, and plate height, H, for each compound.
Answers
Relative retention (α ) is 1.06
What is relative retention ( α)?This factor gives an idea as to how far two compounds can be separated by a given stationary phase. For any two compounds 1 and 2, the relative retention, α is the ratio of their adjusted retention times. Hence, α= t'R2 / t'R1 . relative retention ( α) has to be always more than 1.
For A,
Retention (\(t_r_A\)) = 12.46
Time
Baseline peak width (W) = 0.141
Number of theoretical plates, N(A) = 16 (\(t_r\)/w)²
= 16(12.46/0.141)²
= 124,944.7
For B,
Retention Time (\(t_r_B\)) = 13.12
Baseline peak width (w) = 0.272
Number theoretical plates, N(B) = 16 (\(t_r\)/w)²
= 16 (13.12/0.272)²
= 37,226.3
\(\textup{Adjusted retention time,} t_R_'=t_R-t_m\)
\(\textup{Retention factor,} k=\frac{t_R_'}{t_m}\)
For A
\(t_R\)' = (12.46 min) - (1.265min) = 11.195 min
Retention factor = 11.95/1.265 = 8.85
For B
\(t_R\)' = (13.12 min) - (1.265 min) = 11.855
Retention Factor = 11,855/ 1,265 = 9.37
\(\textup{Relative Retention }, \alpha =\frac{t_R_'(B)}{t_R_'(A)}\)
\(=\frac{9.37}{8.85} = 1.06\)
Hence, relative retention (α) is 1.06
To learn more about the relative retention ( α) here:
https://brainly.com/question/16949600
#SPJ1
Question Progress
The points A, B, C and D lie in order on a straight line.
AB BD = 2:3
AC: CD = 2:1
Work out AB: BC: CD
Give your answer in its simplest form.
Optional working
Answer:
D
Answers
The complete line ratio AB : BC : CD is 6 : 2 : 5
How to determine the complete ratio?From the question, we have the following parameters that can be used in our computation:
AB : BD = 2 : 3
AC : CD = 2 : 1
Rewrite them as
x ⇒ AB : BD = 2 : 3
y ⇒ AC : CD = 2 : 1
These ratios can be represented using the following equation
2x + 3x = 2y + y
Evaluate the sum
5x = 3y
Simplify
x = 3/5y
So, we have
AB : BC : CD
This gives
AB : CD - BD : CD
So, we have
2x : y - x : y
Solving further, we have
2 * 3/5y : y - 3/5y : y
Evaluate
6/5y : 2/5y : y
Multiply through by 5
6y : 2y : 5y
Divide through by y
6 : 2 : 5
Hence, the ratio is 6 : 2 : 5
Read more about ratio at
https://brainly.com/question/1781657
#SPJ1
Pls help with this answer

Answers
When b is 3, the value of the expression \(2b^3 + 5\) is 59.
To evaluate the expression\(2b^3 + 5\) when b is 3, we substitute the value of b into the expression and perform the necessary calculations.
Given that b = 3, we substitute this value into the expression:
\(2(3)^3 + 5\)
First, we evaluate the exponent, which is 3 raised to the power of 3:
2(27) + 5
Next, we perform the multiplication:
54 + 5
Finally, we add the two terms:
59
Therefore, when b is 3, the value of the expression \(2b^3 + 5\) is 59.
In summary, by substituting b = 3 into the expression \(2b^3 + 5\), we find that the value of the expression is 59.
It's important to note that the provided equation has multiple possible solutions for x, but when b is specifically given as 3, the value of x is approximately 3.78.
It's important to note that in this equation, we substituted the value of b and solved for x, resulting in a specific value for x. However, if we wanted to solve for b given a specific value of x, we would follow the same steps but rearrange the equation accordingly.
For more such question on expression visit:
https://brainly.com/question/1859113
#SPJ8
Question 3 The manager of an ice cream store finds that 55 of the 153 people who tried the new flavor are buying it. Use the data to estimate the probability that a person who tries the new flavor will buy it. Round your answer to 3 digits after the decimal point. __________________
Question 4 In Monopoly one rolls a pair of dice. Find the probability of getting a sum of 4 . Round your answer to 3 digits after the decimal point. ______________________
Answers
The probability that a person who tries the new flavor will buy it is approximately 0.359. The probability of getting a sum of 4 when rolling a pair of dice is approximately 0.083.
For the first question, the probability that a person who tries the new flavor will buy it can be estimated by dividing the number of people who bought it by the total number of people who tried it. In this case, out of 153 people who tried the new flavor, 55 bought it.
Probability of buying = Number of people who bought / Total number of people who tried
Probability of buying = 55 / 153 ≈ 0.359
Therefore, the estimated probability that a person who tries the new flavor will buy it is approximately 0.359.
Now, let's move on to the second question.
In Monopoly, when rolling a pair of dice, we need to find the probability of getting a sum of 4. To determine this probability, we first need to identify all the possible outcomes that can result in a sum of 4 when rolling two dice.
The possible combinations that result in a sum of 4 are: (1, 3), (2, 2), and (3, 1). There are three favorable outcomes.
The total number of outcomes when rolling two dice is given by the product of the number of outcomes for each die. Since each die has six sides, there are 6 possible outcomes for each die, resulting in a total of 6 × 6 = 36 possible outcomes.
Probability of getting a sum of 4 = Number of favorable outcomes / Total number of possible outcomes
Probability of getting a sum of 4 = 3 / 36 = 1 / 12 ≈ 0.083
Therefore, the probability of getting a sum of 4 when rolling a pair of dice is approximately 0.083.
To know more about probability calculations, refer here:
https://brainly.com/question/18763226#
#SPJ11
Let f = xy-yz; v = (2y; 2z; 4x + z); and w = (3z2 ; 2x2 -y2 ; y2 ).
Find (a) curl [grad (f 2 )] ; (b) [(curl v) x w] .w; (c) [(grad f) x v] .v:
Answers
The final answers are (0, 0, 0), 4x²y³ - 9y²z² + 2y⁵, and 6x²y - 6xyz - 2xz² + 4x²z + 2yz² - 2z³ respectively.
a) curl [grad (f²)]
First, calculate grad (f²).
grad (f²) = (2xy-2yz, 2xy-2xz, 2yz-2xz)
Now, calculate the curl of grad (f²).
curl of grad (f²) = (0, 0, 0)
The final answer is (0, 0, 0).
b) [(curl v) x w].w
First, calculate curl v.
curl v = (0, -4x-2y, -2z)
Now,
calculate [(curl v) x w].[(curl v) x w] = (2y(2z) - (4x+2y)(y²), -(4x+2y)(3z² - y²) - 2z(2y), (4x+2y)(y²) - 3z²(2y))
= (-4xy² + 2yz² + 4x²y - 2y³, -12x²z - 6yz² - 4xy² - 2z³ + 2y³, 8x²y - 6yz² - 6y²z)
cw = (3z²y², -2x²y² + y⁴, y⁴)
Then, calculate [(curl v) x w].w.[(curl v) x w].w
= (-12x²z - 6yz² - 4xy² - 2z³ + 2y³)(3z²y²) + (8x²y - 6yz² - 6y²z)(y⁴) + (-4xy² + 2yz² + 4x²y - 2y³)(y⁴)
The final answer is 4x²y³ - 9y²z² + 2y⁵.
c) [(grad f) x v].v
First, calculate grad f.
grad f = (y, x-z, -y)
Now, calculate
[(grad f) x v].[(grad f) x v] = (2z²y - 4y²x - 2yz², 4x³ - 8x²z - 2z³ + 2xy², 4xy² - 8xyz + 2xz² + 4y²z)
= (-2z(2xy-yz), 2x(2x²-2xz+z²-2y²), 2y(2x²-2yz+y²))
= (-2z(xy-yz), 2x(x²-xz+z²-y²), 2y(x²-yz+y²))
= (2yz-zxy, xz-x²+xy²-y², -xy+y²-yz)cv
= (3z², 2x²-y², y²)
Then, calculate
[(grad f) x v].v.[(grad f) x v].v = (2yz-zxy)(2y) + (xz-x²+xy²-y²)(2z) + (-xy+y²-yz)(4x+z)
The final answer is 6x²y - 6xyz - 2xz² + 4x²z + 2yz² - 2z³.
After performing the required calculations, we get the final answers as (0, 0, 0), 4x²y³ - 9y²z² + 2y⁵, and 6x²y - 6xyz - 2xz² + 4x²z + 2yz² - 2z³ respectively.
To know more about the curl, visit:
brainly.com/question/32516691
#SPJ11
solve for c.
4(3+c)+c=c+4
Answers
Answer:
c=-2
Step-by-step explanation:
4(3+c)+c=c+4
distribute the 4
12+4c+c=c+4
subtract c on both sides
12+4c=4
subtract 12 on both sides
4c=-8
c=-2
Step-by-step explanation:
subtract c and distribute
12 + 4 c = 4
4c = - 8
solve for c
In the ale the original price are reduced by 15%
a. Calculate the ale price of a book that ha an original price of $12
b. Calculate the original price of a jacket that ha a ale price of $38. 25
Answers
Answer:b
Step-by-step explanation:
helphelp help help hel

Answers
Answer:
Awhhhhhhhhhh
No ideas yet
Answer:
B
Step-by-step explanation:
Multiply and Dividing are usually first not adding and Subtracting
What is the probability that a randomly selected airfare between these two cities will be more than $450?
Answers
The probability that a randomly selected airfare between these two cities will be more than $450 is 0.2033.
Given:
Mean (μ) = $387.20
Standard deviation (σ) = $68.50
To find the probability that a randomly selected airfare between Philadelphia and Los Angeles will be more than $450,
calculate the area under the normal distribution curve above the value of $450.
Step 1: Standardize the value of $450.
To standardize the value, we calculate the z-score using the formula:
z = (X - μ) / σ
z = ($450 - $387.20) / $68.50
z= 0.916
So, the area to the right of the z-score approximately equals 0.2033.
Therefore, the probability that a randomly selected airfare between these two cities will be more than $450 is 0.2033.
Learn more about Probability here:
https://brainly.com/question/28146696
#SPJ4
The question attached here seems to be incomplete, the complete question is:
Suppose the round-trip airfare between Philadelphia and Los Angeles a month before the departure date follows the normal probability distribution with a mean of $387.20 and a standard deviation of $68.50. What is the probability that a randomly selected airfare between these two cities will be more than $450?
0.0788
0.1796
0.2033
0.3669
Solve the equation.
(x+3)^2-5=0
And
Solve the equation.
(2x+1)(x-5)=0
Answers
The solution to equation (x+3)² - 5 = 0 is x = -3 +√5 and x = -3 - √5. The solution to equation (2x+1)(x-5)=0 is x = -1/2 and x = 5.
What is a quadratic function?The quadratic function is defined as a function containing the highest power of a variable is two.
To determine the solution to equation (x+3)² - 5 = 0
(x+3)² - 5 = 0
x² + 9 + 6x - 5= 0
x²+ 6x + 4 = 0
Using the quadratic formula to solve the above quadratic function
\(x=\dfrac{-b\pm \sqrt{b^2-4ac}}{2a}\)
\(x=\dfrac{-6\pm \sqrt{6^2-4\cdot \:1\cdot \:4}}{2\cdot \:1}\)
\(\sqrt{6^2-4\cdot \:1\cdot \:4}\) = 2√5
x = -6± 2√5 / 2
x = -3± √5
x = -3 +√5 and x = -3 - √5
To determine the solution to equation (2x+1)(x-5)=0
(2x+1)(x-5)=0
(2x+1) = 0 and (x-5)=0
2x = -1 and x = 5
x = -1/2 and x = 5
Thus, the solution to equation (x+3)² - 5 = 0 is x = -3 +√5 and x = -3 - √5. The solution to equation (2x+1)(x-5)=0 is x = -1/2 and x = 5.
Learn more about quadratic function here:
brainly.com/question/14083225
#SPJ1
Find the missing
measure of the
triangle given the
angle measurements.
75°, 30°, y
Answers
Answer:
75
Step-by-step explanation:
If a triangle = 180 degrees, then subtract 105 by 180, and you get 75!
12
28.
Find the sum: 1.3 +2.4 +3.5+ ... to n terms. 2
Answers
Let S denote the sum
\(1\cdot3+2\cdot4+3\cdot5+\cdots+n\cdot(n+2)\)
We can condense this to sigma notation:
\(S=\displaystyle\sum_{k=1}^nk(k+2)\)
Expand the summand:
\(S=\displaystyle\sum_{k=1}^nk^2+2\sum_{k=1}^nk\)
Recall the Faulhaber formulas,
\(\displaystyle\sum_{k=1}^nk=\frac{n(n+1)}2\)
\(\displaystyle\sum_{k=1}^nk^2=\frac{n(n+1)(2n+1)}6\)
So we have
\(S=\dfrac{n(n+1)(2n+1)}6+n(n+1)=\boxed{\dfrac{n(n+1)(2n+7)}6}\)
An ordered pair for a point that is 4 units away from point Q(2,-2.5)

Answers
Answer:
(-2, -2.5)
Step-by-step explanation:
There are an infinite number of such points. One of the easiest to write coordinates for is the one that is on the same horizontal line. Its x-value will be 4 less that that of Q.
(-2, -2.5) is one such point
_____
Additional comment
All of the points that could be solutions are on that portion of a circle with radius 4 and center Q that lies in quadrant III. They make up the red arc in the attachment.

3(x–6)–1/2(8x+10)=–25

Answers
The solution to the equation 3(x-6) - 1/2(8x+10) = -25 is x = 2.
To simplify the equation 3(x-6) - 1/2(8x+10) = -25, we can start by applying the distributive property and then combining like terms.
First, let's distribute the 3 and the -1/2 to the terms inside the parentheses:
3(x-6) - 1/2(8x+10) = 3x - 18 - (4x + 5)
Now, let's simplify further by combining like terms:
3x - 18 - 4x - 5 = -x - 23
So, the simplified equation is -x - 23 = -25.
To solve for x, we can isolate the variable by adding 23 to both sides of the equation:
-x - 23 + 23 = -25 + 23
This simplifies to:
-x = -2
Finally, we can solve for x by multiplying both sides of the equation by -1:
(-1)(-x) = (-1)(-2)
This gives us:
x = 2
Therefore, the solution to the equation 3(x-6) - 1/2(8x+10) = -25 is x = 2.
For more such questions on equation visit:
https://brainly.com/question/17145398
#SPJ8
I WILL GIVE YOU BRAINLIST
Use academic language and/ or numbers and symbols to explain your choice

Answers
With regards to the probabilities of getting each color in the game played by Larry and Jessica, the correct answer is B. No, the probabilities are NOT equally likely.
How to find the probabilities ?In the game being played by Larry and Jessica, the colors that can be picked by either person include:
Red Blue GreenThe probability of picking blue is:
= Number of blue sections / Total sections
= 3 / 8
The probability of picking red is :
= 3 / 8
The probability of picking green is:
= 2 / 8
= 1 / 4
The probabilities are therefore not equally likely.
Find out more on probabilities at https://brainly.com/question/28109373
#SPJ1
A parking lot that has 520 spaces
was filled with 390 cars. What
percent of the parking lot was full?
Answers
Answer:
75%
Step-by-step explanation:
390/520 = 0.75 =75
hope that helps ;)
Answer:
75%
Step-by-step explanation:
390/520×100%
0.75×100%
75%
Two functions are graphed on the coordinate plane.Which represents where f(x) = g(x)?12Iy10sex)+Of(4) = g(4) and f(0) = g(0)Of(-4) = g(-4) and f(0) = g(0)Of(-4) = g(-2) and f(4) = g(4)Of(0) = g(4) and f(4) = g(-2)6f(x)1234 56 x-6 -5 -4 -3 -2 -12-4L6-8-10--12Mark this and returnSave and ExitNextSubmit
Answers
The expression f(x)=g(x) indicate the points in the graph where the two functions intersect, i.e. when they cross
In this graph, g(x) cuts f(x) in two points (-4,4) and (0,4)
Now f(x) is a contstant, it has no slope and is equal to 4, always.
Since g(x) cuts f(x) in point (-4,4), you can say that f(-4)= g(-4)
Second cut is in point (0,4), this means f(0)= g(0)
The correct option is number 2 f(-4)= g(-4) and f(0)= g(0)
The product of two consecutive integers is 19 more than their sum. Find the integers.A. 5,6 B. -4,-3 C. 4,5 or -4,-3 D. 5,6 or -4 , -3
Answers
The answer to the question is C, which is integers 4,5 or -4,-3.
Let's call the first integer x and the second integer x+1 (since they are consecutive).
According to the problem, we can set up the following equation:
x(x+1) = x + (x+1) + 19
We can simplify this equation by expanding the left side and combining like terms on the right side:
x^2 + x = 2x + 20
Now we can move all the terms to one side and get a quadratic equation:
x^2 - x - 20 = 0
We can factor this equation as (x-5)(x+4) = 0, which means the solutions for x are 5 and -4.
Therefore, the two consecutive integers are either 4,5 or -4,-3.
The long answer includes all the steps of solving the quadratic equation:
x^2 - x - 20 = 0
We can use the quadratic formula to solve for x:
x = (-b ± √(b^2 - 4ac)) / 2a
In this case, a=1, b=-1, and c=-20.
x = (1 ± √(1 - 4(1)(-20))) / 2(1)
x = (1 ± √81) / 2
x = (1 ± 9) / 2
The two solutions for x are 5 and -4.
Therefore, the two consecutive integers are either 4,5 or -4,-3.
The product of two consecutive integers is 19 more than their sum. To find the integers, let's represent them as x and x+1.
To know more about integer visit:
https://brainly.com/question/15276410
#SPJ11
What is the domain? I need help on this problem

Answers
The domain of the function \(f(x) = \sqrt{\frac{1}{3}x + 2\) is (d) x ≥ -6
How to determine the domain of the functionFrom the question, we have the following parameters that can be used in our computation:
\(f(x) = \sqrt{\frac{1}{3}x + 2\)
Set the radicand greater than or equal to 0
So, we have
1/3x + 2 ≥ 0
Next, we have
1/3x ≥ -2
So, we have
x ≥ -6
Hence, the domain of the function is (d) x ≥ -6
Read more about domain at
https://brainly.com/question/31900115
#SPJ1
BRAINLEST MR D QUIZ PRE ALGEBRA!!!!!!!!
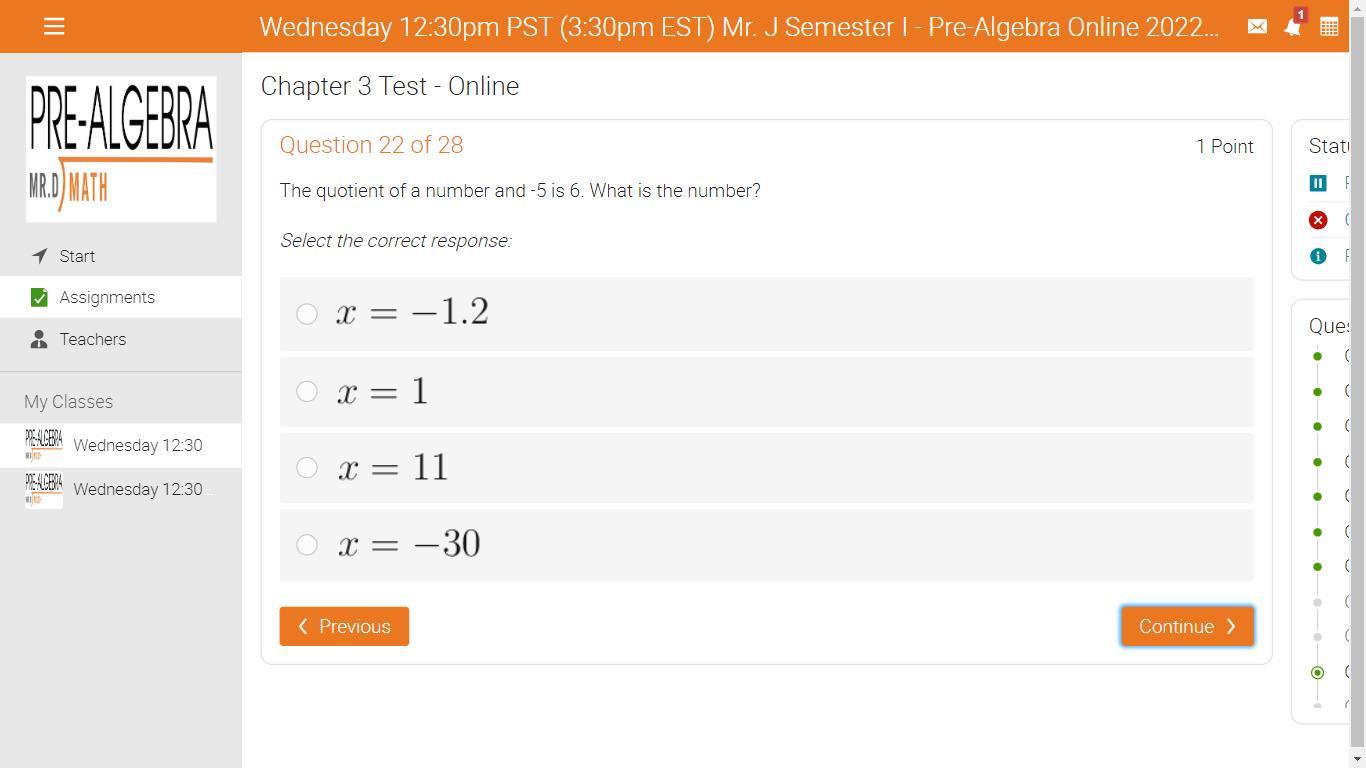




Answers
Answer is the following:
B
someone help meh plzz

Answers
Answer:
1) 229 Teens
2) (12,-2)
ements
.
2
Lin has a bag with the following bead:.
2 beads are red
3 beads are blue
4 beads are yellow
Lin will randomly select a bead from the bag.
What is the probability that she selects a blue bead?
Answers
Answer:
Probability that she selects a blue bead
= 3/9
= 1/3
Step-by-step explanation:
Please mark me brainliest
An \( \bar{X} \) chart is used to track a process with binary outcome variables. True or False
The reasons for holding inventory include all the following EXCEPT Multiple Choice it serves as a buffer
Answers
The statement is false. A process chart is a graphical tool used to monitor processes in production and service industries. The average process performance and the upper and lower limits are calculated and displayed on an X-bar chart, which is a common process chart.
An \( \bar{X} \) chart is NOT used to track a process with binary outcome variables. The statement is false.
Explanation: A process chart is a graphical tool used to monitor processes in production and service industries. The average process performance and the upper and lower limits are calculated and displayed on an X-bar chart, which is a common process chart. The values for a variable can be collected and analyzed using process charts. An \( \bar{X} \) chart, commonly known as an x-bar chart, is used to monitor a process with continuous numerical data. A binary outcome variable, on the other hand, has only two possible outcomes (e.g., yes/no, pass/fail, etc.). As a result, binary data is unsuitable for an \( \bar{X} \) chart, which is only used to monitor continuous numerical data.
Variables are values that may be altered in a process, which can affect its results. Variables may be categorized as continuous or discrete, depending on whether they can be measured or counted. Discrete variables, such as the number of employees working on a task or the number of items produced in a process, are quantifiable and can be measured precisely. Continuous variables, such as the temperature, length, or weight, can take on any value over a range and are therefore less specific. The reasons for holding inventory include serving as a buffer against uncertainties, ensuring product availability and preventing stockouts, reducing lead times and transportation costs, taking advantage of discounts and bulk purchasing opportunities, and providing a hedge against inflation and price fluctuations.
To know more about X-bar chart visit: https://brainly.com/question/31861365
#SPJ11
Use synthetic division to test one potential root. enter the numbers that complete the division problem. −5 1 6 −7 −60 a −c −60 1 b −d −60 a = b = c = d =
Answers
Synthetic division to test one potential root. the numbers that complete the division problem are a = 1 b = 1 c = -12 d = -50
To use synthetic division to test the potential root, we have to arrange the polynomial coefficients in descending order and use the potential root as the divisor.
then the polynomial is written as:
1x^3 + 6x^2 - 7x - 60
Assume that the potential root is x = -5.
-5 | 1 6 -7 -60
|___-5_-5__10
1 1 -12__-50
Now the numbers that complete the division problem are:
a = 1 b = 1 c = -12 d = -50
Therefore, the polynomial can be written as (x - 5)(x^3 + x^2 - 12x - 50)
and the numbers that complete the division problem are a = 1 b = 1 c = -12 d = -50
To learn more about Synthetic division:
https://brainly.com/question/29612237
#SPJ4