▶ Use the part of the nutrition label on the right. If protein contains approximately 4 calories per gram, find the percent of calories from protein from one serving of this food. (Round to the nearest tenth of a percent.) ww. The percent of calories from protein from one serving of this food is %. Nutritional information per serving Calories 277 Protein Carbohydrate Fat 11g 45g 6g
Answers
The percent of calories from protein from one serving of the food is found as 15.88%.
What is defined as the term percentage?A relative value representing the hundredth part of any quantity One percent (denoted 1%) is one hundredth part; thereby the, 100 percent describes the entire amount and 200 % identifies twice the given amount.
For the given information;
The total calories is 277.
Nutrients information in food.
Protein - 11g
Carbohydrate - 45g
Fat - 6g
The protein contains 4 calories per gram.
Thus, 11 grams of protein contains 4×11 = 44 calories per grams.
Find the % content of protein out of 277 calories;
p% of 277 = 44
p% = (44×100)/277
p% = 15.88%.
Therefore, the percentage of protein contained in the serving food is 15.88%.
To know more about the percentage, here
https://brainly.com/question/24877689
#SPJ9
Related Questions
The average daily sleep time for a domestic cat is 11.5 hours. What is this in hours and minutes?
b) An African lion sleeps 20% more than the cat. What is the sleep time of the African lion?
mathematics PLEASE HELP
Answers
Part A
1 hour = 60 minutes
0.5 hours = 30 minutes (multiply both sides by 0.5)
11.5 hours = 11 hours + 0.5 hours
11.5 hours = 11 hours + 30 minutes
11.5 hours = 11 hours, 30 minutes is the answer
========================================
Part B
20% = 20/100 = 0.20
The lion sleeps 20% more than the cat, which means the lion sleeps 0.20*11.5 = 2.3 hours more
This leads to 11.5 + 2.3 = 13.8 hours being the total time the lion sleeps
1 hour = 60 minutes
0.8 hours = 48 minutes (multiply both sides by 0.8)
13.8 hours = 13 hours + 0.8 hours
13.8 hours = 13 hours + 48 minutes
13.8 hours = 13 hours, 48 minutes is the amount of time the lion sleeps
Find the distance between
a) The lines 4x-6y=10 & −2x+3y=5
b) The point (3,5) and the line 4x+3y=−6
Answers
a) The result is false, indicating that the point (0, 0) does not lie on Line 2. The distance between the lines is undefined since they do not intersect.
b) The distance between the point (3, 5) and the line 4x + 3y = -6 is approximately 4.2 units.
To find the distance between two lines, we can use the formula derived from the distance between a point and a line.
The formula states that the distance between two lines is equal to the perpendicular distance between any point on one line and the other line.
Given the equations of the lines:
Line 1: 4x - 6y = 10
Line 2: -2x + 3y = 5
Let's find the point of intersection between these two lines.
We can solve the system of equations:
4x - 6y = 10 ...(1)
-2x + 3y = 5 ...(2)
Multiplying equation (2) by 2, we get:
-4x + 6y = 10 ...(3)
Adding equations (1) and (3), we have:
0 = 20
The result is an inconsistent system, indicating that the lines are parallel and do not intersect.
Since the lines are parallel, the distance between them is constant.
To find this distance, we can select any point on one of the lines and substitute it into the equation of the other line.
Let's consider the point (0, 0) on Line 1:
-2(0) + 3(0) = 5
0 = 5
b) To find the distance between a point and a line, we can use the formula for the perpendicular distance between a point and a line.
The formula states that the distance between a point (x₀, y₀) and a line Ax + By + C = 0 is given by:
d = |Ax₀ + By₀ + C| / √(A² + B²)
Given the point (3, 5) and the line 4x + 3y = -6, we can calculate the distance using the formula:
A = 4, B = 3, C = -6, x₀ = 3, y₀ = 5
Substituting these values into the formula, we get:
d = |4(3) + 3(5) + (-6)| / √(4² + 3²)
= |12 + 15 - 6| / √(16 + 9)
= |21| / √25
= 21 / 5
= 4.2
For similar questions on distance
https://brainly.com/question/26046491
#SPJ8
Ryan exponential function of the form f(t)= a×b^t to represent the following situation. assume t is in years. an account has an initial investment of $91,000, increasing by 3.76% per year. F(T)=_________
Answers
Answer
\(f(t) = 91000 *1.0376^t\)
Explanation
Given
\(a = 91000\) -- initial
\(r = 3.76\%\)
Required
The exponential equation
The exponential equation is:
\(y =ab^t\)
Where
\(b=1 +r\)
We used minus, because the rate increases..
So, we have:
\(b=1 +3.76\%\)
\(b=1 +0.0376\)
\(b=1.0376\)
So:
\(y =ab^t\)
\(y = 91000 *1.0376^t\)
Hence:
\(f(t) = 91000 *1.0376^t\)
explain how to write function rule from the table below. Then write a function fule. x=0,2,4,6. y=2,1,0,-1
Answers
Both representations are equivalent and describe the same function.the function rule for this table is: f(x) = -1/2 x 2
What do you mean by Slope in Graph ?
The slope or gradient of a line is a number that describes both the direction and the steepness of the line. A slope of a line is the change in y coordinate with respect to the change in x coordinate.
To write a function rule for a given array, we need to determine how the values in the output column (y) relate to the values in the input column (x).
Looking at the given data, we notice that the output values decrease by 1 every time the input value increases by 2. This suggests that the function is linear and that we can use the slope-intercept form of a linear equation to represent it. .
The slope-intercept form of a linear equation is y = mx b, where m is the slope and b is the y-intercept. In this case, the slope m is -1/2 because the output values decrease by 1 every time the input values increase by 2. The y-intercept b is 2 because the output value is 2 when the input value is 0.
Therefore, the function rule for this table is:
f(x) = -1/2 x 2
where x is the input value and f(x) is the output value.
Alternatively, we can also represent the function in table entries, for example:
f(0) = 2
f(2) = 1
f(4) = 0
f(6) = -1
Both representations are equivalent and describe the same function.
Learn more about Slope of y-Coordinate here
https://brainly.com/question/17024646
#SPJ1
of Write the equation of this line in slope-intercept form.
Write your answer using integers, proper fractions, and improper fractions in simplest form.

Answers
We want to get the equation on the graphed line.
The equation of the line is: y = 8*x - 9
Then the line is something like:
y = 8*x + b
To find the value of b, we can use one of the two points we got above.
The point (0, -9) means that when x = 0, y is equal to -9, then we can write:
-9 = 8*0 + b
-9 = b
Finally, the equation of the line is:
y = 8*x - 9
What is graphed line?When displaying information that changes over time, a line graph, also referred to as a line chart or a line plot, is frequently used.It can be visualized using a series of points connected by straight lines. The "x-axis" and the "y-axis" are two of its axes. An informational change over time is graphically represented by a line graph. It is a graph that was created by connecting points with line segments. An informational change over time is graphically represented by a line graph. It is a graph that was created by connecting points with line segments. A line graph, also referred to as a line plot or a line chart, is a graph in which individual data points are connected by lines.To learn more about line plot refer to:
https://brainly.com/question/30143735
#SPJ1
i have 4 questions on here and i don’t understand it.

Answers
Answer:
Step-by-step explanation:
y = mx + b, where "m" - slope, "b" - y-intercept
Slopes of parallel lines are the same and y-intercepts are different.
P ( \(x_{1}\) , \(y_{1}\) )
y - \(y_{1}\) = m( x - \(x_{1}\) )
~~~~~~~~~~~~
y = 6x + 1 , m = 6
( - 7, 1 )
y - 1 = 6[x - ( - 7 )]
y = 6(x + 7) + 1
y = 6x + 43

Pablo’s hair was 1/2 inch long.Jason’s hair was 3 times as long. How long is Jason’s hair?PLEASE HELP QUICK
Answers
Answer:
Step-by-step explanation:
so you have 1/2 times 3 = 1.5
About 20% of the kids will attend an overnight summer camp, will attend day camp, and 0.22 will attend no summer camp at all. Which group of kids is
the greatest?
Ovemight Camp
O Day Camp
No Camp
Answers
If a and B are the root of the equation 3x^2 - x - 5 = 0, find a + B
Answers
Answer:
a + B = \(\frac{1}{3}\)
Step-by-step explanation:
the sum of the roots a + B = - \(\frac{b}{a}\)
where a and b are the coefficients in the standard quadratic equation
ax² + bx + c = 0 ( a ≠ 0 )
3x² - x - 5 = 0 ← is in standard form
with a = 3 and b = - 1 , then
a + B = - \(\frac{-1}{3}\) = \(\frac{1}{3}\)
If you were to open an account that will pay you 3.5% interest with a deposit of $150 so find the following ending amount for each period at the end of 2 years
Answers
Answer:
FV= $160.68
Step-by-step explanation:
Giving the following information:
Initial investment= $150
Interest rate= 3.5% compounded annually
Number of periods= 2
To calculate the future value, we need to use the following formula:
FV= PV*(1+i)^n
PV= present value
i= interest rate
n= number of periods
FV= 150*(1.035^2)
FV= $160.68
ve the system and find the numbers.
11) One number is four more than a second number. Two times the first number is 4 more than four
times the second number.
A) 7 and 3
C) 6 and 2
D) - 6 and -10
B) 5 and 1
Answers
Answer:
6 and 2
Step-by-step explanation:
Let the first and second numbers be x and y respectively.
x = 4 + y..... (1)
2x = 4 + 4y...... (2)
Using the substitution method from Eqn(1) into (2)
Anywhere we see x in Eqn(2) we substitute it with 4 + y
I. E. 2(4 + y) = 4 + 4y
8 + 2y = 4 + 4y
Collect like terms.
8 - 4 = 4y - 2y
4 = 2y
.: y = 2
Put y = 2 in Eqn(1), and we have;
x = 4 + 2
x = 6.
.: the first number is 6 and the second number is 2.
Option C is correct.
Give me all the answer with formula

Answers
Answers
A)534.990
B)2.679
C)88.611
D)705.149
E)7905.432
F)3.771
Any number over 5 add 1
hope this helps

Urgent, please help
I'll mark brainliest

Answers
Answer:
Step-by-step explanation:
The function rule g(x) after the given transformations of the graph of f(x)=4x is g(x) = -1/2x
Transformation techniques
Transformation is the process of changing the position of an object on the xy-plane.
Given the parent function
f(x) = 4x
If the function is not reflected over x-axis, the resulting function will be f(x) = -4x
If the resulting function is compressed by a factors of 1/8, hence the final function rule will be;
g(x) = 1/8(-4x)
g(x) = -1/2x
Hence the function rule g(x) after the given transformations of the graph of f(x)=4x is g(x) = -1/2x
Learn more on transformation here: brainly.com/question/4289712
#SPJ6
a triangular field has boundaries of lengths 170m,195m and 210m,find the size of the largest interior angle of the field
Answers
The size of the largest interior angle of the field is 69.86°
What is cosine law?The law of cosines relates the lengths of the sides of a triangle to the cosine of one of its angles.
Given that, a triangular field has boundaries of lengths 170m, 195m and 210m, we need to find the size of the largest interior angle of the field,
We know that, angle opposite to the largest side is largest,
Therefore,
The largest angle is opposite is 210 m side, (say c)
Using the cosine rule,
210² = 170²+195²-2(170)(195)cosC
CosC = 170²+195²-210² / 2(170)(195)
CosC = 22825 / 66300
CosC = 0.344268477
C = 69.86°
Hence, the size of the largest interior angle of the field is 69.86°
Learn more about cosine rule, click;
https://brainly.com/question/26952873
#SPJ9
Ax^2+bx+c=2(x-1)(2x+3)+(x^2-4x+2)
Answers
The quadratic form ax² + bx + c for the given set of factors is 5x² - 2x - 4.
How do we determine the quadratic equation (ax^2+bx+c) from a given set of factors?The quadratic equation that takes the form ax^2+bx+c can be determined from a given set of factors by applying the rule:
a + (b + c) = a + b + cLet us expand the eqaution: 2(x-1) (2x+3)
Ax^2+bx+c = 2(x-1) (2x+3) + x^2-4x+2So, we have:
2(x - 1)(2x + 3) ⇒ 4x² + 2x - 6.
= 4x² + 2x - 6 + x² - 4x + 2
Now, let us group like terms, we have:
= 4x² + x² + 2x - 4x - 6 + 2
= 5x² - 2x - 4
Therefore, we can conclude that the quadratic form ax² + bx + c for the given set of factors is 5x² - 2x - 4.
Learn more about quadratic equations here:
https://brainly.com/question/25841119
#SPJ1
Dimitri has let out 40m of his kite string, which makes an angle of 72° with the horizontal ground. If the kite flies directly over Sarah's head, what is the distance between Dimitri and Sarah?

Answers
Using the cosine ratio, the distance between Dimitri and Sarah is calculated as approximately 12.4 m.
How to Apply the Cosine Ratio?The cosine ratio is a trigonometric ratio that represents the ratio of the length of the adjacent side to the length of the hypotenuse in a right triangle. It is calculated by dividing the length of the adjacent side by the length of the hypotenuse.
Using the cosine ratio, we have:
Reference angle (∅) = 72 degrees
Hypotenuse length = 40 m
Adjacent length = distance between Dimitri and Sarah = x
Plug in the values:
cos 72 = x/40
x = cos 72 * 40
x ≈ 12.4 [to one decimal place]
Learn more about Cosine Ratio on:
https://brainly.com/question/15793827
#SPJ1
Simplify the expression. What classification describes the resulting polynomial?
(8x2 + 3x) − (12x2 − 1)
Answers
The simplified expression is \(-4x^2 + 3x + 1\), which is a quadratic polynomial. Option D.
To simplify the expression \((8x^2 + 3x) - (12x^2 - 1)\), we can distribute the negative sign to each term within the parentheses:
\(8x^2 + 3x - 12x^2 + 1\)
Next, we can combine like terms by adding or subtracting coefficients of similar powers of x:
\((8x^2 - 12x^2) + 3x + 1\)
Simplifying further, we have:
\(-4x^2 + 3x + 1\)
The resulting polynomial \(-4x^2 + 3x + 1\) is a quadratic polynomial since it has a highest power of x^2 (the exponent of x is 2), which is\(-4x^2.\)Quadratic polynomials are polynomials of degree 2 and can be represented by a parabola when graphed.
In summary, the simplified expression \((8x^2 + 3x) - (12x^2 - 1)\) simplifies to \(-4x^2 + 3x + 1\) , which is a quadratic polynomial. So Option D is correct
For more question on quadratic visit:
https://brainly.com/question/1214333
#SPJ8
Note the complete question is

solve 2/3(x-40)^2 = 24
Answers
Answer: 46, 34
Step-by-step explanation:
2/3(x-40)^2 = 24
(x-40)^2 = 24/(2/3)
(x-40)^2 = 36
x - 40 = 6
x = 46
x - 40 = -6
x = 34
To solve the equation 2/3(x-40)^2 = 24, start by isolating the variable x.
First, simplify the left side by multiplying both sides by 3/2:
(3/2) * 2/3 (x-40)^2 = (3/2) * 24
(x-40)^2 = 36
Next, take the square root of both sides (remember to include both positive and negative square roots, since x can be either positive or negative):
x-40 = ±6
Adding 40 to both sides gives:
x = 40 ± 6
So the main answers are:
x = 46 or x = 34
To support these answers, we can substitute each value back into the original equation and verify that it satisfies the equation:
If x = 46:
2/3(46-40)^2 = 24
2/3(6)^2 = 24
2/3 (36) = 24
24 = 24 (true)
If x = 34:
2/3(34-40)^2 = 24
2/3(-6)^2 = 24
2/3 (36) = 24
24 = 24 (true)
Therefore, the main answers are x = 46 and x = 34, and our supporting answers show that both solutions satisfy the original equation.
Learn more about this equation, here
https://brainly.com/question/30943926?
24. This week, Cristina eamed P594.65 from selling burgers for 35 days. How much he will earn in 5 days?
A. P84.95
B. P94.95
C. P104.95
D. P114.95
25. You have four quizzes in your math subject in a quarter. You got 88.75 for the first quiz, 85.5, 90.5, and 87.25 in the second, third and fourth quizzes, respectively. What is your average in the four quizzes this quarter?
A. 86.5
B. 88
C. 89.5
D. 90 26. Amberich put P580.00 into a savings account for one year. The rate of interest on the account was 6.5%. How much was the interest for one year in pesos and centavos?
A. P67.70
B. P37.70
C. P57.70
D. P17.70
24. This week , Cristina eamed P594.65 from selling burgers for 35 days . How much he will earn in 5 days ?
A. P84.95
B. P94.95
C. P104.95
D. P114.95
25. You have four quizzes in your math subject in a quarter . You got 88.75 for the first quiz , 85.5 , 90.5 , and 87.25 in the second , third and fourth quizzes , respectively . What is your average in the four quizzes this quarter ?
A. 86.5
B. 88
C. 89.5
D. 90
26. Amberich put P580.00 into a savings account for one year . The rate of interest on the account was 6.5 % . How much was the interest for one year in pesos and centavos ?
A. P67.70
B. P37.70
C. P57.70
D. P17.70
Answers
Question 24 :
P594.65 : 35 days
P594.65/7 : 35/7 days
P84.95 : 5 days
⇒ A. P84.95
Question 25 :
88.75 + 85.5 + 90.5 + 87.25 / 4
352/4
88
⇒ B. 88
Question 26 :
I = P × r × t
I = 580.00 × 0.065 × 1
I = P37.70
⇒ B. P37.70
I need this in 2 mins plz hurry to get brainly

Answers
each year, 40% of a salmon population is extracted from a farming pond. at the beginning of the next year, the pond is stocked with an additional fixed amount of n caught wild salmon. let pn denote the amount of fish at the beginning of the n-th year assume that the initial salmon population on the pond is p0=5000
a. Write a recursion to describe Pn. b. Determine the value of N so the amount of fish remains constant at the beginning of each year.
Answers
(a) \(p_{n} =p_{n-1}-0.4p_{0} +n\)
(b) \(n = {\frac{p_{n-1} -p_{n} }{0.4p_{0}} }\)
Given,
Initial salmon population \(p_{0}=5000\)
Extraction rate = 40% per year
(a) Formula for recursion,
For the first year,
\(p_{1} =p_{0} -0.4p_{0}+n\)
For the second year,
\(p_{2} =p_{1} -0.4p_{0} +n\)
For the n'th year,
\(p_{n} =p_{n-1}-0.4p_{0} +n\)
(b) The value of n,
From the above equation,
\(p_{n} =p_{n-1}-0.4p_{0} +n\)
\(n = {\frac{p_{n-1} -p_{n} }{0.4p_{0}} }\)
Learn more about Recursion here
https://brainly.in/question/634885
#SPJ4
Each year, 40% of the salmon population is extracted from a farming pond.
a. The recursion to describe Pn is Pn = 0.6 Pn-1 + N.
b. The value of N is 3000.
What is recursion?According to the recursion equation, the quantity of fish at the start of the nth year. Pn, is equal to 0.6 times the quantity at the start of the year before, Pn-1, plus the constant quantity of wild salmon that is restocked every year, N.
b. We can set the recursion equation equal to the initial population of 5000 fish in order to get the value of N that ensures the quantity of fish stays constant at the start of each year. Now we have the equation.
0.6Pn-1 + N = 5000. Solving for N gives us N = 3000.
Therefore, a. Pn = 0.6 Pn-1 + N. b. 3000.
To learn more about recursion, refer to the link:
https://brainly.com/question/16385026
#SPJ1
Solve the inequality c+2/5≥11/5, and write the solution in interval notation
Answers
Answer:
[9/5,∞)
Step-by-step explanation:
Rearrange variables to the left side of the equation: c ≥ 11/5-2/5
Calculate: c ≥ 9/5
Express solution set in interval notation: [9/5,∞)
You play a game of double roulette. You place a bet of 10 chips that the
spinner will land on a number between 1 and 12. The payoffs in this particular
game are as follows:
• You win 25 chips (the original 10 chips plus 15 more) if the spinner lands
on a number from 1-12.
• You keep your 10 chips if the spinner lands on 0 or 00.
• You lose your 10 chips if the spinner lands on any other number.
You place the same bet 50 times. Your expected winnings at the end of the
50 games is:
A. break even.
O B. win 15 chips.
O c. lose 45 chips.
O D. lose 24 chips.
O E. lose 79 chips.
Answers
Answer: The correct answer is O E. lose 79 chips.
Brainliest pwease if the answer is correct! <3 ~~
what are the similarities and differences in linear and exponential in intercepts?
what are the similarities and differences in linear and exponential in domain and range?
what are the similarities and differences in linear and exponential in asymptotes?
what are the similarities and differences in linear and exponential in misc.?
Answers
Answer:
What is a linear function? A linear function is a function whose graph is a straight line. The rate of change of a linear function is constant. The function shown in the graph below, y = x + 2, is an example of a linear function.
Graph of linear function
Graph of linear function
A linear function has a constant rate of change. The rate of change is the slope of the linear function. In the linear function shown above, the rate of change is 1. For every increase of one in the independent variable, x, there is a corresponding increase of one in the dependent variable, y. This gives a slope of 1/1 = 1.
A linear function is typically given in the form y = mx + b, where m is equal to the slope, or constant rate of change.
Examples of linear functions include:
If a person drives at a constant speed, the relationship between the time spent driving (independent variable) and the distance traveled (dependent variable) will remain constant.
Assuming no change in price, the relationship between the number of pounds of bananas a person buys (independent variable) and the total cost of the bananas (dependent variable) will remain constant.
If a person earns an hourly wage at their job, the relationship between the time spent working (independent variable) and the amount earned (dependent variable) will remain constant.
Save Timeline Autoplay Speed Normal
Video Quiz Course
71K views
Exponential Functions
What is an exponential function? An exponential function is a function that involves exponents and whose graph is a smooth curve. The rate of change in an exponential function is not constant. The functions shown in the graph below, y = 0.5x and y = 2x, are examples of exponential functions.
Graphs of exponential functions
Graphs of exponential functions
An exponential function does not have a constant rate of change. The rate of change in an exponential function is the value of the independent variable, x. As the value of x increases or decreases, the rate of change increases or decreases as well. Rather than a constant change, as in the linear function, there is a percent change.
An exponential function is typically given in the form y = (1 + r)x, where r represents the percent change.
Examples of exponential functions include:
Step-by-step explanation:
Question 9 What are the disadvantages of using a histogram instead of a dot plot?
Answers
Answer:
A histogram shows the frequencies of data in different intervals. So, you cannot read individual values off a histogram alone. With a detailed enough dot plot, you could get very close to the actual team payrolls for each league. A histogram does not give this precision.
Step-by-step explanation:
Plato sample answer
Find the distance between the points P and Q shown.
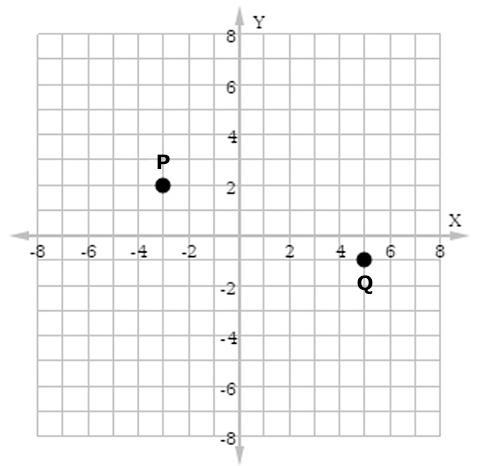

Answers
The distance between points P and Q is √73
How to find the distance between the two points?First, remember that the distance between two points whose coordinates are (a, b) and (c, d) is given by:
distance = √( (a - c)² + (b - d)²)
Here we can see that the coordinates of the two shown points are:
P = (-3, 2)
Q = (5, -1)
Replacing that in the distance formula we will get:
distance = √( (-3 - 5)² + (2 + 1)²)
distance = √( (-8)² + (3)²)
distance = √73
So the correct option is the third one.
Learn more about distance at:
https://brainly.com/question/7243416
#SPJ1
Joe has $800 to spend on a new dining room table and chairs. If the sales tax is 8%, how much can he afford to spend on the table and chairs?
Answers
Answer:
no
Step-by-step explanation:
800x8%=64
64+800=864
Answer:
$736
Step-by-step explanation:
800 x (100% - 8%)
800 x 92%
=736
Can I please get some help I’ve been stuck on this question for a while!

Answers
Using the radius of the Ferris wheel and the angle between the two positions, the time spent on the ride when they're 28 meters above the ground is 12 minutes
How many minutes of the ride are spent higher than 28 meters above the ground?The radius of the Ferris wheel is 30 / 2 = 15 meters.
The highest point on the Ferris wheel is 15 + 4 = 19 meters above the ground.
The time spent higher than 28 meters is the time spent between the 12 o'clock and 8 o'clock positions.
The angle between these two positions is 180 degrees.
The time spent at each position is 10 minutes / 360 degrees * 180 degrees = 6 minutes.
Therefore, the total time spent higher than 28 meters is 6 minutes * 2 = 12 minutes.
Learn more on a Ferris wheel here;
https://brainly.com/question/30524034
#SPJ1
ABC is a right angled triangle. if B = 90°, AC = 96 cm, C = 30°.
Answers
The length of side AB in right angled triangle ABC will be 48 cm.
What is a right angled triangle?Every triangle with one 90° angle is said to have a right angle. The triangle with a right angle is known as a right triangle because a right angle is 90 degrees.The longest side of a right angle is known as the hypotenuse, and it is opposite the right angle.
Given,
∠B= 90°, AC = 96 cm, ∠C = 30°
Now, we know, Trignometric ratio sin θ in triangle can be given by-:
sin θ = \(\frac{perpendicular}{hypotenuse}\)
From given figure,
Perpendicular= AB= ?
and Hypotenuse= AC = 96
and θ= 30°
Hence,
sin 30° =\(\frac{perpendicular}{hypotenuse}\)= \(\frac{AB}{96}\)
\(\frac{1}{2} = \frac{AB}{96}\) (∵ sin 30°= 1/2)
\(AB=\frac{96}{2}\)
\(AB= 48 cm\)
Thus, AB= 48 cm
Learn more about right angled triangle here:
https://brainly.com/question/3772264
#SPJ1
Correct Question:ABC is a right angled triangle. if B = 90°, AC = 96 cm, C = 30°. Find AB = ?

45% of the enrolment in a class is 18. Find the enrolment of the class.
Answers
Answer:
The enrollment is 40.
Step-by-step explanation:
\(.45x = 18\)
\(x = 40\)