Tyler borrowed $600. After one year, he paid the whole loan back, with a total maturity value of $640. What was the interest rate of the loan?
Answers
SOLUTION:
Step 1:
In this question, we are given the following:
Tyler borrowed $600. After one year, he paid the whole loan back, with a total maturity value of $640. What was the interest rate of the loan?
Step 2:
The details of the solution are as follows:
\(\begin{gathered} Principal\text{ = \$ 600} \\ Amount\text{ = \$ 640} \\ Interest\text{ = Amount - Principal = \$ 640 - \$ 600 = \$ 40} \end{gathered}\)\(Using\text{ Simple Interest = }\frac{Principal\text{ x Rate x Time}}{100}\)\(Then,\text{ we have that the Rate = }\frac{100\text{ x Simple Interest }}{Principal\text{ x Time}}\)\(\begin{gathered} where\text{ Simple Interest = \$ 40 ,} \\ Time\text{ = 1 year} \\ Principal\text{ = \$ 600} \end{gathered}\)\(Rate\text{ =}\frac{100\text{ x 40}}{600\text{ x 1}}=\frac{4000}{600}=\text{ 6}\frac{2}{3}\text{ \%}\)CONCLUSION:
The interest rate on the loan =
\(6\text{ }\frac{2}{3}\text{ \%}\)Related Questions
What is the equation of the line that passes through the point (-3, 7) and has a slope of -5/3?
Answers
The equation of the line that passes through the point (-3, 7) and has a slope of -5/3 is y - 7 = (-5/3)(x + 3).
We are given the point (-3, 7) and the slope of the line as -5/3.The slope-intercept form of a line is y = mx + b where m is the slope and b is the y-intercept.
To obtain the equation of the line, we need to substitute the values of slope and point in the slope-intercept form and solve for b.(7) = (-5/3)(-3) + b 21/3 = b.
Now we have the value of b, and we can substitute the values of m and b in the slope-intercept form.y = (-5/3)x + 21/3 is the equation of the line in slope-intercept form.
To obtain the equation in the standard form Ax + By = C, we multiply each term by 3.3y = -5x + 7Add 5x to both sides5x + 3y = 7.
This is the equation of the line in standard form.
For more question on equation
https://brainly.com/question/17145398
#SPJ8
Lorena's backpack has a mass of 15,000 grams.
What is the mass of Lorena's backpack in kilograms?
Lorena's backpack weighs
kilograms.
Answers
Answer:
1 kg = 1000 grams
15,000 grams = 15kg
Solve the inequality for x.
4x – 8 > 40
Answers
Answer:
x is greater then 12
Step-by-step explanation:
Answer:
X > 12
Step-by-step explanation:
4x > 40 + 8
4x > 48
Divide both sides by 4
X > 12
Pls be careful with this, I am not sure if the sign changes
if a line has the points (3,4) and (3,8) and it is dilated by a Scale Factor of 3.How long will the image of the line become?

Answers
The length of the image of the line, after being dilated by the scale factor, would be B. 12 units.
How to find the length after dilation ?To find the length of the image of the line after dilation, we need to calculate the distance between the two points on the dilated line.
The dilated line's points will be (3, 4 x 3) and (3, 8 x 3), which simplify to (3, 12) and (3, 24).
Then find the distance using the distance formula :
= √((x2 - x1) ² + (y2 - y1) ²)
= √((3 - 3) ² + (24 - 12) ²)
= √ (0 + 144)
= √144
= 12
Find out more on dilation at https://brainly.com/question/7354974
#SPJ1
Please help need quickly

Answers
The answer for number 1 is 1.72. The answer for number 2 is 4.83.
So for number one, the first thing you need to do is determine how many sides are on the first shape. Since it is a pentagon, and one pentagon has 5 sides, then you use the following formula to determine the volume of a\(V = \frac {1}{4} \times \sqrt{5 \times (5 +2 \times \sqrt {5})} \times (10)^{2} \times 10\) pentagon: \(\alpha = \frac{1}{4} \times \sqrt[]{5(5+2 \sqrt[]{5}) \alpha ^{2} }\) And in this case, the area would be 1.72, because if you insert the side lengths, the equation would look like this: \(V = \frac {1}{4} \times \sqrt{5 \times (5 +2 \times \sqrt {5})} \times (10)^{2} \times 10\) , which if you solve this equation, then you will end up with the answer of 1.72.
For number two, the first thing you need to do is determine how many sides are on the second shape. Since it is an octagon, and the prefix oct means 8, the number of sides on the shape is 8. If the perimeter is 64, and there are 8 sides, you can divide 64 by 8 to get 8. The equation to solve for the area should look like this: \(V = 2(1 + \sqrt{2}) \times s^{2} \times h\). Now all you need to do is insert the side lengths to the proper places. It should then look like this: \(V = 2(1 + \sqrt{2}) \times 8^{2} \times 8\), after you solve the equation, you should end up with 4.83.
A three section spinner shown, the three diagram shows the possible outcomes for the town spins.What is the probability of spinning the same number in both spins?

Answers
Answer:
\(Pr = \frac{1}{3}\)
Step-by-step explanation:
Given
\(n = 9\) --- total outcomes
Required
The probability of same number in both spins
The list of same outcomes are:
\(L = \{(1,1),(2,2),(3,3)\}\)
\(n(L) = 3\) ----- The number of outcomes
So, the probability is:
\(Pr = \frac{n(L)}{n}\)
\(Pr = \frac{3}{9}\)
\(Pr = \frac{1}{3}\)
help please thank you

Answers
Milly is in charge of a spaghetti dinner fundraiser. She must buy enough spaghetti to feed at least 75 people. Each box of spaghetti cost $2.29, and will serve 8 people. What is the least amount she must spend to have enough spaghetti?
A. $18.32
B. $20.61
C. $21.47
D. $22.90
Answers
Los 1600 euros de alquiler de un terreno se reparten entre tres ganaderos que llevan alli a pastar sus ovejas. Como no tienen el mismo número de ovejas, deciden pagar proporcionalmente al número de ovejas de cada uno. Si el primero tiene 120 Ovejas,el segundo 72 y el tercero 68. ¿ Cuánto paga cada uno?
Answers
So, each farmer pays the following amounts: The first farmer pays 738.40 euros. The second farmer pays 443.04 euros. The third farmer pays 418.56 euros
What is proportion?Proportion refers to the equality of two ratios. In other words, when two ratios are set equal to each other, they form a proportion. A proportion is typically written in the form of two fractions separated by an equals sign, such as a/b = c/d. Proportions are commonly used in mathematics to solve problems involving ratios and proportions, such as finding missing values or scaling up or down a given quantity.
Here,
To find out how much each farmer pays, we need to determine the proportion of the total rent that each farmer owes based on the number of sheep they have. First, we need to find the total number of sheep:
120 + 72 + 68 = 260
The first farmer has 120 sheep, which is 46.15% of the total number of sheep (120/260). Therefore, the first farmer owes 46.15% of the rent:
0.4615 x 1600 = 738.40 euros
Similarly, the second farmer has 72 sheep, which is 27.69% of the total number of sheep (72/260). Therefore, the second farmer owes 27.69% of the rent:
0.2769 x 1600 = 443.04 euros
The third farmer has 68 sheep, which is 26.15% of the total number of sheep (68/260). Therefore, the third farmer owes 26.15% of the rent:
0.2615 x 1600 = 418.56 euros
To know more about proportion,
https://brainly.com/question/29474065
#SPJ1
Complete question:
The rent of 1600 euros for a piece of land is divided among three farmers who graze their sheep there. As they do not have the same number of sheep, they decide to pay proportionally according to the number of sheep each has. If the first one has 120 sheep, the second 72, and the third 68. How much does each one pay?
The slope of the line perpendicular to the line: y= ½ x+ 10
Question 14 options:
-2
10
2
1/2
Answers
Answer: -2
Step-by-step explanation:
The slope of the line perpendicular to another slope is the opposite sign and flipped(reciprocal)
The slope of the line = 1/2
So the perpendicular slope = -2
If f(x)=x-9 and g(x)=-6x-3 which statement is true

Answers
Answer:
-1 is not in the domain of (f o g)(x)
Step-by-step explanation:
f(x) = sqrt(x - 9)
g(x) = -6x - 3
(f o g)(x) = f(g(x)) = sqrt(g(x) - 9)
(f o g)(x) = sqrt(-6x - 3 - 9)
(f o g)(x) = sqrt(-6x - 12)
Let x = -1:
(f o g)(-1) = sqrt(-6(-1) - 12)
(f o g)(-1) = sqrt(6 - 12)
(f o g)(-1) = sqrt(-6)
Since sqrt(-6) is not a real number, -1 is not in the domain of (f o g)(x).
work out the area of the circle
take pi to be 3.142 give your answer to 1 decimal place
radius 8
Answers
The area of the circle with the given radius is 201.088 square units.
What is area of a circle?The area of a circle is the space occupied by the circle in a two-dimensional plane. Alternatively, the space occupied within the boundary/circumference of a circle is called the area of the circle. The formula for the area of a circle is A = πr², where r is the radius of the circle.
Given that, the radius of a circle is 8 units.
Here, area of a circle
= 3.142×8²
= 3.142×64
= 201.088 square units
Therefore, the area of the circle is 201.088 square units.
Learn more about the area of a circle here:
brainly.com/question/28642423.
#SPJ9
Solve the equation. 72n–6 = 1
Answers
here you go i think im right

Juan is learning about like terms in his math class. He must check all the combinations below that are like terms which ones should he check
Answers
Step-by-step explanation:
please attach a picture for us to see the choices
2 x - 5 + 7 y - 3 = 9 x - 1 - y -8 Solve For X AND Y (SHOW WORK PLEASE)
(Just so people can get points :D)
Answers
Answer:
\(\large\boxed{\sf x = \frac{8y+1}{7} }\)
\(\large\boxed{\sf x = \frac{7x-1}{8} }\)
Group the Variable's:
2 x - 5 + 7 y - 3 = 9 x - 1 - y -8
2x -9x + 7y +y = -1 -8 +5 + 3
-7x + 8y = -1
From this find x and y
For X
-7x + 8y = -1
-7x = -1 -8y
7x = 8y + 1
x = (8y +1)/7
For Y
-7x + 8y = -1
8y = -1 +7x
y = (7x -1)/8
-9(-2x-3) simplified
Answers
Answer:
18x +27
Step-by-step explanation:
-9(-2x-3)
Distribute
-9 * -2x -9*(-3)
18x +27
What is the length of the
diagonal
of 144in²?
of a square with an area
HELP ASAP PLEASE SEE THIS I BEG!!!
Answers
The length of the diagonal of a square with an area of 144 in² is approximately 16.97 inches.
Calculating the length of diagonalThe diagonal of a square can be calculated using the Pythagorean theorem, which states that the square of the length of the diagonal (d) of a right triangle is equal to the sum of the squares of the lengths of its two other sides (a and b):
d² = a² + b²
In the case of a square, both of its sides have the same length (let's call it s), so we can rewrite the equation as:
d² = s² + s²
d² = 2s²
To find the length of the diagonal (d), we can take the square root of both sides:
d = √(2s²)
Now, we can use the area of the square to find the length of its side (s):
144 in² = s²
s = √144 in²
s = 12 in
Substituting s = 12 in into the equation for the diagonal, we get:
d = √(2s²) = √(2(12 in)²) = √(2 * 144 in²) = √288 in ≈ 16.97 in
Hence, the length of the diagonal is 16.97 inches.
Learn more on Calculating the length of diagonal here: https://brainly.com/question/25125518
#SPJ1
what is the product -9x(5-2x) A. -18x^2-45x B. -18x^2-45x C. -18x^2-45x D. 18x-45x
Answers
Answer:
18x^2-45x
Step-by-step explanation:
-9x(5-2x)
-9x*5=-45x
-9x*-2x=18x^2
18x^2-45x
coordinate plane with trapezoids TRAP and T prime R prime A prime P prime and points W, X, Y, and Z with points located at T 1 comma 1, R 2 comma 3, A 4 comma 3, P 5 comma 1, T prime 2 comma one half, R prime 2 and one half comma one and one half, A prime 3 and one half comma one and one half, P prime 4 comma one half, W 2 and one half comma negative one and one half, X 0 comma 3, Y 5 comma negative 1, and Z 6 comma 1
Trapezoid TRAP was dilated by a scale factor of one half to create trapezoid T'R'A'P'.
Which point is the center of dilation?
W
X
Y
Z
Answers
Answer:
Answer: X
Step-by-step explanation:
Answer:
B. X
BTW Jimin is living mochi!
Step-by-step explanation:

What is the perimeter of a square that has an area equal to 64
Answers
Answer:
P=4a=4·64=256
kira,boris,deshuan have a total of $119 in their wallets. Boris has 3 times waht deshuan has. Deshuan has $6 more than kira. How much do they have in their wallets.
Answers
Kira has $19, Boris has $75, and Deshuan has $25.
How much money does each person have in their wallets?We will denote as follows:
Kira has as K, Boris has as B, and Deshuan has as D.From information, we can set up equations:
B = 3D (Boris has 3 times what Deshuan has)
D = K + 6 (Deshuan has $6 more than Kira)
K + B + D = 119 (The total amount of money they have is $119)
Substituting equation 2 into equation 1, we have:
B = 3(K + 6)
Substituting equations 1 and 2 into equation 3:
K + 3(K + 6) + (K + 6) = 119
K + 3K + 18 + K + 6 = 119
5K + 24 = 119
5K = 119 - 24
5K = 95
K = 95 / 5
K = 19
Using equation 2, we can find D:
D = K + 6
D = 19 + 6
D = 25
Using equation 1, we can find B:
B = 3D
B = 3 * 25
B = 75.
Read more about equation
brainly.com/question/22688504
#SPJ1
suppose y varies directly as x, and y=48 when x=6. find x when y=72.
Answers
Using the constant of proportionality the value of x when y is 72 is 9.
What is a COP?
The ratio between two quantities that are directly proportional is the constant of proportionality. When two quantities grow and shrink at the same rate, they are directly proportional.
If y varies directly as x, it means that y can be expressed as a constant multiple of x, i.e., y = kx, where k is the constant of proportionality.
To find k, we can use the given information that y = 48 when x = 6 -
y = kx
48 = k(6)
k = 8
Now that we know k, we can use it to find x when y = 72 -
y = kx
72 = 8x
x = 9
Therefore, when x = 72, the value of x = 9.
To learn more about constant of proportionality from the given link
https://brainly.com/question/27598477
#SPJ1
The area of a square is 36 sq.cm, then its perimeter is a) 24 cm b) 6 cm c) 144 cm d) 36 cm
Answers
Answer:
a
Step-by-step explanation:
the perimeter (P) of a square is the sum of the 4 congruent sides.
the area of a square is calculated as
area = s² ( s is the length of a side )
here area is 36 , then
s² = 36 ( take square root of both sides )
s = \(\sqrt{36}\) = 6
then
P = 4s = 4 × 6 = 24 cm
simplify 3a/a^2-9a+18-2/a-6

Answers
You got this!!!
Answer:
A or C
Step-by-step explanation:
A broker has calculated the expected values of two different financial instruments X and Y. Suppose that E(x)= $100, E(y)=$90 SD(x)= 90$ and SD(y)=$8. Find each of the following.
a. E(X+ 10) and SD(X+ 10)
b. E(5Y) and SD(5Y)
c) E(X+ Y) and SD(X+ Y)
d) What assumption must you make in part c?
Answers
Expectation is linear, meaning
E(a X + b Y) = E(a X) + E(b Y)
= a E(X) + b E(Y)
If X = 1 and Y = 0, we see that the expectation of a constant, E(a), is equal to the constant, a.
Use this property to compute the expectations:
E(X + 10) = E(X) + E(10) = $110
E(5Y) = 5 E(Y) = $450
E(X + Y) = E(X) + E(Y) = $190
Variance has a similar property:
V(a X + b Y) = V(a X) + V(b Y) + Cov(X, Y)
= a^2 V(X) + b^2 V(Y) + Cov(X, Y)
where "Cov" denotes covariance, defined by
E[(X - E(X))(Y - E(Y))] = E(X Y) - E(X) E(Y)
Without knowing the expectation of X Y, we can't determine the covariance and thus variance of the expression a X + b Y.
However, if X and Y are independent, then E(X Y) = E(X) E(Y), which makes the covariance vanish, so that
V(a X + b Y) = a^2 V(X) + b^2 V(Y)
and this is the assumption we have to make to find the standard deviations (which is the square root of the variance).
Also, variance is defined as
V(X) = E[(X - E(X))^2] = E(X^2) - E(X)^2
and it follows from this that, if X is a constant, say a, then
V(a) = E(a^2) - E(a)^2 = a^2 - a^2 = 0
Use this property, and the assumption of independence, to compute the variances, and hence the standard deviations:
V(X + 10) = V(X) ==> SD(X + 10) = SD(X) = $90
V(5Y) = 5^2 V(Y) = 25 V(Y) ==> SD(5Y) = 5 SD(Y) = $40
V(X + Y) = V(X) + V(Y) ==> SD(X + Y) = √[SD(X)^2 + SD(Y)^2] = √8164 ≈ $90.35
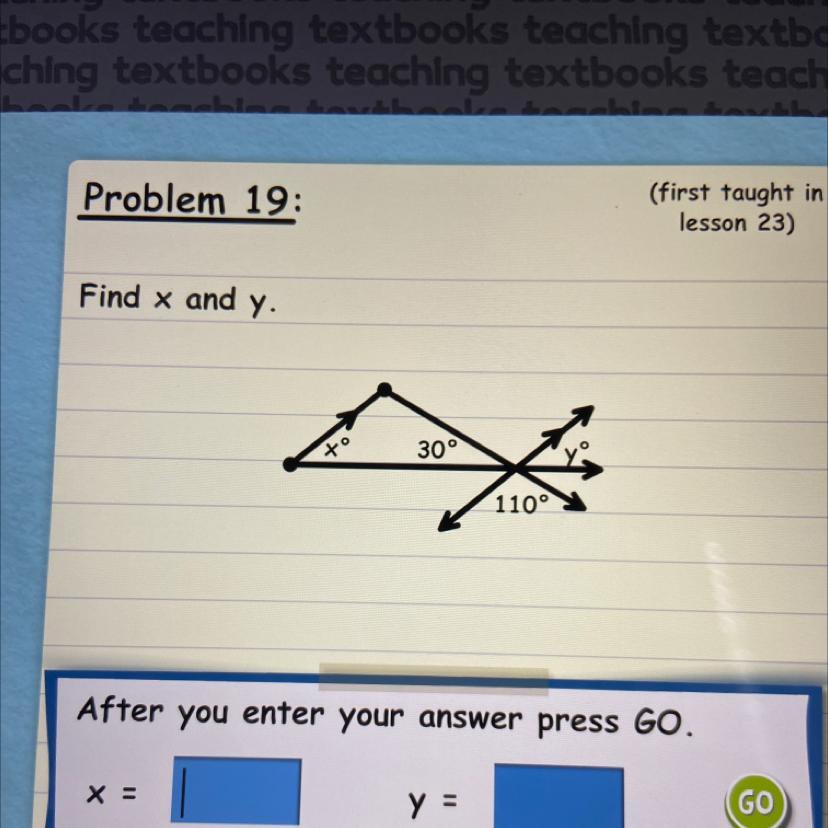
find x and y pls help
Answers
30 + 110 + 30 + 110 = 280
360-280 = 80
2y = 80
y = 40 degrees
If y is 40. Then the angle next to 30 is also y due to vertically opposite angles.
30 + 40 = 70
The line with 2 arrows is parallel to the line with x degrees. So, I use co-interior angles adds to 180.
In that c shaped, we got the bottom angle to be 70 & the top to be 110 because co-interior angles =180
Forget the c shape exists, and move onto the triangle that's visible. The top angle is 110 as found.
Angles in triangle add to 180.
180 - 110 - 30 = 40.
Thus, x = 40 degrees
There's a short cut I realise now because we can have alternate angles are equal; creating a z shape to find what x is equal to & that turns out to be the same value as y. ( due to vertically opposite angles)
Hope this helps!
(b) 1 kilogram 2.2 pounds, express your answer from part (a) in pounds. Answer: lbs [2]
Answers
Answer:
37
Step-by-step explanation:
Willy Wonka makes a 13 metre chocolate bar as
he believes that chocolate is best made as a giant piece rather than small packaged pieces. The chocolate bar is then cut into 4/11 of a metre. How many bars does this make?
Answers
The 35.75 bars are made from the 13 metre chocolate bar
How to determine how many bars the giant piece makes?
A fraction is a part of a whole. In arithmetic, the number is expressed as a quotient, in which the numerator is divided by the denominator
Given that:
Willy Wonka makes a 13 metre chocolate bar
The chocolate bar is then cut into 4/11 of a metre
Since she cut the chocolate bar into 4/11 of a metre and she makes 13 metre bar. The number of bars will be:
Number of bars = 13 / (4/11)
Number of bars = 35.75
Therefore, the 35.75 bars are made
Learn more about fractions on:
brainly.com/question/11562149
#SPJ1
Use the order of operations to evaluate this expression (-2+1)

Answers
Answer:
12
Step-by-step explanation:
2²×3
I hope its correct
Answer:
4
\( {( - 2 + 1)}^{2} + 5(12 \div 3) - 9 \\ 2 + 1 + 5 \times 4 - 9 \\ 3 + 20 - 9 \\ 23 - 9 \\ 14\)
pls help!! Thank you!

Answers
Answer:
C
Step-by-step explanation:
\(\left(\frac{f}{g} \right)(x)=\frac{f(x)}{g(x)} \\ \\ \frac{f(x)}{g(x)}=\frac{\sqrt[3]{2x}}{2x+1}\)
The denominator cannot equal 0, so:
\(2x+1 \neq 0 \implies x \neq -\frac{1}{2}\)