Thomas has a bag with 7 green marbles, 5 blue marbles, and 4 red marbles. For each part below, if the marble selected is replaced before the next marble is drawn, find the probability for the given draw :
A red marble?
A red or a green marble?
An Orange marble?
Answers
Using it's concept, the probabilities are given as follows:
A. 1/4.B. 11/16.C. 0.What is a probability?A probability is given by the number of desired outcomes divided by the number of total outcomes.In this problem, the number of marbles is given by:7 + 5 + 4 = 16.Then:For item a, 4 are red, hence the probability is 4/16 = 1/4.For item b, 4 are red and 7 are green, hence the probability is 11/16.For item c, there are no orange marbles, hence the probability is of 0.
Related Questions
Using simplex method to solve the following problems: (Manual calculations and then confirm your calculation by any software) Max. Z=5A+4B Subject to constraints: 6 A+4 B≤24, A+2 B≤6,−A+B≤1, B≤2, A, B≥0
Answers
Using the simplex method, the maximum value of Z=5A+4B is found to be 19.2 when A=3.6 and B=1.2. The calculations can be confirmed by using any software that solves linear programming problems.
To solve the given linear programming problem using the simplex method, we start by converting the problem into standard form. We introduce slack variables to convert the inequalities into equations.The initial tableau is as follows:
| A | B | S1 | S2 | S3 | S4 | RHS
------------------------------------------
Z | -5 | -4 | 0 | 0 | 0 | 0 | 0
------------------------------------------
S1 | 6 | 4 | 1 | 0 | 0 | 0 | 24
S2 | 1 | 2 | 0 | 1 | 0 | 0 | 6
S3 | -1 | 1 | 0 | 0 | 1 | 0 | 1
S4 | 0 | 1 | 0 | 0 | 0 | 1 | 2
We perform the simplex iterations until the optimal solution is reached. After applying the simplex method, the final tableau is obtained as follows:
| A | B | S1 | S2 | S3 | S4 | RHS
------------------------------------------------------
Z | 0 | 1.8 | 0.2 | -1 | -0.4 | 0.4 | 19.2
------------------------------------------------------
S1 | 0 | 0 | 0 | 1.5 | -1 | 1 | 3
S2 | 1 | 0 | -0.5 | 0.5 | 0.5 | -0.5 | 1.5
A | 1 | 0 | 0.5 | -0.5 | -0.5 | 0.5 | 0.5
S4 | 0 | 0 | 1 | -1 | -1 | 1 | 1
From the final tableau, we can see that the maximum value of Z is 19.2 when A=3.6 and B=1.2. This solution satisfies all the constraints of the problem. The calculations can be verified using any software that solves linear programming problems, which should yield the same optimal solution.
Learn more about linear programming here:- brainly.com/question/30763902
#SPJ11
2 fractions with different denominators that add up to 6 1/3
Answers
The two fractions with denominators of 2 and 6 that add up to 19/3 are 3/6 and 29/3, respectively.
First, let's break down what that mixed number means in terms of fractions. 6 1/3 is the same as 19/3.
Now, we need to find two fractions with different denominators that add up to 19/3. One way to do this is to use a common denominator. To find a common denominator, we need to find the least common multiple (LCM) of the two denominators.
Let's say we want to find two fractions that add up to 19/3, with denominators of 2 and 6. The LCM of 2 and 6 is 6. So, we can rewrite the fractions with a denominator of 6:
1/2 = 3/6
x/6
Now we need to find the value of x that makes the sum of the fractions equal to 19/3:
3/6 + x/6 = 19/3
To solve for x, we can multiply both sides by 6:
3 + x = 38/3
Then, we can subtract 3 from both sides:
x = 29/3
To know more about fraction here
https://brainly.com/question/10354322
#SPJ4
Lush Gardens Co. bought a new truck for $58,000. It paid $6,380 of this amount as a down payment and financed the balance at 4.88% compounded semi-annually. If the company makes payments of $1,800 at the end of every month, how long will it take to settle the loan? years months Express the answer in years and months, rounded to the next payment period
Answers
it will take approximately 3 years and 8 months to settle the loan.
To calculate the time it will take to settle the loan, we can use the formula for the future value of an ordinary annuity:
FV = P * ((1 + r)ⁿ - 1) / r
Where:
FV is the future value of the annuity (loan amount)
P is the payment amount ($1,800)
r is the interest rate per period (4.88% per annum compounded semi-annually)
n is the number of periods
The loan amount is the difference between the purchase price and the down payment:
Loan amount = $58,000 - $6,380 = $51,620
We need to solve for n, so let's rearrange the formula and solve for n:
n = (log(1 + (FV * r) / P)) / log(1 + r)
Substituting the values, we have:
n = (log(1 + ($51,620 * 0.0488) / $1,800)) / log(1 + 0.0488)
Using a calculator, we find:
n ≈ 3.66
This means it will take approximately 3.66 years to settle the loan. Since the company makes monthly payments, we need to convert this to years and months.
Since there are 12 months in a year, the number of months is given by:
Number of months = (n - 3) * 12
Substituting the value of n, we have:
Number of months = (3.66 - 3) * 12 ≈ 7.92
Rounding up to the next payment period, the company will take approximately 8 months to settle the loan.
Therefore, it will take approximately 3 years and 8 months to settle the loan.
Learn more about future value here
https://brainly.com/question/30787954
#SPJ4
Describe the end behavior of the function -2x^3-13x^2+8x+52
Answers
Answer:
Step-by-step explanation:
lim x -> +oo -2x³ - 13x² + 8x + 52
x³ (-2 -13/x + 8/x² + 52/x³)
+oo³ (-2 - 13/+oo + 8/+oo² + 52/oo³)
+oo(-2 + 0 + 0 + 0)
-oo
lim x -> -oo -2x³ - 13x² + 8x + 52
x³ (-2 -13/x + 8/x² + 52/x³)
-oo³ (-2 - 13/-oo + 8/-oo² + 52/-oo³)
-oo(-2 + 0 + 0 + 0)
+oo
WILL REWARD ASAP An error was made while solving the inequnlily -4(2x - 3) > 5x - 14. Choose the
step where the error was made
A. 8x + 12 = St - 14
B. -13+ 12 > -14
C. 1326
D.2
Answers
Answer:
a.
Step-by-step explanation:
-4 times 2x is -8x, not 8x.
Isabella worked 50 hours over the past two weeks, and she gets paid by the hour. During the first week of the two-week span, she worked 30 hours and got paid $285.00.
Answers
Isabella earns $9.50 per hour. She would've earned $475 for the entire 50 hours.
Answer:
Isabella earns $9.50 per hour. She earned $475 for the 50 hours.
Step-by-step explanation:
When planning a well-balanced long hair design, consider the proportional relationships between size, shape, texture and:
Answers
A well-balanced long hair design should consider the proportional relationships between size, shape, texture, and color to create a harmonious and visually pleasing hairstyle that flatters the client's features and personal style.
When planning a well-balanced long hair design, it is important to consider the proportional relationships between size, shape, texture, and color. These four elements work together to create a harmonious and visually pleasing hairstyle.
Size refers to the overall scale of the hairstyle, which can range from small and delicate to large and voluminous. It's important to consider the size of the client's head and face, as well as the desired level of impact.
Shape refers to the outline or silhouette of the hairstyle, which can be angular or rounded, symmetrical or asymmetrical. The shape should be chosen to flatter the client's face shape and features, as well as to create a balanced overall look.
Texture refers to the surface quality of the hair, which can be smooth or rough, sleek or tousled. Texture can be used to add interest and movement to the hairstyle, and should be chosen to complement the client's natural hair texture and the overall design.
Color refers to the hue, saturation, and tone of the hair, which can range from natural to bold and vibrant. Color can be used to enhance the shape and texture of the hairstyle, and should be chosen to flatter the client's skin tone and personal style.
In summary, a well-balanced long hair design should consider the proportional relationships between size, shape, texture, and color to create a harmonious and visually pleasing hairstyle that flatters the client's features and personal style.
To know more about hair design refer here:
https://brainly.com/question/31438531
#SPJ11
Can someone please explain how to do this problem:
n(x)=4x+15; n(x)=7
Thanks
Answers
Answer:
-2
Step-by-step explanation:
Of the last 14 contestants on a game show, 7 qualified for the bonus round. Considering this data, how many of the next 10 contestants would you expect to qualify for the bonus round?
a. 7/10
b. 3/7
c. 1/2
d. 1/3
Answers
Answer:
c. 1/2
Step-by-step explanation:
7 out of 14 contestants did last time, which is 1/2 of the contestants. You would assume that the same proportion would qualify when there are 10 contestants, so you would assume that 1/2 of 10 would also qualify for the bonus round.
A ___ is a system of basic rules and principles by which a government is organized.
Answers
Answer:
A Constitution is a system of basic rules and principles by which a government is organized.
1) Arnold has $200 in his bank account. His bank
account earns interest at a rate of 2% each year.
After 15 years, how much money will Arnold have
in his bank account (assuming no withdrawals or
deposits)?
Answers
Answer:
P is the principal amount, $200.00.
r is the interest rate, 2% per year, or in decimal form, 2/100=0.02.
t is the time involved, 15....year(s) time periods.
So, t is 15....year time periods.
To find the simple interest, we multiply 200 × 0.02 × 15 to get that:
The interest is: $60.00
what is 3 and 1/6 as an improper fraction
Answers
Answer:
Step-by-step explanation: 3×6+1,3×6 is 18+1 is 19/6 is your answer
find the exact value of cos-1 (sqrt 3/2)
Answers
The exact value of cos^-1( √3/2) = 30°
What is trigonometric ratio?The trigonometric functions are real functions which relate an angle of a right-angled triangle to ratios of two side lengths.
There are special angles in trigonometry, this angles have exact value and can be obtained without using calculator. Examples of this calculator are; 30°, 60°, 45°
sin30 = 1/2
cos 30 = √3/2
tan 30 = 1/√3
cos 60 = 1/2
sin 60 = √3/2
tan 60 = √3
Therefore, if cos^-1( √3/2) = x
cos x = √3/2
cos 30 = √3/2
cos x = cos 30
therefore x = 30
learn more about trigonometric ratio from
https://brainly.com/question/24349828
#SPJ1
20
Use the box and whisker plot to answer the following question.
73
81 84
70
→
65 70 75
80
Low temperatures (°F)
85
87
王
90
What is the interquartile range IQR of the data set? (IQR = Q3 - Q1)
Answers
The interquartile range IQR for the given data set is IQR=10
What is block and whisker plot?A box and whisker plot, often known as a box plot, shows a data set's five-number summary. The minimum, first quartile, median, third quartile, and maximum make up the five-number summary. A box is drawn from the first quartile to the third quartile in a box plot. At the median, a vertical line passes through the box.
How do you calculate box and whisker plot?Finding the median, lower quartile, and upper quartile of a given set of data (Q2, Q1, and Q3) is the first stage in creating a box-and-whisker figure. Now that you know how to calculate the interquartile range (IQR). The distance between the upper and lower quartiles is known as the interquartile range.
Lower data set:
70 73 81 84
Q1=73+81/2=77
Middle data set
65 70 75 80
Q2= 70+75/2=72.5
Upper data set
85 87 90
Q3=87
IQR=Q3-Q1=87-77=10
To learn more about box plot visit the link:
https://brainly.com/question/1523909
#SPJ1
The balance in two separate bank accounts grows each month at different rates. The growth rates for both accounts are represented by the functions f(x) = 2x and g(x) = 4x + 12. In what month is the f(x) balance greater than the g(x) balance? (2 points)
a
Month 6
b
Month 5
c
Month 4
d
Month 3
Answers
Pretty sure it's the 6 month
Suppose f(x) = - 3x² + 9x − 2. Compute the following:
A.) ƒ( − 2) + f(1) =
B.) ƒ( − 2) – ƒ(1) =
Answers
Step-by-step explanation:
\( f(x) = - 3 {x}^{2} + 9x - 2\)
A) f(-2) + f(1) = -32 + 4 = -28
B) f(-2) - f(1) = -32 - 4 = -36
Another 15 percent will be correct numbers, but no one is home and the answering machine picks up. In that case, the student is instructed to simply hang up and move on to the next phone number. Each of these calls takes about two minutes.
Answers
Each of these calls takes approximately two minutes, and when the answering machine picks up, the student must hang up and move on to the next phone number.
According to the given problem,15% will be correct numbers, but no one is home and the answering machine picks up. In that case, the student is instructed to simply hang up and move on to the next phone number. Each of these calls takes about two minutes.
Therefore, when the answering machine picks up, the student needs to hang up and move on to the next phone number, so no other time is wasted. The student may have difficulty at first, but with practice, the student will become more efficient and learn how to handle different situations effectively.
In addition, the student may learn how to better communicate and persuade people to support the cause or buy the product. Students will learn that rejection is a common occurrence in life, and that it is essential to persevere in the face of adversity.
Eventually, the student will be able to handle any situation and become a skilled salesperson. This ability can also be useful in other areas of life, such as job interviews and presentations.
In conclusion, making phone calls to solicit donations or sell a product is a valuable experience for students.
It teaches students the essential skills of perseverance, effective communication, and rejection management. It also allows students to become better salespeople, which can be beneficial in various aspects of life. Each of these calls takes approximately two minutes, and when the answering machine picks up, the student must hang up and move on to the next phone number.
Learn more about: calls
https://brainly.com/question/17325905
#SPJ11
You roll two (standard, six-sided) dice. What is the probability that you do not roll "double sixes"?
Answers
(a) Write an expression for a Riemann sum of a function f on an interval [a, b]. Explain the meaning of the notation that you use.
(b) If f(x)⩾ 0, what is the geometric interpretation of a Riemann sum? Illustrate with a diagram.
(c) If f(x) takes on both positive and negative values, what is the geometric interpretation of a Riemann sum? Illustrate with a diagram.
Answers
(a) The expression for a Riemann sum of a function f on an interval [a, b] is Δx = (b-a)/n
(b) If f(x)⩾ 0, then the geometric interpretation of a Riemann sum is infinity
(c) If f(x) takes on both positive and negative values, then the geometric interpretation of a Riemann sum is infinity
Riemann sums are an important tool in calculus for approximating the area under a curve. They are used to estimate the value of a definite integral, which represents the area bounded by the curve and the x-axis on a given interval. In this explanation, we will discuss the expression for a Riemann sum, its notation, and its geometric interpretation.
(a) Expression for a Riemann sum:
A Riemann sum is an approximation of the area under a curve using rectangles. We divide the interval [a, b] into n subintervals, each of length Δx=(b−a)/n. The notation used to represent this is:
Δx = (b-a)/n
(b) Geometric interpretation of a Riemann sum when f(x)⩾ 0:
If f(x) is always non-negative, the Riemann sum represents an approximation of the area between the curve and the x-axis on the interval [a, b].
Each rectangle has a positive area, which contributes to the overall area under the curve. The sum of the areas of the rectangles approaches the true area under the curve as the number of subintervals n approaches infinity.
(c) Geometric interpretation of a Riemann sum when f(x) takes on both positive and negative values:
When f(x) takes on both positive and negative values, the Riemann sum represents the net area between the curve and the x-axis on the interval [a, b].
Each rectangle may have a positive or negative area, depending on the sign of f(xi).
The positive areas represent regions where the curve is above the x-axis, and the negative areas represent regions where the curve is below the x-axis.
In conclusion, Riemann sums are used to approximate the area under a curve on an interval [a, b]. The expression for a Riemann sum involves dividing the interval into n subintervals and approximating the area under the curve using rectangles.
To know more about Riemann sum here.
https://brainly.com/question/30241844
#SPJ4
My brain is destroying itself so i need a little help I give brain award

Answers
help pls last question
The relationship between the number of days and
the height of a banana tree represents a linear
function because the banana tree's growth is
constant. Alani works at the garden center and
wants to write an equation to track the height of the
banana tree after d days. She writes the following
equation:

Answers
The equation allows us to chart the tree's development over time and forecast its ultimate height.
what is function ?A function is a mathematical formula that allots each input from one set, known as the domain, to precisely one output from another set, known as the range. A function can be used to model relationships between variables, answer issues, and make predictions. It can be represented by an equation, a graph, a table, or a verbal description. Numerous branches of mathematics, as well as disciplines like physics, engineering, finance, and computer science, make extensive use of functions. Typical instances of functions include: When a function is graphed, it always changes at the same rate and forms a straight line. They are frequently used to simulate relationships between two variables that are related linearly, such as time and location.
given
After d days, Alani created the following algorithm to measure the height of the banana tree:
h = d + 8
Since this function is linear, the banana tree's development will remain constant over time. According to the calculation, the tree starts out at a height of 8 units, and it increases by 1 unit per day after that.
We can enter a figure for d (the number of days) and solve for h using this equation (the height of the tree after d days). For instance, we can enter d = 10 into the calculation to get the height of the tree after 10 days:
h = 10 + 8
h = 18
Therefore, the altitude of the banana plant after 10 days would just be 18 units. This equation allows us to chart the tree's development over time and forecast its ultimate height.
To know more about function visit:
https://brainly.com/question/28193995
#SPJ1
Choose the correct answer.
Find the quadratic equation given the points (6,0), (-1,0), and (7,4).
h(x) = 1/2(x + 1)(x − 6)
h(x) = 2(x+6)(x − 1)
h(x) = 2(x + 1)(x - 6)
h(x):1/2(x+6) (x - 1)
Answers
The quadratic equation given the points (6,0), (-1,0), and (7,4).
The correct answer is h(x) = 1/2(x + 1)(x - 6).
To find the quadratic equation given the points (6,0), (-1,0), and (7,4), we can use the general form of a quadratic equation, which is \(h(x) = ax^2 + bx + c.\)
First, let's substitute the coordinates of the given points into the equation to create a system of equations:
For the point (6,0):
\(0 = a(6)^2 + b(6) + c ---- (1)\)
For the point (-1,0):
\(0 = a(-1)^2 + b(-1) + c ---- (2)\)
For the point (7,4):
\(4 = a(7)^2 + b(7) + c ---- (3)\)
We now have a system of three equations with three unknowns (a, b, c). We can solve this system to find the values of a, b, and c.
Solving the system of equations (1), (2), and (3), we find:
a = 1/2
b = -3/2
c = 0
Thus, the quadratic equation that satisfies the given points is:
h(x) = 1/2(x + 1)(x - 6).
For similar question on quadratic equation.
https://brainly.com/question/30916775
#SPJ11
twelve less than the product of three and a number
Answers
The phrase "twelve less than the product of three and a number" can be translated into an algebraic expression 3x - 12. We can use this expression to find the value of the expression for a given value of x or to write and solve an equation involving this expression.
The phrase "twelve less than the product of three and a number" can be translated into an algebraic expression. To do this, we need to assign a variable to the unknown number and then use multiplication and subtraction to represent the given information.
Let x be the unknown number. The product of three and x is 3x. Twelve less than 3x is 3x - 12. Therefore, the algebraic expression for "twelve less than the product of three and a number" is 3x - 12. This expression represents a value that is 12 less than three times the number x.
For instance, if we know that a number is 7, we can use this expression to find the value of "twelve less than the product of three and 7."3x - 12 = 3(7) - 12= 21 - 12= 9Therefore, the value of "twelve less than the product of three and 7" is 9. We can also use this expression to write an equation and solve for x. For example, if we know that the value of "twelve less than the product of three and a number" is 33, we can write an equation:3x - 12 = 33Then, we can solve for x:3x = 33 + 123x = 45x = 15. Therefore, the unknown number is 15.
To summarize, the phrase "twelve less than the product of three and a number" can be translated into an algebraic expression 3x - 12. We can use this expression to find the value of the expression for a given value of x or to write and solve an equation involving this expression.
Learn more about product from the given link
https://brainly.com/question/20451763
#SPJ11
find the value of each variable

Answers
Answer:
x = 16 y= 16√3
Step-by-step explanation:
we know that a angle is 60 degrees. Thanks to this information we can say that x is half the hypothenuse, so is value is 16.
y can be express as √3/2 x 32 = 16√3
Find the greatest common factor.
2ax2 + 2ax + 2a

Answers
The greatest common factor of the expression 2ax² + 2ax + 2a is 2a.
What is an expression?An expression is a way of writing a statement with more than two variables or numbers with operations such as addition, subtraction, multiplication, and division.
Example: 2 + 3x + 4y = 7 is an expression.
We have,
2ax² + 2ax + 2a
There are three terms.
The greatest common factor in each term is 2a
Now,
2a (x² + x + 1)
Thus,
The expression with the greatest common factor is 2a (x² + x + 1).
Learn more about expressions here:
https://brainly.com/question/3118662
#SPJ1
Find the future value of an account compounded quarterly at 5.67% for 4 years, if $7,000 was deposited into the account.
Answers
Step 1) : We know that,
\(\begin{cases}P = 7000& \\r = 5.6 \% & \\ n = 4 & \\ t = 4 & \end{cases}\)
Step 2) : Formulate and Substitute:
\(Substitute\begin{cases}P = 7000& \\ r = 5.6 \% & into \: formula \\ n = 4 & \\ t = 4 & \end{cases}\)
\(\bf\longrightarrow{F = P * (1 + \frac{r}{n} )^{(n*t)}} \)
Put the values according to the formula:
\(\bf\longrightarrow{F = 7000 * (1 + \frac{ \frac{5.67}{100} }{4} )^{(4*4)}}\)
Step 3) : Evaluate the equation/expression:
\(\bf\longrightarrow{8768.1}\)
Pls help i will mark brainliest if correctシ♡︎

Answers
Answer:
so
Step-by-step explanation:
it would be (3,1) is (-3,1)
next is (3,4) it would be (-3,4)
(1,4) is (-1,4)
have a nice day and if its right brainlest please?
Find three consecutive even numbers
such that the sum of three times the
smaller number and five times the larger
number is 40 more than twice the median
number.
Answers
Answer:
1, 4, 9
Step-by-step explanation:
1 x 3 = 3
9 x 5 = 45
total = 48
median = 4
twice median = 4×2
= 8
sum = 8 + 40 = 48
48=48
Write an exponential function to model the following situation.
A population of 130,000 grows 3% per year for 16 years.
How much will the popluation be after 16 years?
Write an exponential function in terms of x.
y=10

Answers
Answer:
y = 130,000(1.03)ˣ
Step-by-step explanation:
The general form of an exponetial function is y = abˣ, where a is the initial value (when x = 0), and b is the multiplier (the growth or decay factor). Here, we start with a population of 130,000, so our initial value a = 130,000. A growth of 3% each year represents a growth factor of 1.03, so after x years, the population y should be y = 130,000(1.03)ˣ.
The exponential function in terms of x and y is y = 130,000(1.03)ˣ
What is an exponential function ?The exponential function is a mathematical function denoted by
y = abˣ
where a is the initial value (when x = 0)
and b is the multiplier (the growth or decay factor).
population starts from 130,000, so
Initial value a = 130,000.
A growth of 3% each year represents a growth factor of 1.03,
so after x years, the population y should be
y = 130,000(1.03)ˣ
Therefore , The exponential function in terms of x and y is y = 130,000(1.03)ˣ
To know more about exponential function
https://brainly.com/question/11487261
#SPJ2
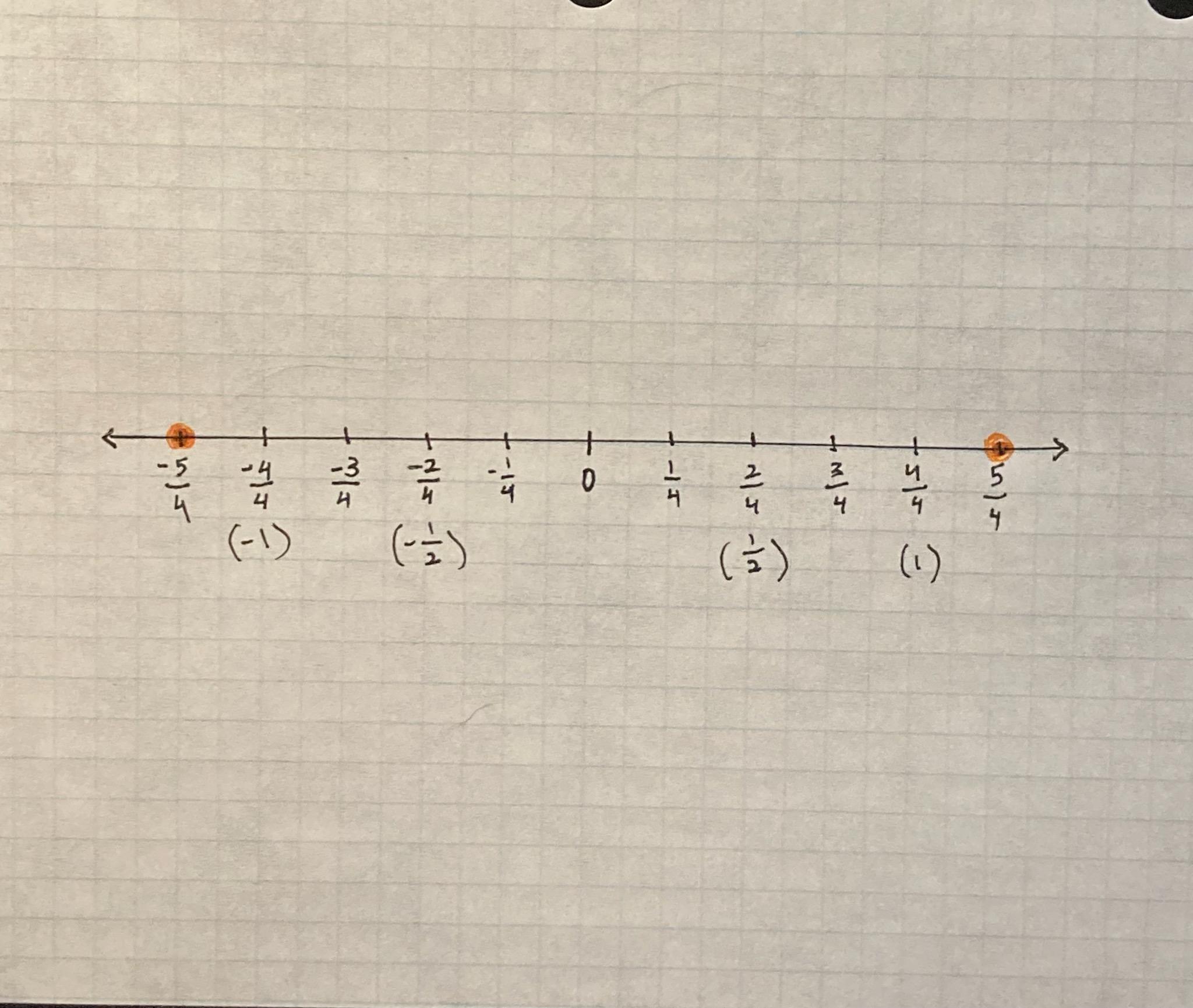
Represent -5 / 4 and 5 / 4 on number line
Answers
Answer:
Image included:
Step-by-step explanation: