These graphs represent functions f and g. Which ordered pair represents (f + g)(6) on the graph of the combined function? A. (12,14) B. (6,7) C. (6,14) D. (12,10)

Answers
Considering the given graphs, the ordered pair that represents (f + g)(6) is given by:
C. (6,14)
How to find the value of (f + g)(6)?This value is given by:
(f + g)(6) = f(6) + g(6).
The ordered pair is: (6, f(6)).
Looking at the graphs, we have that:
For function f, we have that when x = 6, y = 6, hence f(6) = 6.For function g, we have that when x = 6, y = 8, hence g(6) = 8.Then:
(f + g)(6) = f(6) + g(6) = 6 + 8 = 14.
Which means that option C is correct.
More can be learned about functions at https://brainly.com/question/25537936
#SPJ1
Related Questions
3x^2+5x^2
Tyyyy
Sorry about the bad photo quality.

Answers
Answer:
8x^2
7y^2+ 4y^3
3d^3+ 4d^2
Here is a scale drawing of a garden. Tom wants to plant a tree in the garden according to the following rules: It must be 4 m from A and 2 m from CD. Place a cross where Tom can plant the tree. D 2.5 cm A 4 cm C B 1 cm represents 2m

Answers
Answer:
the cross is the blue color

please help me im stuck

Answers
Answer:
a = -0.5
Step-by-step explanation:
\( \rm Solve \: for \: a: \\ \rm \longrightarrow - \dfrac{1}{4}a - 4 = \dfrac{7}{4}a - 3 \\ \\ \rm Put \: each \: term \: in \: - \dfrac{1}{4}a - 4 \: over \: the \\ \rm common \: denominator \: 4: \\ \rm - \dfrac{a}{4} - 4 = - \dfrac{a}{4} - \dfrac{16}{4} : \\ \rm \longrightarrow - \dfrac{a}{4} - \dfrac{16}{4} = \dfrac{7}{4}a - 3 \\ \\ \rm - \dfrac{a}{4} - \dfrac{16}{4} = \dfrac{ - a - 16}{4} : \\ \rm \longrightarrow \dfrac{ - a - 16}{4} = \dfrac{7}{4}a - 3 \\ \\ \rm Put \: each \: term \: in \: \dfrac{7}{4}a - 3 \: over \: the \\ \rm common \: denominator \: 4: \\ \rm \dfrac{7a}{4} - 3 = \dfrac{7a}{4} - \dfrac{12}{4} : \\ \rm \longrightarrow \dfrac{ - a - 16}{4} = \dfrac{7a}{4} - \dfrac{12}{4} \\ \\ \rm \dfrac{7a}{4} - \dfrac{12}{4} = \frac{7a - 12}{4} : \\ \rm \longrightarrow \dfrac{ - a - 16}{4} = \dfrac{7a - 12}{4} \\ \\ \rm Multiply \: both \: sides \: by \: 4: \\ \rm \longrightarrow \dfrac{ - a - 16}{ \cancel{4}} \times \cancel{4}= \dfrac{7a - 12}{ \cancel{4}} \times \cancel{4} \\ \\ \rm \longrightarrow -a - 16 = 7 a - 12 \\ \\ \rm Subtract \: 7 a \: from \: both \: sides: \\ \rm \longrightarrow (-a - 7 a) - 16 = (7 a - 7 a) - 12 \\ \\ \rm -a - 7 a = -8 a: \\ \rm \longrightarrow -8 a - 16 = (7 a - 7 a) - 12 \\ \\ \rm 7 a - 7 a = 0: \\ \rm \longrightarrow -8 a - 16 = -12 \\ \\ \rm Add \: 16 \: to \: both \: sides: \\ \rm \longrightarrow (16 - 16) - 8 a = 16 - 12 \\ \\ \rm 16 - 16 = 0: \\ \rm \longrightarrow -8 a = 16 - 12 \\ \\ \rm 16 - 12 = 4: \\ \rm \longrightarrow -8 a = 4 \\ \\ \rm Divide \: both \: sides \: of \: -8 a = 4 \: by \: -8: \\ \rm \longrightarrow
\dfrac{ - 8a}{ - 8} = \dfrac{4}{ - 8} \\ \\ \rm \dfrac{ - 8}{ - 8} = 1: \\ \rm \longrightarrow a = - \dfrac{4}{ 8} \\ \\ \rm - \dfrac{4}{ 8} = - \dfrac{1}{2} : \\ \rm \longrightarrow a = - \dfrac{1}{2} \\ \\ \rm \longrightarrow a = - 0.5\)
A fan is marked up 40% on the original price. The original price was $20. What is the new price of the fan before sales tax
Answers
Question 14 of 16
Identify the domain of the function shown in the graph
A. All positive integers
B. All real numbers
6. All positive real numbers
D. X>=0

Answers
B, because you would just assume the line continues forever on both sides.
What is the surface area of the triangular prism?
A.60 square feet
B.72 square feet
C.82 square feet
D.84 square feet

Answers
A rectangular prim mut have a bae with an area of no more than 27 quare meter. The width of the bae mut be 9 meter le than the height of the prim. The length of the bae mut be 6 meter more than the width of the bae. Find the maximum height of the prim. Let x = the height of the prim
x – 9 =
Answers
The rectangular prism will have a maximum height of prism is 12m and inequality is \(x^{2} -12x+0\leq 0\)
a) To find maximum height of prism
As per question,
x = height of prism
Area of rectangular base = Length x Width
Thus, A = \(\leq 27\)
Now,
L x W \(\leq 27\) (inequality Z)
Given, W = x - 9 (equation 1)
L = W + 6 (equation 2)
Substituting equation 1 in equation 2
We get, L = (x-9)+6
= x-3 (equation 3)
Substituting, equation 1 and equation 3 in the inequality Z
we get, \((x-3)(x-9) = x^{2} -12x+27\)
subtracting 27 we get \(x^{2} -12x+0\leq 0\)
Thus, the coordinates will be (0,12)
As given in question, width of base must be 9 meters less than height of prism. Therefore,
The solution of x interval comes out as (9,12)
Hence, the height of prism is 12m
b) selecting inequality that represents problem
Length = x-3
Width = x-9
Multiplying, we get \((x-3)(x-9) = x^{2} -12x+27\)
Area will come out as \(\leq 27\)
The final expression will be, \(x^{2}-12x+27\leq 27\)
Now subtracting 27 from the expression we get,
\(x^{2} -12x+0\leq 0\)
Note that the full question is:
A rectangular prism must have a base with an area of no more than 27 square meters. The width of the base must be 9 meters less than the height of the prism. The length of the base must be 6 meters more than the width of the base. Find the maximum height of the prism.
Let x = the height of the prism
x – 9 = the width of the base
A rectangular prism must have a base with an area of no more than 27 square meters. The width of the base must be 9 meters less than the height of the prism. The length of the base must be 6 meters more than the width of the base. Find the maximum height of the prism.
Let x = the height of the prism
x – 9 = the width of the base
x -3 = the length of the prism
Select the inequality that represents the problem.
x2 – 3 x – 81 ≤ 0
x2 – 3 x – 27 ≤ 0
x2 – 12 x – 27 ≤ 0
x2 – 12 x ≤ 0
To learn more about prisms: https://brainly.com/question/23766958
#SPJ4
Guys I had to construct a icosahedron for geometry class. I included a image so how does it look????? I’m really worried and i just want it to look good enough.

Answers
Answer:
it looks great! i'd give you an a+
honestly I couldn't make that
find the equation of the tangent plane to f(x, y) = x2 − 2xy 3y2 having slope 2 in the positive x direction and slope 2 in the positive y direction.
Answers
The equation of the tangent plane to f(x, y) = x^2 - 2xy + 3y^2, with slopes 2 in the positive x direction and 2 in the positive y direction, is 2x + 4y - 8 = 0.
To locate the equation of the tangent airplane to the floor described with the aid of the feature f(x, y) = \(x^2 - 2xy + 3y^2\), we need to decide the gradient vector and consider it at a given point.
The gradient vector will grant the ordinary vector to the tangent plane, and by way of the use of the slope information, we can discover the equation of the plane.
Calculate the partial derivatives of the feature with recognize to x and y:
f_x = 2x - 2y
f_y = -2x + 6y
Set up a device of equations the use of the given slope information:
f_x = 2
f_y = 2
Solve the machine of equations to discover the factor where the slopes are satisfied:
2x - 2y = 2 --> x - y = 1 --> x = y + 1
-2x + 6y = 2 --> -x + 3y = 1 --> -x = 1 - 3y --> x = 3y - 1
Setting the two expressions for x equal to every other:
y + 1 = 3y - 1
2 = 2y
y = 1
Substitute y = 1 into both expression for x:
x = 1 + 1
x = 2
Therefore, the factor the place the slopes are comfy is (2, 1).
Evaluate the gradient vector at the factor (2, 1):
grad(f) = (f_x, f_y) = (2x - 2y, -2x + 6y)
= (2(2) - 2(1), -2(2) + 6(1))
= (2, 4)
The ordinary vector to the tangent airplane is the gradient vector (2, 4).
Using the point-normal structure of the equation for a plane, the equation of the tangent airplane is:
2(x - 2) + 4(y - 1) + d = 0
To decide the price of d, alternative the coordinates of the factor (2, 1):
2(2 - 2) + 4(1 - 1) + d = 0
0 + 0 + d = 0
d = 0
The equation of the tangent airplane is:
2(x - 2) + 4(y - 1) = 0
Simplifying the equation, we have:
2x - 4 + 4y - 4 = 0
2x + 4y - 8 = 8
For similar question on tangent plane:
https://brainly.com/question/31433124
#SPJ11
In the diagram, the measures of 22, 23 and 26 are 40°. The measure of 21
is 140°. Are lines cand d parallel?

Answers
Answer:
c. Yes because ∠2 and ∠6 are congruent.
Step-by-step explanation:
From the picture attached,
m(∠2) = m(∠3) = m(∠6) = 40°
m(∠1) = 140°
Since (∠2 ≅ ∠6) (corresponding angles)
Therefore, by the converse theorem of corresponding angles lines c and d are parallel.
Option c is the answer.
Harry maid fruit punch using 2 parts Orange juice to 3 parts of soda water. The amount of soda water that Henry used is directly proportional to the amount of orange juice used. How many cups of orange juice should henry use with 18 cups of soda water?
Answers
Answer:
Step-by-step explanation:
j represents the amount of juice
2 : 3 :: j : 18 proportion
3j = (2)(18)
PLEASE HELP ASAP I GIVE BRAINLIEST TO FIRST CORRECT ANSWER (MATH)
Maryann is tracking the change in her vertical jump over 6 months. Use the table to write a linear function that models her jump distance.

Answers
Let inches be x
y = 1/2x + 16
Consider a male restroom design with minimum plumbing requirements of 12 water closets and 13 lavatories, which one of the following is closest to the minimum space required with considering urinal substitution? Select one: O a. 222 b. 219 c. 237 d. 249
Answers
none of the provided options (a, b, c, d) appear to be accurate or close to the minimum space required.
To determine the minimum space required for a male restroom design with the given plumbing requirements, we need to consider the minimum space required for water closets and lavatories.
The minimum space required for water closets is typically around 30-36 inches per unit, and for lavatories, it is around 24-30 inches per unit.
Since the design requires a minimum of 12 water closets and 13 lavatories, we can estimate the minimum space required as follows:
Minimum space required for water closets = 12 water closets * 30 inches = 360 inches
Minimum space required for lavatories = 13 lavatories * 24 inches = 312 inches
Adding these two values together, we get a total minimum space requirement of 672 inches.
Among the given options, the closest value to 672 inches is option d) 249. However, this value seems significantly lower than the expected minimum space requirement.
To know more about minimum visit:
brainly.com/question/21426575
#SPJ11
PLEASE help im struggling
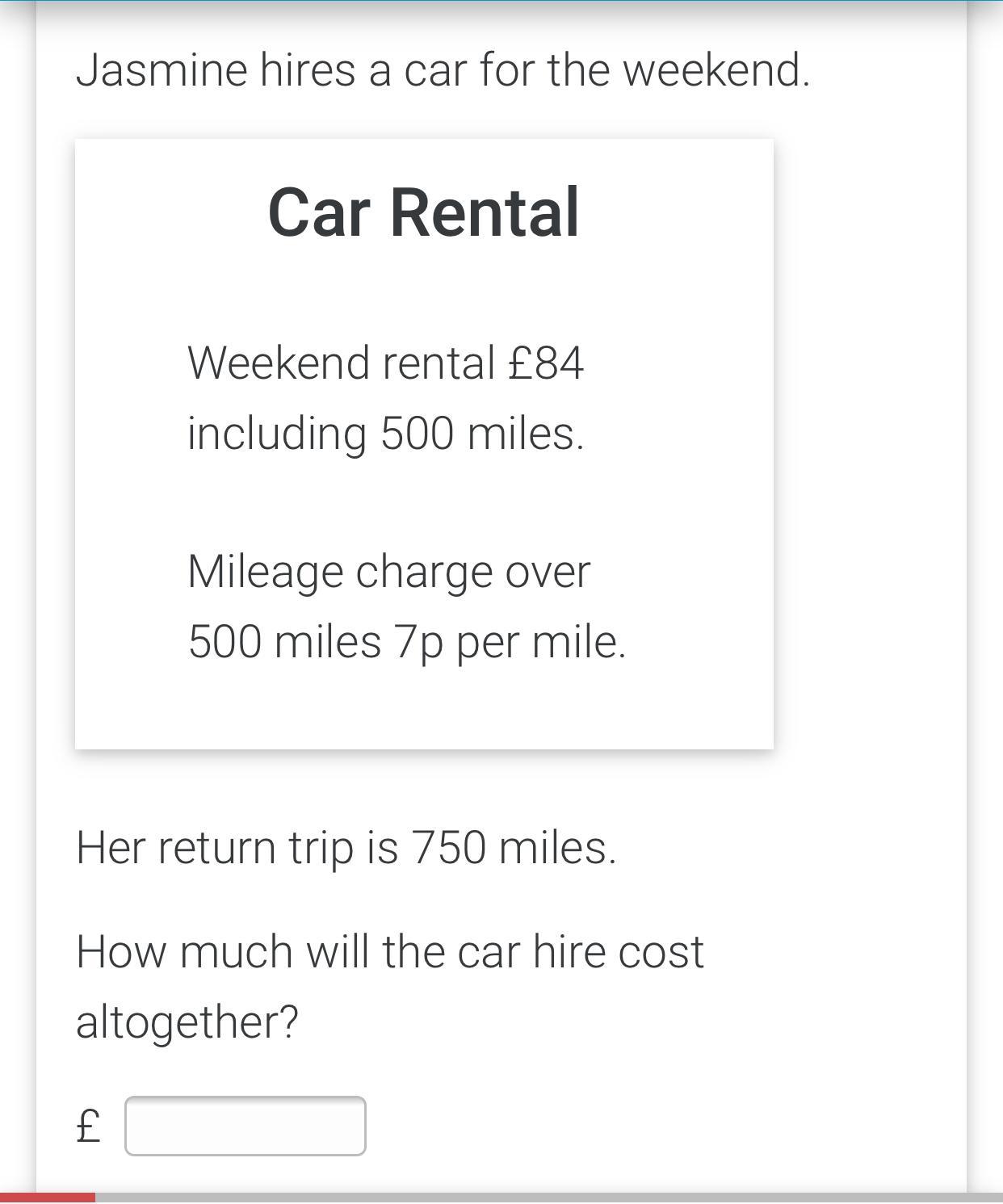
Answers
PLEASE HELP ME FAST!!! ( It's about y -intercept)

Answers
Answer:
The slope is 3
Step-by-step explanation:
(3x)-->slope=3
The average height of 10 trees is 2.6 meters. One of the trees grows from 1.7 meters to 2.2 meters. Calculate the new average of the 10 trees, giving your answer to two decimal places.
Answers
After the growth of one tree,the new average height of the 10 trees is 2.65 meters.
What is the new average height of the 10 trees?
To calculate the new average height of the 10 trees after the growth of one tree, we need to find the total height of all the trees, including the growth of the specific tree, and then divide it by the total number of trees.
Given:
Average height of 10 trees = 2.6 meters
We can calculate the total height of the 10 trees before the growth:
Total height before = Average height * Total number of trees = 2.6 meters * 10 = 26 meters
Now, we need to consider the growth of the specific tree. The growth is from 1.7 meters to 2.2 meters, which means the tree has grown by 2.2 - 1.7 = 0.5 meters.
To calculate the new total height, we add the growth to the previous total height:
New total height = Total height before + Growth = 26 meters + 0.5 meters = 26.5 meters
Since the total number of trees remains the same (10 trees), we can calculate the new average height:
New average height = New total height / Total number of trees = 26.5 meters / 10 = 2.65 meters
Therefore, the new average height of the 10 trees, after the growth of one tree, is 2.65 meters.
Learn more about Average height
brainly.com/question/31293464
#SPJ11
Ms reynolds has 5 pounds of clay for her art club. If she divides the clay into 1 third pound pieces, how many pieces will she have?
Answers
Answer:
15
Step-by-step explanation:
Total quantity of clay = 5 pound
Now, Reynolds divides the clay into 1 third pound pieces.
this means each piece of clay weighs = 1/3 pounds
Therefore, number of pieces of clay = Total weight of clay/ weight of each piece
= 5/(1/3) = 15
Answer the following questions concerning the poset ({3, 5, 9, 15, 24, 45}, D (the divisibility relation on the set) 1. Find the maximal elements 3 5 9 15 24 45 2. Find the minimal elements 3 5 9 15 24 45 3. Find the minimum, if it exists 3 5 9 15 24 45 none 4. Find the maximum, if it exists 03 05 09 15 24 45 none 5. Find all upper bounds of {3,5} 3 5 9 15 24 45 none 6. Find the least upper bound of {3,5} if it exists 3 5 9 15 24 45 none 7. Find all lower bounds of {15,45} 3 5 9 15 24 45 none 8. Find the greatest lower bound of {15,45} if it exists. 03 5 9 15 24 45 none
Answers
2. The minimal elements of the poset are: 3, 5, 9, 15, 24, 45.
3. The minimum does not exist since there is no element in the set that is less than or equal to all other elements in the set.
4. The maximum does not exist since there is no element in the set that is greater than or equal to all other elements in the set.
5. All upper bounds of {3,5} are: 9, 15, 24, 45.
6. The least upper bound of {3,5} is 15.
7. All lower bounds of {15,45} are: 3, 5, 9.
8. The greatest lower bound of {15,45} is 15.
PLEASE PLEASE AND ANWER FAST PLEASE!

Answers
Answer:
Option A. The student incorrectly used < instead of > for the second inequality. The correct inequality is >.
Step-by-step explanation:
Defintions:Boundary lines that are often used in systems of linear inequalities determine the location of the solutions region in a coordinate plane.
Solid boundary lines are used for the equal sign, "=," and the inequality symbols "≥" and "≤." This implies that the points along the line (endpoints) are included as solutions to the given system. Dashed boundary lines are used for inequality symbols, ">" and "<." Using the dashed lines as the boundary implies that the points along the line (endpoints) are not included as solutions to the given system. Solution:Given the systems of linear inequalities: y ≤ 0.5x + 1 and y = -2x + 1:
In order to determine what is the appropriate inequality symbols for both equations, we must first set the second equation with an equal sign. Then, choose a test point (not in either lines) to determine which half-plane region must be shaded.
Test point: (0, 0)
Substitute the test point into both equations to determine whether it satisfies the given equation:
y ≤ 0.5x + 10 ≤ 0.5(0) + 1
0 ≤ 0 + 1
0 ≤ 1 (True statement).
y = -2x + 20 = -2(0) + 2
0 = 0 + 2
0 = 2 (False statement). Therefore, the half-plane region that doesn't contain the test point must be shaded. Hence, we must use the ">" symbol for this equation, as we're shading "above" where the values for y is greater than the y-intercept, (0, 2).
Therefore, the correct answer is Option A: The student incorrectly used < instead of > for the second inequality. The correct inequality is > (greater than symbol).
In an online survey,42% of teens between the ages of 13 and 17 said they can text while blindfolded. Estimate the number of teens who can text while blindfolded from a class of each of the following number of students. 100 students.
Answers
Answer:
42 students
Step-by-step explanation:
1.) If 42% of students or 42 out of 100 students said they could text blindfolded it means 42 out of the 100 students surveyed can text blindfolded.
A percentage is out of 100
The population of a city grows exponentially at rate of 8% per year.Find the number of years it takes for the population to be doubled Give your answer correct to the nearest whole number
Answers
Answer:
It will take 9 years for the population to double
Step-by-step explanation:
Exponential Growth
The natural growth of some magnitudes can be modeled by the equation:
\(P=P_o(1+r)^t\)
Where P is the actual amount of the magnitude, Po is its initial amount, r is the growth rate and t is the time.
The population of a city grows at a rate of r=8% = 0.08 per year. We are required to find when (t) the population will double, or P=2Po.
Substituting in the equation:
\(2P_o=P_o(1+0.08)^t\)
Simplifying:
\(2=(1.08)^t\)
Taking logarithms:
\(\log 2=\log (1.08)^t\)
Applying the exponent property of logs:
\(\log 2=t\log (1.08)\)
Solving for t:
\(\displaystyle t=\frac{\log 2}{\log (1.08)}\)
Calculating:
\(t\approx 9\)
It will take 9 years for the population to double
How is dividing each side of > x greater than 0 by a negative value different from dividing each side by a positive value?
Answers
Step-by-step explanation:
When you divide both sides of an inequality by a positive number, the inequality sign remains the same. When you divide both sides of an inequality by a negative number, you must reverse the inequality sign.
For example:
8 > 2 is a true inequality.
Let's divide both sides by 2:
4 > 1 is also a true inequality. The inequality sign remained > since we divided by a positive number.
Now let's start again with
8 > 2, and divide both sides by -2.
-4 > -1 is false. When the inequality sign remains the same after dividing both sides by a negative number, the inequality is no longer true. To keep the inequality true, we must change the inequality sign when dividing both sides by a negative number.
8 > 2
Divide both sides by -1.
-4 < -1 is true.
In a class of students, there are 20 girls and 20 men, making a sum of 40 students in total. Among those students are two girls G1,G2, and two men M1, M2. All 40 students are randomly assigned into 10 study groups of 4 students each. (1) What is the probability that both girls G1, G2 are both assigned to the same group? (2) If the group where G1 has been assigned to has exactly 2 women, what is the probability that G2 is also in this group? (3) What is the probability that G1 is in a group with M1 and G2 is in a group with M2?
Answers
1) the probability that both girls G1, G2 are both assigned to the same group 0.0043, or 0.43 percent. 2) the probability that G2 is also in this group 0.0364, or 3.64 percent . 3) the probability is:P (G1 is in a group with M1, and G2 is in a group 0.8897, or 88.97 percent.
(1) The probability that both girls G1, G2 are both assigned to the same groupLet's assume that we are going to make a random selection. There are 40 individuals in the class, and we will select 4 at a time. As a result, there are C(40,4) ways to select 4 individuals, which equals 91,390 ways.
To place the two girls G1, G2 in a single group, there are C(38,2) ways to select two people from the remaining 38 individuals. As a result, the total number of ways to assign G1 and G2 to a single group is C(38,2) × C(36,2) × C(34,2) × C(32,2) × C(30,2) × C(28,2) × C(26,2) × C(24,2) × C(22,2) × C(20,2). That's 214,277,650,957,810,000.
So the probability is as follows:P (both girls in the same group) = C(38,2) × C(36,2) × C(34,2) × C(32,2) × C(30,2) × C(28,2) × C(26,2) × C(24,2) × C(22,2) × C(20,2) / C(40,4) × C(36,4) × C(32,4) × C(28,4) × C(24,4) × C(20,4) × C(16,4) × C(12,4) × C(8,4) × C(4,4)= 194,779,921 / 45,379,776,000= 0.0043, or 0.43 percent
(2)We already know that G1 has been assigned to a group with 2 women. As a result, there are C(20,2) ways to select 2 women to be in G1's group. Among the remaining 36 students, there are C(34,1) ways to select 1 additional woman and C(16,1) ways to select 1 man.
The number of ways to create this group is C(20,2) × C(34,1) × C(16,1). To calculate the probability, we need to divide by the number of ways to put G1 in a group with 2 women, which is C(40,4) / C(18,2).
So the probability is:P (G2 is in a group with G1, which has 2 women) = C(20,2) × C(34,1) × C(16,1) / (C(40,4) / C(18,2))= 4,080 / 111,930= 0.0364, or 3.64 percent
(3) Let's assume that we are going to make a random selection. There are 40 individuals in the class, and we will select 4 at a time. As a result, there are C(40,4) ways to select 4 individuals, which equals 91,390 ways. We need to determine the number of ways to put G1 in a group with M1 and G2 in a group with M2. As a result, we must choose 2 more people to join G1 and M1 and 2 more people to join G2 and M2.
There are C(38,2) ways to choose these two people, and C(36,2) ways to choose two more people from the remaining 36. The number of ways to create these groups is C(38,2) × C(36,2).
So the probability is:P (G1 is in a group with M1, and G2 is in a group with M2) = C(38,2) × C(36,2) / C(40,4)= 81,324 / 91,390= 0.8897, or 88.97 percent.
Know more about probability here,
https://brainly.com/question/31828911
#SPJ11
Let $a$, $b$, $c$, $d$, and $e$ be positive integers. The sum of the four numbers on each of the five segments connecting "points" of the star is 28. What is the value of the sum $a + b + c + d + e$?

Answers
Answer:
i dunno
Step-by-step explanation:
somebody help
Answer:
Step-by-step explanation:
\(a+c=23\\a+d=20\\e+c=21\\b+e=24\\d+b=22\\\)
...
√9pr / (pr)^-3/2 =
A. √3pr
B. 3/pr
C. 3√pr
D. 3pr
E. 3p²r²
Answers
Answer:
E
Step-by-step explanation:
=√9pr / (pr)^-3/2
=3√(pr) × (pr)^3/2
= 3p²r²
Answer:
E. 3p²r²
Step-by-step explanation:
√9pr / (pr)^-3/2=
3(pr)^1/2*(pr)^3/2=
3(pr)^(1/2+3/2)=
3(pr)^2=3p^2r^2
what verbal (written) scale from inches to feet would represent a map whose representative fraction (rf) scale is 1:48,000? (1 foot
Answers
If the representative fraction scale is 1:48000, then the verbal scale from inches to feet is 1 inch represents 0.00025 feet.
We have to find the verbal (written) scale from inches to feet that represents a map with a representative-fraction (RF) scale of 1:48,000,
We use the formula:
⇒ Verbal scale = RF × Inches per foot,
We know that the RF scale is 1:48,000, which means that one unit on the map represents 48,000 units in the real world.
There are 12 inches in a foot,
So, we can convert the verbal scale to inches-per-foot by dividing by 12:
⇒ Inches per foot = (Verbal scale)/(12),
⇒ Inches per foot = (1/48000) × (12/1) = 0.00025
Therefore, the verbal scale from inches to feet is : 1 inch represents 0.00025 feet.
Learn more about Scale here
https://brainly.com/question/30530331
#SPJ4
Please help me I need to finish this :)

Answers
Estimate: 42 x 58 im not good a estimating problems
Answers
Answer:
2,400
Step-by-step explanation:
A person has utility function u(x, y) = 100xy + x + 2y. Suppose that the price per unit of x is $2, and that the price per unit of y is $4. The person receives $1 000 that all has to be spent on the two commodities x and y. Solve the utility maximization problem
Answers
Answer:
x = 250
y = 125
u(x,y) = 3125500
Step-by-step explanation:
As given,
The utility function u(x, y) = 100xy + x + 2y
\(P_{x}\) = 2 , \(P_{y}\) = 4
Now,
Budget constraint -
\(P_{x}\) x + \(P_{y}\) y = 1000
⇒2x + 4y = 1000
So,
Let v(x, y) = 2x + 4y - 1000
Now,
By Lagrange Multiplier
Δu = Δv
⇒< 100y + 1, 100x + 2 > = < 2, 4 >
By comparing, e get
100y + 1 = 2 ........(1)
100x + 2 = 4 .........(2)
Divide equation (2) to equation (1) , we get
\(\frac{100y + 1}{100x + 2} = \frac{1}{2}\)
⇒2(100y+1) = 1(100x+2)
⇒200y + 2 = 100x + 2
⇒200y = 100x
⇒2y = x
Now,
As 2x + 4y = 1000
⇒2x + 2(2y) = 1000
⇒2x + 2x = 1000
⇒4x = 1000
⇒x = 250
Now,
As 2y = x
⇒2y = 250
⇒y = \(\frac{250}{2}\) = 125
∴ we get
x = 250
y = 125
Now,
u(250, 125) = 100(250)(125) + 250 + 2(125)
= 3125000 + 250 + 250
= 3125000 + 500
= 3125500
⇒u(250, 125) = 3125500
The maximized value of the utility function is $3125500
The utility function is given as:
\(U(x,y) = 100xy + x + 2y\)
The prices per unit are also given as:
\(P_x = 2\)
\(P_y = 4\)
When a person receives $1000, then the budget function is:
\(2x + 4y = 1000\)
Divide through by 2
\(x + 2y = 500\)
Differentiate the utility function with respect to x and y
\(U'(x) = 100y + 1\)
\(U'(y) = 100x + 2\)
So, we have:
\(U'(x) = P_x\) and \(U'(y) = P_y\)
The above equations become
\(100y + 1 = 2\) and \(100x + 2 = 4\)
Divide both equations
\(\frac{100y + 1}{100x + 2} = \frac 24\)
Reduce the fractions
\(\frac{100y + 1}{100x + 2} = \frac 12\)
Cross multiply
\(100x + 2 = 200y + 2\)
Subtract 2 from both sides
\(100x = 200y\)
Divide both sides by 100
\(x = 2y\)
Recall that:
\(x + 2y = 500\)
So, we have:
\(2y + 2y = 500\)
\(4y=500\)
Divide through by 4
\(y=125\)
Recall that:
\(x = 2y\)
So, we have:
\(x = 2 \times 125\)
\(x = 250\)
Also, we have:
\(U(x,y) = 100xy + x + 2y\)
Substitute the values of x and y, in the above function
\(U(250,125) = 100 \times 250 \times 125 + 250 + 2 \times 125\)
\(U(250,125) = 3125500\)
Hence, the maximized value of the utility function is 3125500
Read more about utility functions at:
https://brainly.com/question/24922430
Calculate the lower confidence limit (LCL) and upper confidence limit (UCL) of the mean for each of the following. bar x= 160, n = 436, sigma = 30, and alpha = 0.01 bar x = 70, n = 323, sigma = 4, and alpha = 0.05 LCL =
Answers
LCL and UCL values of both scenarios are (158.61,161.39),(69.65,70.35) respectively.
To calculate the lower confidence limit (LCL) and upper confidence limit (UCL) for each given scenario, you'll need to use the following formula:
LCL = X - (z * (sigma / √n))
UCL = X+ (z * (sigma / √n))
where X is the sample mean, n is the sample size, sigma is the population standard deviation, and z is the z-score corresponding to the desired confidence level (1 - alpha).
First Scenario:
X = 160, n = 436, sigma = 30, alpha = 0.01
1. Find the z-score for the given alpha (0.01).
For a two-tailed test, look up the z-score for 1 - (alpha / 2) = 1 - 0.005 = 0.995.
The corresponding z-score is 2.576.
2. Calculate LCL and UCL.
LCL = 160 - (2.576 * (30 / √436)) ≈ 158.61
UCL = 160 + (2.576 * (30 / √436)) ≈ 161.39
First Scenario Result:
LCL = 158.61
UCL = 161.39
Second Scenario:
X= 70, n = 323, sigma = 4, alpha = 0.05
1. Find the z-score for the given alpha (0.05).
For a two-tailed test, look up the z-score for 1 - (alpha / 2) = 1 - 0.025 = 0.975.
The corresponding z-score is 1.96.
2. Calculate LCL and UCL.
LCL = 70 - (1.96 * (4 / √323)) ≈ 69.65
UCL = 70 + (1.96 * (4 / √323)) ≈ 70.35
Second Scenario Result:
LCL = 69.65
UCL = 70.35
Learn more about LCL and UCL here: brainly.com/question/29048041
#SPJ11