There are 16 sweets in a bowl.
4 of the sweets are blackcurrant.
5 of the sweets are lemon.
7 of the sweets are orange.
Anna, Ravi and Sam each take at random one sweet from the bowl.
Work out the probability that the 5 lemon sweets are still in the bowl.
Answers
Answer:
5
Step-by-step explanation:
Related Questions
an estate agent earns 7% commission on the selling price of a farm . Calculate the commission that he will earn on a farm that was sold for 2,8 million
Answers
The commission of the agent is 196000
How to determine the commission of the agentFrom the question, we have the following parameters that can be used in our computation:
Commission percentage = 7%
Earnings = 2.8 million
Using the above as a guide, we have the following:
Commission = 7% * Earnings
Substitute the known values in the above equation, so, we have the following representation
Commission = 7% * 2.8 million
Evaluate
Commission = 196000
Hence, the commission is $196000
Read more about commission at
https://brainly.com/question/26283663
#SPJ1
scores on an exam were normally distributed. ten percent of the scores were below 62 and 80% were below 81. find the mean and standard deviation of the scores.
Answers
the mean (μ) of the scores is approximately 297.51, and the standard deviation (σ) is approximately 184.09.
To find the mean and standard deviation of the scores, we can use the information about the normal distribution and the given percentiles.
Let's denote the mean as μ and the standard deviation as σ.
From the information provided:
1. Ten percent of the scores were below 62. This corresponds to the percentile 10%.
Using a standard normal distribution table or a calculator, we can find the z-score corresponding to the 10th percentile, which is approximately -1.28.
Using the z-score formula: z = (X - μ) / σ, where X is the score, we have:
-1.28 = (62 - μ) / σ
2. Eighty percent of the scores were below 81. This corresponds to the percentile 80%.
Using a standard normal distribution table or a calculator, we can find the z-score corresponding to the 80th percentile, which is approximately 0.84.
Using the z-score formula: z = (X - μ) / σ, where X is the score, we have:
0.84 = (81 - μ) / σ
Now we have a system of equations with two variables (μ and σ):
Equation 1: -1.28 = (62 - μ) / σ
Equation 2: 0.84 = (81 - μ) / σ
Solving this system of equations will give us the values of μ and σ.
From Equation 1, we can rearrange it to get:
62 - μ = -1.28σ
Substituting this expression into Equation 2:
0.84 = (81 - (-1.28σ)) / σ
0.84 = (81 + 1.28σ) / σ
0.84σ = 81 + 1.28σ
0.84σ - 1.28σ = 81
-0.44σ = 81
σ ≈ -81 / -0.44
σ ≈ 184.09
Substituting the value of σ into Equation 1:
62 - μ = -1.28 * 184.09
62 - μ ≈ -235.51
μ ≈ 62 + 235.51
μ ≈ 297.51
Therefore, the mean (μ) of the scores is approximately 297.51, and the standard deviation (σ) is approximately 184.09.
Learn more about standard deviation here
https://brainly.com/question/13498201
#SPJ4
A box has 11 marbles, 3 of which are white and 8 of which are red. A sample of 4 marbles are selected randomly from the box without replacement. What is the probability that exactly 2 are white and 2 are red
Answers
14/99
Select 1 marble; the chance that it is white is 4/12. Select a 2nd marble; the chance that it is white is 3/11. Select a 3rd; the chance it is white is 2/10. Select a 4th; the chance it is red is 8/9. Select a 5th; the chance it is red is 7/8. The chance of getting this specific set of 5 marbles in this order is (4/12)×(3/11)×(2/10)×(8/9)×(7/8)=(2×7)/(11×10×9).
This specific set could occur in the permutation of 5 things taken 5 at a time where 3 are identical (white), and the other 2 are also identical (red). The formula for this is 5!/(3!2!)=10.
Combining the chance of getting white, white, white, red, red with the number of ways 3 white and 2 red could have been distributed in the draw of 5 marbles gives the answer:
{(2×7)/(11×10×9)}×10=14/99
A similar process will show that the chance of getting 5 red marbles is 7/99; 4 white and 1 red is 1/99; 2 white and 3 red is 42/99; and 1 white and 4 red is 35/99.
1 x (3 + 7) = (1 x 3) + (1 x 7) is an example of which property?
Answers
Answer:
distributive property
Step-by-step explanation:
they distribute the 1 to the 3 and 7 to make it easier
hope this helps
The expression 1 x (3 + 7) = (1 x 3) + (1 x 7) is an example of the distributive property of the addition.
What is Algebra?The analysis of mathematical representations is algebra, and the handling of those symbols is logic.
This property states that multiplying the total of two or so more arithmetic operations by a number will provide the same outcome as multiplying each required infrastructure by the number separately and then joining the results together.
The expression is given below.
1 x (3 + 7) = (1 x 3) + (1 x 7)
The expression is an example of the distributive property of the addition.
More about the Algebra link is given below.
https://brainly.com/question/953809
#SPJ6
what fraction is the greatest in this row? 12/4, 4/4, 2 2 / 4, 1/4. also 2 2 /4 is a mixed number
Answers
Answer:
12/4
Step-by-step explanation:
4/4 is 1
1/4 is well 1/4
2 2/4 is 2 1/2
12/4 is 3
the greatest fraction is 3
Answer:
12/4
Step-by-step explanation:
What is the slope of the line represented by the equation y = - 2/3 - 5x?
-5
-2/3
2/3
5
Answers
Answer:
The slope is the bit attached to the x:
In this case, it is -5.
Step-by-step explanation:
Answer: -5
just took the quiz
consider this function: f(x)=-4x+7 . which polynomial is equivalent to f(f(x))?
Answers
The polynomial equivalent to the given function f(f(x)) is 16x - 21.
What is degree of a polynomial?The largest exponential power in a polynomial equation is called the polynomial's degree. Each polynomial's degree is determined only by its variables; coefficients are should be disregarded. x is the variable with the biggest power of n in an nth degree polynomial function with real coefficients, where n accepts whole integer values.
The given function is f(x)=-4x+7.
To find the value of f(f(x)) substitute the value of x = f(x).
(f(x)) = f(-4x + 7)
= -4(-4x + 7) + 7
= 16x - 21
Therefore, the polynomial equivalent to the given function f(f(x)) is 16x - 21.
Learn more about degree of polynomial here:
https://brainly.com/question/29182596
#SPJ1
Let z denote a random variable having a normal distribution with = 0 and = 1. Determine each of the following probabilities. P(−0.5 < z < 0.87) P(−0.87 < z < −0.5)
P(−0.87 < z < −0.5)
P(−0.5 < z < 0.87
Answers
Given that z denotes a random variable having a normal distribution with mean (μ) = 0 and standard deviation (σ) = 1, we can use the standard normal distribution table (also known as the z-table) to determine the probabilities of the given intervals.
P(−0.5 < z < 0.87) = 0.2974 - 0.1915 = 0.1059
To get this answer, we use the z-table to find the area under the standard normal distribution curve between z = -0.5 and z = 0.87. The z-table provides the area to the left of any given z-value, so we subtract the area to the left of z = -0.5 from the area to the left of z = 0.87 to get the area between those two values.
P(−0.87 < z < −0.5) = 0.1915 - 0.0668 = 0.1247
To get this answer, we use the z-table to find the area under the standard normal distribution curve between z = -0.87 and z = -0.5. Again, we subtract the area to the left of z = -0.87 from the area to the left of z = -0.5 to get the area between those two values.
P(−0.87 < z < −0.5) = 0.0668
To get this answer, we simply use the z-table to find the area under the standard normal distribution curve between z = -0.87 and z = -0.5.
P(−0.5 < z < 0.87) = 0.1059
This is the same answer as the first probability since the intervals are symmetric about z = 0.
Know more about probabilities here:
https://brainly.com/question/30034780
#SPJ11
Solve with explanation

Answers
The length of arc FH in the circle 8.37 units.
We know that,
In mathematics, a circle is a closed two-dimensional shape that consists of all the points in a plane that are a fixed distance (called the radius) from a given point (called the center). Circles are used in many areas of mathematics and science, including geometry, trigonometry, and physics. They have important applications in areas such as engineering, architecture, and computer graphics.
Here,
To find the length of arc FH, we first need to find the measure of angle FGH in degrees. Since the sum of angles in a triangle is 180 degrees, we can use the fact that angles FGH and FGF are supplementary to find:
m∠FGF = 180 - m∠FGH = 180 - 86 = 94 degrees
Since FGF is an inscribed angle that intercepts arc FH, the measure of arc FH is twice the measure of angle FGF. So we have:
m(arc FH) = 2 × m∠FGF = 2 × 94 = 188 degrees
To find the length of arc FH, we need to know the circumference of circle G. Since we know that FG = 16 units, we can use this to find the radius of the circle:
r = FG/2 = 16/2 = 8 units
The circumference of the circle is then:
C = 2πr = 2π(8) = 16π
To find the length of arc FH, we need to find the fraction of the circumference that arc FH represents, and then multiply this by the total circumference. Since the measure of arc FH is 188 degrees out of a total of 360 degrees in the circle, we have:
Length of arc FH = (188/360) × C
Substituting the value of C, we get:
Length of arc FH = (188/360) × 16π
Simplifying and rounding to the nearest hundredth, we get:
Length of arc FH ≈ 8.37 units
To know more about circle,
brainly.com/question/26533660
#SPJ1
Work out the mean of √50, √18 and √8.
Give your answer in the form of a√2.
Show full working out.
Thank you!! <3
Answers
\(\frac{\sqrt{50}+\sqrt{18}+\sqrt{8}}{3} = \frac{10}{3} . \sqrt{2}\)
ok done. Thank to me :>
What is the slope – intercept form of the linear equation 7x – y = 14?
Answers
Answer:
y=7x+14
Step-by-step explanation:
-y=14-7x
y=-14+7x
y=7x-14
Simplify 7root 3divide by root 10 +root3
Answers
\(\huge{\colorbox{pink}{Hope It Helps You ! }}\)

Answer:
\(\sqrt{30}-3\)
Step-by-step explanation:
Write the given expression in a numerical format:
\(\frac{7\sqrt{3}}{\sqrt{10}+\sqrt{3}}\)
A logical first step to take in this problem is to convert the denominator to a rational value. Currently, the denominator is an irrational value, meaning that it is a never-ending value. One wants it to be a rational value. This can be done by multiplying the denominator by its conjugate. The conjugate of this number is simply the denominator with the second addend times negative one. Remember to multiply both the numerator and denominator by this value, as a number over itself is the same as multiply by (1) Use this idea here:
\(\frac{7\sqrt{3}}{\sqrt{10}+\sqrt{3}}\)
\(=\frac{7\sqrt{3}}{\sqrt{10}+\sqrt{3}}*\frac{\sqrt{10}-\sqrt{3}}{\sqrt{10}-\sqrt{3}}\)
Simplify,
\(=\frac{7\sqrt{3}}{\sqrt{10}+\sqrt{3}}*\frac{\sqrt{10}-\sqrt{3}}{\sqrt{10}-\sqrt{3}}\)
\(=\frac{7\sqrt{3}(\sqrt{10}-\sqrt{3})}{(\sqrt{10}+\sqrt{3})(\sqrt{10}-\sqrt{3})}\)
\(=\frac{7\sqrt{30}-7\sqrt{3*3}}{\sqrt{10*10}-\sqrt{3*3}+\sqrt{10*3}-\sqrt{10*3}}\)
Note that any value times itself under the radical is equal to the number, meaning (\(\sqrt{a*a}=a\)). Apply this to the problem,
\(=\frac{7\sqrt{30}-7\sqrt{3*3}}{\sqrt{10*10}-\sqrt{3*3}+\sqrt{10*3}-\sqrt{10*3}}\)
\(=\frac{7\sqrt{30}-7*3}{10-3}\)
\(=\frac{7\sqrt{30}-21}{7}\)
\(=\sqrt{30}-3\)
Randomly select a painted rock from a bag containing 4 purple rocks, 3 green rocks, 3 orange rocks, and 2 blue rocks.
Answers
Answer:
i got a orange
Step-by-step explanation:
the unit value of a cubic centimeter is the same as which metric measurement?
Answers
Answer: The unit value of a cubic centimeter (cm^3) is the same as the metric measurement of a milliliter (mL).
This is because 1 milliliter is equal to 1 cubic centimeter. In other words, if you have a cube that measures 1 centimeter on each side, its volume would be 1 cubic centimeter, which would also be equivalent to 1 milliliter of volume.
This relationship between cm^3 and mL is commonly used in scientific and medical measurements involving liquids and gases.
The unit value of a cubic centimeter (cc) is equivalent to one milliliter (mL) in the metric system. Both cubic centimeters and milliliters are used to measure volume, and their conversion is straightforward: 1 cc = 1 mL.
The metric system uses base units such as meters, liters, and grams, and applies prefixes like kilo-, centi-, and milli- to indicate larger or smaller units of measurement.
Cubic centimeters are often used to measure the volume of solid objects or the capacity of containers, while milliliters are more commonly used to measure the volume of liquids. However, both units represent the same volume and can be used interchangeably.
It is important to understand the difference between volume measurements and other metric measurements, such as length or mass. For instance, meters are used to measure length or distance, and grams are used to measure mass or weight. These units cannot be directly converted to cubic centimeters or milliliters, as they represent different physical properties.
In summary, a cubic centimeter (cc) is a unit of volume in the metric system that is equivalent to one milliliter (mL). Both units can be used to measure volume, and they have a simple conversion of 1 cc = 1 mL. Understanding the relationship between these units and other metric measurements is essential for accurately quantifying and comparing different physical properties.
To learn more about a cubic centimeter, refer:-
https://brainly.com/question/16670888
#SPJ11
How many solutions does this equation have. QUESTION BELOW please help

Answers
Answer:
one solution
Step-by-step explanation:
the solution to a system of equations given graphically is at the point of intersection of the 2 lines, that is (1, 2 )
Since there is only 1 point of intersection then there is only one solution.
im not sure what to say there is my question
Answers
The cube root of 60 is 20
Answers
False, the actual cube root of 60 is 3.9.
The result can be shown in multiple forms. Exact Form: 3√60 60 3. Decimal Form: 3.91486764
Answer:
False
Step-by-step explanation:
The cube root of 60 cannot be simplified, as there are no factors to factor out of the equation. This makes the statement false.
Yoni drove a total of 9/24 of a mile on Tuesday and Wednesday. She drove 1/6 of a mile on Tuesday.
How far did Yoni drive on Wednesday?
9/24mile
1/6 mile
5/24mile
24 miles
Answers
As per the total distance, the distance that Yoni drive on Wednesday is option (c) 5/24 miles.
To find out how far Yoni drove on Wednesday, we must first determine the total distance she drove over the two days.
Tuesday, Yoni drove 1/6 of a mile, which can be expressed as 6/24 of a mile. Wednesday, she drove an additional 9/24 of a mile.
This means that, in total, she drove 15/24 of a mile.
Since we already know that Yoni drove 6/24 of a mile on Tuesday, we can subtract this amount from the total distance she drove over the two days to find out how far she drove on Wednesday.
Subtracting 6/24 of a mile from 15/24 of a mile, we get 9/24 of a mile, which is the distance Yoni drove on Wednesday.
Therefore, Yoni drove 5/24 of a mile on Wednesday
Then the correct option is (c).
To know more about total here.
https://brainly.com/question/14286201
#SPJ4
How many lines of symmetry does this triangle have? 10 ОА. о OB. 1 O c. 2 OD. 3 O E 4
Answers
Answer: D
Step-by-step explanation: to know symmetry you must draw line from vertices of the figure ad the triangle has 3 vertices so you will draw 3 lines
Nicky is trying to drink 2.5 liters of water a day. She drank 0.878 liters after breakfast,
1.2 liters after lunch, and 0.75 liters before dinner. How much did she drink
all together?
Answers
Answer:
2.8 liters
She drank 2.8 liters all together.
Step-by-step explanation:
0.878+1.2+0.75= 2.8
Nicky drank a total of 2.828 liters of water throughout the day.
To find out how much water Nicky drank altogether, you can add up the amounts of water she drank throughout the day.
0.878 liters after breakfast.
1.2 liters after lunch.
0.75 liters before dinner
Adding these values together:
0.878 + 1.2 + 0.75 = 2.828
Therefore, Nicky drank a total of 2.828 liters of water throughout the day.
To learn more on Addition click:
https://brainly.com/question/29560851
#SPJ6
Using ONLY the 16 rules of Natural Deduction (you cannot use logical equivalences), prove the following: P→ (QVR) ¬(P→Q) :. R
Answers
To prove the argument P → (Q ∨ R), ¬(P → Q) :. R using only the 16 rules of Natural Deduction, we can proceed as follows:
1) Assume P → (Q ∨ R) and ¬(P → Q) as premises.
2. Assume ¬R as an additional assumption for a proof by contradiction.
3. Using the conditional elimination rule (→E) on (1), we get Q ∨ R.
4. Assume Q as an additional assumption.
5. Using the disjunction introduction rule (∨I) on (4), we have Q ∨ R.
6. Assume P as an additional assumption.
7. Using the conditional elimination rule (→E) on (1) with (6), we get Q ∨ R.
8. Using the disjunction elimination rule (∨E) on (3), (5), and (7), we derive R.
9. Using the reductio ad absurdum rule (¬E) on (2) and (8), we conclude ¬¬R.
10. Using the double negation elimination rule (¬¬E) on (9), we obtain R.
11. Using the conditional introduction rule (→I) on (6)-(10), we infer P → R.
12. Using the disjunctive syllogism rule (DS) on (2) and (11), we obtain Q.
13. Using the conditional elimination rule (→E) on (1) with (6), we derive Q ∨ R.
14. Using the disjunction elimination rule (∨E) on (3), (12), and (13), we derive R.
15. Using the reductio ad absurdum rule (¬E) on (2) and (14), we conclude ¬¬R.
16. Using the double negation elimination rule (¬¬E) on (15), we conclude R.
Therefore, we have successfully derived R from the given premises using only the 16 rules of Natural Deduction.
To learn more about logical equivalences click on,
https://brainly.com/question/32717781
#SPJ4
A department store sells a pair of shoes with an 87% markup.
If the store buys the shoes for $19.00, what is the markup price?
Answers
Answer:
35.53
Step-by-step explanation:
19.00x 87%=16.53
16.53+19.00= 35.53
Simplify this fraction (multi choice)
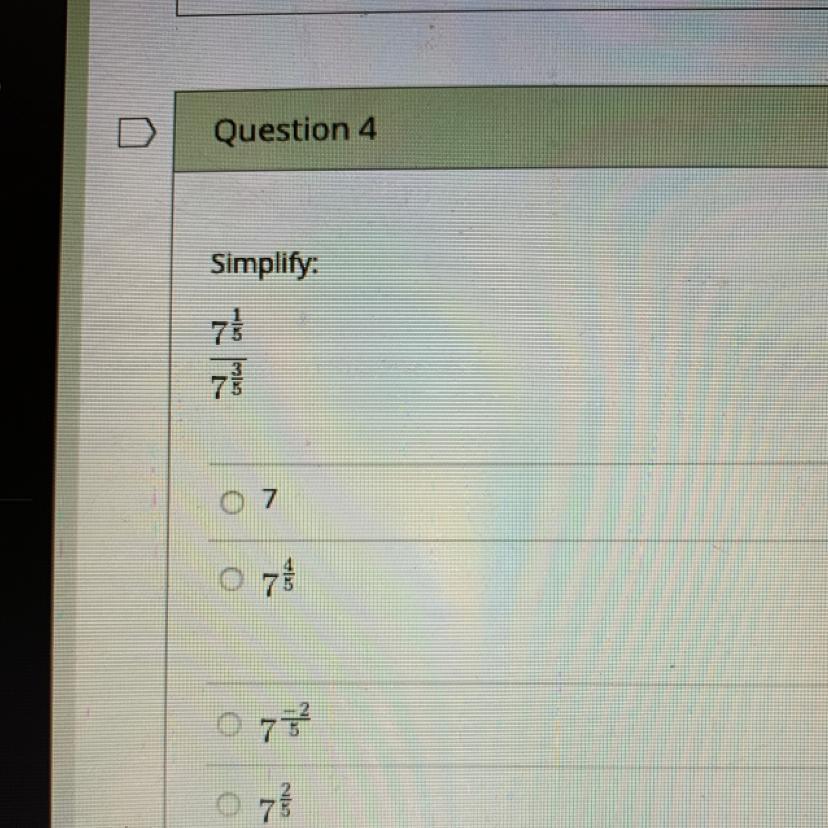
Answers
1/5 - 3/5 = -2/5 as exponent
It would be 7^-2/5
The third response is correct
approximately how many kilocalories are in a snack bar that contains 6 grams of fat, 10 grams of carbohydrate, and 2 grams of protein?
Answers
Answer:
Therefore, there are approximately 102 kilocalories in the snack bar.
Step-by-step explanation:
One gram of fat provides 9 kilocalories (kcal), while one gram of carbohydrate and protein provide 4 kcal each. So, to find the total number of kilocalories in the snack bar, we can use the following formula:
Total kcal = (grams of fat * 9) + (grams of carbohydrate * 4) + (grams of protein * 4)
Plugging in the values, we get:
Total kcal = (6 * 9) + (10 * 4) + (2 * 4)
Total kcal = 54 + 40 + 8
Total kcal = 102
Therefore, there are approximately 102 kilocalories in the snack bar.
The answer is approximately 120 kilocalories in a snack bar that contains 6 grams of fat, 10 grams of carbohydrate, and 2 grams of protein.
What are kilocalories? Kilocalories, also known as calories, are a unit of energy used to calculate the amount of energy in food. The number of kilocalories in food is calculated based on the number of grams of protein, carbohydrate, and fat it contains. Kilocalories are used by the body to fuel its processes and maintain its vital functions. The energy in food is released when it is digested, absorbed, and transported to the cells where it is used to produce ATP, the body's primary source of energy. ATP is used to power cellular processes such as metabolism, respiration, and movement. How many kilocalories are in a snack bar that contains 6 grams of fat, 10 grams of carbohydrate, and 2 grams of protein? To calculate the number of kilocalories in a snack bar that contains 6 grams of fat, 10 grams of carbohydrate, and 2 grams of protein, we need to know the number of kilocalories per gram of each macronutrient. Protein and carbohydrates each contain 4 kilocalories per gram, while fat contains 9 kilocalories per gram. To calculate the number of kilocalories in the snack bar, we multiply the number of grams of each macronutrient by the number of kilocalories per gram and then add them together. Here is the calculation:6 grams of fat x 9 kilocalories per gram of fat = 54 kilocalories10 grams of carbohydrate x 4 kilocalories per gram of carbohydrate = 40 kilocalories2 grams of protein x 4 kilocalories per gram of protein = 8 kilocalories. Total kilocalories = 54 + 40 + 8 = 102Therefore, a snack bar that contains 6 grams of fat, 10 grams of carbohydrate, and 2 grams of protein has approximately 102 kilocalories.
A snack bar with 6 grams of fat, 10 grams of carbohydrate, and 2 grams of protein has approximately 102 kilocalories. This is calculated as follows: (6 grams fat x 9 kcal/g) + (10 grams carbohydrate x 4 kcal/g) + (2 grams protein x 4 kcal/g).
Therefore, answer is 120 kilocalories.
To know more about carbohydrates visit : https://brainly.com/question/29775112
#SPJ11
a scuba diver dived 54ft below sea level to find an oyster. he then swam up 20 ft to open the oyster to see if it contained a pearl. how many ft below sea level was he when opened the oyster
Answers
Answer:
The diver was 34 feet below sea level
simplify 2 by 5 into 4 by 7 + 21 by 35
Answers
\( \frac{2}{5} \times \frac{4}{7} + \frac{21}{35} \\ = \frac{8}{35} + \frac{21}{35} \\ = \frac{8 + 21}{35} \\ = \frac{29}{35} \)
Answer:\( \frac{29}{35} \)
Hope it helps.
Do comment if you have any query.
Consider the function g(x)=−(x−1)^3−2. Which ordered pair lies on the inverse of the function?
(62,−3)
(−4, 123)
(3, 1)
(3,−6)
Answers
The ordered pair lie on the inverse of the function is (62,−3).
Option A is the correct answer.
What is a function?A function is a relationship between inputs where each input is related to exactly one output.
Example:
f(x) = 2x + 1
f(1) = 2 + 1 = 3
f(2) = 2 x 2 + 1 = 4 + 1 = 5
The outputs of the functions are 3 and 5
The inputs of the function are 1 and 2.
We have,
f(x) = -(x - 1)³ - 2
The inverse of f(x).
y = -(x - 1)³ - 2
interchange x and y and solve for y.
x = -(y - 1)3 - 2
(y - 1)³ = -2 - x
(y - 1)³ = -(2 + x)
Cuberoot on both sides.
y - 1 = ∛-(2 + x)
y = ∛-(2 + x) + 1
Now,
Substitute in the inverse of g(x).
(62, -3) = (x, y)
(−4, 123) = (x, y)
(3, 1) = (x, y)
(3,−6) = (x, y)
So,
y = ∛-(2 + x) + 1
y = ∛-(2 + 62) + 1
∛-1 = -1
y = -1∛64 + 1
y = -1 x 4 + 1
y = -4 + 1
y = -3
So,
(62, -3) ______(1)
And,
y = ∛-(2 + x) + 1
y = ∛-(2 - 4) + 1
∛-1 = -1
y = ∛(-2 + 4) + 1
y = ∛2 + 1
y = 1.26 + 1
y = 2.26
So,
(-4, 2.26) _______(2)
And,
y = ∛-(2 + x) + 1
y = ∛-(2 + 3) + 1
∛-1 = -1
y = -1∛5 + 1
y = -1 x 1.71 + 1
y = -1.71 + 1
y = -0.71
So,
(3, -0.71) _______(3)
And,
y = ∛-(2 + x) + 1
y = ∛-(2 + 3) + 1
∛-1 = -1
y = -1∛5 + 1
y = -1 x 1.71 + 1
y = -1.71 + 1
y = -0.71
So,
(3, -0.71) ______(4)
Thus,
From (1), (2), (3), (4) we see that,
(62, -3) is the solution to the inverse of g(x).
Learn more about functions here:
https://brainly.com/question/28533782
#SPJ1
• Determine whether the two triangles are congruent or not using the Hypotenuse-
Leg Theorem.
• Show all of your work to earn full credit.
• Use the "moutain" icon to upload a picture of your hand-written work.
A
-8
-4
y₁
0
-4
-8
B
-C
D
E
14
TI
F
X
8

Answers
The two triangles are not similar by HL theorem.
What are similar triangles?Similar triangles are triangles that have the same shape, but their sizes may vary. All equilateral triangles, squares of any side lengths are examples of similar objects. In other words, if two triangles are similar, then their corresponding angles are congruent and corresponding sides are in equal proportion.
each small square on the graph is 2 units,
from ΔABC BC = 8 - 4 which is 4
AB = 0 -- 8 which is 8
by Pythagoras
AC^2 = AB^2 +BC^2
AC^2 = (8)^2 + (4)^2
AC^2 = 64 + 16
AC^2 = 80
AC = \(\sqrt{80}\) which is 4\(\sqrt{5}\)
In ΔDEF,
DE = 2 --8 which is 10
EF = 6 - 2 which is 4
By Pythagoras
DF^2 = DE^2 + EF^2
DF^2 =(10)^2 + (4)^2
DF^2 = 100 + 16
DF^2 = 116
DF = \(\sqrt{116}\)
The hypotenuse AC is not equal to the hypotenuse DF. Therefore the two triangles are not similar by HL theorem.
In conclusion the two triangles are not similar by HL theorem
Learn more about similar triangles: https;//brainly.com/question/11920446
#SPJ1
what mistake did they make?

Answers
Answer:
this is not a good angle cant see it that well
Step-by-step explanation:
Answer:
the mistake is that the person added 2x by-4x which should've been -2x
Step-by-step explanation:
-4x+2x is basically saying 2-4 which is -2
Solve for b 15=3b
Please I need help
Answers
15/3 = 3b/b
5= b
Isolate the variable by diving 3 on both sides