The school cafeteria sells two kinds of wraps: vegetarian and chicken. The vegetarian wrap costs $1.00 andthe chicken wrap costs $3.10. Today they made $268.30 from the 136 wraps sold. How many of the wraps
sold were vegetarian?
a. 57 wraps
c. 63 wraps
d. 75 wraps
b. 73 wraps
Answers
Answer:
I think it is 73
Step-by-step explanation:
Because i tryed all of the other numbers and they didnt work
HOPE THIS HELP !!!!!!!!!!!!!!!! :)
Related Questions
Stuck on this question:
Solve 6(2y-3)-10=2y
Answers
Answer:
Exact form: y = \(\frac{14}{5}\)
Decimal form: y = 2.8
Step-by-step explanation:
Distribute:
6(2y - 3) - 10 = 2y
12y - 18 - 10 = 2y
Subtract the numbers:
12y - 18 - 10 = 2y
12y - 28 = 2y
Add 28 to both sides:
12y - 28 = 2y
12y - 28 + 28 = 2y + 28
Simplify:
Add the numbers
12y = 2y + 28
Subtract 2y from both sides:
12y = 2y + 28
12y - 2y = 2y + 28 - 2y
Simplify:
Combine like terms
10y = 28
Divide both sides by the same factor:
10y = 28
10y/10 = 28/10
Simplify:
Cancel terms that are in both the numerator and denominator
Divide the numbers
y = 14/5
Math 7 Review:
Question 4
What is the solution to 2(x−4)=16?
Answers
Answer:
x = 12
Step-by-step explanation:
2(x-4)=16
2 times x +2 times -4 = 16
2x -8 =16
2x -8 (+8) =16 (+8)
2x = 24
2x (divided by 2) = 24 (divided by 2)
x = 12
Diane has a rope that is 8 meters long. She cuts away a piece that is 1.67 meters long. How long is the remaining piece of rope?
Answers
Answer:
6.33 m
Step-by-step explanation:
Total length of the rope = 8 m
Cut length of the rope = 1.67 m
We need to find the remaining piece of the rope.
Remaining piece = Total length - cur length
= 8 m - 1.67 m
= 6.33 m
So, 6.33 m is the remaining piece of the rope.
compare 0.8 and 7/10
Answers
Answer:
O.8 is greater than 7/10
Step-by-step explanation:
0.8 is in the tenths Place So you know that equals 80 ones Out of one hundred. So you have 80/100 if you divide 80 and 100 by ten it reduces to 8/10. You know that 8/10 is greater than 7/10. Hope this helped .
Age of Automobile (years)
5
3
1
8
10. ) The table shows the ages of automobiles
in the parking lot.
a. The range of the ages is 12 years. Find
the missing age.
10
6
9
?
b. ) Find and interpret the interquartile range.
lot The range and interartile
Answers
a. To find the missing age, we can calculate the range of the ages. The range is the difference between the maximum and minimum values. Given that the range is 12 years, we can subtract the minimum age from the maximum age to find the missing age:
Maximum age - Minimum age = Range
10 - 5 = 12
The missing age is 7 years.
b. The interquartile range (IQR) is a measure of statistical dispersion that represents the range of the middle 50% of the data. To find the IQR, we need to calculate the first quartile (Q1) and the third quartile (Q3).
In this case, the ages in the parking lot are: 5, 3, 1, 8, 10. We need to arrange them in ascending order: 1, 3, 5, 8, 10.
To find Q1, we need to determine the median of the lower half of the data: 1, 3, 5. The median of this set is 3.
To find Q3, we need to determine the median of the upper half of the data: 5, 8, 10. The median of this set is 8.
The IQR is calculated as the difference between Q3 and Q1:
Q3 - Q1 = 8 - 3 = 5
Interpreting the interquartile range, we can say that the middle 50% of the ages of automobiles in the parking lot has a spread of 5 years. It gives us an idea of the dispersion of ages within the central portion of the data set.
To know more about the range and interquartile range, click here: brainly.com/question/29204101
#SPJ11
Jordan bought 3.8 pounds of turkey, 2.2 pounds of cheese, and 3.6 pounds of egg salad for a party. What was the total cost before sales tax? Round your answer to the nearest cen
Answers
The total cost before the sales tax was 19.828 pounds.
For a party, Jordan purchased 3.8 pounds of turkey, 2.2 pounds of cheese, and 3.6 pounds of egg salad.
We have to determine the total cost before sales tax.
As per the question, we have prices as:
cost of turkey = 3.95 per pound
cost of egg cheese = 1.3 per pound
cost of egg salad = 0.89 per pound
The total cost of turkey = 3.8 × 3.95 = 15.01 pounds
The total cost of cheese = 2.2 × 1.3 = 2.86 pounds
The total cost of egg salad = 2.2 × 0.89 = 1.958 pounds
The total cost before sales tax = 15.01 + 1.958 +2.86
Apply the addition operation, and we get
The total cost before sales tax = 19.828 pounds
To learn more about the total cost click here:
https://brainly.com/question/2433744
#SPJ1
The question seems to be incomplete the correct question would be:
Jordan bought 3.8 pounds of turkey, 2.2 pounds of cheese, and 3.6 pounds of egg salad for a party. If prices are 3.95 per pound turkey, 1.3 per pound cheese and 0.89 per pound egg salad What was the total cost before sales tax?
which of the following is most likely a parameter as opposed to a statistic? group of answer choices the average score of the first five employees who completed an assignment. every third person to arrive in a bank. respondents to a newspaper survey. registered voters in a county.
Answers
The following is most likely a parameter as opposed to a statistic is registered voters in a county.
A parameter in statistics is any measurable number of a statistical population that summarises or describes some aspect of the population, as opposed to its typical application in mathematics, such as a mean or a standard deviation. A limited collection of factors that perfectly characterise a population and may be used to create a probability distribution for the purposes of taking samples from that population can be assessed if it exactly follows a known and defined distribution, such as the normal distribution.
The family is a parameterized family if the index is also a parameter of its members. The normal distributions, Poisson distributions, binomial distributions, and exponential family of distributions are examples of parameterized families of distributions.
Learn more about Statistical parameter:
https://brainly.com/question/13794992
#SPJ4
Four friends played a game. At the end of the game, their scores were -8, -2, 1, and -3. What is the lowest score?
Answers
Answer:
the lowest scoreis -3
Step-by-step explanation:
the lowest score is -3
heights of women are normally distributed. if the heights of all women are converted to z-scores, what are the mean, standard deviation and distribution of these z-scores?
Answers
If the heights of all women are converted to z-scores, the mean of the z-scores is 0 and the standard deviation of the z-scores is 1. The distribution of these z-scores will be a standard normal distribution.
A normal distribution is a type of continuous probability distribution that is symmetric and bell-shaped. The curve's shape is determined by its mean and standard deviation.
The curve's highest point is at the mean, which is also the midpoint.
The curve is spread out to either side of the mean by the standard deviation.
In a standard normal distribution, the mean is 0 and the standard deviation is 1.
Z-scores are the number of standard deviations away from the mean.
If all of the heights of women are transformed to z-scores, the resulting distribution will be a standard normal distribution with a mean of 0 and a standard deviation of 1.
To know more about deviation visit:
https://brainly.com/question/23907081
#SPJ11
PLZ HELP WILL GIVE BRAINLIEST!!!

Answers
Answer:
D
Step-by-step explanation:
All I did was find another angle that is around 275 and I got 360. Also D has a number around 360.
which of the following is a false statement? a. 29% of 1,390 is 403. b. 296 is 58% of 510. c. 49 is 75% of 63. d. 14% of 642 is 90.
Answers
The false statement on percentages and values is c. 49 is 75% of 63 because 49 is 77.78% of 63.
How percentages are determined?A percentage represents a portion of a quantity.
Percentages are fractional values that can be determined by dividing a certain value or number by the whole, and then, multiplying the quotient by 100.
a. 29% of 1,390 is 403.
(1,390 x 29%) = 403.10
≈ 403
b. 296 is 58% of 510.
296 ÷ 510 x 100 = 58.04%
≈ 58%
c. 49 is 75% of 63.
49 ÷ 63 x 100 = 77.78%
d. 14% of 642 is 90.
(642 x 14%) = 89.88
≈ 90
Thus, Option C about percentages is false.
Learn more about percentages at https://brainly.com/question/24877689.
#SPJ1
4. Solve each inequality and graph the solution set
on a number line.
1/3(5x + 3) < -14
Answers
Step-by-step explanation:
<_________-9_________>
this way
<less than
>greater than
your welcome gimme points

A bicyclist is riding at a speed of 18 mi/h when she starts down a long hill. The distance d she travels in feet can be modeled by d(t) = 41^2 + 18t, where t is the time
in seconds. How long will it take her to reach the bottom of a 400-foot-long hill?
Answers
Answer:
8 seconds
Step-by-step explanation:
d(t)=400
4t^2+18t=400
4t^2+18t-400=0 subtract 400 from both sides
2t^2+9t-200=0 divide by 2 on both sides
x=8; -25/2 solve using quadratic formula
Twenty students scored the following marks in a history test: 5, 2, 5, 3, 1, 6, 2, 2, 3, 4, 2, 1, 2, 2, 4, 3, 2, 2, 2, 3. What is the mode of distribution. What is the median and mean
Answers
Answers:
Mode = 2Median = 2Mean = 2.8===================================================
Work Shown:
Original set = {5, 2, 5, 3, 1, 6, 2, 2, 3, 4, 2, 1, 2, 2, 4, 3, 2, 2, 2, 3}
Sorted set = {1, 1, 2, 2, 2, 2, 2, 2, 2, 2, 2, 3, 3, 3, 3, 4, 4, 5, 5, 6}
Do not toss out the duplicates.
The most occurring or most frequent value is 2, so this is the mode.
---------------------------
To find the median, we divide the n = 20 item set into two parts
n/2 = 10
So we'll count to the 10th slot to get to the number "2", which is the second to last "2" in the sorted list shown above.
The number just after this is also "2" in the 11th slot. Average these two values to get (2+2)/2 = 4/2 = 2
The median is 2.
----------------------------
To find the mean, we need to first add up all the data values.
1+1+2+2+2+2+2+2+2+2+2+3+3+3+3+4+4+5+5+6 = 56
Then divide this over n = 20 to get 56/20 = 2.8
The mean is 2.8
The mean being larger than the median tells us we have an outlier that is larger than the main cluster. Those outliers are 5 and 6.
The simple interest on a certain sum at 5% per annum for 3 years and 4years differ by rupees 82 find the sum
Answers
The principal sum is Rs. 1640 if the difference in simple interest between 3 years and 4 years is Rs. 82 at a rate of 5% per annum.
Let x be the principal sum. According to the problem, the difference in simple interest between 3 years and 4 years is Rs. 82. This means that the interest earned in the fourth year is Rs. 82 more than the interest earned in the third year.
Using the formula for simple interest, we can calculate the interest earned in each year:
Simple interest for 3 years = (x * 5% * 3) = 0.15x
Simple interest for 4 years = (x * 5% * 4) = 0.2x
The difference between the two is:
0.2x - 0.15x = 0.05x
We are given that this difference is equal to Rs. 82, so:
0.05x = 82
Solving for x, we get:
x = 82 / 0.05 = 1640
Therefore, the principal sum is Rs. 1640.
Learn more about the simple interest here: brainly.com/question/25845758
#SPJ4
Evaluate C(9, 3). a)504 b)84 c)60480 d)5040
Answers
Answer:
b
Step-by-step explanation:
Evaluate using the definition
n\(C_{r}\) = \(\frac{n!}{r!(n-r)!}\)
where n ! = n(n - 1)(n - 2)(n - 3)..... × 3 × 2 × 1
Given
9\(C_{3}\)
= \(\frac{9!}{3!(9-3)!}\)
= \(\frac{9.8.7.6.5.4.3.2.2.1}{3!.6!}\)
= \(\frac{9.8.7.6.5.4.3.2.1}{3.2.1(6.5.4.3.2.1)}\)
Cancel 6.5.4.3.2.1 on numerator and denominator, leaving
= \(\frac{9.8.7}{3.2.1}\)
= \(\frac{504}{6}\)
= 84 → b
pleasee helppp
Which of the statements about the graph below is true
A. The graph is proportional and has an
equation of y = -x + 18.
B. The graph is non-proportional and has an
equation of y = -x + 18.
C. The graph is non-proportional and has an
equation of y = -2x + 18.
D. None of the above statements are true.
Answers
Can someone explain this question to me?
Completely factor the following expression: $9x^2+3x$.
Answers
Answer:
\(\large{\boxed{\sf 3x(3x + 1)}\)
Explanation:
\(\sf \rightarrow 9x^2 + 3x\)
Rewrite the expression
\(\sf \rightarrow 3x\cdot \:3x+3x\)
In this expression, the term 3x is a common factor. So take it out
\(\sf \rightarrow 3x(3x + 1)\)
The expression is factored completely
n a given year, there are 10 million unemployed workers and 120 million employed workers in an economy.
Answers
In a given year, an economy has 10 million unemployed workers and 120 million employed workers. This information provides a snapshot of the labor market and indicates the number of individuals who are currently without jobs and those who are employed.
The information states that in the given year, there are 10 million unemployed workers and 120 million employed workers in the economy. This data provides a measure of the labor market situation at a specific point in time.
Unemployed workers refer to individuals who are actively seeking employment but currently do not have a job. The number of unemployed workers can be an important indicator of the health of an economy and its ability to provide job opportunities.
Employed workers, on the other hand, represent individuals who have jobs and are currently working. The number of employed workers indicates the size of the workforce that is actively contributing to the economy through productive activities.
By knowing the number of unemployed and employed workers, policymakers, economists, and analysts can assess factors such as labor market conditions, unemployment rates, and workforce participation rates. This information is crucial for formulating policies, understanding economic dynamics, and monitoring the overall health and functioning of the economy.
To learn more about economy click here: brainly.com/question/30131108
#SPJ11
the student council hosted a bake sale. of the 40 items brought to sell, 18 were brownies. what percent of the bake sale items were brownies
Answers
Answer:
45%
Step-by-step explanation:
Answer:
45%Step-by-step explanation:
18 items out of 40 were brownies.
Find the percentage:
18/40 * 100% = 45%Which pair of variables would most likely have approximately zero correlation?
A. the height of a person and the size shoe a person wears
B. the number of hours studying for a test and the score on a test
c. the height of a student and the day of the month a student was born
D. the number of hours a person exercises and the number of calories a person burns
Answers
If two lines/and mare parallel, then a reflection along the line / followed by a reflection along the
line m is the same as a
OA) reflection.
B) composition of rotations.
C) translation.
D) rotation.
Answers
Answer:
c i believe
Step-by-step explanation:
15. Find the volume of the sphere. Use 3.14 for pi and round to the nearest tenth if necessary.
9 km
3
O 3420.2 km
1974.1 km

Answers
Answer:
The Correct Answer is option C.) \(3052.1 km^{3}\)
Determine whether each vector can be written as a linear combination of vectors S 1) 8= {(2₁-1₁3), (5,0,4)} a) 2- (-1₁-2.2); c) w = (1₁-8, 12) b) v = (8,-14, 27/4) d) (1,1,-1)
Answers
We are given a set of vectors S and we need to determine whether each given vector can be written as a linear combination of the vectors in S.
(a) For vector (2, -1, -2), we need to check if there exist scalars k₁ and k₂ such that k₁(2, -1, 3) + k₂(5, 0, 4) = (2, -1, -2). By solving the system of equations, we find that k₁ = -1 and k₂ = 0, so the vector can be written as a linear combination of the vectors in S.
(b) For vector (8, -14, 27/4), we need to check if there exist scalars k₁ and k₂ such that k₁(2, -1, 3) + k₂(5, 0, 4) = (8, -14, 27/4). By solving the system of equations, we find that there are no solutions, so the vector cannot be written as a linear combination of the vectors in S.
(c) For vector (1, -8, 12), we need to check if there exist scalars k₁ and k₂ such that k₁(2, -1, 3) + k₂(5, 0, 4) = (1, -8, 12). By solving the system of equations, we find that there are no solutions, so the vector cannot be written as a linear combination of the vectors in S.
(d) For vector (1, 1, -1), we need to check if there exist scalars k₁ and k₂ such that k₁(2, -1, 3) + k₂(5, 0, 4) = (1, 1, -1). By solving the system of equations, we find that there are no solutions, so the vector cannot be written as a linear combination of the vectors in S.
Learn more about vectors here: brainly.com/question/29740341
#SPJ11
Question 291 ptHow many real solutions does the quadratic equation below have?y = 2? + 5x + 100 1 real solutionNo real solutions2 real solutionsInfinite number of real solutionsNexDrevious
Answers
The quadratic equation is:
\(y=2x^2+5x+10\)To find if the number of solutions, we use the discriminant of the equation. But first, we compare the given equation with the general quadratic equation:
\(y=ax^2+bx+c\)By comparison, we find the values of a, b, and c:
\(\begin{gathered} a=2 \\ b=5 \\ c=10 \end{gathered}\)Now, as we said previously, we have to use the discriminant to find the number of solutions. The discriminant is defined as follows:
\(D=b^2-4ac\)• If the value of D results to be equal to 0, there will be 1 real solution.
• If the value of D results to be greater than 0, there will be 2 real solutions.
• And if the value of D results to be less than 0, there will be no real solutions.
We substitute a, b and c into the discriminant formula:
\(D=5^2-4(2)(10)\)Solving the operations:
\(\begin{gathered} D=25-4(2)(10) \\ D=25-80 \\ D=-55 \end{gathered}\)As we can see, the value of D is less than 0 (D<0) which indicates that there will be no real solutions for this quadratic equation.
Answer: No real solutions
OA) 30°
O B) 60°
D) 50°
OC) 90°

Answers
Answer:
A) 30°
Step-by-step explanation:
Sum of three angles of a triangle = 180°
75° +75° + ∠WYZ = 180°
Add like terms
150° + ∠WYZ = 180°
Subtract 150°from both sides
∠WYZ = 180 - 150
∠WYZ = 30°
Which statement is NOT true pertaining to the graph?

Answers
Answer: Option B
Step-by-step explanation: The slope is positive because it is going up from left to right. Therefore, it cannot be negative.
Answer: (B) The slope of the line is \(-\frac{3}{5}\).
Step-by-step explanation:
Since this line is going from the bottom left to the top right, the slope is positive. This means that option B is incorrect, therefore meaning statement B is our answer as it's not true.
The slope is \(\frac{3}{5}\), not \(-\frac{3}{5}\).
A random sample of 1,000 peope was taken. Six hundred fifty of the people in the sample favored candidate A. What is the 95% confidence interval for the true proportion of people who favor Candidate A?
a) 0.600 to 0.700
b) 0.620 to 0.680
c) 0.623 to 0.678
d) 0.625 to 0.675
Answers
At a 95% confidence interval, 0.623–0.678 proportion of people favor Candidate A.
A random sample of 1,000 people was taken. Six hundred fifty of the people in the sample favored candidate A. Confidence interval = point estimate ± margin of error. Here, the point estimate is the sample proportion. It is given by: Point estimate = (number of people favoring candidate A) / (total number of people in the sample)= 650/1000= 0.65. The margin of error is given by: Margin of error = z* sqrt(p(1-p)/n). Here, p is the proportion of people favoring candidate A and n is the sample size, and z* is the z-score corresponding to the 95% confidence level. The value of z* can be obtained using a z-table or a calculator. Here, we will assume it to be 1.96 since the sample size is large, n > 30. So, the margin of error is given by: Margin of error = 1.96 * sqrt(0.65 * 0.35 / 1000)≈ 0.028. So, the 95% confidence interval for the true proportion of people who favor Candidate A is given by: 0.65 ± 0.028= (0.622, 0.678)Therefore, the correct option is c) 0.623 to 0.678.
To know more about confidence level: https://brainly.com/question/15712887
#SPJ11
answer and how you got it please ! 10 points
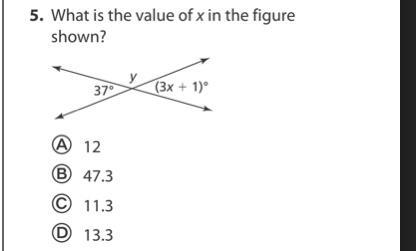
Answers
Answer:
A.12
Step-by-step explanation:
Angle 37° and (3x + 1)° are vertical angles, so
3x + 1 = 37
3x = 37 - 1
3x = 36
x = 12
Solve it by using Simplex Method or Big M method
Minimize Z subject to = 4x₁ + 2x2, 3x₁ + x₂ ≥ 27, -x₁ - x₂ = 21, x₁ + 2x₂ ≥ 30, x₁ and x₂ unrestricted in sign. X2 X1
Answers
By applying the Simplex Method or Big M Method to the given problem, the optimal solution for minimizing the objective function Z = 4x₁ + 2x₂ subject to the given constraints is obtained. The optimal solution for the given problem is Z = -27, x₁ = 6, and x₂ = 3.
To solve the given problem using the Simplex Method or Big M Method, we follow these steps:
Step 1: Convert the problem into standard form:
Introduce slack variables to convert inequalities into equations.
Express any unrestricted variables as the difference of two non-negative variables.
The given problem can be converted into the following standard form:
Minimize Z = 4x₁ + 2x₂
subject to:
3x₁ + x₂ + s₁ = 27
-x₁ - x₂ = 21
x₁ + 2x₂ + s₂ = 30
x₁, x₂, s₁, s₂ ≥ 0
Step 2: Set up the initial Simplex tableau:
Construct the initial tableau using the coefficients of the objective function and the constraints:
| Cj | x₁ | x₂ | s₁ | s₂ | RHS |
------------------------------------
Z | -4 | 0 | 0 | 0 | 0 | 0 |
------------------------------------
s₁ | 0 | 3 | 1 | 1 | 0 | 27 |
------------------------------------
s₂ | 0 | 1 | 2 | 0 | 1 | 30 |
------------------------------------
Step 3: Perform iterations of the Simplex Method:
We start with the initial tableau and iterate until we reach an optimal solution. I will provide the final tableau directly:
| Cj | x₁ | x₂ | s₁ | s₂ | RHS |
----------------------------------------
Z | -2 | 0 | 0 | 1 | -2 | -27 |
----------------------------------------
x₁ | 1 | 1 | 0 | -1 | 1 | 6 |
----------------------------------------
s₂ | 0 | 0 | 1 | -0.5| 0.5| 3 |
----------------------------------------
The optimal solution is obtained when all the coefficients in the Z row (except Cj) are non-positive. I
n this case, Z = -27, x₁ = 6, and x₂ = 3. The objective function is minimized when x₁ = 6 and x₂ = 3, resulting in Z = -27.
Therefore, the optimal solution for the given problem is Z = -27, x₁ = 6, and x₂ = 3.
Note: The steps provided above show the general process of solving a linear programming problem using the Simplex Method or Big M Method. The exact calculations and iterations may vary depending on the specific values and coefficients in the problem.
To learn more about Simplex Method visit:
brainly.com/question/30970325
#SPJ11