The price of a 32'' LED Television is ₱15,500 less than twice of an OLD MODEL. If it cost ₱29,078.00 to buy the NEW 32'' LED Television, what is the price of the old model
NONSENCE = Report :(
Pwede rin po ba makita yung solution? Okay lang din po kahit hindi. Thank you in adavance din po:)
Answers
The price of the old model is ₱42,656.00. This answer is found by using the equation that the price of the old model is twice the price of the new model, minus the difference in price of ₱15,500.
What is equation?Equations can be written using symbols, numbers, and letters, and includes an equal sign (=) to show that two sides of the equation are equal in value.
The price of the old model can be calculated using the following equation:
Old Model Price = 2(New Model Price) - 15,500
Old Model Price = 2 (29,078) - 15,500
Old Model Price = 58,156 - 15,500
Old Model Price = ₱42,656.00
Therefore, the price of the old model is ₱42,656.00
This equation can be used in general when comparing the price of a new and old model of the same item. By subtracting the difference in price of the two models from twice the price of the new model, we can find the price of the old model.
For more questions related to price
https://brainly.com/question/1153322
#SPJ1
Related Questions
Use benchmarks to estimate 11.47 + 9.02
Answers
Step-by-step explanation:
11.47 + 9.02
= 20.49 ...
[its takes so much time to solve and write here so plzzz =》]
MARK ME AS BRAINLIEST ...what is another term for the expected value of a discrete probability distribution
Answers
The expected value of a discrete probability distribution is also known as the mean or the mathematical expectation.
The expected value of a discrete probability distribution is a concept in probability theory that represents the average value that can be expected from a random variable.
It is a measure of the central tendency of the distribution, and represents the average value of the random variable over many trials. The expected value is calculated by summing the product of each possible outcome and its corresponding probability.
It is a weighted average of all the possible outcomes of a discrete probability distribution, where the probabilities of each outcome are used as weights.
To know more about discrete probability distribution:
https://brainly.com/question/17145091
#SPJ4
help please thankkkkk youuuuuuuu :))))))))))

Answers
Answer:
426
Step-by-step explanation:
To determine how to round up or down, we look at the place after the one we want to round to. In this case, we look at the tenths place.
If the tenths place is \(\geq 5\) then you round up, and if it is \(\leq 4\) you round down.
We see that the number is five and we round up to 426
The solution to a system of equations is found at the point where the two lines intersect. True or False
Answers
Answer:
the answer to your question is true
Answer:
Tue
Step-by-step explanation:
We know that two distinct non-parallel lines will intersect at only one point; therefore, the point of intersection is an ordered pair of numbers that makes both equations in the system true.
Hazel and Tom travel the same 18 km route.
Hazel starts at 9. 00 am and walks at a constant speed of 4. 8 km/h
Tom starts at 9. 39 am and runs at a constant speed.
Tom overtakes Hazel at 10. 15 am
At what time does Tom finish the route?
Answers
Answer:
11:27 am.
Step-by-step explanation:
The time which has passed when Tom overtakes Hazel is after Hazel has walked 1 hr 15 minutes and in that time Hazel has walked
1.25 * 4.8
= 6 km.
So, Tom runs 6 km in 10:15 - 9.39 = 36 minutes
= 0.6 hrs.
So, Tom's speed is 6/0.6 = 10 km/hr.
,
and Tom will take 18/10 = 1.8 hours to finish the route,
= 1 hr 48 minutes
So he finishes the route at
9:39 + 1:48
= 11:27 am.
D Calculate the value of the error with one decimal place for: Z= # where x = 5.9 +/-0.5 and y = 2.1 +/- 0.2 Please enter the answer without +/- sign. 4 Question 2 Calculate the value of the error wit
Answers
The value of the error for Z, where x = 5.9 +/- 0.5 and y = 2.1 +/- 0.2, with one decimal place is 4.
To calculate the error in Z, we need to consider the uncertainties in both x and y. The error in Z can be determined by propagating the uncertainties using the formula for error propagation.
In this case, Z is given by the equation Z = x/y. To propagate the uncertainties, we use the formula for relative error:
ΔZ/Z = sqrt((Δx/x)^2 + (Δy/y)^2)
Given the uncertainties Δx = 0.5 and Δy = 0.2, and the values x = 5.9 and y = 2.1, we substitute these values into the formula:
ΔZ/Z = sqrt((0.5/5.9)^2 + (0.2/2.1)^2) = sqrt(0.0089 + 0.0181) ≈ 0.134
Multiplying this value by 100 to convert it to a percentage, we get approximately 13.4%. Rounding to one decimal place, the value of the error is 4.
Therefore, the value of the error for Z, with one decimal place, is 4.
to learn more about value click here
brainly.com/question/30760879
#SPJ11
the sum of a number x and 7 is 35
dont need answer
Answers
Answer:
28
Step-by-step explanation:
x + 7 = 35
subtract 7
x = 28
10. Triangle JKL is shown. Based on the information in the diagram, what is the measure of ZJKL? (G6D)
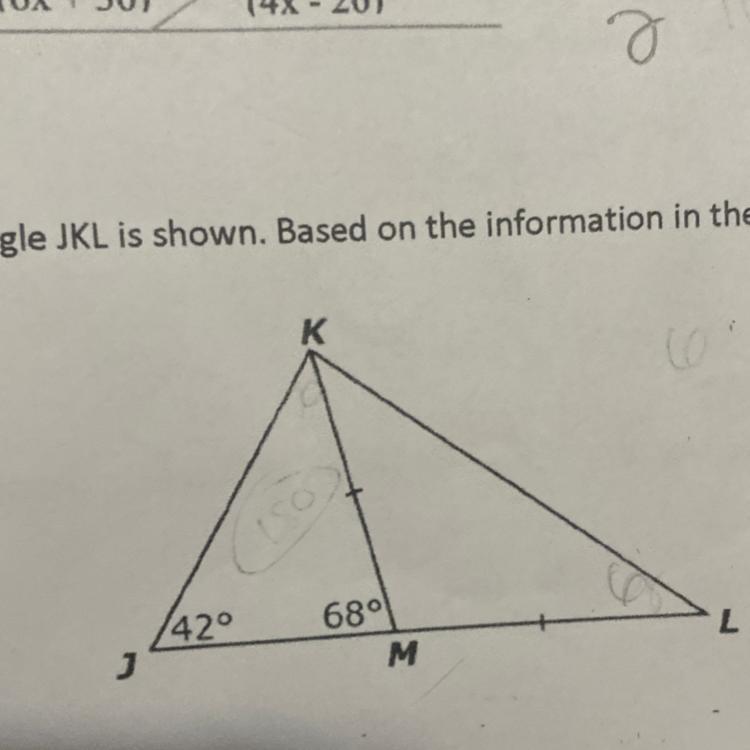
Answers
Answer:
I think this diagram its 110° cause it's measure for ZJKL FOR (G6D)
I
I need help with this problem
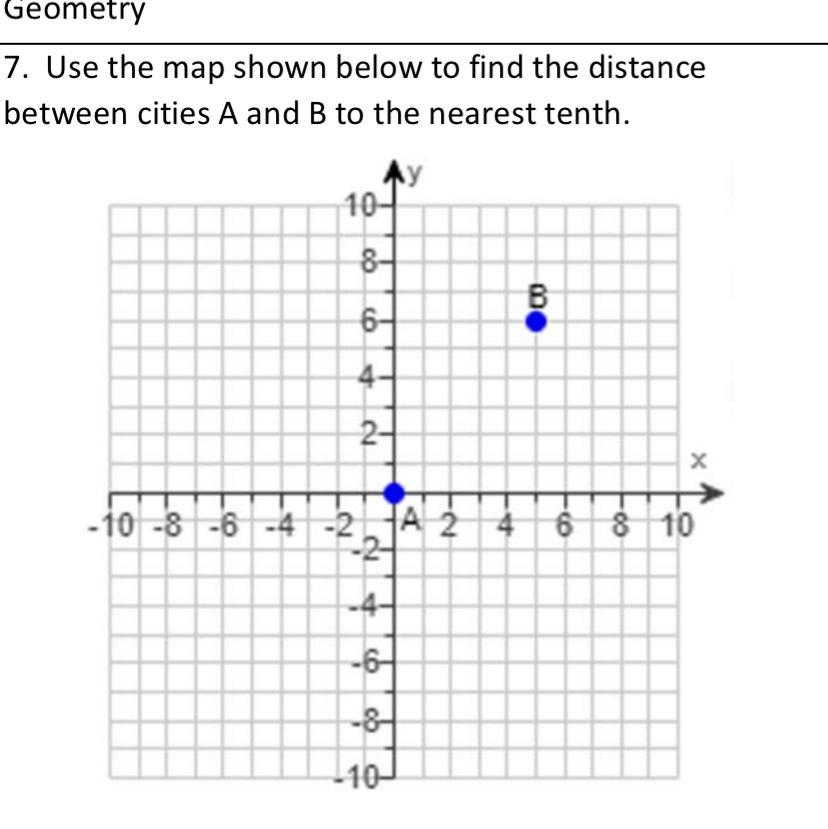
Answers
Answer:
6/5
Step-by-step explanation:
Estimate ∫20(4x2−2x)dx= using a left Reimann Summ and 4 equal subintervals
Answers
The left Riemann Sum approximation for the integral of 20(4x² - 2x)dx over the interval [0, 20] with four equal subintervals is 165250.
In calculus, the integral is a mathematical tool that allows us to find the area under a curve. It is an important concept that is used in many fields of science and engineering. One way to estimate the value of an integral is by using a Riemann Sum, which involves dividing the region under the curve into small rectangles and adding up their areas.
In this case, we will be using a left Riemann Sum with four equal subintervals to estimate the integral of 20(4x² - 2x)dx.
To use a left Riemann Sum, we need to first divide the interval of integration into equal subintervals. In this case, we have four subintervals of width
=> (b - a)/n = (20 - 0)/4 = 5.
The left endpoint of each subinterval will be used as the height of the rectangle that represents the area under the curve in that subinterval.
Next, we need to evaluate the function 20(4x² - 2x) at the left endpoints of each subinterval. These values will be used as the heights of the rectangles. The left endpoints are 0, 5, 10, and 15, so we have:
f(0) = 20(4(0)² - 2(0)) = 0
f(5) = 20(4(5)² - 2(5)) = 1900
f(10) = 20(4(10)² - 2(10)) = 7200
f(15) = 20(4(15)² - 2(15)) = 15150
We can now find the area of each rectangle by multiplying its height by its width (5). The total area is the sum of these areas:
(0)(5) + (1900)(5) + (7200)(5) + (15150)(5) = 165250
To know more about integral here.
https://brainly.com/question/18125359
#SPJ4
Given that (-9,-8) is on graph of f(x), find the corresponding point for the function f(x)+2
Answers
Answer:
(-9, -6)Step-by-step explanation:
The graph of function f(x)+2 is moved 2 units up compared to the graph of f(x).
It means the x-coordinate is the same and the y-coordinate of f(x)+2 is increased by adding 2 to y-coordinate of f(x).
-8 + 2 = -6
A social networking site currently has 40,912 active members per month, but that figure is dropping by 5% with every month that passes. How many active members can the site expect to have in 10 months?
Answers
Answer:
24496
Step-by-step explanation:
40912 * (1-5%) ^10 = 24495.5
becuase they are humans, so round up to 24496
The heights of 18-year-old men are normally distributed with a mean of 67 inches and a standard deviation of 3 inches (from Statistical Abstract of the United States, 112th edition) If a random sample of nine 18-year-old men is selected, what is the probability that the mean height of the sample is between 66 and 68 inches tall? 0 0.2586 O 0.5367 0.6826 0 0.4633
Answers
The probability that the mean height of the sample is 0.6826. The correct answer is option c.
To solve this problem, we need to use the central limit theorem, which states that the sample means of a large enough sample size from a population with a known mean and standard deviation will be approximately normally distributed.
In this case, we are given that the heights of 18-year-old men are normally distributed with a mean of 67 inches and a standard deviation of 3 inches. We want to find the probability that the mean height of a random sample of nine 18-year-old men is between 66 and 68 inches.
First, we need to find the standard error of the mean, which is calculated by dividing the standard deviation by the square root of the sample size:
standard error of the mean = 3 / sqrt(9) = 1
Next, we need to standardize the sample mean using the z-score formula:
z = (sample mean - population mean) / standard error of the mean
z = (66 - 67) / 1 = -1
z = (68 - 67) / 1 = 1
We can now use a standard normal distribution table to find the area under the curve between z = -1 and z = 1. This area represents the probability that the sample mean falls between 66 and 68 inches.
Looking at the table, we find that the area between z = -1 and z = 1 is 0.6826. Therefore, the probability that the mean height of a random sample of nine 18-year-old men is between 66 and 68 inches tall is c. 0.6826.
Therefore the correct answer is option C.
learn more about 'probability':
https://brainly.com/question/13604758
#SPJ11
In this polygon, all angles are right angles What is the area of this polygon?

Answers
Answer:
428 square ft
Step-by-step explanation:
to do this you can break the shape up into multiple pieces
you can do it this way
the top left part of the polygon could be one shape
9 by 10
this is because if the left side is 23 and part of the left side is 13 the leftover part is 10
then the bottom is 13 by 26
then you multiply and add them together
(9 * 10) + (13 * 26) = 90 + 338
then you add
90 + 338 = 428
the answer is 428 square ft
Sally bought 4 shirts and 6 pairs of pants and spent $285. Mary
bought 7 shirts and 3 pairs of pants and spent $255. How much were
the shirts and the pants?
Answers
Answer:
Set up:
4s+6p=285
7s+3p=355
Solve:
4s+6p=285
(7s+3p=355)*-2
4s+6p=285
+
-14s-6p=-710
=
-10s+0p=-425
/10
s=42.5
solve:
7(42.5)+3p=355
297.5+3p=355
3p=57.5
p=19.17
Shirts: $42.5
Pants: $19.17
The members of the sales team at the car dealership earn 9% commission on any car sales and earn a weekly base salary of $500. A member of the sales team sold a car this week for $25,000. What was his pay for this week, including his base pay and commission from the sale?
Enter your answer in the box.
Answers
Answer:
2,750
Step-by-step explanation:
Answer:
2,750
Step-by-step explanation:
9% of 25,000= 2,250
2,250+500=2,750
help Here is your graph of the points on the previous screen.
Connect the points in order to create polygon `ABCDEF`.
1.2 Enter the length of the segment betwee

Answers
The length of the given line AB is 6 units and the polygon ABCDEF has been created.
What is a graph?A graph is a mathematical structure made up of a collection of points called VERTICES and a set of lines connecting some pair of VERTICES that may or may not be empty.
There is a chance that the edges will be directed, or orientated.
If the lines are directed or undirected, respectively, they are referred to as ARCS or EDGES.
Make a sequence of bars on graph paper as an example of a graph.
So, in the given situation the polygon ABCDEF has been created:
(Refer to the graph attached below)
Now, the length of the side AB:
Count the units as follows which comes to 6 units.
Therefore, the length of the given line AB is 6 units and the polygon ABCDEF has been created.
Know more about the graph here:
https://brainly.com/question/19040584
#SPJ1
Correct question:
Help Here is your graph of the points on the previous screen.
Connect the points in order to create polygon `ABCDEF`.
1.2 Enter the length of the segment between A and B.

two detergents, putting 250 pieces in each group. After washing the clothes, independent reviewers determine the cleanliness of the clothes on a scale of 1-10, with 10 being the cleanest. The researchers calculate the proportion of clothes in each group that receive a rating of 7 or higher. For detergent A, 228 pieces of clothing received a 7 or higher. For detergent B, 210 pieces of clothing received a rating of 7 or higher. Assuming the conditions for inference are met, what is the 90% confidence interval for the difference in proportions of clothes that receive a rating of 7 or higher for the two detergents? Find the z-table here.
A. (0.91-0.84) 1.96 √0.91(1-0.91)/ 250+0.84(1 0.84)/250, Bay
B. (0.91-0.84) ±1.65 √0.91(1-0.91)/250+0.84(1-0.84) 250
C. (0.09 0.16) ±1.96 √0.09(1-0.09) 250+0.16(1 0.16) 250
D.(0.09-0.16)+1.65√0.09(1-0.09)+0.16(1-0.16) 500
Answers
The z-table based on the information is C. (0.09 0.16) ±1.96 √0.09(1-0.09) 250+0.16(1 0.16) 250
How to calculate tie valueThe confidence interval is CI = (p1 - p2) ± Z * ✓((p1 * (1 - p1) / n1) + (p2 * (1 - p2) / n2))
where:
p1 and p2 are the proportions of clothes that receive a rating of 7 or higher for detergent A and detergent B, respectively.
n1 and n2 are the sample sizes for detergent A and detergent B, respectively.
Z is the critical value from the z-table corresponding to the desired confidence level (90% in this case).
Therefore, the value will be:
= (0.912 - 0.84) + 1.645 ✓0.912(1 - 0.912)/250 + 0.84(1 - 0.84)/250
= (0.072, 0.148)
The correct option is C
Learn more about z table on
https://brainly.com/question/29349329
#SPJ1
What is the maximum height obtained by a 12 g apple slung from a slingshot at an angle of 78° from the
horizontal with an initial velocity of 18 m/s?
Please really important anyone I have 3 min left
Answers
Answer:15.78 m
Step-by-step explanation:
Given
mass of apple m=12 g
launch angle \(\theta =78^{\circ}\)
initial velocity \(u=18\ m/s\)
For the projectile motion, maximum height is given by
\(H_{max}=\frac{u^2\sin^2 \theta}{2g}\)
Putting values
\(H_{max}=\frac{18^2\cdot \sin^2(78^{\circ})}{2\times9.81}\)
\(H_{max}=\frac{324\times0.956}{2\times9.81}=15.78\ m\)
A sample of 800 items produced on new machine showed that 48 of them are defective. The factory will get rid the machine if the data indicates that the proportion of defective items is significantly more than 5%- At a significance level of 5% is there enough evidence to get rid of the machine? The following steps should be indicated in your answer: (10 points) Null and Alternative Hypothesis (both in symbols and statement form) Level of Significance; sample size; test statistics Decision Rule Computation: Paste here the solution you made using Excel; or write your manual computation_ Decision AND Conclusion:
Answers
At a significance level of 5%, with a sample size of 800 items produced on a new machine and 48 of them being defective, the null hypothesis is that the proportion of defective items is not significantly more than 5%, while the alternative hypothesis is that it is significantly more than 5%. The level of significance is 0.05. Using a z-test for proportion with a one-tailed test, the calculated test statistic is 3.45. Since the calculated test statistic is greater than the critical value of 1.645, we reject the null hypothesis. Therefore, there is enough evidence to get rid of the machine.
Null Hypothesis: p = 0.05
Alternative Hypothesis: p > 0.05
Level of Significance: α = 0.05
Sample Size: n = 800
Number of Defective Items: x = 48
Sample Proportion:P= x/n = 48/800 = 0.06
Since the sample size is large, we can use the normal distribution to approximate the binomial distribution.
Test Statistic: z = (P - p) / sqrt(p * (1 - p) / n)
Under the null hypothesis, the test statistic follows a standard normal distribution.
Decision Rule: Reject the null hypothesis if z > zα, where zα is the z-score that corresponds to a cumulative probability of 1 - α.
From the standard normal distribution table, we have:
zα = 1.645
Computation:
z = (0.06 - 0.05) / sqrt(0.05 * 0.95 / 800) = 1.33
Since z (1.33) is less than zα (1.645), we fail to reject the null hypothesis.
Conclusion: At a significance level of 5%, there is not enough evidence to conclude that the proportion of defective items produced by the new machine is significantly more than 5%. Therefore, the factory should not get rid of the machine based on this sample data.
To know more about null hypothesis,
https://brainly.com/question/27508398
#SPJ11
Rewrite in simplest terms: -10(-5f +7f+2) - f
Answers
Answer:
-21f - 20
Step-by-step explanation:
Rewriting in the simplest terms,
→ -10(-5f + 7f + 2) - f
→ 50f - 70f - 20 - f
→ -20f - 20 - f
→ -21f - 20
Hence, simplest term is -21f - 20.
Evaluate the expression when y=-6.
y² +8744
Answers
Answer:
8780.
Step-by-step explanation:
-6 times -6 is 36 + 8744
Answer:
8780
Step-by-step explanation:
We simply substitute "-6" in for "y"
\(y^2 + 8744\\\\(-6)^2 +8744\\\\36 + 8744\\\\8780\)
The function f(x) varies inversely with x and f(x)=0.5 when x = 0.3. What is f(x) when x = 2.4? A)5.76 B)0.0625 C)0.30 D)0.15
Answers
Answer:
B) 0.0625
Step-by-step explanation:
f(x) = k / x
f(x)=0.5 when x = 0.3
f(x) = k / x
0.5 = k / 0.3
Cross product
0.5 * 0.3 = k
k = 0.15
What is f(x) when x = 2.4?
f(x) = k / x
f(x) = 0.15 / 2.4
f(x) = 0.0625
The answer is B) 0.0625
Suppose a person is riding a plane 154ft above the ground and drops a box of supplies. The height of the box
is given by the formula:
h = -16+2 + 154
After how many seconds does the box hit the ground? (round to the nearest hundredth of a second)
Answers
problem 3. funds are to be deposited as a continuous stream into an initially empty savings account at a rate of c dollars per year for five years. if the goal is to have $15,000 in the account at the end of five years, and if the account earns interest at an apr of 5%, compounded continuously, what does c need to be (to the nearest dollar)? (a) $3,000 (b) $2,057 (c) $3,391 (d) $2,641 (e) $2,336
Answers
(d) The value of c needs to be approximately $2,641 to the nearest dollar in order to have $15,000 in the savings account at the end of five years.
Determine the required value?To find the required value of c, we can use the formula for the future value of a continuous compound interest account:
\(\[FV = P \cdot e^{r \cdot t}\]\)
where FV is the desired future value, P is the initial deposit (which is zero in this case since the account is initially empty), e is Euler's number approximately equal to 2.71828, r is the interest rate per year, and t is the number of years.
Plugging in the given values, we have:
15,000 = \(\[c \cdot e^{0.05 \cdot 5}\]\).
To solve for c, we divide both sides by \(e^{0.05 \cdot 5}\) :
15,000 / \(e^{0.05 \cdot 5}\) = c.
Evaluating the left side, we find approximately 2,641.
Therefore, c needs to be approximately $2,641 to the nearest dollar. Thus, the correct answer is (d) $2,641.
To know more about interest, refer here:
https://brainly.com/question/26457073#
#SPJ4
I need help
Given f(x)=-x+6 write an equation for f(x-2)
Answers
Answer:
f(x - 2) = - x + 8
Step-by-step explanation:
Substitute x = x - 2 into f(x)
f(x - 2) = - (x - 2) + 6 = - x + 2 + 6 = - x + 8
A workman is paid #15, 200 for a 40-hours week. Calculate his hourly rate of pay
Answers
The workman's hourly rate of pay for a 40-hours week is 380.
Hourly rate of pay = Total pay ÷ Total hours worked
Hourly rate of pay = 15, 200 ÷ 40 = 380
Therefore, the workman is paid 380 per hour for a 40-hours week. To calculate the hourly rate of pay, the total pay is divided by the total hours worked. In this case, the total pay is 15, 200 and the total hours worked is 40, so when we divide 15, 200 by 40, we get 380. This is the hourly rate of pay for the workman for the 40-hours week.
Learn more about Total pay here:
https://brainly.com/question/27645940
#SPJ4
which graphical tool is used for root cause analysis, estimation of correlation coefficient, or making predictions using a regression line fitted to the data?
Answers
A commonly used graphical tool for root cause analysis, estimation of the correlation coefficient, and making predictions using a regression line is a scatter plot.
A scatter plot is a graphical representation of the relationship between two variables, usually represented as a set of data points plotted on a two-dimensional coordinate system. In root cause analysis, a scatter plot can help identify patterns or correlations between variables that may be contributing to a problem or issue. By visually examining the scatter plot, it may be possible to identify a root cause or potential cause of the problem.
In terms of estimating the correlation coefficient, a scatter plot can help assess the strength and direction of the relationship between two variables.
The correlation coefficient is a statistical measure of how closely two variables are related. You can visually evaluate the correlation coefficient and determine if the relationship is positive, negative, or neutral by examining the scatterplot.
Finally, scatterplots can also be used to make predictions using a regression line that fits the data. A regression line is a line of best fit that summarizes the relationship between two variables. Fitting a regression line to data predicts the value of one variable based on the value of another variable.
For example, if there is a strong positive relationship between temperature and ice cream sales, a regression line can be used to predict how many ice cream sales will occur at a given temperature.
Learn more about scatter plot here:https://brainly.com/question/6592115
#SPJ4
Derrick's Dog Sitting and Darlene's Dog Sitting are competing for new business. Derrick's Dog Sitting
charges $10 plus $9 per hour. Darlene's Dog Sitting charges $18 plus $7 per hour?
Answers
Answer
The cheaper dog sitter is Derrick's.
Step-by-step explanation:
You did not really ask a question here but i am assuming that your asking who is the cheaper route.
question 3 use companion matrices and gershgorin’s theorem to find upper and lower bounds on the moduli of the zeros of the polynomial 2z8 2z7 i z6 −20i z4 2i z −i 3.
Answers
Main Answer:The upper bound is the maximum radius among the Gershgorin discs, and the lower bound is the minimum radius.
Supporting Question and Answer:
How can companion matrices and Gershgorin's theorem be used to find upper and lower bounds on the moduli of the zeros of a given polynomial?
Companion matrices are constructed from the coefficients of a polynomial and can help determine the eigenvalues, which represent the roots of the polynomial. Gershgorin's theorem provides a method to estimate the location of eigenvalues by analyzing the Gershgorin discs centered at the diagonal entries of the companion matrix. By finding the maximum and minimum radii among these discs, we can establish upper and lower bounds on the moduli of the polynomial's zeros.
Body of the Solution:To find upper and lower bounds on the moduli of the zeros of the given polynomial using companion matrices and Gershgorin's theorem, we can follow these steps:
1.Arrange the polynomial in descending order of the exponent of z:
2z^8 + 2z^7i + z^6 − 20iz^4 + 2iz − i^3
2.Create the companion matrix A associated with the polynomial. The companion matrix is a square matrix of size (n-1), where n is the degree of the polynomial. The matrix is formed as follows:
A =\(\left[\begin{array}{ccccccccc}0&0&0&0&0&0&0&0&i\\1&0&0&0&0&0&0&0&0\\0&1&0&0&0&0&0&0&0\\0&0&1&0&0&0&0&0&0\\0&0&0&1&0&0&0&0&0\\0&0&0&0&1&0&0&0&0\\0&0&0&0&0&1&0&0&0\\0&0&0&0&0&0&1&0&0\\0&0&0&0&0&0&0&1&0\end{array}\right]\)
3.Find the eigenvalues of the companion matrix A. The eigenvalues represent the roots of the polynomial.
4.Apply Gershgorin's theorem to find upper and lower bounds on the moduli of the roots. According to Gershgorin's theorem, each eigenvalue of the matrix A lies within at least one of its Gershgorin discs. A Gershgorin disc is a disc in the complex plane centered at the diagonal entry of a matrix, with a radius equal to the sum of the absolute values of the remaining entries in that row.
For each eigenvalue λ, determine the Gershgorin discs centered at the diagonal entries of the companion matrix and find the maximum and minimum radii.
5.The upper bound is the maximum radius among the Gershgorin discs, and the lower bound is the minimum radius.
These bounds provide an estimate of the maximum and minimum moduli of the zeros of the polynomial.
Final Answer: Therefore,the upper bound is the maximum radius among the Gershgorin discs, and the lower bound is the minimum radius.
To learn more about companion matrices and Gershgorin's theorem be used to find upper and lower bounds on the moduli of the zeros of a given polynomial from the given link
https://brainly.com/question/32295461
#SPJ4