The pounds of bananas sold each week at all Metro Seattle Alberstons stores as a function of price, p, in dollars/pound(lb.) is given by.
q(p) = 100e^(1.5(5-p))
1. What is the price elasticity of demand for bananas at $.20/lb. ?
(nearest 0.01)
2. What is the price elasticity of demand for bananas at $1/lb. ?
3. At what price is the maximum revenue per week achieved?
+/- $0.01
4. What is that maximum revenue per week?
5. How many pounds will be sold each week at that optimal price
Answers
140.55 pounds will be sold approximately each week at the optimal price of $0.67/lb.
How will you solve all the parts of this question?To find the price elasticity of demand for bananas at $0.20/lb, we need to use the formula:
According to the given data:
E(p) = -p(q'/q(p))
where q' is the derivative of q(p) with respect to p.
First, we need to find q'(p):
\(q'(p) = -225e^(1.5(5-p))\)
Then, we can plug in the values:
E(0.20) = -0.20(q'(0.20)/q(0.20))
= \(-0.20(-225e^(1.5(5-0.20)) / 100e^(1.5(5-0.20)))\)
= 2.70
Therefore, the price elasticity of demand for bananas at $0.20/lb is 2.70.
To find the price elasticity of demand for bananas at $1/lb, we can use the same formula:
E(p) = -p(q'/q(p))
First, we need to find q'(p):
\(q'(p) = -225e^(1.5(5-p))\)
Then, we can plug in the values:
E(1) = -1(q'(1)/q(1))
= \(-1(-225e^(1.5(5-1)) / 100e^(1.5(5-1)))\)
= 0.68
Therefore, the price elasticity of demand for bananas at $1/lb is 0.68.
To find the price that maximizes revenue, we need to find the value of p that makes revenue, R(p), maximum.
Revenue is given by:
R(p) = pq(p)
= \(p100e^(1.5(5-p))\)
To maximize revenue, we need to find the critical point of R(p) by taking its derivative and setting it equal to zero:
\(R'(p) = 100e^(1.5(5-p)) - 150pe^(1.5(5-p)) = 0\)
Simplifying this expression, we get:
\(2e^(1.5(5-p)) - 3pe^(1.5(5-p)) = 0\)
2 = 3p
p = 2/3
Therefore, the price that maximizes revenue is $0.67/lb.
To find the maximum revenue per week, we can plug this price back into the revenue equation:
\(R(2/3) = (2/3)*100e^(1.5(5-2/3))\)
= $167.56
Therefore, the maximum revenue per week is $167.56.
To find how many pounds will be sold each week at the optimal price of $0.67/lb, we can plug this price back into the demand equation:
\(q(2/3) = 100e^(1.5(5-2/3))\)
= 140.55
Therefore, approximately 140.55 pounds will be sold each week at the optimal price of $0.67/lb.
To know more about elasticity of demand visit:
brainly.com/question/28790459
#SPJ1
Related Questions
How many games are played in a 4 team round robin tournament? (Each team
plays every other team only once.)
Answers
Answer: 6
Step-by-step explanation:
If we call each team, A, B, C and D, each team has to play each other team once. Let's call each pairing between 2 teams the 2 teams' letters next to each other, e.g. AB is A playing against B. A has to play against B, C and D so we have AB, AC and AD. So we have 3 so far.
We have already counted that B is playing A but we haven't counted B playing C and D yet so we also have BC and BD. So we have 5 in total
Lastly, C needs to play D, we have already counted C playing B and C playing A so we have CD left. In total that gives 6.
Now we have already included D playing every other team so we don't include any other pairings.
In total, now every team has played every other team giving a total of 6.
(another way of solving this is doing "3!2 but if you haven't learnt factorials yet stick to the first method.
Answer:
6 games.
Step-by-step explanation:
The answer is the number of combinations of 2 from 4
= 4*3 / 2*1
= 6.
Which graph represents the function f(x) = 2^x-1 + 2?


Answers
The graph that represents the exponential function f(x) = 2^(x - 1) + 2 is given as follows:
Graph A.
How to define an exponential function?An exponential function has the definition presented as follows:
y = ab^x.
In which the parameters are given as follows:
a is the value of y when x = 0.b is the rate of change.The function for this problem is defined as follows:
f(x) = 2^(x - 1) + 2
It has an horizontal asymptote at y = 2, which removes the option C.
The parameter b is of b = 2, meaning that the function is increasing, which removes option D.
The numeric value of the function when x = 0 is given as follows:
f(0) = 2^(0 - 1) + 2
f(0) = 1/2 + 2
f(0) = 5/2
f(0) = 2.5.
Hence graph A is correct.
More can be learned about exponential functions at brainly.com/question/2456547
#SPJ1
The values in the table represent a function.
A 2-column table with 5 rows. The first column is labeled x with entries negative 6, 7, 4, 3, negative 5. The second column is labeled f of x with entries 8, 3, negative 5, negative 2, 12.
Use the drop-down menus to complete the statements.
The ordered pair given in the first row of the table can be written using function notation as
.
f(3) is
.
f(x) = –5 when x is
.
Answers
The correct answers are:
f(-6) = 8f(3) = -2f(x) = -5 when x is 4What is the function?Functions are expressions separated by an equal sign. They have both dependent and independent variables.
How to solve* Lets explain how to solve the problem
- The table of the function has two column
# First column labeled x with entries:
-6 , 7 , 4 , 3 , -5
# Second column labeled f(x) with entries:
8 , 3 , -5 , -2 , 12
∴ The ordered pairs of the function f(x) are:
(-6 , 8) , (7 , 3) , (4 , -5) , (3 , -2) , (-5 , 12)
* Lets complete the missing
∵ The value of x in the first row is -6
∵ The value of f(x) in the first row is 8
∴ The function notation in the 1st row is f(-6) = 8
- The ordered pair given in the first row of the table can be
written using function notation as f(-6) = 8
∵ The ordered pair whose x = 3 is (3 , -2)
∴ The value of f(x) when x = 3 is -2
∴ f(3) = -2
∵ The ordered pair whose f(x) = -5 is (4 , -5)
∴ The value of x when f(x) = -5 is 4
∴ f(x) = -5 when x is 4
To learn more about the function visit, https://brainly.com/question/28278690
What is the square root of -1?
Answers
Answer:
there isn't an answer, you can't get the square root of negative numbers. the sqaure root of a negative number does not exist in the set of Real Numbers
Rewrite this equation in slope- intercept from: 4y - 3x = 4
Answers
We are given the following equation
\(4y-3x=4\)We are asked to write the equation in slope-intercept from
Recall that slope-intercept is given by
\(y=mx+b\)Where m is the slope and b is the y-intercept.
So let us convert the given equation into the slope-intercept form by solving for y.
\(4y-3x=4\)Add 3x on both sides of the equation
\(\begin{gathered} 4y-3x+3x=3x+4 \\ 4y=3x+4 \end{gathered}\)Divide both sides of the equation by 4.
\(\begin{gathered} \frac{4y}{4}=\frac{3}{4}x+\frac{4}{4} \\ y=\frac{3}{4}x+1 \end{gathered}\)The slope is 3/4 and the y-intercept is 1
Therefore, the slope-intercept form of the given equation is
\(y=\frac{3}{4}x+1\)use differentials to estimate the amount of paint needed to apply a coat of paint 0.05 cm thick to a hemispherical dome with diameter 56 m. (round your answer to two decimal places.)
Answers
78.4π cm³ is the amount of paint needed to apply a coat of paint 0.05 cm thick to a hemispherical dome with diameter 56 m.
As we are in a hemisphere, we are aware that V= 2/3πr³. So,
dV= (2/3)3πr²dr = 2πr²dr
The required amount of paint is now
ΔV≈ dV= 2πr²dr
Given that the
diameter is 56 cm and that dr=0.05 cm and r=28 cm, the following is true:
dV= 2π(28)²(0.05)= 78.4π cm³
In geometry, the diameter of a circle is defined as any straight line segment with ends on the circle and that passes through its center. The longest chord of the circle is another name for it. Each of the two approaches can be used to determine the diameter of a sphere. The most straightforward way to begin is to use a ruler to measure the distance between the circle's outside corners beginning at its center. That would be the diameter.
Learn more about diameter here brainly.com/question/13624974
#SPJ4
Answer pls my state test is cominggggg
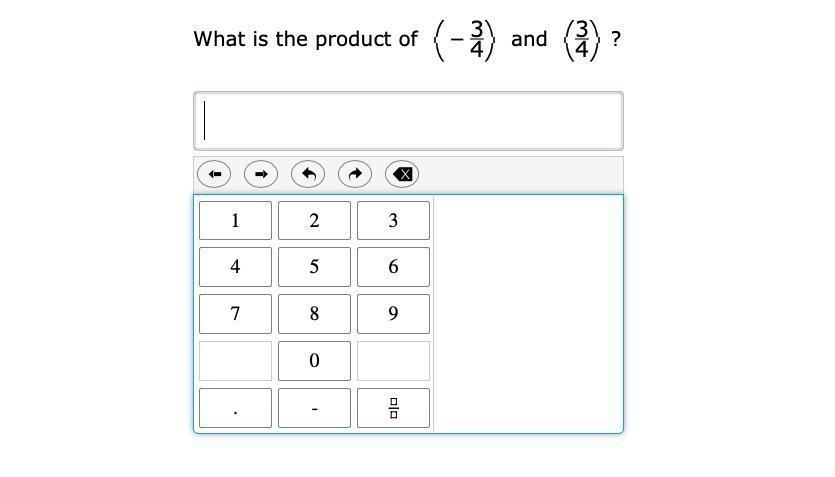
Answers
(-3/4) x (3/4)
---When we multiply fractions, we multiply horizontally/across
(-3 x 3) / (4 x 4)
-9 / 16
Answer: -9/16
Hope this helps!
Solve this problem using the Pythagorean Theorem. An extension ladder with 3 five-foot extensions is leaned against a wall with the base of the ladder 5 feet from the base of the wall. How high on the wall does the top of the ladder sit?
Answers
Given :-
An extension ladder with 3 five-foot extensions is leaned against a wall with the base of the ladder 5 feet from the base of the wall.To find :-
How high on the wall does the top of the ladder sit?Solution :-
Using Pythagoras Theorem ,
5² = 3² + h²25 = 9 + h ²h² = 25 - 9h ²= 16 h =√16 h = ±4 h = 4A payday loan store charges $45 for a one-month loan of $540. What annual interest rate is this equivalent to?
Answers
does anyone know the answer ?????

Answers
Answer:
4.4
Step-by-step explanation:
sin 15° = x/17
Multiply sin 15° by 17.
(Using a calculator)
The coordinates of three vertices of a rhombus are (-3, 0), (0, 5) and (3, 0). What are the coordinates of the fourth vertex?
Answers
SOLUTION
Given the question in the image, the following are the solution steps to answer the question.
STEP 1: Write the given coordinates
\(A(-3,0),B(0,5),C(3,0),D(x,y)\)STEP 2: State the side properties of a rhombus
In a rhombus, all sides are equal. This means that the length of the sides are equal and therefore the distances of the vertices apart will be the same.
And also, Diagonals of rhombus bisect each other. This implies that:
Co-ordinates of mid-points of AC= Co-ordinates of mid-points of BD
STEP 3: Find the distances of the sides
Midpoints of AC will be calculated as:
\(\begin{gathered} \mathrm{Midpoint\:of\:}\left(x_1,\:y_1\right),\:\left(x_2,\:y_2\right):\quad \left(\frac{x_2+x_1}{2},\:\:\frac{y_2+y_1}{2}\right) \\ \left(x_1,\:y_1\right)=\left(-3,\:0\right),\:\left(x_2,\:y_2\right)=\left(3,\:0\right) \\ =\left(\frac{3-3}{2},\:\frac{0+0}{2}\right) \\ =\left(0,\:0\right) \end{gathered}\)Midpoints of BD will be calculated as:
\(\begin{gathered} \mathrm{Midpoint\:of\:}\left(x_1,\:y_1\right),\:\left(x_2,\:y_2\right):\quad \left(\frac{x_2+x_1}{2},\:\:\frac{y_2+y_1}{2}\right) \\ \left(x_1,\:y_1\right)=\left(0,\:5\right),\:\left(x_2,\:y_2\right)=\left(x,\:y\right) \\ =\left(\frac{x+0}{2},\:\frac{y+5}{2}\right) \\ =\left(\frac{x}{2},\:\frac{y+5}{2}\right) \end{gathered}\)Since midpoints are the same as mentioned above, this means that:
\(\begin{gathered} \left(\frac{x}{2},\:\frac{y+5}{2}\right)=(0,0) \\ \frac{x}{2}=0,x=0 \\ \frac{y+5}{2}=0,y+5=0,y=-5 \\ \\ \therefore(x,y)=(0,-5) \end{gathered}\)Hence, the coordinates of the fourth vertex is given as:
\(\)is company name, state and age a categorical variable?
Answers
Answer:
yes
Step-by-step explanation:
you can classify people into categories by name, state, and age. Hope this helps!
PLZ CHECK MY ANSWER. Round your answer to the nearest tenth.
I chose D.
A: 72.56 cm^2
B: 80.29 cm^2
C: 60.66 cm^2
D: 70.32 cm^2

Answers
Answer:
D. \(70.34 cm^2\)
Step-by-step explanation:
Area of sector of a circle is given as θ/360*πr²
Where,
r = radius = 12 cm
θ = 56°
Use 3.14 as π
Plug in the values into the formula and solve
\( area = \frac{56}{360}*3.14*12^2 \)
\( area = 70.34 \)
Area of the sector ABC = \( 70.34 cm^2 \)
The answer is D
pls Help me now plssssss

Answers
z > 34. Which of the following statements is the best way to describe the value of z? (3 points) The value of z is less than 34. The value of z is more than 34. The value of z has a maximum of 34. The value of z has a minimum of 34.
Answers
Answer:
ans: value of z is greater than 34
Step-by-step explanation:
draw the number line as shown in picture

Need to know if f(x)=x-4 and g(x)=3x+5, find (f+g) (x)
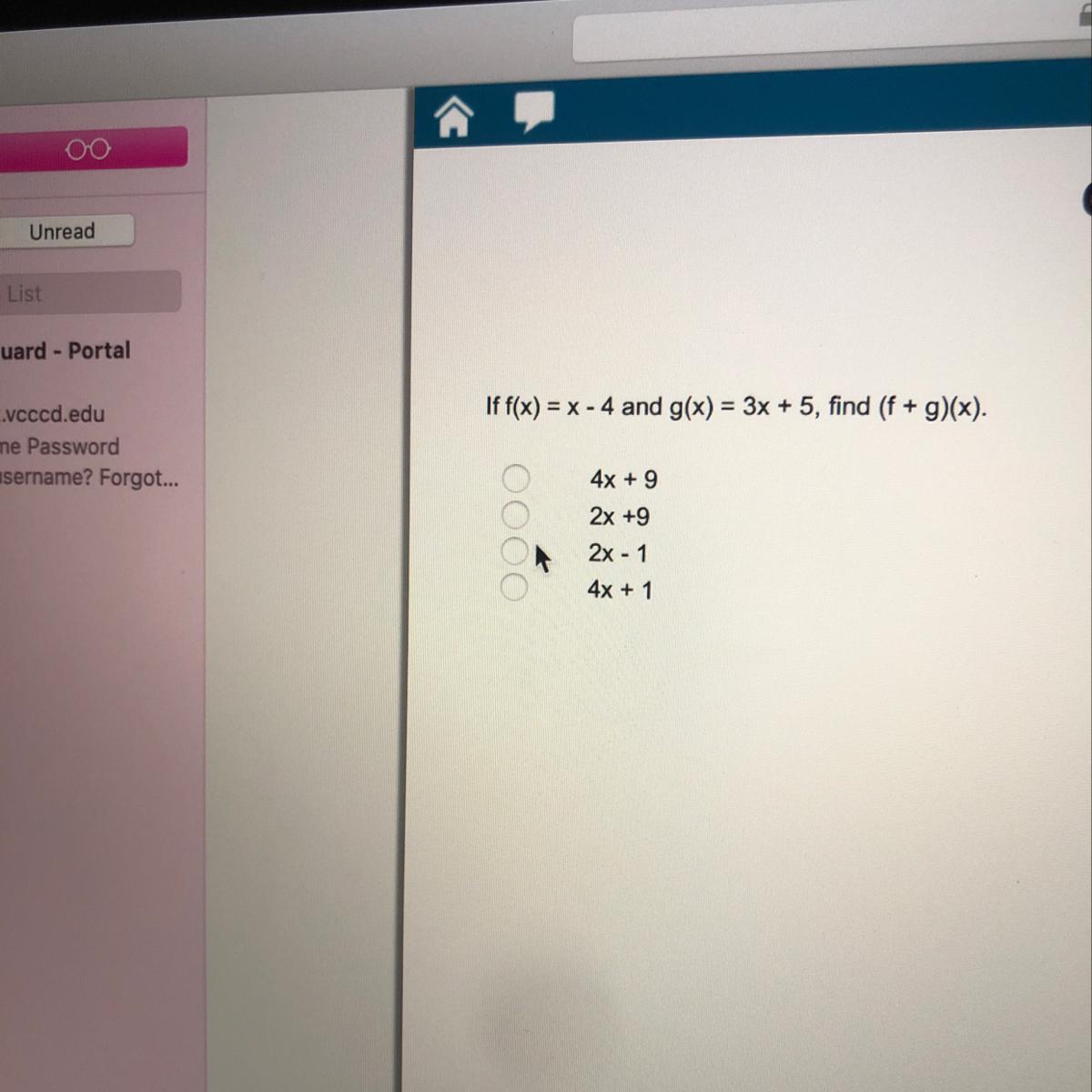
Answers
Answer:
4x+1
Step-by-step explanation:
f(x)=x-4 and g(x)=3x+5,
(f+g) (x) = x-4 +3x+5
Combine like terms
= 4x+1
Colah Company purchased $2,000,000 of Jackson, Inc., 6% bonds at par on July 1, 2021, with interest paid semi-annually. Colah determined that it should account for the bonds as an available-for-sale investment. At December 31, 2021, the Jackson bonds had a fair value of $2,300,000. Colah sold the Jackson bonds on July 1, 2022 for $1,800,000.
Required:
1. Prepare Colah’s journal entries for the following transactions:
The purchase of the Jackson bonds on July 1.
Interest revenue for the last half of 2021.
Any year-end 2021 adjusting entries.
Interest revenue for the first half of 2022.
Any entries necessary upon sale of the Jackson bonds on July 1, 2022, including updating the fair-value adjustment, recording any reclassification adjustment, and recording the sale.
2. Complete the following table to show the effect of the Jackson bonds on Colah’s net income, other comprehensive income, and comprehensive income for 2021, 2022, and cumulatively over 2021 and 2022.
Answers
If Colah Company purchased $2,000,000 of Jackson, Inc., 6% bonds at par on July 1, 2021. The journal entries for the purchase of the Jackson bonds on July 1 is: Debit Available-for-sale securities $2,000,000
Credit Cash $2,000,000.
What is journal entry?Journal entry is used by companies to record their day to day business transaction.
July 1 2021
Debit Available-for-sale securities $2,000,000
Credit Cash $2,000,000
December 31 2021
Debit Cash $60,000
Credit Interest income $60,000
(6% ×$2,000,000 / 2)
December 31 2021
Debit Available-for-sale securities $300,000
Unrealized Gain - Other comprehensive income $300,000
($2,000,000 - $2,300,000)
June 30 2022
Debit Cash $60,000
Credit Interest income $60,000
(6% ×$2,000,000 / 2)
The entry necessary upon sale of the Jackson bonds on July 1, 2022 is:
July 1 2022
Debit Available-for-sale securities $2,300,000
Unrealized Gain-Other comprehensive income account $300,000
Credit Cash $1,800,000
Credit Realized Loss on the sale of Available-for-sale securities $200,000
[$1,800,000 - $2,000,000= $200,000 (Realized Loss)]
2. Table to show the effect of the Jackson bonds on Colah’s net income,
2021 2022 Total
Net Income $60 ,000 ($140,000) ($80,000)
( 60,000 - $200,000= -$140,000)
OCI $300,000 $300,000
Comprehensive Income $360,000 ($140,000) $220,000
Therefore the journal entries for the purchase of the Jackson bonds on July 1 is: Debit Available-for-sale securities $2,000,000
Credit Cash $2,000,000.
Learn more about journal entry here:https://brainly.com/question/28390337
#SPJ1
Standard deviation is ________. a mean of squared differences the square root of the variance unit-free the covariance
Answers
Standard deviation is the square root of the variance. A mean of squared differences the square root of the variance unit-free the covariance.
What is standard deviation?The standard deviation is a measure of variance from the mean that includes spread, dispersion, and spread. The standard deviation displays a "typical" deviation from the mean. It is a well-liked measure of variability because it retains the original units of measurement from the data set. There is a small variation when data points are near the mean, and a large variation when they are far from the mean. The values' distance from the mean is determined by the standard deviation. The most common method of measuring dispersion is standard deviation, which is based on all values.
Hence, standard deviation is the square root of the variance.
Learn more about standard deviation here:
https://brainly.com/question/23907081
#SPJ4
|x–5|=|x+5| If you answer this question before 2:35 pm on July 28, 2020, I will give 10 points!!
Answers
Answer:
\(\boxed{x=0}\)
Step-by-step explanation:
\(|x-5|=|x+5|\)
Solve absolute value.
There are two possibilities.
First possibility:
\(x-5=x+5\\0=10\)
No solution.
Second possibility:
\(x-5=-(x + 5)\\x-5=-x-5\\2x=0\\x=0\)
Kristy's yearly salary is $75,000. How do you express this number in
scientific notation?
Answers
Answer:
7.5000
Step-by-step explanation:
Help
Complete the puzzle so that each row, column, and diagonal adds up to the same total.

Answers
Complete the puzzles with values -4, 0, -3 and 2
How to complete the puzzle?Since each row, column, and diagonal adds up to the same total.
For the 1st row, the total is:
3 + (-2) + (-1) = 0
That means each row, column, and diagonal will always adds up to 0.
3 + w + 1 = 0
w = -3-1
w = -4
w + y + 4 = 0
-4 + y + 4 = 0
y = 0
-1 + 4 + t = 0
3 + t = 0
t = -3
1 + h + t = 0
1 + h + (-3) = 0
h -2 = 0
h = 2
(Check the attached image for labeling)
Thus, complete the puzzle with the values accordingly
Learn more about puzzle on:
brainly.com/question/24168477
#SPJ1

The weight of the square is 10 grams. How much heavier is the circle than the square?
Answers
By adding the weight of the triangle, the weight of the circle heavier than that of the square.
How to determine how much heavier the circle is than the squareThe linear equation that results from examining the weights will contain the following parameters.
Let t stand for the triangle.
Let c stand for the circle.
Let's use the shape square.
left side of the equation = 6s + 3t
right side of the equation = 3s + 3c
equating both sides
6s + 3t = 3s + 3c
6s - 3s + 3t = 3s + 3c
3s + 3t = 3c
3(s + t) = 3c
dividing all through by 3
s + t = c
10 + t = c
Because of the resulting equation, we can conclude that the the circle is weightier than the square by the weight of the triangle
Learn more about equations at:
brainly.com/question/22688504
#SPJ1

5/12x6/15=30/180
How do I simplify?
Answers
Answer:
0.167
Step-by-step explanation:
if you divide 30/180 it will get you a decimal of 0.166666667 but if you were to divide 180 by 30 you will get 6
technecaly your answer would be 0.166666667 but you only take the first number in the decimal which is 0.1 then take six 0.16 then add the 7 0.167
that should end up being your answer if i did the math right
Answer:
Step-by-step explanation:
5/12 * 6/15 =30/180
1/6=1/6
which is true, Right-hand side is equal to left-hand side
need urgent help!
it’s a calculus question that has to do with periodic functions

Answers
Step-by-step explanation:
I can't draw it for you ( since no Desmos) , but I can tell you what it would look like
Cos wave beginning at 3 pm value ( x=0) = 75 (max)
center line at 65
Amplitude =10 :
max value 75 min value 55
One cycle will occur every 360/15 = 24 hours (x)
Then warmest will be at t = 0 (which is 3 PM) and 24 hours later = 75°
coldest will be at x = 12 hours (which will be 3 AM) = 55 degrees
at 7 pm you will be 4 hrs from the start which is 1/6th of the cycle or
pi / 3 the temp should read 65 + 10 cos (pi/3) = 70
at 7 AM : this is 16 hrs out of 24 hr cycle = 16 / 24 * 2 pi = 4 /3 pi
temp should be 65 + 10 cos (4/3 pi) = 60 degrees
Answer:
Step-by-step explanation:
For other question

Hey i need help on these
Determine if the following side lengths will form a triangle using the Triangle Inequality Theorem.
33.7, 62.9, 96.6
A.The three sides do not form a triangle.
B. The three sides do form a triangle.
Answers
Answer:
A. no triangle
Step-by-step explanation:
You want to know if the Triangle Inequality Theorem allows a triangle from side lengths 33.7, 62.9, and 96.6.
Triangle inequality theoremThe theorem requires for any side lengths a, b, c, this inequality must hold:
a + b > c
ApplicationUsing the given side lengths, we have ...
33.7 +62.9 > 96.6
96.6 > 96.6 . . . . . . . . False
The three sides do not form a triangle.
__
Additional comment
These side lengths will join ends to ends to form a "triangle" that looks like a line segment of length 96.6 with a point on it at 33.7 from one end. Most authors do not recognize this figure as a triangle. For those authors who write the theorem as a +b ≥ c, these segments will form a triangle.
You should check your curriculum materials to see whether your Triangle Inequality Theorem uses the > or the ≥ relation.
#95141404393
How many 11-card hands are possible with a 20-card deck?
Answers
There is only 1 possible 11-card hand that can be formed from a 20-card deck.
To determine the number of 11-card hands possible with a 20-card deck, we can use the concept of combinations.
The number of combinations, denoted as "nCk," represents the number of ways to choose k items from a set of n items without regard to the order. In this case, we want to find the number of 11-card hands from a 20-card deck.
The formula for combinations is:
nCk = n! / (k!(n-k)!)
Where "!" denotes the factorial of a number.
Substituting the values into the formula:
20C11 = 20! / (11!(20-11)!)
Simplifying further:
20C11 = 20! / (11! * 9!)
Now, let's calculate the factorial values:
20! = 20 * 19 * 18 * ... * 2 * 1
11! = 11 * 10 * 9 * ... * 2 * 1
9! = 9 * 8 * 7 * ... * 2 * 1
By canceling out common terms in the numerator and denominator, we get:
20C11 = (20 * 19 * 18 * ... * 12) / (11 * 10 * 9 * ... * 2 * 1)
Performing the multiplication:
20C11 = 39,916,800 / 39,916,800
Finally, the result simplifies to:
20C11 = 1
Consequently, with a 20-card deck, there is only one potential 11-card hand.
for such more question on combinations
https://brainly.com/question/28065038
#SPJ8
1. Javier scored 78% on the test. Write this
percent as a fraction in simplest form and
as a decimal.
Answers
As a fraction: 39/50
As a decimal: .78
Answer:39/50
Step-by-step explanation:
i beg u guys pleas help

Answers
Answer:
a^-7
Step-by-step explanation:
Option C. a^-7 is the answer.
Use the function f(x) to answer the questions:
f(x) = 2x2 − 5x + 3
Part A: What are the x-intercepts of the graph of f(x)? Show your work.
Part B: Is the vertex of the graph of f(x) going to be a maximum or a minimum? What are the coordinates of the vertex? Justify your answers and show your work.
Part C: What are the steps you would use to graph f(x)? Justify that you can use the answers obtained in Part A and Part B to draw the graph.
Answers
The x-intercepts of the graph of f(x) are x = 3/2 and x = 1,the Vertex of the graph of f(x) is (5/4, 3/8), and it is a minimum point, The vertex is at (5/4, 3/8). This is the minimum point of the graph.
Part A: To find the x-intercepts of the graph of f(x), we set f(x) equal to zero and solve for x.
2x^2 - 5x + 3 = 0
To factor this quadratic equation, we look for two numbers that multiply to give 3 (the coefficient of the constant term) and add up to -5 (the coefficient of the linear term). These numbers are -3 and -1.
2x^2 - 3x - 2x + 3 = 0
x(2x - 3) - 1(2x - 3) = 0
(2x - 3)(x - 1) = 0
Setting each factor equal to zero, we get:
2x - 3 = 0 --> x = 3/2
x - 1 = 0 --> x = 1
Therefore, the x-intercepts of the graph of f(x) are x = 3/2 and x = 1.
Part B: To determine whether the vertex of the graph of f(x) is a maximum or a minimum, we look at the coefficient of the x^2 term, which is positive (2 in this case). A positive coefficient indicates that the parabola opens upwards, so the vertex will be a minimum.
To find the coordinates of the vertex, we can use the formula x = -b/2a. In the equation f(x) = 2x^2 - 5x + 3, the coefficient of the x term is -5, and the coefficient of the x^2 term is 2.
x = -(-5) / (2*2) = 5/4
Substituting this value of x back into the equation, we can find the y-coordinate:
f(5/4) = 2(5/4)^2 - 5(5/4) + 3 = 25/8 - 25/4 + 3 = 3/8
Therefore, the vertex of the graph of f(x) is (5/4, 3/8), and it is a minimum point.
Part C: To graph f(x), we can use the information obtained in Part A and Part B.
- The x-intercepts are x = 3/2 and x = 1. These are the points where the graph intersects the x-axis.
- The vertex is at (5/4, 3/8). This is the minimum point of the graph.
We can plot these points on a coordinate plane and draw a smooth curve passing through the x-intercepts and the vertex. Since the coefficient of the x^2 term is positive, the parabola opens upwards, and the graph will be concave up.
Additionally, we can consider the symmetry of the graph. Since the coefficient of the linear term is -5, the line of symmetry is given by x = -(-5) / (2*2) = 5/4, which is the x-coordinate of the vertex. The graph will be symmetric with respect to this line.
By connecting the plotted points and sketching the curve smoothly, we can accurately graph the function f(x).
For more such questions on Vertex .
https://brainly.com/question/28747454
#SPJ8
Please awnser asap I will brainlist

Answers
Using simultaneous equation, the solution to the system of linear equations are 1223 $10 tickets, 1332 $20 tickets, and 763 $30 tickets were sold.
How many tickets of each kind has been sold?Let's solve the problem step by step.
Let:
x = number of $10 tickets sold
y = number of $20 tickets sold
z = number of $30 tickets sold
From the given information, we can form the following equations:
Equation 1: x + y + z = 3318 (Total number of tickets sold)
Equation 2: y = x + 109 (109 more $20 tickets than $10 tickets were sold)
Equation 3: 10x + 20y + 30z = 61760 (Total sales from ticket sales)
We can use these three equations to solve for the values of x, y, and z.
First, let's substitute Equation 2 into Equation 1:
x + (x + 109) + z = 3318
2x + 109 + z = 3318
2x + z = 3209 (Equation 4)
Now, let's substitute the value of y from Equation 2 into Equation 3:
10x + 20(x + 109) + 30z = 61760
10x + 20x + 2180 + 30z = 61760
30x + 30z = 59580
x + z = 1986 (Equation 5)
We now have a system of equations (Equations 4 and 5) with two variables (x and z). We can solve this system to find the values of x and z.
Multiplying Equation 4 by 30, and Equation 5 by 2, we get:
60x + 30z = 96270 (Equation 6)
2x + 2z = 3972 (Equation 7)
Now, subtract Equation 7 from Equation 6:
(60x + 30z) - (2x + 2z) = 96270 - 3972
58x + 28z = 92298
Simplifying, we have:
29x + 14z = 46149 (Equation 8)
Now, we can solve Equations 5 and 8 simultaneously:
x + z = 1986 (Equation 5)
29x + 14z = 46149 (Equation 8)
Multiplying Equation 5 by 14, and Equation 8 by 1, we get:
14x + 14z = 27804 (Equation 9)
29x + 14z = 46149 (Equation 8)
Now, subtract Equation 9 from Equation 8:
(29x + 14z) - (14x + 14z) = 46149 - 27804
15x = 18345
Divide both sides of the equation by 15:
x = 18345 / 15
x = 1223
Substituting the value of x into Equation 5, we can find z:
1223 + z = 1986
z = 1986 - 1223
z = 763
Now that we have the values of x and z, we can substitute them back into Equation 1 to find y:
1223 + y + 763 = 3318
y + 1986 = 3318
y = 3318 - 1986
y = 1332
Therefore, the solution to the problem is:
x = 1223 (number of $10 tickets sold)
y = 1332 (number of $20 tickets sold)
z = 763 (number of $30 tickets sold)
Learn more on system of linear equation here;
https://brainly.com/question/13729904
#SPJ1