The population of a city can be modeled by ( P(t)=40 e^{0.05 t} thousand persons, where ( t ) is the number of years after ( 2000 . Approximately how rapidly will the city's population be changing in 2027? The population will be changing by thousand persons/year. (Enter your answer rounded to at least three decimal places)
Answers
The city's population is changing at a rate of approximately [insert answer] thousand persons/year in 2027.
To find the rate of change of the population in 2027, we need to calculate the derivative of the population function with respect to time (t) and evaluate it at t = 27 (since 2027 is 27 years after 2000).
Taking the derivative of the population function P(t) = 40e^(0.05t) with respect to t, we use the chain rule and obtain P'(t) = 40 * 0.05 * e^(0.05t).
Substituting t = 27 into the derivative expression, we have P'(27) = 40 * 0.05 * e^(0.05 * 27). Evaluating this expression will give us the approximate rate of change of the city's population in 2027, rounded to at least three decimal places.
Learn more about function here: brainly.com/question/30660139
#SPJ11
Related Questions
98.42 divided by 1.8
Answers
Answer:
4921/90
Step-by-step explanation:
Carl earned grades of 62,78,59,and 89 on four math testWhat is the mean of his grade
Answers
The is the average of a set of given numbers or data.
It can be found by adding all the numbers/data together and then dividing it by the number of items in the set. Which can also be represented by the equation;
\(\operatorname{mean}=\frac{Sum\text{ of all the data}}{\text{Number of Data}}\)So for our question we have the grades 62, 78, 59 and 89. All in all we have 4 grades.
In order for us to find the mean we must first add all our grades together, so we have;
\(62+78+59+89=288\)Now that we have the total of the grades which is 288, we will now divide it by the number of grade there is (which is 4) in order to find the mean.
\(\operatorname{mean}=\frac{288}{4}=72\)Therefore our MEAN is 72.
And now we can say that the average grade that Carl have in all 4 math test is 72.
I need help trying to find the area of this triangle please help.

Answers
Answer:
28 ft²
Step-by-step explanation:
You want the area of a triangle with a base of 7' and a height of 8'.
Area formulaThe formula for the area of a triangle is ...
A = 1/2bh
where b is the base of the triangle, and h is the height perpendicular to the base.
Here, it is convenient to use the side marked 7' as the base of the triangle. The distance marked 8' is the perpendicular distance from the base to the top of the triangle. So, the area is ...
A = 1/2(7 ft)(8 ft) = 28 ft²
The area of the triangle is 28 square feet.
__
Additional comment
As you can see, the vertex opposite the base does not need to lie above the base segment. All that matters is the perpendicular distance from that vertex to the base line.
Solve for p.
–
19p–2p+16p+12=
–
18
p=
Answers
Answer:
6
BRAINLIEST, PLEASE!
Step-by-step explanation:
-19p - 2p + 16p + 12 = -18
-5p + 12 = -18
-5p = -30
p = 6
Answer:
p = 6
Step-by-step explanation:
Given
- 19p - 2p + 16p + 12 = - 18 ( simplify left side )
- 5p + 12 = - 18 ( subtract 12 from both sides )
- 5p = - 30 ( divide both sides by - 5 )
p = 6
5x – 9 = 3x + 7
Solve for x
Answers
Answer:
Solving for x
5x - 9 = 3x + 7
subtract 3x from both sides
-3x -3x
2x - 9 = 7
get rid of constants by adding 9 on both sides since addition is the inverse operation of subtraction
+9 +9
2x = 16
divide by 2 since that is the inverse operation of multiplication
/2 /2
x = 8
The solution for ''x'' for the expression ( 5x – 9 = 3x + 7
What is an expression?Expressions can be evaluated, meaning that they can be simplified to a single value. The value of an expression depends on the values of any variables or numbers within the expression.
To solve for x in equation 5x - 9 = 3x + 7, we want to isolate x on one side of the equation. We can do this by performing the same operation on both sides of the equation, in order to keep the equation balanced.
First, we can simplify both sides of the equation by combining like terms:
5x - 9 = 3x + 7
2x - 9 = 7
Next, we can add 9 to both sides of the equation to isolate the variable term:
2x = 16
Finally, we can divide both sides of the equation by 2 to get the value of x:
x = 8
Therefore, the solution to the equation 5x - 9 = 3x + 7 is x = 8.
To know more about an expression follow
https://brainly.com/question/25968875
#SPJ2
Given: NM // XZ
We know that side NM is
v to side XZ. If
Prove: AXYZ ~ ANYM
we consider side NY the transversal for these parallel
lines, we create angle pairs. Using the
v. we can state that
ZYXZ is congruent to ZYNM. We know that angle
XYZ is congruent to angle
v by the reflexive
property. Therefore, triangle XYZ is similar to triangle
NYM by the
similarity theorem.
M

Answers
A transversal is a straight line that intersects two parallel lines forming four angles at each point of intersection. Thus the required answers to the given question are:
i. Side NM is parallel to side XZ.
ii. Using the corresponding property
iii. <XYZ is congruent to <NYM
iv. ΔXYZ is similar toΔNYM by SAS theorem
Two or more angles are said to be congruent if and only if they have equal measure. This property can be used to relate equal angles.
While a transversal is a straight line that intersects two parallel lines forming four angles at each point of the intersection.
The required answers to the question are as stated below:
i. Side NM is parallel to side XZ.
ii. The corresponding property
iii. <XYZ is congruent to <NYM
iv. ΔXYZ is similar toΔNYM by SAS theorem
For more clarifications on congruent angles of plane shapes, visit: https://brainly.com/question/3717644
#SPJ1
Answer: For the first box it is parallel, for the second box it is the corresponding angles theorem, the third is NYM, and the last one is AA.
Step-by-step explanation: 1.) Since line NM has an arrow on it's line and XZ has an arrow on it's line, that means that those two line are parallel to each other. 2.) Since both triangle contain the same transversal line (NY) and the corresponding angles theorem states that if a transversal intersects parallel lines, the corresponding angles are congruent. From this we can conclude that this means that the angle <YXZ has is congruent to the angle <YNM. Therefore we use the corresponding angles theorem. 3.) Answer 2 basically answers box number three but since we concluded that Angles of <YXZ and <YNM are both congruent <NYM would be your answer its just flipped. 4.) We use the AA theorem, because the AA theorem states that if an angle or angle(s) of one triangle is congruent to another angle/angle(s) of another triangle, then the triangles are similar (congruent). Therefore we would use the AA theorem.
Hope this helps :)
By the way this is the answer for EDGE in 2023
Every 2 days a scientist spends 1.25 hours observing dolphins and 3.5 hours observing sea lions at this rate how many total hours will the scientist spend observing dolphins and sea lions in 6 days?
Answers
Answer:
Step-by-step explanation:
in the course of 6 days, the scientist spends every 2 days observing
So for example starting on monday, that would be monday-saturday except only monday, wed, and friday.
Thats three days
3(1.25+3.5)= 14.25 hours
I need help determining whether some of these numbers are irrational and rational
Then on the other one i need to determine whether the decimal is terminating or repeating if you can help that would be great and I will be giving brainiest


Answers
The rational numbers are: 6.31245689, 1/6, √16, 1.3, 0, and √7569
The irrational numbers are: √2, π, √21.
The terminating decimals are:1/2, 1/5, 5/8, 12.567, and 1/4
The repeating decimals are:1/9, 6/9, 7/22, 9/11 and .3
How to determine the difference between the numbersA rational number can be written as a fraction, where both the numerator and denominator are integers. Rational numbers can be expressed as finite decimals or repeating decimals.
The following are rational numbers: 6.31245689, 1/6, √16, 1.3, 0, and √7569
While irrational numbers are those that cannot be expressed in this way and have non-repeating, non-terminating decimal representations.
The following are irrational numbers: √2, π, √21.
Finite decimals are decimals that have a terminating number of digits after the decimal point.
The following are all finite decimals:1/2, 1/5, 5/8, 12.567, and 1/4
Repeating decimals, on the other hand, are decimals that have a pattern of digits that repeat indefinitely after the decimal point. The following are repeating decimals:1/9, 6/9, 7/22, 9/11 and .3
In conclusion, rational numbers have a decimal expansion that either stop or repeats, while irrational numbers have a decimal expansion that goes on non-stop without repeating. A decimal is repeating if and only if it can be written as a fraction whose denominator is not divisible by any prime other than 2 or 5.
Read more about numbers here:https://brainly.com/question/5796983
#SPJ1
What is irrational statement?
Answers
An irrational statement is a kind of genuine number that can't be addressed as a basic portion. It can't be communicated in that frame of mind of a proportion. If N is silly, N isn't equivalent to p/q where p and q are numbers and q isn't equivalent to 0. Model: √2, √3, √5, √11, √21, π(Pi) are nonsensical.
A number can't be communicated as a portion of any numbers and. . Unreasonable numbers have decimal developments that neither end nor become occasional. Each supernatural number is irrational.
If a square root is not an ideal square, then it is viewed as an unreasonable number. These numbers can't be composed as a portion because the decimal doesn't end (non-terminating) and doesn't rehash an example (non-repeating).
Learn more about irrational statement:
https://brainly.com/question/29204809
#SPJ4
Jordan bought a $300,000 house, paying 10% down, and getting a 30 year loan with 3% interest for the remaining amount.
How much is the loan amount going to be?
What will her monthly payments be?
How much interest will she pay over the life of the loan?
Answers
Answer:
The down payment that Jordan made on the house was $300,000 x 10% = $30,000. So, he financed $300,000 - $30,000 = $270,000.
where
M = monthly payment
P = loan amount ($270,000)
r = monthly interest rate (3% / 12 months = 0.003)
n = number of payments (30 years x 12 months/year = 360)
This comes out to a monthly payment of approximately $1,173.38.
To simplify
The loan amount is- $270,000
Her monthly payment will be- $1,173.38
The amount of interest she will pay is- $8,100
Erik and Nita are playing a game with numbers. In the game, they each think of a random number from 0 to 20. If the difference between their two numbers is less than 10, then Erik wins. If the difference between their two numbers is greater than 10, then Nita wins. Use the information in the interactive and what you know about absolute value inequalities to better understand the game.
Answers
Question:
Write an algebraic statement that represents all the ways your player will win. Be sure to define your variable
Answer:
Erica:
\(0 \leq |x - y| < 10\)
Nita:
\(10 < |x - y| \leq 20\)
Step-by-step explanation:
Given
Players: Erica & Nita
Range: 0 to 20
Represent Erica with x and Nita with y
For Erica to win;
The difference between x and y must be less than 10 but greater than or equal to 0
i.e.
\(0 \leq x - y \leq 10\) or \(0 \leq y - x \leq 10\)
These two expressions can be merged together to be:
\(0 \leq |x - y| < 10\)
For Nita to win;
The difference between x and y must be greater than 10 but less than or equal to 20
i.e.
\(10 < x - y \leq 20\) or \(10 < y - x \leq 20\)
These two expressions can be merged together to be:
\(10 < |x - y| \leq 20\)
√144² =
√9 + √16 =
√9 + √16 × √400 =
Answers
Answer:
Step-by-step explanation
√144²
12²
144
√9 + √16
3 + 4
7
√9 + √16 × √400 =
3 + 2^{4} x 5
3 + 80
83
Celo used the simple interest formula uppercase A = uppercase P (1 + r t) to calculate the interest he earned on his savings last month. Which equation is equivalent to the simple interest formula? uppercase P = StartFraction uppercase A Over 1 + r t EndFraction uppercase P = StartFraction 1 + r t Over uppercase A EndFraction uppercase P = uppercase A minus (1 + r t) uppercase P = uppercase A + (1 + r t)
Answers
Answer:
uppercase P = StartFraction uppercase A Over 1 + r t EndFraction
P = A/(1+rt)
Step-by-step explanation:
A. uppercase P = StartFraction uppercase A Over 1 + r t EndFraction
P = A/(1+rt)
B. uppercase P = StartFraction 1 + r t Over uppercase A EndFraction
P = (1+rt)/A
C. uppercase P = uppercase A minus (1 + r t)
P = A - (1+rt)
D. uppercase P = uppercase A + (1 + r t)
P = A + (1 + rt)
Simple interest formula celo used
A = uppercase P (1 + r t)
A = P(1 + rt)
Where,
A = final balance
P = principal
r = interest rate
t = time (years)
Make p the subject of the formula
A = P(1 + rt)
P = A/(1 + rt)
Answer:
The correct answer is A.
Step-by-step explanation:
P= A/ 1+rt
Select the correct statement about the function represented by the table.
Plz help!

Answers
Answer:
I believe that it is B
a committee of five men and four women is to be formed from 9 men and women find the number of ways which the committee can be a) formed b) formed if it must have two particular men
Answers
Answer: This can be done in 9C5 ways (9 choose 5), which is calculated as 9!/(5! 4!) = 126 ways.
determine a cartesian equation for the curve given in parametric form by x(t) = 4 ln(9t), y(t) = √ t .
Answers
To determine a cartesian equation for the curve given in parametric form by x(t) = 4 ln(9t), y(t) = √ t, we need to eliminate the parameter t.
Here are the following steps:
First, we can solve for t in terms of y by squaring both sides of the equation y(t) = √ t:y^2 = tNext, we can substitute this expression for t into the equation for x(t):
x(t) = 4 ln(9t) = 4 ln(9(y^2))
Using the properties of logarithms, we can simplify this expression:
x(t) = 4 ln(9) + 4 ln(y^2) = 4 ln(81y^2)
Finally, we have eliminated the parameter t and obtained a Cartesian equation in terms of x and y:
x = 4 ln(81y^2) = 8 ln(9y)
Therefore, the cartesian equation for the curve given in parametric form by x(t) = 4 ln(9t), y(t) = √ t is x = 8 ln(9y).
To learn more about “cartesian equation” refer to the https://brainly.com/question/31405851
#SPJ11
A circle has an area of 149 cm². Calculate the radius of the circle. Give your answer correct to 1 decimal place.
Answers
Step-by-step explanation:
pi r^2 = circle area
pi r^2 = 149
r^2 = 149 / pi
r = 6.9 cm
Find YZ.
X 2x + 3
27-
Y
3x - 1
N

Answers
===============================================
Explanation:
The two pieces XY and YZ add to segment XZ
XY + YZ = XZ
(2x+3) + (3x-1) = 27
2x+3 + 3x-1 = 27
(2x+3x) + (3-1) = 27
5x+2 = 27
5x = 27-2
5x = 25
x = 25/5
x = 5
Then,
XY = 2x+3 = 2*5+3 = 10+3 = 13YZ = 3x-1 = 3*5-1 = 15-1 = 14As a check: XY + YZ = 13+14 = 27 which confirms the answer.
each tile in the model represents 1/4. How many titles make a group of 3/4
Answers
Answer:
3 tiles
because 1/4+1/4+1/4=3/4
I hope this helps you!
for the math experts again!
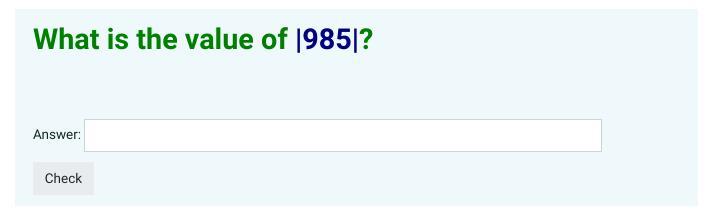
Answers
Answer:
985
Step-by-step explanation:
The absolute value of a number is its distance from 0. This means a number would be itself if it was positive and if it was negative it would be its opposite. Since 985 is positive, |985| would just be 985.
Conrad bought 21 stamps from a hobby shop. He gave 3/7 of them to his brother. He put
the rest of the stamps in his collection book. How many of the stamps did he put in his
collection book?
Answers
a new brand of sausage is tested on 700 randomly selected consumers in grocery stores with 630 saying they like the product, the others saying they do not. what is the empirical probability that a consumer will like this brand of sausage?
Answers
The empirical probability that a consumer will like this brand of sausage is 0.9 or 90%.
The ratio of the number of times an event occurs to all possible trials is the empirical probability that the event will occur. The occurrence in this instance is comparable to the brand of sausage.
The total number of consumers who tested was 700, and 630 of them rated the sausage favorably. As a result, the empirical likelihood that a customer will enjoy this brand of sausage is:
Empirical Probability = Number of consumers who liked the sausage / Total number of consumers tested
Empirical Probability = 630 / 700
Empirical Probability = 0.9
Therefore, the empirical probability that a consumer will like this brand of sausage is 0.9 or 90%.
To learn more about empirical probability visit the link below:
https://brainly.com/question/15412351
#SPJ4
Find a vector parallel to the line of intersection of the planes given by the equations 2x - 3y + 5z = 2 and 4x + y - 3z = 7
Answers
The vector <-32, 44, 22> is parallel to the line of intersection of the two planes given by the equations 2x - 3y + 5z = 2 and 4x + y - 3z = 7.
To find a vector parallel to the line of intersection of the planes given by the equations 2x - 3y + 5z = 2 and 4x + y - 3z = 7, we first need to find the direction vector of the line of intersection.
We can find the direction vector by taking the cross product of the normal vectors of the two planes. The normal vector of the plane 2x - 3y + 5z = 2 is <2, -3, 5>, and the normal vector of the plane 4x + y - 3z = 7 is <4, 1, -3>. Taking the cross product of these two vectors, we get:
<2, -3, 5> × <4, 1, -3> = <-16, 22, 11>
This vector <-16, 22, 11> is the direction vector of the line of intersection of the two planes.
To find a vector parallel to this line, we can simply multiply the direction vector by a scalar. For example, we can choose a scalar of 2 to get:
2<-16, 22, 11> = <-32, 44, 22>
Therefore, the vector <-32, 44, 22> is parallel to the line of intersection of the two planes given by the equations 2x - 3y + 5z = 2 and 4x + y - 3z = 7.
Learn more about line of intersection here
https://brainly.com/question/31743634
#SPJ11
Please answer correctly! I will mark you Brainliest!

Answers
Answer:
4.1 inches
I would appreciate Brainliest, but no worries.
Answer:
6
Step-by-step explanation:
the formula for the sphere's volume is \(\frac{4}{3} *\pi *r^3\)
so when you set that equal to 288\(\pi\), you get 6 as the radius
Pls Reply before tommorrow
1. A bathtub is being filled at a rate of 2.5 gallons per minute. The bathtub will
hold 20 gallons of water.
a. How long will it take to fill the bathtub?
b. Is the relationship described linear, inverse, exponential, or neither? Write
an equation relating the variables.
2. Suppose a single bacterium lands on one of your teeth and starts reproducing
by a factor of 4 every hour.
a. After how many hours will there be at least 1,000,000 bacteria in the new
colony?
b. Is the relationship described linear, inverse, exponential, or neither? Write
an equation relating the variables.
Answers
1.
a.
It will take 8 minutes to fill the bathtub.
b.
The relationship described is linear.
The equation is 20 = 2.5t + 0.
2.
a.
It will take approximately 4.807 hours to have at least 1,000,000 bacteria in the new colony.
b.
The relationship described is exponential,
The equation is Number of bacteria = initial number of bacteria x (reproduction factor)^(time/hour)
We have,
1.
a.
To fill the bathtub, we need 20 gallons of water.
The rate at which the water is being filled is 2.5 gallons per minute.
Using the formula:
time = amount/rate
we get:
time = 20/2.5 = 8 minutes
b.
The relationship described is linear.
The equation relating the variables can be written as:
amount of water = rate x time + initial amount
where the rate is 2.5 gallons per minute, the initial amount is 0 gallons, and the amount of water is 20 gallons.
So, the equation is:
20 = 2.5t + 0
where t is the time in minutes.
2.
a.
The relationship described is exponential.
The equation relating the variables can be written as:
number of bacteria = initial number of bacteria x (reproduction factor)^(time/hour)
where the initial number of bacteria is 1, the reproduction factor is 4, and we need to find the time it takes to reach 1,000,000 bacteria.
So, we have:
1,000,000 = 1 x 4^(time/hour)
Taking the logarithm of both sides, we get:
log(1,000,000) = log(4^(time/hour))
6 = (time/hour) x log(4)
time/hour = 6/log(4)
time = (6/log(4)) x hour
time ≈ 4.807 hours
b.
The relationship described is exponential, and the equation relating the variables is:
Number of bacteria = initial number of bacteria x (reproduction factor)^(time/hour)
where the initial number of bacteria is 1, the reproduction factor is 4, and t is the time in hours.
Thus,
1.
a.
It will take 8 minutes to fill the bathtub.
b.
The relationship described is linear.
The equation is 20 = 2.5t + 0.
2.
a.
It will take approximately 4.807 hours to have at least 1,000,000 bacteria in the new colony.
b.
The relationship described is exponential,
The equation is Number of bacteria = initial number of bacteria x (reproduction factor)^(time/hour)
Learn more about equations here:
https://brainly.com/question/17194269
#SPJ1
Two cars travel at the same speed to different destinations. Car A reaches its destination in 12 minutes. Car B reaches its destination in 18 minutes. Car B travels 4 miles farther than Car A. How fast do the cars travel? Write your answer as a fraction in simplest form.
Answers
Answer:
chatGPT
Step-by-step explanation:
Let's denote the speed of each car as v, and the distance that Car A travels as d. Then we can set up two equations based on the information given:
d = v * (12/60) (since Car A reaches its destination in 12 minutes)
d + 4 = v * (18/60) (since Car B travels 4 miles farther than Car A and reaches its destination in 18 minutes)
Simplifying the equations by multiplying both sides by 60 (to convert the minutes to hours) and canceling out v, we get:
12v = 60d
18v = 60d + 240
Subtracting the first equation from the second, we get:
6v = 240
Therefore:
v = 240/6 = 40
So the cars travel at a speed of 40 miles per hour.
the inside diameter (in inches) of 50 lightweight snaps used in assembling computer cases are measured and sorted with the following resulting data: 0.0395 0.0443 0.0450 0.0459 0.0470 0.0485 0.0486 0.0487 0.0489 0.0496 0.0499 0.0500 0.0503 0.0504 0.0504 0.0516 0.0529 0.0542 0.0550 0.0571 (a) compute the sample mean and sample variance. (b) find the sample upper and lower quartiles. (c) find the sample median. (d) construct a box plot of the data. (e) find the 5th and 95th percentiles of the inside diameter.
Answers
(a) the sample mean is 0.0494 and the sample variance is 0.000016, (b) the upper quartile is 0.04775, and the lower quartile is 0.0510, (c) the sample median is 0.04975, (d) boxplot is attached, and (e) the 5th and 95th percentiles of the inside diameter are 0.03974 and 0.056995 respectively.
(a) The mean = sum of all values divided by the number of values
μ = (x1 + x2 + ..... + xn)/n
n = 20
μ = (0.0395 + 0.0443+ 0.0450 + ... + 0.0550 + 0.0571)/20
μ = 0.9878/20
μ = 0.0494
(b) Variance = sum of squared deviations from the mean divided by n-1
s² = {(x1-μ)² + (x2-μ)² + .... (xn - μ)²)/(n-1)
s² = {(0.0395-0.0494)² + (0.0443-0.0494)² + .... +(0.0571-0.0494)²}/19
s² = 0.000016
(b) The minimum is 0.0395 and the maximum is 0.0571.
since the number of data is even, the median will be the average of two middle values.
M = Q2 = (0.0496+0.0499)/2 = 0.04975
Now, the first quartile is the median of the data values below the median
so Q1 = (0.0470+0.0485)/2 = 0.04775
And third quartile will be the median of the data values above the median
Q3 = (0.0504+0.0516)/2 = 0.0510
(c) Since we know that the number of data values is even, the median will be the average of the two middle values of the data set
so M = (0.0496+0.0499)/2
or M = 0.04975
(d) The boxplot is at maximum and minimum values. It will start in Q1 and end in Q3 and has a vertical line at the median or Q2.
The boxplot is attached.
(e) The 5th percentile means 0.05(n+1)th data value
or = 0.05(20+1) = 1.05th data value
5th percentile = 0.0550 + 0.05(0.0443-0.0395) = 0.03974
similarly,
95th percentile = 0.0550 + 0.95(0.0571-0.0550) = 0.056995
Therefore, (a) the sample mean is 0.0494 and the sample variance is 0.000016, (b) the upper quartile is 0.04775, and the lower quartile is 0.0510, (c) the sample median is 0.04975, and (e) the 5th and 95th percentiles of the inside diameter are 0.03974 and 0.056995 respectively.
To read more about quartile:
https://brainly.com/question/15642066
#SPJ4

I NEED HELP PLEASE !!!!

Answers
Answer:
No, all of her work is correct.
Step-by-step explanation:
Answer:
No, all of her work is correct.
Step-by-step explanation:
All of her work is correct.
The first step is showing factorization of √50
The second step is simplifying the factorization
The third step is simplifying the entire radical.
When you take a square root of a square, they cancel out, so:
√5² = 5
We multiply it with our leftover √2 and we get:
5√2
what is the percent change if the number of infraction drops from 25 to 15
Answers
To find the percentage change, we have to divide
\(\frac{25}{15}=1.66\ldots\)
Prove that the points (a, -3a), (2a, a) and (0, -2a) form a scalene trqingle
Answers
Answer:
See below.
Step-by-step explanation:
Remember that a scalene triangle has lengths of different values.
Therefore, we just need to find the length or distance from each point to the next. If the three distances we acquire are different, then we prove that the point do indeed form a scalene triangle.
Let's let A be (a, -3a), B be (2a, a), and C be (0, -2a).
So, let's find each of the side lengths using the distance formula:
\(d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2\)
Side AB:
Let's let A:(a, -3a) be (x₁, y₁) and let's let B:(2a, a) be (x₂, y₂). Substitute this into our formula:
\(d=\sqrt{(2a-a)^2+(a-(-3a))^2\)
Subtract:
\(d=\sqrt{(a)^2+(4a)^2\)
Square:
\(d=\sqrt{a^2+16a^2}\)
Add:
\(d=\sqrt{17a^2}\)
Simplify:
\(d=\sqrt{a^2}\cdot \sqrt{17}\\d=|a|\sqrt{17}\)
So:
\(\overline {AB}=|a|\sqrt{17}\)
Note: We need the absolute value because anything squared will be positive, and if you take the square root of something positive, the result will be positive. The absolute value ensures that the a value will be positive no matter what a is to begin with.
Side BC:
Let's let C:(0, -2a) be (x₁, y₁) and let's let B:(2a, a) be (x₂, y₂).
\(d=\sqrt{(2a-0)^2+(a-(-2a)^2}\)
Subtract:
\(d=\sqrt{(2a)^2+(3a)^2}\)
Square:
\(d=\sqrt{4a^2+9a^2}\)
Add:
\(d=\sqrt{13a^2}\)
Simplify:
\(d=\sqrt{a^2}\cdot \sqrt{13}\\d=|a|\sqrt{13}\)
Therefore:
\(\overline{BC}=|a|\sqrt{13}\)
Side AC:
Let's let A:(a, -3a) be (x₁, y₁) and let's let C:(0, -2a) be (x₂, y₂).
\(d=\sqrt{(0-a)^2+(-2a-(-3a))^2\)
Subtract:
\(d=\sqrt{(-a)^2+(a)^2}\)
Square:
\(d=\sqrt{a^2+a^2}\)
Add:
\(d=\sqrt{2a^2}\)
Simplify:
\(d=|a|\sqrt2\)
Therefore:
\(\overline{AC}=|a|\sqrt2\)
So, our three side lengths are:
\(\overline {AB}=|a|\sqrt{17}\text{, }\overline{BC}=|a|\sqrt{13}\text{, and } \overline{AC}=|a|\sqrt2\)
We can see that the three side lengths are different since they do not equal to same thing.
Therefore, we can deduce that the triangle must be scalene.
And we're done!