the pH of a liquid is measure of how acidic or basic it is. the concentration of hydrogen ions in a liquid is labeled [H+]. use the formula pH=-log[H+] to find the pH level, to the nearest tenth, of a liquid with [H+] about 4.3 x 10^-14
13.4
14.6
-13.4
-14.6
any other answers from the Exponential and Logarithmic functions unit test part one? really struggling, offering 20 points and brainliest
Answers
pH = -log(4.3 x 10^-14)
pH = -(-13.37)
pH = 13.4
Therefore, the pH level of the liquid with [H+] about 4.3 x 10^-14 is 13.4 to the nearest tenth.
Related Questions
Find the equation of the line.
Use exact numbers.

Answers
Answer:
y = - 3x + 7
Step-by-step explanation:
the equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
calculate m using the slope formula
m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₁, y₁ ) = (0, 7) and (x₂, y₂ ) = (2, 1) ← 2 points on the line
m = \(\frac{1-7}{2-0}\) = \(\frac{-6}{2}\) = - 3
the line crosses the y- axis at (0, 7 ) ⇒ c = 7
y = - 3x + 7 ← equation of line
The equation of the line in fully simplified slope-intercept form is y = -2.8x + 7
Writing the equation of the line in slope-intercept form.The linear graph represents the given parameter
For the graph, we have the points
(0, 7) and (25, 0)
A linear equation is represented as
y = mx + c
Where
c = y when x = 0
So, we have
y = mx + 7
Using the point (2.5, 0) on y = mx + 7, we have
m(2.5) + 7 = 0
2.5m + 7 = 0
Evaluate
m = -2.8
So, we have
y = -2.8x + 7
Hence, the equation of the line in fully simplified slope-intercept form is y = -2.8x + 7
Read more about linear relation at
brainly.com/question/30318449
#SPJ1
If $300 is invested at an interest rate of 3.5% per year , find the amount of the investment at the end of 15 years for the following compounding methods
Answers
You need to multiply 3.5 x 15 = 52.5%
Using the present value approach, solve the following:
Tom has $100 in a bank account that pays a guaranteed 5% interest rate each year. How much would Tom have at the end of Year 3?
Answers
Answer:
Step-by-step explanation:
$100x0.5x1=$5
Solve the equation p + 19 = 51
Answers
Answer:
p=32
Step-by-step explanation:
Answer:
Step-by-step explanation:
p+19=51
p=51-19
p=32
David works as a firefighter, receiving an annual salary of $47,730.00. He also owns stocks that pay a dividend. Last year, David earned
$72,175.00 in total income. What was the amount of David's unearned income?
Answers
Answer:
$24,445.00
Step-by-step explanation:
hope it helps
What is the area of the figure in the picture plsssss i neeed it rn tysmm

Answers
Answer:
141
Step-by-step explanation:
a simple random sample of size n is obtained from a population whose size is and whose population proportion with a specified characteristic is describe the sampling distribution of . round the standard deviation of the sampling distribution to three decimal places.
Answers
The sampling distribution of is a binomial distribution with parameters n and p, where n is the sample size and p is the population proportion of the specified characteristic.
The mean of the sampling distribution is np and the standard deviation is \($\sqrt{np(1-p)}$\).
Sampling Distribution of a Simple Random SampleRound the standard deviation to three decimal places, it is \($\sqrt{np(1-p)}$ = $\sqrt{n \cdot p \cdot (1-p)} \approx \sqrt{n \cdot p \cdot 0.99}$\).
The sampling distribution of is a binomial distribution with parameters n and p, where n is the sample size and p is the population proportion of the specified characteristic. The mean of the sampling distribution is np and the standard deviation is \($\sqrt{np(1-p)}$\). This means that for a given sample size n and population proportion p, the standard deviation of the sampling distribution can be calculated by taking the square root of the product of n, p, and (1-p).
Learn more about sampling distribution: https://brainly.com/question/12892403
#SPJ4
HELP HELP HELP
Write the inequality in interval notation and graph.

Answers
The inequality in interval notation is [ -1, ∞ ) from the given equation x ≥ - 3
What is interval notation ?Continuous sets of real numbers can be represented using interval notation by the numbers that bound them. When written, intervals resemble ordered pairs in several ways. They do not, however, intend to indicate any particular location. Instead, they serve as a concise approach to express an inequality or a set of inequalities.A set of real numbers known as an interval in mathematics contains all real numbers falling inside any two of the set's numbers. For instance, the interval containing 0, 1, and all integers in between is the set of values x satisfying 0 x 1.a collection of all real numbers. The interval notation (,) can be used to denote a function's domain as only real values.Given data :
x ≥ - 3
x is greater than or equal to - 3, so - 3 is our smallest value of the interval so it goes on the left. Since there is no upper endpoint (it is ALL values greater than or equal to 3),
we put the infinity symbol on the right side. The boxed end on 3 indicates a closed interval.
In interval notation it is [ -1, ∞ )
Learn more about Interval notation refer to ;
https://brainly.com/question/26025356
#SPJ1
What is the correct answer for this question?

Answers
well, there are 3 feet per 1 yard, so we can say that the field is either
90 feet (30yd) by 48 feet
or
30 yards by 16 yards(48 feet)
90ft * 48ft = 4320 ft²
30yd * 16yd = 480 yd².
Lois and her team used the number of red candies in a
small 9 ounce bag to predict the number of red candies in
a large 33 ounce bag. If Lois counted 12 red candies in the
small bag, Predict the number of red candies in the large bag.
Use proportional reasoning and show algebraic work.
Answers
Using proportional reasoning, we can predict that there will be 40 red candies in the large bag.
Using proportional reasoning, we can set up and solve the following equation to determine the number of red candies in the large bag:
P = Proportion of red candies in the large bag
R = Red candies in the small bag
R × (33/9) = P
12 × (33/9) = P
P = 40 red candies in the large bag
Therefore, using proportional reasoning, we can predict that there will be 40 red candies in the large bag.
To learn more about the proportional relationship visit:
brainly.com/question/12917806.
#SPJ1
The diameter of a circle is the length of its radius.
Answers
Answer:
diameter = 2(radius)
Step-by-step explanation:
not sure what your question is but the diameter of a circle is equal to 2 times the length of its radius
Un camión va cargado con 3796 kg de patatas. En una frutería descarga 6 sacos de 50 kg cada uno. ¿ Cuanto pesa ahora la carga del camión?
Answers
The current weight of the truck is given as follows:
3496 kg.
How to obtain the current weight of the truck?The current weight of the truck is obtained applying the proportions in the context of the problem.
The initial weight of the truck is given as follows:
3796 kg.
The weight removed from the truck is given as follows:
6 x 50 = 300 kg.
Hence the current weight of the truck is given as follows:
3796 - 300 = 3496 kg.
More can be learned about proportions at https://brainly.com/question/24372153
#SPJ1
A scale drawing of a square has a side length of 5 centimeters. The drawing has a scale of 1 cm : 5 ft. Find the actual perimeter and area of the square.
Answers
area =729 feet square and perimeter = 108 feet
Given the side of a square is 9 cm.
The scale is given as :
1 cm = 3 feet Since 1 cm represents 3 feet.
Therefore, 9 cm will represent = 9 x 3 feet= 27 feet
So the side of the square is 27 feet.Therefore, its area = Side x side= 27 x 27 = 729 feet square
And the perimeter = 4 x side= 4 x 27= 108 feet
What is perimeter?In the conventional system of measurement, an inch is a unit of length. Either in or " can be used to represent length in inches. For instance, 16 in or 16" can be used to write 5 inches. In both the British imperial and American customary systems of measurement, the inch serves as a unit of length. It is equivalent to 1/12 of a foot or 1/36 of a yard. a unit of length that is equivalent to 1/12 foot, or 2.54 centimeters, in length. In the conventional system of measurement, an inch is a unit of length. Either in or " can be used to represent length in inches.To learn more about perimeter refer to:
https://brainly.com/question/19819849
#SPJ1
What is the distance between the points M (-21,-7) and N (-5,-7)?
A.28 units
B.16 units
C.26 units
D.14 Units

Answers
This is past due, need help fast

Answers
Answer:
Step-by-step explanation: 1 foot= 12 inches
so length 5.5 inches= 0.458 feet or 5.5/12
width 4 inches= 0.33 feet or 4/12
area 4x5.5= 1.833 feet or 22/12
What is the 21st term of the sequence with a1 = -6 and d = 4?
choices-
74
70
78
-40
Answers
Answer:
\(\boxed {\boxed {\sf 74}}\)
Step-by-step explanation:
The nth term of an arithmetic sequence can be found using the following formula.
\(a_n=a_1+(n-1)d\)
Where n is the term, a₁ is the first term, and d is the common difference.
We want to find the 21st term, we know the first term is -6, and the common difference is 4.
\(n= 21\\a_1= -6 \\d=4\)
Substitute the values into the formula.
\(a_{21}=-6+(21-1)4\)
Solve according to PEMDAS: Parentheses, Exponents, Multiplication, Division, Addition, Subtraction.
Solve inside the parentheses.
\(a_{21}=-6+(20)4\)
Multiply 20 and 4.
\(a_{21}= -6+80 \\\)
Add -6 and 80.
\(a_{21}=74\)
The 21st term of the sequence is 74
Answer:
SoluTion :-Here we will use the formula
\( \tt \: a_n \: = a_1 +( n - 1)\: \times d\)
\( \tt\: a_{21} = −6+(21−1)4\)
By using PEMDAS
\( \tt \: a_{21} = - 6 + (20)4\)
\( \tt \: a_{21} \: = - 6 + 80 = 74\)
\( \huge \tt \bigodot \: \: 74\)
I tried solving this but I accidentally tried figuring out what it would be if it was decreased by 15% instead of increased so now I confused myself an I need help.

Answers
Answer:
120 magazines
Step-by-step explanation:
1.15x = 138
x = 120 magazines before increase
Check:
before increase + increase = sold
120 + 120(.15) = 138
120 + 18 = 138
138 = 138
A printing service charges a set up fee of 14.50 for each order and 8 cent more for each copy the total cost is C in dollars for and order of X copies is given by the following function C(X)=0.08x+14.50. What is the total cost for 40 copies Please help
Answers
Step-by-step explanation:
In order to get the answer first you figure out the type of equation. In this case this is a linear equation, y=mx+b
The B or the y-intercept/base is the first step. Here, it says a fee of 14.50 is needed for each order.
The mx part will be the .08 cents needed for each copy.
y= .08x+14.50 is the equation....
The copies needed is 40 which is the x in the situation:
y=.08(40)+14.50....
.08 * 40 is 3.2 then add the base of 14.50 which is: 17.70 all together
Please help asap, i need this really badly

Answers
In the given diagram, the area of the given rectangle is 36√5. The correct option is the third option 36√5
Calculating the area of a rectangleFrom the question, we are to determine the area of the given rectangle
Using the formula for calculating the area of a rectangle,
Area = l × w
Where l is the length
and w is the width
From the given diagram,
l = 6√10
w = 3√2
Thus,
The area of the rectangle is
Area = 6√10 × 3√2
Area = 6 × 3√10×2
Area = 18√20
Simplify further
Area = 18√4×5
Area = 18√4 × √5
Area = 18×2 × √5
Area = 36 × √5
Area = 36√5
Hence, the area is 36√5
Learn more on Calculating the area of a rectangle here: https://brainly.com/question/25292087
#SPJ1
which one of the following is a solution to |2x-1|>3 A.2, B.-1, C.-2, D.1, E.None of these
Answers
Therefore x>2 or x<-2
—> E
If P(x) = 2x² (x + 3) − (7x − 10); Q(x) = 8(x − 1) (x² + x + 1) − 3x² + 4; R(x) = (2x + 1)² - (2x² + 7)
and S(x) = 2(5x³+6) + x(x - 11) then prove that {P(x) + Q(x)} − {R(x) + S(x)} is a zero polynomial.
Answers
The expression {P(x) + Q(x)} − {R(x) + S(x)} is a zero polynomial.
How to prove that {P(x) + Q(x)} − {R(x) + S(x)} is a zero polynomial.From the question, we have the following polynomial that can be used in our computation:
P(x) = 2x² (x + 3) − (7x − 10)
Q(x) = 8(x − 1) (x² + x + 1) − 3x² + 4
R(x) = (2x + 1)² - (2x² + 7)
S(x) = 2(5x³+6) + x(x - 11)
Add the expressions P(x) and Q(x)
So, we have
P(x) + Q(x) = 2x² (x + 3) − (7x − 10) + 8(x − 1) (x² + x + 1) − 3x² + 4
Evaluate
P(x) + Q(x) = 10x³ + 3x² - 7x + 6
Add the expressions R(x) and S(x)
So, we have
R(x) + S(x) = (2x + 1)² - (2x² + 7) + 2(5x³+6) + x(x - 11)
R(x) + S(x) = 10x³ + 3x² - 7x + 6
So, we have
P(x) + Q(x)} − {R(x) + S(x)} = 10x³ + 3x² - 7x + 6 - (10x³ + 3x² - 7x + 6)
Evaluate
P(x) + Q(x)} − {R(x) + S(x)} = 0
Hence, the expression {P(x) + Q(x)} − {R(x) + S(x)} is a zero polynomial.
Read more about polynomial at
https://brainly.com/question/7693326
#SPJ1
determine the inclination of the following straight line
1. y=x+3 2) 3x-2y = 6
Answers
The inclination of the line represented by the equation y = x + 3 is 1, and the inclination of the line represented by the equation 3x - 2y = 6 is 3/2.
To determine the inclination (or slope) of a straight line, we can examine the coefficients of the variables x and y in the equation of the line.
The inclination represents the ratio of how much y changes with respect to x.
Equation: y = x + 3
In this equation, the coefficient of x is 1, which means that for every increase of 1 in x, y also increases by 1.
This indicates that the inclination of the line is positive, meaning it slopes upwards as x increases.
Since the coefficient of x is 1, the inclination can be expressed as 1/1 or simply 1.
Equation: 3x - 2y = 6
To determine the inclination, we need to rearrange the equation in slope-intercept form (y = mx + b), where m represents the slope.
First, isolate y:
-2y = -3x + 6
Divide the entire equation by -2 to solve for y:
y = (3/2)x - 3
Now we can observe that the coefficient of x is 3/2.
This indicates that for every increase of 1 in x, y increases by 3/2. Therefore, the inclination of this line is positive, indicating an upward slope.
The inclination can be expressed as 3/2.
For similar question on inclination.
https://brainly.com/question/29723347
#SPJ11
What type of transformation is shown below?

Answers
The transformation applied to the triangle is a rotation.
Which type of transformation is applied to the triangle?Here we have a transformation that maps the blue triangle into the orange one.
Remember that neither a translation nor a dilation change the orientation of the figure, so we can discard these two.
If it was a reflection, we should be able to find a line of symmetry between the two figures, and we can't do that here, then we can conclude that the transformation applied was a rotation.
Learn more about transformations at:
https://brainly.com/question/4289712
#SPJ1
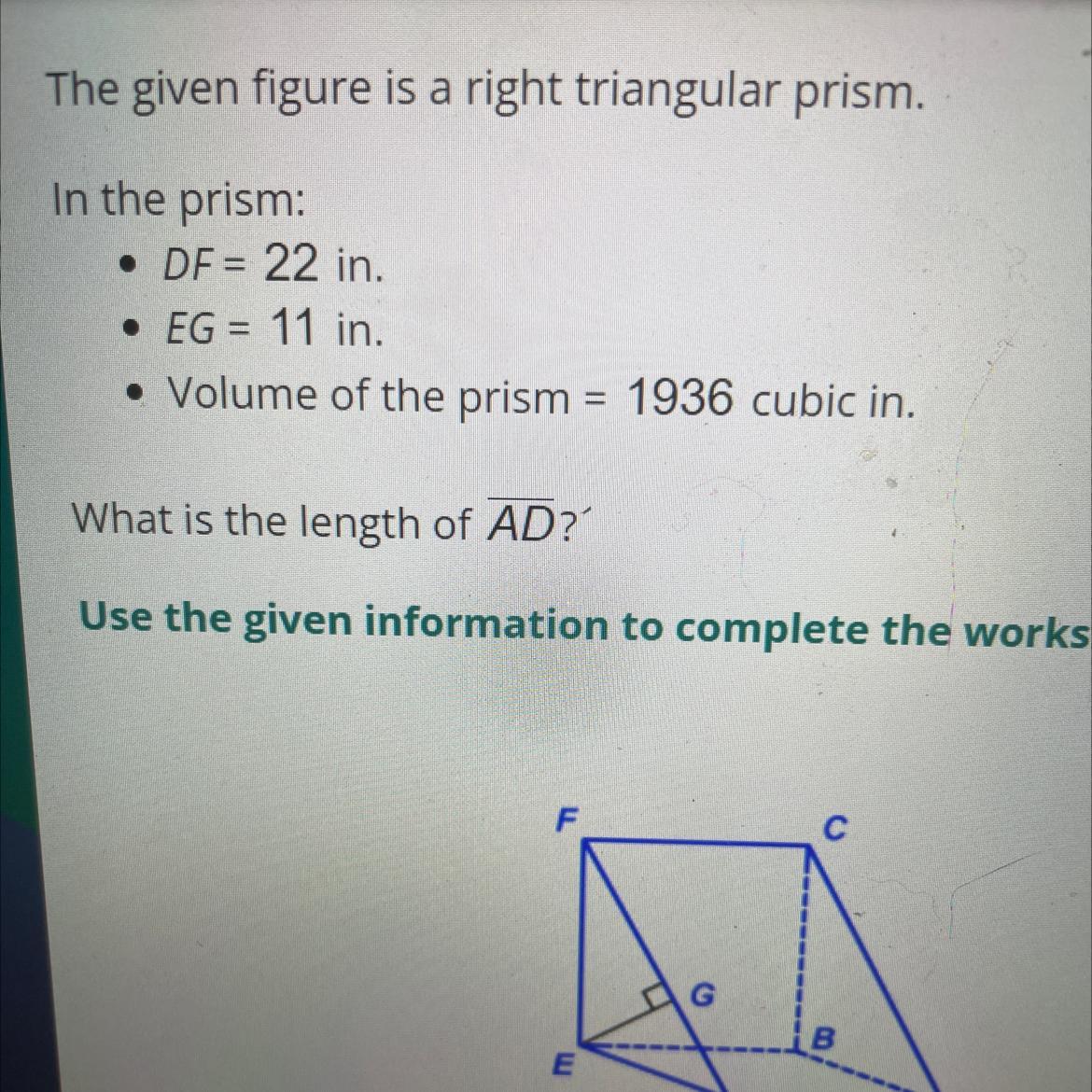
The given figure is a right triangular prism.
In the prism:
• DF = 22 in.
• EG = 11 in.
• Volume of the prism = 1936 cubic in.
What is the length of AD?'
Use the given information to complete the worksheet.
Thank you!
Answers
The length of side AD is,
⇒ AD = 16
We have to given that;
The given figure is a right triangular prism.
In the prism:
• DF = 22 in.
• EG = 11 in.
• Volume of the prism = 1936 cubic in.
Since, Volume of right triangular prism is,
V = 1/2 (bh) x L
Substitute all the values we get;
V = 1/2 (22 × 11) × AD
1936 = 121 × AD
AD = 16
Thus, The length of side AD is, 16
Learn more about the multiplication visit:
https://brainly.com/question/10873737
#SPJ1
It has taken me 32 minutes to drive 48 km. If the total length of my journey is 146 km and I maintain the same speed, estimate how much longer I will be driving for?
Answers
It has taken me 32 minutes to drive 48 km. If the total length of my journey is 146 km and I maintain the same speed, then you can estimate that you will be driving for approximately 65.344 minutes longer to complete the remaining 98 km of your journey.
To estimate how much longer you will be driving for, we can use the concept of proportionality. We know that the time taken to drive 48 km is 32 minutes. Let's use this information to find the time it would take to drive 146 km.
We can set up a proportion to find the time:
(time taken for 48 km) / (48 km) = (time taken for 146 km) / (146 km)
Let's solve for the time taken for 146 km:
(time taken for 146 km) = (time taken for 48 km) * (146 km / 48 km)
Substituting the given values:
(time taken for 146 km) = 32 minutes * (146 km / 48 km)
Calculating the value:
(time taken for 146 km) ≈ 32 minutes * 3.042
(time taken for 146 km) ≈ 97.344 minutes
Therefore, it would take approximately 97.344 minutes to drive 146 km at the same speed.
To estimate how much longer you will be driving for, we can subtract the initial time of 32 minutes from the estimated time of 97.344 minutes:
Additional time = 97.344 minutes - 32 minutes
Additional time ≈ 65.344 minutes
For more such information on: speed
https://brainly.com/question/24739297
#SPJ8
Please explain how to do it too ill give brainliest

Answers
Answer:
x = 90
Step-by-step explanation:
The given diagram shows a circle with intersecting chords, KM and JL.
To find the value of x, we can use the Angles of Intersecting Chords Theorem.
According to the Angles of Intersecting Chords Theorem, if two chords intersect within a circle, the angle formed at the intersection point is equal to half the sum of the measures of the arcs intercepted by the angle and its corresponding vertical angle.
Let the point of intersection of chords KM and JL be point P.
As the chords are straight lines, angle x° forms a linear pair with angle JPM.
Note: We cannot use the Angles of Intersecting Chords Theorem to find the value of x directly, since we have not been given the measures of the arcs KJ and ML. Therefore, we need to use the theorem to find m∠JPM first.
From inspection of the given diagram:
\(m\overset\frown{JM}=30^{\circ}\)\(m\overset\frown{LK}=(2x - 30)^{\circ}\)Using the Angles of Intersecting Chords Theorem, we can calculate the measure of angle JPM (shown in orange on the attached diagram):
\(\begin{aligned}m \angle JPM &=\dfrac{1}{2}\left(m\overset\frown{JM}+m\overset\frown{LK}\right)\\\\&=\dfrac{1}{2}\left(30^{\circ}+(2x-30)^{\circ}\right)\\\\&=\dfrac{1}{2}\left(30^{\circ}+2x^{\circ}-30^{\circ}\right)\\\\&=\dfrac{1}{2}\left(2x^{\circ}\right)\\\\&=x^{\circ}\end{aligned}\)
As angle JPM forms a linear pair with angle x°, the sum of the two angles equals 180°:
\(\begin{aligned}m \angle JPM+x^{\circ}&=180^{\circ}\\\\x^{\circ}+x^{\circ}&=180^{\circ}\\\\2x^{\circ}&=180^{\circ}\\\\\dfrac{2x^{\circ}}{2}&=\dfrac{180^{\circ}}{2}\\\\x^{\circ}&=90^{\circ}\\\\x&=90\end{aligned}\)
Therefore, the value of x is 90, which means that the two chords intersect at right angles.

By converting 3pt/2 from radians to degrees, the answer will be
A. 190°
B.'150°
C. 270°
D. 290°
Answers
Answer:
C
Step-by-step explanation:
To convert radians to degrees
degree = radian × \(\frac{180}{\pi }\) , then
\(\frac{3\pi }{2}\) × \(\frac{180}{\pi }\) ( cancel π on numerator/ denominator )
= \(\frac{3(180)}{2}\)
= \(\frac{540}{2}\)
= 270° → C
Rachel, a 45-year-old female, bought a $120,000, 20-year life insurance policy through her employer. Rachel is paid biweekly.
How much is deducted from each of her paychecks for life insurance?
$63.14
$68.40
$81.05
$87.80
Answers
Answer: the answer is $63.14.
Step-by-step explanation:
I took the test and got it correct.
Answer:
$63.14
Explanation:
I got it right on the test

One year Ron had the lowest ERA (earned-run average, mean number of runs yielded per nine innings pitched)
of any male pitcher at his school, with an ERA of 3.06. Also, Karla had the lowest ERA of any female pitcher at the
school with an ERA of 3.28. For the males, the mean ERA was 5.086 and the standard deviation was 0.998. For
the females, the mean ERA was 4.316 and the standard deviation was 0.558. Find their respective Z-scores.
Which player had the better year relative to their peers, Ron or Karla? (Note: In general, the lower the ERA, the
better the pitcher.)
Answers
Answer:
Ron's ERA has a z-score of -2.03.
Karla's ERA has a z-score of -1.86.
Due to the lower z-score, Ron had a better yean than Karla relative to their peers.
Step-by-step explanation:
Z-score:
In a set with mean \(\mu\) and standard deviation \(\sigma\), the zscore of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.
In this question:
Since the lower the ERA, the better the pitcher, whoever's ERA has the lower z-score had the better year relative to their peers.
Ron
ERA of 3.06, so \(X = 3.06\)
For the males, the mean ERA was 5.086 and the standard deviation was 0.998. This means that \(\mu = 5.086, \sigma = 0.998\)
So
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{3.06 - 5.086}{0.998}\)
\(Z = -2.03\)
Ron's ERA has a z-score of -2.03.
Karla
ERA of 3.28, so \(X = 3.28\)
For the females, the mean ERA was 4.316 and the standard deviation was 0.558. This means that \(\mu = 4.316, \sigma = 0.558\)
So
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{3.28 - 4.316}{0.558}\)
\(Z = -1.86\)
Karla's ERA has a z-score of -1.86.
Which player had the better year relative to their peers, Ron or Karla?
Due to the lower z-score, Ron had a better yean than Karla relative to their peers.
40 decreased by 5%, then increased by 5%
Answers
Answer: 39.9
Step-by-step explanation:
5% of 40 is 2.
40-2=38
1.9 is 5% of 38
38+1.9=39.9
I hope this helps