The painting shown at the right has an area of 440in^2. What is the values of x?
Answers
Answer: x = 9.42 in
Step-by-step explanation:
Area = (4x+9) x
440 = 4x²+9x
4x²+9x-440=0
x = 9+√9²-4x4x(-440)/2x4
x = 9.42 in
Brainliest? <33
Related Questions
Each hot chocolate at Cocoa Land costs $3.25. Chris has $36.99 to buy chocolate and to pay for a music album to listen to while he studies math. The music album costs $9.99. Which inequality can be used to determine how many hot chocolates, h, Chris can buy?
Answers
Answer:
Step-by-step explanation:
A student plans to enroll at the university and plans to continue there until earning a PhD degree (a total time of 9 years). If the tuition for the first 4 years will be $7,200 per year and it increases by 5% per year for the next 5 years, what is the present worth of the tuition cost at an interest rate of 8% per year?
Answers
The present worth of the tuition cost for a student planning to enroll at the university for 9 years, with the first 4 years costing $7,200 per year and a 5% annual increase for the next 5 years, can be calculated at an interest rate of 8% per year. The present worth is $23,455.297.
To calculate the present worth of the tuition cost, we need to consider the time value of money, which accounts for the fact that money in the future is worth less than money in the present. We can use the concept of present value to determine the worth of future cash flows in today's dollars.
For the first 4 years, the tuition cost is constant at $7,200 per year. To find the present value of these cash flows, we can use the formula for the present value of a fixed cash flow series. Applying this formula, we find that the present value of the first 4 years' tuition cost is
\(7,200 + 7,200/(1+0.08) + 7,200/(1+0.08)^2 + 7,200/(1+0.08)^3.\)
For the next 5 years, the tuition cost increases by 5% per year. We can use the concept of future value to calculate the value of these cash flows in the last year of the 9-year period. Applying the formula for future value, we find that the tuition cost in the last year is \($7,200*(1+0.05)^5.\)
Finally, we can sum up the present value of the first 4 years' tuition cost and the future value of the tuition cost in the last year to obtain the total present worth of the tuition cost for the 9-year period.
Learn more about future value here:
https://brainly.com/question/30390035
#SPJ11
school starts in 37 minutes and you live 15 miles from school. what average speed (in miles per hour) would allow you to arrive at school on time?
Answers
The average speed that would allow arriving at school on time is equal to 24.32 miles per hour if school starts in 37 minutes.
The speed that is required to arrive on time can be determined by the following formula;
s = d ÷ t
Here d represents the distance and t represents the time
As the school starts in 37 minutes and the distance is 15 miles, substituting these values in the equation;
s = (15/37) miles per minute
As 1 hour = 60 minutes; multiply by 60 to find the speed in miles per hour as follows;
s = 15/37 × 60
s = 900/37
s = 24.32 miles per hour
Therefore, the average speed that would allow arriving at school on time is calculated to be 24.32 miles per hour.
To learn more about average speed; click here:
https://brainly.com/question/6504879
#SPJ4
Suppose that A and B are two independent events for which P(A) = 0.31 and P(B) = 0.76. What is the probability of (A|B), (B|A), (A and B), and (A or B)?
Answers
The probability of (A and B) is 0.2356, the conditional probability of (A|B) is 0.31, the conditional probability of (B|A) is 0.76, and the probability of (A or B) is 0.8144.
Probability is a measure of the likelihood of a particular event occurring. It is expressed as a number between 0 and 1, where 0 indicates that an event is impossible and 1 indicates that an event is certain to occur.
When events A and B are independent, the probability of them occurring together is the product of their individual probabilities.
P(A and B) = P(A) * P(B) = 0.31 * 0.76 = 0.2356
P(A|B) = P(A and B) / P(B) = 0.2356 / 0.76 = 0.31 (It is same as P(A) because events A and B are independent)
P(B|A) = P(A and B) / P(A) = 0.2356 / 0.31 = 0.76 (It is same as P(B) because events A and B are independent)
P(A or B) = P(A) + P(B) - P(A and B) = 0.31 + 0.76 - 0.2356 = 0.8144
Therefore, the probability of (A and B) is 0.2356, the conditional probability of (A|B) is 0.31, the conditional probability of (B|A) is 0.76, and the probability of (A or B) is 0.8144.
To know more about probability refer to:
brainly.com/question/11234923
#SPJ4
Find the centroid of the region in the first quadrant bounded by the given curves. y = x4, x = y4
Answers
The given bounded region is the set
\(R = \left\{(x,y) ~:~ 0 \le x \le 1 \text{ and } x^4 \le y \le x^{1/4} \right\}\)
assuming the curves are \(y=x^4\) and \(x=y^4\implies y=x^{1/4}\) (since \(x>0\) in the first quadrant).
The coordinates of the centroid are \((\bar x, \bar y)\) where \(\bar x\) and \(\bar y\) are the average values of \(x\) and \(y\), respectively, over the region \(R\). These are given by the ratios
\(\bar x = \dfrac{\displaystyle \iint_R x \, dA}{\displaystyle \iint_R dA} \text{ and } \bar y = \dfrac{\displaystyle \iint_R y\,dA}{\displaystyle \iint_R dA}\)
Compute the area of \(R\).
\(\displaystyle \iint_R dA = \int_0^1 \int_{x^4}^{x^{1/4}} dy \, dx \\\\ ~~~~~~~~ = \int_0^1 \left(x^{1/4} - x^4\right) \, dx \\\\ ~~~~~~~~ = \frac45 - \frac15 = \frac35\)
Integrate \(x\) and \(y\) over \(R\).
\(\displaystyle \iint_R x \, dA = \int_0^1 \int_{x^4}^{x^{1/4}} x \, dy \, dx \\\\ ~~~~~~~~ = \int_0^1 x \left(x^{1/4} - x^4\right) \, dx \\\\ ~~~~~~~~ = \int_0^1 \left(x^{5/4} - x^5\right) \, dx \\\\ ~~~~~~~~ = \frac49 - \frac16 = \frac5{18}\)
\(\displaystyle \iint_R y \, dA = \int_0^1 \int_{x^4}^{x^{1/4}} y \, dy \, dx \\\\ ~~~~~~~~ = \frac12 \int_0^1 \left((x^{1/4})^2 - (x^4)^2\right) \, dx \\\\ ~~~~~~~~ = \frac12 \int_0^1 \left(x^{1/2} - x^8\right) \, dx \\\\ ~~~~~~~~ = \frac12 \left(\frac23 - \frac19\right) = \frac5{18}\)
Then the centroid's coordinates are
\(\bar x = \dfrac{\frac5{18}}{\frac35} = \boxed{\frac{25}{54}} \approx 0.463\)
\(\bar y = \dfrac{\frac5{18}}{\frac35} = \boxed{\frac{25}{54}}\)
help me with this please

Answers
For a given set of side lengths to create a triangle, the sum of any two sides must be greater than the length of the third side.
4 in, 7 in, 12 in = Not Possible
4 + 7 = 11 > 12 = False
4 + 12 = 16 > 7 = True
7 + 12 = 19 > 4 = True
6 mm, 6 mm, 10 mm = Possible
6 + 6 = 12 > 10 = True
6 + 10 = 16 > 6 = True
5 ft, 8 ft, 12 ft = Possible
5 + 8 = 13 > 12 = True
5 + 12 = 17 > 8 = True
8 + 12 = 20 > 5 = True
7 cm, 9 cm, 16 cm = Not Possible
7 + 9 = 16 > 16 = False
7 + 16 = 23 > 9 = True
9 + 16 = 25 > 7 = True
Hope this helps!
Ladies dress blouses are on sale for $18.00. That price is after a 25%
discount. How much money do shoppers save on each blouse they buy?
Answers
Because in your calculator you put 18.00 +25% and it will give you 22.5 and the you subtract 22.5-18 and it will give you 4.5
Answer:
You will get 13$ and 50 Cents
Help me please!!!!!!!!!!!!!!!

Answers
Answer:
y= -x to the power of 2
so the answer is the first one!
Hope this helps!!
XY is an angle bisector of ΔAXN. Find the length of side XN

Answers
The length of XN is 6
What is the angle bisector theorem?According to the triangle angle bisector theorem, any angle in a triangle will divide the opposite side in a ratio equal to the ratio of the sides that contain the angle.A line or ray that divides an angle in a triangle into two equal parts is known as an angle bisector. The two fundamental characteristics of an angle bisector are that each point on it is equally spaced from its sides and that it divides the opposite side of a triangle in proportion to its adjacent sides, which is known as the triangle's angle bisector property.Calculation
By angle bisector theorem
\(\frac{AX}{NX} =\frac{AY}{NY}\)
\(\frac{18}{NX} =\frac{12}{4}\)
\(NX=\frac{18*4}{12} =6\)
Therefore, the length of side NX in the given triangle is 6.
To learn more about the angle bisector theorem, refer to https://brainly.com/question/9742008
#SPJ1
The length of side NX in the given triangle is 6.
What is the angle bisector theorem?According to the triangle angle bisector theorem, any angle in a triangle will divide the opposite side in a ratio equal to the ratio of the sides that contain the angle.A line or ray that divides an angle in a triangle into two equal parts is known as an angle bisector.The two fundamental characteristics of an angle bisector are that each point on it is equally spaced from its sides and that it divides the opposite side of a triangle in proportion to its adjacent sides, which is known as the triangle's angle bisector property.By angle bisector theorem
According to the angle bisector theorem, PQ/PR = QS/RS or a/b = x/y. An angle bisector is a line or ray that divides an angle in a triangle into two equal measures
Therefore, the length of side NX in the given triangle is 6.
To learn more about the angle bisector theorem, refer to:
brainly.com/question/9742008
#SPJ1
a right circular cylinder has a height and radius whose dimensions are each changing. the radius decreases at a rate of 4 ft/min and the height increases at a rate of 2 ft/min. find the rate of change of the surface area of the cylinder the moment when the height is 10 feet an the radius is 8 feet.
Answers
The surface area will decrease at the rate of 50.2 when the height is 10 feet and the radius is 8 feet.
First, let's understand what is right circular cylinder.
A right circular cylinder has parts that are perpendicular to its base and a closed circular surface with two parallel bases on both ends. Another name for it is a right cylinder.
What is the formula for the surface area of right circular cylinder?\(A=2\pi rh+2\pi r^2\).
Also we don't need to remember this we can get this we have 2 parallel bases that is circular so the area of circular part is 2*pi* r^2. For the Perpendicular part if we cut the cylinder then it will make a rectangle.
Volume is always constant whatever we change in radius and height.
dA/dt = 2*pi *(r*dh/dt+h*dr/dt) + 4*pi*r
Given is that dh/dt = 2
dr/dt = -4
we have to change the surface area when r = 8 feet.
h= 10 feet.
dA/dt = 2* pi* (8*2 + 10*(-4)) + 4*pi*8
= 2* pi*(16-40) + 32* pi
= -48*pi+ 32*pi
= -16*pi
= -50.2
The surface area will decrease at the rate of 50.2.
To know more about the right circular cylinder here:
https://brainly.com/question/28448651
#SPJ4
what is the slope (2,6) (-1, -5)
Answers
HOPE THIS HELPS
mark me brainleist pls
From “Making Energy Drinks”, what is the ratio of drink mix to water?
Please help I don’t want some bad grade, please lol.
Answers
Answer:
It should be 3 to 8
Step-by-step explanation:
Answer:
3:8
Step-by-step explanation:
because there are 3 cups of drink mix and 8 cups of water
a city has two water towers. one tower holds 8.4 x 103 gallons of water and the other tower holds 9.5 x 104 gallons of water. what is the combined water capacity of the two towers in scientific notation?
Answers
Total capacity the tower holds 1.034 x 10^5 gallons of water
One tower holds 8.4 x 10^3 gallons of water
Other tower holds 9.5 x 10^4 gallons of water
The combined water capacity of the two towers in scientific notation is,
Total capacity the tower holds = 9.5 x 10^4 + 8.4 x 10^3
= 9.5 x 10^4 + 0.84 x 10^4
= 10.34 x 10^4
= 1.034 x 10^5
To learn more about Capacity click here:
https://brainly.com/question/2740387
#SPJ4
Asap!! step by step pls! After a new firm starts in business, it finds that it’s rate of profit (in hundreds of dollars) after t years of operations is given by P’(t)=3t^2+10t+6. Find the profit in year 4 of the operation.

Answers
Answer:
$ 7800
Step-by-step explanation:
P'(t) = 3t² + 10t + 6
\(P(t) = \int\limits^a_b {P'(t)} \, dt\)
For the 4th year, the limits are [3,4]
\(P(t) = \int\limits^4_3 {3t^2 + 10t + 6} \, dt\\\\= [\frac{3t^3}{3} + \frac{10t^2}{2} + 6t]^{^4}__{3}\\\\\)
\(=[\frac{3(4)^3}{3} + \frac{10(4)^2}{2} + 6(4)]-[\frac{3(3)^3}{3} + \frac{10(3)^2}{2} + 6(3)]\\\\=[\frac{3(64)}{3} + \frac{10(16)}{2} + 24]-[\frac{3(27)}{3} + \frac{10(9)}{2} + 18]\\\\= [64 + 5(16) + 24]-[27+5(9) + 18]\\\\= 168-90\\\\= 78\)
= $ 7800
Identify and explain the processes that are used to show that a function is either a state or path function. Provide an example of each case - state or path - for each process you identify.
Answers
The processes used to determine if a function is a state or path function include integration/differentiation and examining the differential form of the function. Integrating a function with respect to a variable yields a state function, while differentiating a function with respect to a variable yields a path function.
If the differential form of a function involves only state variables, it is a state function. If it involves both state and path variables, it is a path function.
To determine whether a function is a state or path function, we can examine the properties of the function and the variables involved. A state function depends only on the current state of the system and is independent of the path taken to reach that state. In contrast, a path function depends on the path taken to reach a particular state.
One common process used to determine the nature of a function is integration or differentiation. Integrating a function with respect to a variable yields a state function, whereas differentiating a function with respect to a variable yields a path function. For example, integrating the pressure (P) with respect to volume (V) yields a state function called the internal energy (U). On the other hand, differentiating the work (W) with respect to volume (V) yields a path function known as pressure (P).
Another process used is the examination of the differential form of the function. If the differential form of a function involves only state variables, then the function is a state function. For instance, the differential form of the enthalpy (H) involves only state variables (dH = dU + PdV), making it a state function. However, if the differential form involves both state and path variables, the function is a path function. An example is the differential form of heat (Q), which involves both state and path variables (dQ = dU + PdV), indicating that it is a path function.
To know more about state and path functions, refer here:
https://brainly.com/question/13587481#
#SPJ11
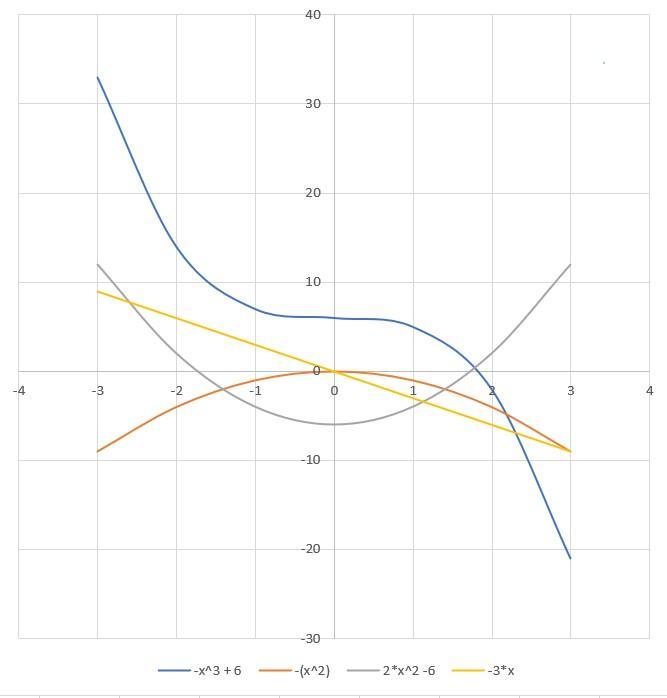
The system of equations graphed below has how many solutions?
A. 0
B. 1
C. Infinitely many
D.2

Answers
Answer:
A
Step-by-step explanation:
Kevin went to a store that offers a 20% discount. He bought a jacket and saved $11.20 after using the coupon. What is the original price of the jacket?
Answers
Answer: $56
Step-by-step explanation:
Let the original price of the jacket be x.
Since Kevin went to a store that offers a 20% discount and bought a jacket and saved $11.20 after using the coupon.
The original price of the jacket goes thus:
20% × x = $11.20
0.2 × x = 11.20
0.2x = 11.20
x = 11.20 / 0.2
x = 56
The original price of the jacket will then be $56
Describe the shape of the distribution.
A. It is symmetric.
B. It is uniform.
C. It is bimodal.
D. It is skewed.

Answers
A local yoga studio charges a sign-fee of $55 plus $10 per class. The second year was studio charges assignment fee of $25 plus $12.50 per class
Answers
Yoga Studio B: y=12.5(10)+25, y=150.
These two numbers are not equal, so Gigi is wrong.
True/False based on the t-test assuming equal variances on the t-testequal worksheet, it is reasonable to assume that the variances are equal?
Answers
Based on the t-test assuming equal variances a. Examining the ratio of the variances, it is reasonable to conclude that the variances are unequal."
It is vital to establish whether or not the variances of the two groups being compared are indeed identical before performing a t-test under the assumption of equal variances. This is significant since the t-calculation statistic depends on the assumption that variances are equal.
One can look at the ratio of the variances between the two groups to evaluate the assumption of equal variances. Typically, it is acceptable to infer that the variances are not equal if the ratio of the variances is more than two or lower than half. One can presume that the variances are equal in the absence of such proof. Since the variances are not equal, it is not logical to infer that they are.
Complete and correct Question:
Based on the t-test assuming equal variances on the T-Test Equal worksheet, is it reasonable to assume that the variances are equal?
a. Examining the ratio of the variances, it is reasonable to conclude that the variances are unequal.
b. Whether the variances are equal or not is not relevant for this situation.
c. Examining the ratio of the variances, it is reasonable to conclude that the variances are equal.
d. It is impossible to determine if the variances are equal given the data we have.
Read more about T-tests on:
https://brainly.com/question/6501190
#SPJ4
Question 7 of 10
Which of the following graphs represents a function that has a negative
leading coefficient? Check all that apply.
Answers
The leading coefficient determines the shape of the graphs, such as how
the characteristic of a function are directed.
Correct response:
The graphs that represents functions that have a negative leading
coefficient are;
Graph A, Graph B, and Graph CMethods by which the correct options are selectedGraph A:
The function in graph A is x³
When the coefficient of x³ is negative, the value of the function rises as x decreases from 0 to -∞, and decreases as x increases from 0 to ∞
Therefore;
The leading coefficient of Graph A is negative.Graph B:
The value of the graph decreases as the magnitude of x increases
therefore, the graph is similar to a quadratic function, such that the leading
coefficient is negative, which inverts the function to give increasing output
with negative value as the value of x increases.
Therefore;
The leading coefficient of the quadratic function in Graph B is negative.Graph C:
The given function in graph C is a linear function having a negative slope,
therefore;
The leading coefficient of x in the function in Graph C is negative.Graph D:
The function of the graph in Graph D, that have y values that increases
exponentially as x increases is a quadratic function.
Given that y increases as the value of x increases, the leading coefficient
(coefficient of x²) is positive.
Therefore;
The graphs that represents functions that have negative leading coefficient are; Graph A, Graph B, and Graph C.Learn more about the factors that depend on leading coefficient of a function here:
https://brainly.com/question/12209936




What is the sum of (4x2+5x – 12) + (7x2 – 6x + 7)?
Answers
Mrs.Ramirez worked with her personal trainer to help develop a nutrition plan. The circle graph shows the recommended percentages for her daily intake. If she will start week 1 eating 1,800 calories, then how many should be from proteins?
Daily food intake:
Fruit: %15
Grains: %15
Protein: %35
Dairy: %10
Vegetables: %25
Answer choices:
A.450
B.350
C.630
D.270
(Please help!)
Answers
Answer:
C
Step-by-step explanation:
\(\frac{35}{100}*1800\\35*18\\\)
630
The portion of protein will be 630.
What is pie chart?The “pie chart” is also known as a “circle chart”, dividing the circular statistical graphic into sectors or sections to illustrate the numerical problems.
Given that a pie chart showing the distribution of the calories of 1800 in different nutrients, we need to find the portion of protein,
Here the portion of protein = 35%
So,
35 / 100 × 1800 = 630
Hence the portion of protein will be 630.
Learn more about pie chart click;
https://brainly.com/question/1109099
#SPJ2
After dieting for 90 days, Ignacio has lost 72 pounds. What number describes his average weight change per day
Answers
Answer:
0.8
Step-by-step explanation:
A tea shop owner mixes 40 cups of water and 70 cups of milk. she calculated and found that she adds 55% of water in tea. is she correct explain with a reason
Answers
Answer:
No
Step-by-step explanation:
Total cups of the mixture = 40 c water + 70 c milk = 110 c
40/110 x 100 = 36.4% water
She is not correct.
One side of a right triangle is 8 cm shorter than the hypotenuse and 7 cm shorter than the third side. Find the lengths of the sides of the triangle.
Answers
Answer:
5 cm
Step-by-step explanation:
Let the shorter side be a, longer side be b, hypotenuse be c
By Pythagorean theorem:
a² + b² = c²
a² + (a + 7)² = (a + 8)²
a² + a² + 14a + 49 = a² + 16a + 64
a² - 2a - 15 = 0
a² + 3a - 5a - 15 = 0
a(a + 3) - 5(a + 3) = 0
(a + 3)(a - 5) = 0
a = -3 or a = 5
Because a is a side length and is a positive number
a = 5
amie has the materials to build a 200 square foot patio. She decided to make it 8 feet wide but needs to figure out the length.
How long should she make her patio so it is 200 square feet?
Answers
She should make her patio 25ft long.
Answer:
By deciding to make the width 8 feet wide, this meant that the figure was to be a rectangule of length X feet by width 8 feet.
A = Lx W
200sq feet = X *8
X = 25 feet.
thus, the patio should be 25 feet long
seven less than eight times a number is -12
Answers
Answer:
19/8
Step-by-step explanation:
8x-7=-12
8x=19
x=19/8
Answer:
8-7n=-12
Step-by-step explanation:
What would the final cost be
for a trick or treat bucket that
originally costs $3.00 if you
have a coupon for 10% off and
you have to pay tax of 7.5%?
Answers
Answer:
4.73
Step-by-step explanation:
10% is 10
so, you have to multiple 3×.10=.30
3-.30=2.70
2.70×.75 because 7.5% is .75
that is 2.0250 and then you have to add 2.0250 with 2.70 that is 4.7250 and if you round it. It is 4.73
Hope that works
Solve the given system by substitution.
n=5m
n=2/3 m-13
Answers
Answer:
n = -15 and m = -3
Step-by-step explanation:
To solve the given system of equation, we will follow the steps below:
n = 5m ----------------------------------------------------------------------------(1)
n = \(\frac{2}{3} m -13\) ---------------------------------------------------------------------(2)
substitute for n= 5m in equation (2)
5m = \(\frac{2}{3} m -13\)
subtract \(\frac{2}{3} m\) from both-side of the equation
5m - \(\frac{2}{3} m\) = \(\frac{2}{3} m\) - \(\frac{2}{3} m -13\)
At the right-hand side of the equation \(\frac{2}{3} m\) will cancel-out leaving us with just -13
5m - \(\frac{2}{3} m\) = -13
\(\frac{15m - 2m }{3}\) = -13
\(\frac{13m}{3}\) = -13
multiply both-side of the equation by 3
3 × \(\frac{13m}{3}\) = -13 × 3
At the left-hand side of the equation 3 will cancel-out 3 leaving is with just 13m
13m = -39
Divide both-side of the equation by 13
13m/13 = -39/13
m = -3
substitute m = -3 in equation (1)
n = 5m
n = 5 (-3)
n = -15
Therefore, n = -15 and m = -3