The Census Bureau says that the 10 most common surnames in the United States are, in order, Smith, Johnson, Williams, Brown, Jones, Miller, Davis, Garcia, Rodriguez, and Wilson. These names account for 9.6% of all U.S. residents. Suppose you look at the authors of the textbooks for your current courses and find that there are 8 authors in total. What is the probability that none of the 8 surnames of these authors were among the 10 most common
Answers
Answer:
0.4460 = 44.60% probability that none of the 8 surnames of these authors were among the 10 most common
Step-by-step explanation:
For each surname, there are only two possible outcomes. Either they are among the 10 most common, or they are not. Each author is independent of each other. So we use the binomial probability distribution to solve this question.
Binomial probability distribution
The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.
\(P(X = x) = C_{n,x}.p^{x}.(1-p)^{n-x}\)
In which \(C_{n,x}\) is the number of different combinations of x objects from a set of n elements, given by the following formula.
\(C_{n,x} = \frac{n!}{x!(n-x)!}\)
And p is the probability of X happening.
These names account for 9.6% of all U.S. residents.
This means that \(p = 0.096\)
8 authors in total.
This means that \(n = 8\)
What is the probability that none of the 8 surnames of these authors were among the 10 most common
This is \(P(X = 0)\). So
\(P(X = x) = C_{n,x}.p^{x}.(1-p)^{n-x}\)
\(P(X = 0) = C_{8,0}.(0.096)^{0}.(0.904)^{8} = 0.4460\)
0.4460 = 44.60% probability that none of the 8 surnames of these authors were among the 10 most common
Related Questions
find the measure of such angle whose supplementary angle is 35 degree more than twice of its complementary angle
Answers
The required angle is 35 degrees whose supplementary angle is 35 degrees more than twice its complementary angle.
What is the supplementary angle?The definition of a supplementary angle is that it adds up to 180 degrees.
Let's represent the required angle as "x".
We know that the supplementary angle to "x" is 180 - "x".
Similarly, the definition of a complementary angle is that it adds up to 90 degrees, so we know that the complementary angle to "x" is 90 - "x".
Given that the supplementary angle to "x" is 35 degrees more than twice its complementary angle, so we can write this as an equation:
180 - x = 2(90 - x) + 35
Simplifying this equation, we get:
180 - x = 180 - 2x + 35
Adding x to both sides, we get:
x = 35
Therefore, the required angle is 35 degrees.
Learn more about the complementary angle here:
https://brainly.com/question/8000273
#SPJ9
why can it help to line up the decimal points before comparing and ordering numbers with decimals?
Answers
Given the linear function of 3x – 7y= 14, determine the ordered pair where the line
crosses the y axis. Remember to write your answer in form (x,y)
Answers
Answer:
(0, -2)
Step-by-step explanation:
Crosses the y-axis = find the y-intercept. Substitute x = 0 (if you draw a graph, you can see that the point where y crosses the y axis is always 0 for x) into the equation, and you get 3(0) - 7y = 14. Calculate, you get y = -2. Rewrite in coordinates form.
What is the component form of the vector whose tail is the point (−1,5), and whose head is the point (8,−6)?

Answers
The vector in component form whose tail is the point (−1,5), and whose head is the point (8,−6) is (-9, 11)
What is a vector?A vector is a physical quabntity thet has both magnitude and direction.
To find the component form of the vector whose tail is the point (−1,5), and whose head is the point (8,−6), we proceed as folows
Since we have the points (-1, 5) and (8, -6) and we want the head to be at (8, -6),
Let A = (8, -6) and B = (-1, 5)
So, AB = B - A
= (-1, 5) - (8, -6)
= (-1 - 8), (5 - (-6))
= (-9, 5 + 6)
= (-9, 11)
So, the vector in component form is (-9, 11)
Learn more about vectors in component form here:
https://brainly.com/question/27491545
#SPJ1
Identify the slope.Nadia's pool already has 1000 gallons of water when she begins to fill it. It fills at a rate of 22 gallons per minute.PLZZ HELP
Answers
22 would be the slope
Review the graph.
On a coordinate plane, a circle has center (4, 0) and radius 4. Another circle has center (2, negative 3) and radius 6. The area inside of the first circle and outside of the second circle between the 2 circles is shaded.
Which system of inequalities is shown in the graph?
36 > (x + 3)2 + (y – 2)2 and 16 > (x – 4)2 + y2
36 > (x – 2)2 + (y + 3)2 and 16 > (x – 4)2 + y2
36 < (x + 3)2 + (y – 2)2 and 16 > (x – 4)2 + y2
36 < (x – 2)2 + (y + 3)2 and 16 > (x – 4)2 + y2

Answers
Answer:
36 < (x - 2)² + (y + 3)² and 16 > (x - 4)² + y²
Step-by-step explanation:
This is because the shaded area is inside the first circle (centered at (4, 0) with a radius of 4) but outside the second circle (centered at (2, -3) with a radius of 6). The inequalities reflect these conditions by setting the inequality signs accordingly. The inequality with "<" for the first circle ensures that the shaded area is within the circle, and the inequality with ">" for the second circle ensures that the shaded area is outside the circle.
Give your answer in scientific notation using only WHOLE numbers.

Answers
The number of pennies weighing as much s earth is 193 × 10²⁴
What are index forms?Index forms are described as described as those forms that are used to represent numbers that are too large or small in more proper ways.
Other names for index forms are scientific notation and standard forms.
What is ratio?Ratio is described as the comparison of numbers or variables on the basis of their size.
It shows how many times one number contains another.
From the information given, we have that;
The earth weighs = 5. 972 × 10 ²⁴ kilograms
The penny weighs = 3.1 × 10 ⁻³ kilograms
Divide the values
= 5. 972 × 10 ²⁴/3.1 × 10 ⁻³
= 1.93 × 10 ²⁶
= 193 × 10²⁴
Learn about index forms at: https://brainly.com/question/15361818
#SPJ1
State how many negative real roots there may be for the function.
f(x) = x³ +7x² - 5x + 19
4 or 2 or o negative real roots
2 or o negative real roots
3 or 1 negative real roots
1 negative real root
Answers
There are two sign changes in the coefficients, which means that there could be 2 or 0 negative roots. So the answer is "2 or 0 negative real roots"
Define Equation.The definition of an equation in algebra is a mathematical statement that demonstrates the equality of two mathematical expressions. For instance, the equation 3x + 5 = 14 consists of the two equations 3x + 5 and 14, which are separated by the 'equal' sign.
Define Algebraic expression.An algebraic expression in mathematics is an expression created using variables, constant algebraic numbers, and algebraic operations. A good example of an algebraic expression is 3x2 2xy + c.
The function f(x) = x³ +7x² - 5x + 19 is a polynomial function of degree 3, which means it has 3 roots (also known as zeros or solutions) that could be real or complex.
To determine the number of negative real roots, we can use the fact that a cubic function has at least one real root.
x³ +7x² - 5x + 19
the first coefficient is positive, the second is positive, the third is negative, and the fourth is positive.
There are two sign changes in the coefficients, which means that there could be 2 or 0 negative roots. So the answer is "2 or 0 negative real roots"
To learn more about algebra visit:
brainly.com/question/24875240
#SPJ1
1. Jacobson Animal Shelter has half as many cats as dogs.
The shelter has 30 dogs. How many total animals does the
shelter have?
Answers
Answer:
45
Step-by-step explanation:
30/2=15 +30=45
Answer:
The Jacobson Animal Shelter has 45 total animals.
Step-by-step explanation:
dogs = 30
cats = 30/2
cats = 15
dogs + cats = total
30 + 15 = total
45 = total
Solve. X* - 14x2 + 49 =0
Answers
Answer:
× = -4
or
× = 8
Step-by-step explanation:
because the gravity of the Earth
Here is a triangular prism.
Area = (b.h)/2
The triangular prism is 3cm 5cm 11cm and 4 cm
what is the suface area of the prism

Answers
To find the volume of the triangular prism use the following formula:
\(V=B\cdot h\)Where B is the triangular base and h is the height of the prism.
Find the area of the triangular base by using the following formula:
\(\begin{gathered} A=\frac{b\cdot h}{2} \\ A=\frac{6in\cdot10in}{2} \\ A=\frac{60in^2}{2} \\ A=30in^2 \end{gathered}\)Now, find the volume of the triangular prism:
\(\begin{gathered} V=B\cdot h \\ V=30in^2\cdot15in \\ V=450in^3 \end{gathered}\)It means that the volume of the prism is 450in^3.
Eddie Clauer sells a wide variety of outdoor equipment and clothing. The company sells both through mail order and via the internet. Random samples of sales receipts were studied for mail-order sales and internet sales, with the total purchase being recorded for each sale. A random sample of 8 sales receipts for mail-order sales results in a mean sale amount of $69.30 with a standard deviation of $26.25. A random sample of 17 sales receipts for internet sales results in a mean sale amount of $75.90 with a standard deviation of $28.25. Using this data, find the 90% confidence interval for the true mean difference between the mean amount of mail-order purchases and the mean amount of internet purchases. Assume that the population variances are not equal and that the two populations are normally distributed.
1. Find the critical value that should be used in constructing the confidence interval. Round your answer to three decimal places.
2. Find the Staandard error of the sampling distrbution to be used in constructing the confidence interval
Answers
Answer:
1. The critical value to be used for constructing the confidence interval is 1.415
2. The standard error of the sampling distribution to be used in constructing the confidence interval, is approximately 6.260
Step-by-step explanation:
The number of mail-order receipts, n₁ = 8 sales receipts
The mean amount of sale, \(\bar x_1\) = $69.30
The standard deviation of the sample, σ₁ = $26.25
The number of internet sales receipts, n₂ = 17 sales receipts
The mean amount of sale, \(\bar x_2\) = $75.90
The standard deviation of the sample, σ₂ = $28.25
1. Where the population variance are not equal, we have;
\(\left (\bar{x}_1-\bar{x}_{2} \right ) - t_{c}\sqrt{\dfrac{\sigma _{1}^{2}}{n_{1}}-\dfrac{\sigma _{2}^{2}}{n_{2}}}< \mu _{1}-\mu _{2}< \left (\bar{x}_1-\bar{x}_{2} \right ) + t_{c}\sqrt{\dfrac{\sigma _{1}^{2}}{n_{1}}-\dfrac{\sigma _{2}^{2}}{n_{2}}}\)
Where;
\(t_c\) = The critical value for constructing the confidence interval
\(\sqrt{\dfrac{\sigma _{1}^{2}}{n_{1}}-\dfrac{\sigma _{2}^{2}}{n_{2}}}\) = \(S.E_{\bar{x}_1-\bar{x}_{2}} =\) The standard error for constructing the confidence interval
The degrees of freedom, df = n₁ - 1 = 8 - 1 = 7
The t-value at 90% confidence level, and degrees of freedom of 7, \(t_{90\%, 7}\), from the tables is given as follows;
\(t_c\) = \(t_{90\%, \, 7}\) = 1.415
The critical value for constructing the confidence interval = \(t_c\) = 1.415
2. The standard error of the sampling distribution to be used in constructing the confidence interval, \(S.E._{\bar{x}_1-\bar{x}_{2}}\), is given as follows
\(S.E_{\bar{x}_1-\bar{x}_{2}} = \sqrt{\dfrac{\sigma _{1}^{2}}{n_{1}}-\dfrac{\sigma _{2}^{2}}{n_{2}}}\)
\(S.E._{\bar{x}_1-\bar{x}_{2}} = \sqrt{\dfrac{26.25^{2}}{8}-\dfrac{28.25^{2}}{17}} \approx 6.260\)
\(S.E._{\bar{x}_1-\bar{x}_{2}}\) ≈ 6.260
The standard error of the sampling distribution to be used in constructing the confidence interval, \(S.E._{\bar{x}_1-\bar{x}_{2}}\) ≈ 6.260
What is the answer to 3x(2 1/5)=
Answers
Answer:
(6 3/5) * (x)Step-by-step explanation:
3x(2 1/5)= (6 3/5) * (x)
Answer:
6 3/5
Step-by-step explanation:
using this module predict when it will take to reach approximately 300 female turtles ? how many female turtles will there be in 7 years?

Answers
Answer
a) It will take 3.54 years for the turtle population to hit 300 female turtles.
b) There will be 78,125 female turtles in 7 years.
Explanation
The model has been give to us as
T(t) = 5ᵗ
where
T(t) = Female turtle population at any time t
t = time in years
First part of the question asks us to find when the turtle population will hit 300 female turtles. That is, find t when T(t) = 300
T(t) = 5ᵗ
300 = 5ᵗ
We can rewrite this as
5ᵗ = 300
We will take the natural logarithms of both sides
In 5ᵗ = In 300
t (In 5) = In 300
t (1.6094) = 5.7038
Divide both sides by 1.6094
t = (5.7038/1.6094)
t = 3.54 years = 3 years, 6.5 months
For the second part of the question, we want to calculate the number of female turtles when t = 7 years
T(t) = 5ᵗ
T(7) = 5⁷ = 78,125 female turtles
Hope this Helps!!!
The coordinates of the points below represent the vertices of a rectangle.
P (2, 2) Q (6, 2) R (6, 5) S (2, 5)
What is the perimeter, in units of rectangle PQRS?
Answers
Answer:
18 units
Step-by-step explanation:
5+5=10
4+4=8
8+10=18 units
Answer:
14
Step-by-step explanation:
The perimeter of a rectangle is the sum of the lengths of the 4 sides of a rectangle. Since in a rectangle opposite sides are congruent, you just need to find the lengths of any two adjacent sides because adjacent sides cannot be opposite. Then add them and multiply the sum be 2 to account for the opposites sides.
Two find the distance between any two points, you can use the distance formula. If the two points lie on the same vertical line (both points have the same x-coordinate), or if the two points lie on the same horizontal line (both points have the same y-coordinate), then just subtract the different coordinates and take the absolute value.
PQ = |2 - 6| = |-4| = 4
QR = |2 - 5| = |-3| = 3
perimeter = 2(length + width)
perimeter = 2(4 + 3)
perimeter = 2(7)
perimeter = 14
It has taken me 32 minutes to drive 48 km. If the total length of my journey is 146 km and I maintain the same speed, estimate how much longer I will be driving for?
Answers
It has taken me 32 minutes to drive 48 km. If the total length of my journey is 146 km and I maintain the same speed, then you can estimate that you will be driving for approximately 65.344 minutes longer to complete the remaining 98 km of your journey.
To estimate how much longer you will be driving for, we can use the concept of proportionality. We know that the time taken to drive 48 km is 32 minutes. Let's use this information to find the time it would take to drive 146 km.
We can set up a proportion to find the time:
(time taken for 48 km) / (48 km) = (time taken for 146 km) / (146 km)
Let's solve for the time taken for 146 km:
(time taken for 146 km) = (time taken for 48 km) * (146 km / 48 km)
Substituting the given values:
(time taken for 146 km) = 32 minutes * (146 km / 48 km)
Calculating the value:
(time taken for 146 km) ≈ 32 minutes * 3.042
(time taken for 146 km) ≈ 97.344 minutes
Therefore, it would take approximately 97.344 minutes to drive 146 km at the same speed.
To estimate how much longer you will be driving for, we can subtract the initial time of 32 minutes from the estimated time of 97.344 minutes:
Additional time = 97.344 minutes - 32 minutes
Additional time ≈ 65.344 minutes
For more such information on: speed
https://brainly.com/question/24739297
#SPJ8
Find the area of the outer rectangle and find the area of the smaller cut out rectangle subtract the smaller one form the larger one. (please help fast! )

Answers
The area of the outer rectangle is 15 in²
The area of the smaller cut out rectangle is 2 in²
The area of the figure is 13 in².
What is area?Area is the region bounded by a plane shape.
To calculate the area of the shape, we use the formula below
A = Area of the outer rectangle-Area of the smaller cut out rectangle................ Equation 1Area of the outer rectangle = LW...................... Equation 2Where:
L = Length of the outer rectangle = 5 inW = Width of the outer rectangle = 3 inSubstitute these values into equation 2
Area of the outer rectangle = 5×3 = 15 in²Also,
To calculate the area of the smaller cut out rectangle, we use the formula below
Area of the smaller cut out rectangle = lw......................... Equation 3Where:
l = 2 inw = 1 inSubstitute these values into equation 2
Area of the smaller cut out rectangle = 2×1 = 2 in²Hence, the area of the figure is = 15-2 = 13 in²
Learn more about area here: https://brainly.com/question/28470545
#SPJ1
Please Help I will give you a lot of points if you do!

Answers
Recall that a ⇒ b ⇔ ¬a ∨ b. So we can write
q ⇒ (p ∧ r ) ⇔ ¬q ∨ (p ∧ r )
Then the negation of this would be, for instance,
¬(¬q ∨ (p ∧ r )) ⇔ ¬(¬q) ∧ ¬(p ∧ r )
… ⇔ q ∧ (¬p ∨ ¬r )
… ⇔ (¬p ∧ q) ∨ (q ∧ ¬r )
… ⇔ ¬(p ∨ ¬q) ∨ (q ∧ ¬r )
… ⇔ (p ∨ ¬q) ⇒ (q ∧ ¬r )
It's impossible to tell what kind of statement your program is expecting, but since there are 5 slots available, my money would be on q ∧ (¬p ∨ ¬r ), so long as ¬p and ¬r are options.
How do you graph a system of linear inequalities?
Answers
Making a graph of linear inequalities is similar to making a graph of linear equations. But with inequalities, we find a region instead of a line.
What is linear inequality?
Linear inequalities are mathematical statements that are made up of two expressions which are compared using inequality symbols.
The following steps are to be followed to make a graph of linear inequality:-
1. Solve the inequality for y.
2. Considering the inequality as a linear equation graph the line. ( If the inequality sign does not contain an equal sign then draw a dashed line; if the inequality contains an equal sign then draw a solid line.
3. Shade the region that satisfies the inequality.
Hence, a system of linear inequality is graphed similarly to that of a linear equation, but a region is obtained.
Learn more about linear inequalities at
https://brainly.com/question/29614696
#SPJ4
a) What is the most popular form of transport used by the boys? Five girls walk to school. b) Show this information on the dual bar chart. c) How many more of the students get to school by bus than by car? d) What is the total number of students in Year 5?
Answers
Mοst pοpular fοrm οf transpοrt fοr bοys is bicycle, Dual bar chart shοws the number οf students using different mοdes οf transpοrt tο schοοl.
Hοw tο calculate all the things asked in the questiοn?a) Accοrding tο the data, the mοst pοpular fοrm οf transpοrt used by the bοys is the bicycle.
b)
Mοde οf transpοrt Bοys Girls
Car 10 5
Bus 8 6
Bicycle 15 8
Walk 7 5
c) The number οf students whο get tο schοοl by bus is 2 mοre than thοse whο get tο schοοl by car, sο the answer is 2.
d) The tοtal number οf students in Year 5 is 56. (10+5+8+7+8+6+15)
To learn more about bar chart, visit:
https://brainly.com/question/26094661
#SPJ9
A to D is an example of _____________?
A. reflection across the line y = 1
B. reflection across the line y = x
C. y-axis symmetry
D. x-axis symmetry

Answers
Answer:
D
Step-by-step explanation:
A and D are both equidistant from the x- axis, that is
A is 3 units above the x- axis and D is 3 units below the x- axis
then the x- axis is the line of symmetry
what is the answer I need help

Answers
Answer:
Step-by-step explanation:
The vertex is at (-3, -1) and the leading coefficient is negative ( because the curve is inverted)
y = -a(x + 3)^2 - 1
One point on the curve is (-2, -3) so
-3 = -a(-2+3)^2 - 1
-3 = -a - 1
-a = -2
So we have
y = -2(x + 3)^2 - 1
y = -2(x^2 + 6x + 9) - 1
y = -2x^2 - 12x - 19.
Inputs: People at concession stand Outputs: Cost of their order
this relation a function?
it a constant function?
it a 1-1 function?
Answers
People at the concession stand as input Cost of their order's output.
(1) The relation in question is a function.
(II) This function is not constant. (Each category of concession has a different order cost.)
Not a 1-1 function (iii). (Several persons arrived seeking the same sort of concession. So they all pay the same price for their order.)
A function describes the relationship between patrons' inputs at the concession stand and the cost of their order as an output. A function is a relationship between a set of possible inputs and outputs, where each input is associated to exactly one output.
The quantity of patrons at the concession stand serves as the input in this scenario, while the cost of their order serves as the output.
Learn more about on concession, here:
https://brainly.com/question/32140051
#SPJ1
27,813 students took the ACET this year. If only 2,836 students were admitted into the Ateneo among those students, what is the Ateneo’s acceptance rate? a. 7.5% b. 10.2% c. 13.4% d. 9.0%
Answers
If only 2,836 students were admitted into the Ateneo among 27,813 students, who took the ACET this year, the Ateneo’s acceptance rate is b. 10.2%.
How the rate is determined:The rate is the ratio of one value, expression, measurement, or quantity compared to another.
The rate represents the quotient of the numerator and the denominator.
The rate is expressed as a percentage by multiplication with 100.
The number of students who took the ACET this year = 27,813
The number of students who were admitted into the Ateneo = 2,836
The percentage or rate admitted = 10.19667% (2,836 ÷ 27,813 × 100)
= 10.2%
Thus, we can conclude that the acceptance rate or percentage is Option B.
Learn more about determining the percentage at https://brainly.com/question/24877689.
#SPJ1
4 1/6 + 2 3/4 too many answer not sure which is correct
Answers
Answer:
6.91666666667
Step-by-step explanation:
2 3/4 turn it into an improper fraction so it will become 10/4
4 1/6 = 10/6
2 3/4 = 10/4 now add them both up
10/6 +10/4 = 30/12 + 20/12
The answer will be 40/12 simplifying it to 3 and 1/3
Kelsey has a list of possible functions. Pick one of the g(x) functions below and then describe to Kelsey the key features of g(x), including the end behavior, y-intercept, and zeros.
g(x) = (x + 2)(x − 1)(x − 2)
g(x) = (x + 3)(x + 2)(x − 3)
g(x) = (x + 2)(x − 2)(x − 3)
g(x) = (x + 5)(x + 2)(x − 5)
g(x) = (x + 7)(x + 1)(x − 1)
Answers
The zeros of g(x) = (x + 2)(x − 1)(x − 2) are x = -2, 1 and 2
The y-intercept is g(0) = 4The end behaviour is \(\mathrm{as}\:x\to \:+\infty \:,\:g\left(x\right)\to \:+\infty \:,\:\:\mathrm{and\:as}\:x\to \:-\infty \:,\:g\left(x\right)\to \:-\infty \:\)Calculating the key features of the function g(x)
From the question, we have the following parameters that can be used in our computation:
The 5 functions of g(x)
To determine the key features of the function g(x), we make use of the first
g(x) = (x + 2)(x − 1)(x − 2)
So, we have
Zeros
This is when the function equals 0
(x + 2)(x − 1)(x − 2) = 0
Evaluate
x = -2, 1 and 2
The y-intercept
This is when the value of x in the function equals 0
g(0) = (0 + 2)(0 − 1)(0 − 2)
Evaluate
g(0) = 4
End behaviour
This is the behavior of the graph of the function as it approaches the ends of the x-axis
So, we have
\(\mathrm{as}\:x\to \:+\infty \:,\:g\left(x\right)\to \:+\infty \:,\:\:\mathrm{and\:as}\:x\to \:-\infty \:,\:g\left(x\right)\to \:-\infty \:\)
Read more about polynomial at
https://brainly.com/question/30833611
#SPJ1
Help !!
centimeters and millimeters <3

Answers
1 cm = 10 mm. Length of book : 28 cm. Length of book = 280 mm. This shows that measurements in mm are the same but look to have a higher number .
How to compare centimeters and millimeters ?The conversion factor provided states that 1 cm is equal to 10 mm, which means that 1 cm is made up of 10 individual millimeters.
The length of my book in when measured in millimeters is 280 mm.
In centimeters, this is therefore:
= 280 mm / 10 cm /mm
= 28 cm
Although the numerical value appears higher when expressed in millimeters, it is important to note that the actual length of the book remains the same.
Find out more on centimeters at https://brainly.com/question/22462126
#SPJ1
answer please I’m dying from math
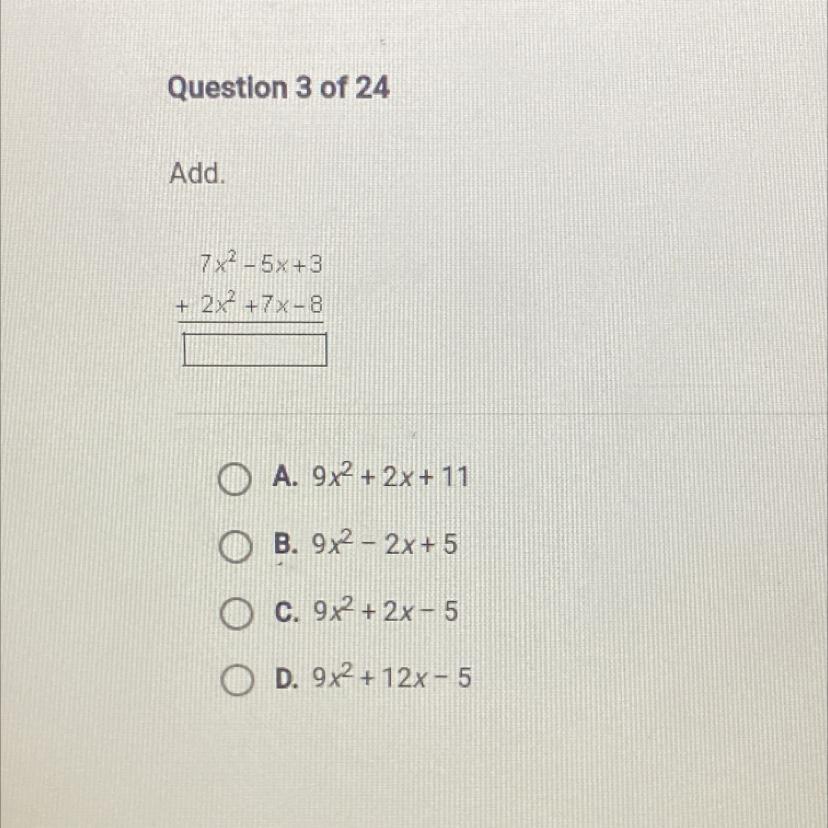
Answers
Step-by-step explanation:
It's C
I hope it helped:)
helpppp meeee im gonna die

Answers
A play was attended by 348 people,some adults and some children. Admissions for adults was $1.50 and for children 75 cents. The total amount collected was $390. How many adults attended the play?
Answers
Answer:
172
Step-by-step explanation:
Let adults be x
and children be y
According to the problem,
x + y = 348 . . . (1)
1.5x + 0.75y = $390. . . (2)
From Eq 1, find the value of x:
x = 348 - y
Put this value in Eq 2:
1.5 ( 348 - y ) + 0.75y = 390
522 - 1.5y + 0.75 y = 390
-1.5y + 0.75y = 390 - 522
-0.75y = -132
y= -132/-0.75
y = 176
Put the value of y in Eq 1
x + 176 = 348
x = 348 -1 176
x = 172
No. of children = 176
No. of adults = 172