the capacity of a cylinder varies jointly with its height and the square of its radius. if a cylinder with a radius of 3 3 centimeters and a height of 6 6 centimeters has a capacity of 169.56 169.56 cubic centimeters, what will the capacity be of a cylinder with radius 2 2 centimeters and height 7 7 centimeters?

Answer:

these .56 because they are bigger

Step-by-step explanation:

hope this helps

Answer:

Step-by-step explanation:

6085 rounded to the nearest hundred is 6100

51,672 rounded to the nearest ten thousand is 50,000

The amount of the worker monthly tax will equals to K4,850.

An income tax refers to the tax imposed on individuals or entities in respect of the income earned by them. It is computed as the product of a tax rate times the taxable income. They may vary by type or characteristics of the taxpayer and the type of income

To calculate the worker's monthly tax, we need to determine the amount of salary that falls into each tax bracket and then calculate the tax owed for each bracket:

The worker's monthly tax is then computed as:

=  K1,500 + K3,000 + K350

= K4,850.

Read more about monthly tax

brainly.com/question/30689717

#SPJ1

Answer:

area of shaded portion=56 - 1/2 ×3×4=56-6= 50 yd^2

He stands 6 feet tall, and his shadow extends 9 feet. if the building's shadow extends for 322.5 feet.

Now 6/9 = X/322.5

9X = 1935

X = 215ft

The Height of the building = 215ft

Consider an object AB of height h such that its shadow of length s is formed and angle of elevation is θ.

The object AB depicts a right triangle in which ∠ABO = 90o.

Now we know that in trigonometry, the tangent ratio for an angle is equal to the opposite side to that angle divided by its adjacent side.

So, for the angle of elevation θ, we have

=> tan θ = AB/OB

Putting AB = h and OB = s, we get

=> tan θ = h/s

=> s/h = 1/tan θ

=> s = h/tan θ

Learn more about Shadow here:

https://brainly.com/question/15021258

#SPJ4

Answer:

the mean is 1.875

Step-by-step explanation:

1+1+1+2+2+2+3+3=15

15/8 = 1.875

mean Is sum of all numbers divided by total amount of numbers

Answer:

36

Step-by-step explanation:

12 times 3 = 36

Answer:

1/120

Explanation:

The number of possible toppings = 6

Each topping can only be used once.

We want to find the probability that you get 3 toppings.

Therefore, the probability that you get 3 toppings is:

The probability that you get 3 toppings is 1/120.

The 2 sides of the equation are not equal; hence, I cannot prove them to be true.

Answer:

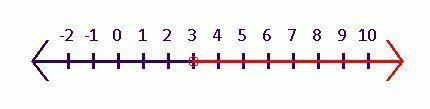
Please refer to the attached image in the answer area.

Step-by-step explanation:

The given inequality is:

\(0.3(x -4) > -0.3\)

To find:

The graph of inequality on the number line.

Solution:

First of all, let us simplify the inequality.

\(0.3(x -4) > -0.3\)

Dividing it with 0.3 on the both sides:

\(x -4 > -1\\\text{Adding 4 on both sides}\\\Rightarrow x > 3\)

i.e. all the values x > 3 will be our solution. There is no equal sign in the inequality so 3 will not be included in the solution.

Please refer to the attached image for the solution graph of the given inequality.

3 has an empty circle drawn over it which signifies that 3 is not included in the solution set.

Red line shows that all the values greater than 3 are included in the solution.

First, let's calculate the z-score for the value 2.025 liters using the formula:

z = (x - μ) / σ

Where:

x = the value we want to calculate the probability for (2.025 liters)

μ = the mean of the process (2.050 liters)

σ = the standard deviation of the process (0.020 liters)

Plugging in the values:

z = (2.025 - 2.050) / 0.020

Simplifying:

z= -0.025 / 0.020

z = -1.25

Now, we can look up the probability corresponding to a z-score of -1.25 in the standard normal distribution table or use a calculator.

Using a standard normal distribution table, the probability is approximately 0.1056. This means that the probability of randomly selecting an output from the process that is less than 2.025 liters is approximately 0.1056 or 10.56%.

Alternatively, you can use a calculator or statistical software to find the probability directly by looking up the cumulative distribution function (CDF) of the standard normal distribution at -1.25. The result will also be approximately 0.1056 or 10.56%.

Learn more about Cumulative Distribution Function here:

https://brainly.com/question/30402457

#SPJ11

Answer: 9 hours

Step-by-step explanation: divide 450 total miles by how many miles you drive per hour (50).

The correct option regarding the 95% confidence interval for this problem is given as follows:

A. The confidence interval from the first simulation is (0.187, 0.237), and the confidence interval from the second simulation is (0.209, 0.261). For the first trial, we are 95% confident the true proportion of wins with the new game strategy is between 0.187 and 0.237. For the second trial, we are 95% confident the true proportion of wins with the new game strategy is between 0.209 and 0.261.

A confidence interval of proportions has the bounds given by the rule presented as follows:

\(\pi \pm z\sqrt{\frac{\pi(1-\pi)}{n}}\)

In which the variables used to calculated these bounds are listed as follows:

The confidence level is of 95%, hence the critical value z is the value of Z that has a p-value of \(\frac{1+0.95}{2} = 0.975\), so the critical value is z = 1.96.

The parameters for the first simulation are given as follows:

\(n = 1000, \pi = \frac{212}{1000} = 0.212\)

Hence the lower bound of the interval is of:

\(0.212 - 1.96\sqrt{\frac{0.212(0.788)}{1000}} = 0.187\)

The upper bound is of:

\(0.212 + 1.96\sqrt{\frac{0.212(0.788)}{1000}} = 0.237\)

For the second simulation, the parameters are given as follows:

\(n = 1000, \pi = \frac{235}{1000} = 0.235\)

Hence the lower bound of the interval is of:

\(0.235 - 1.96\sqrt{\frac{0.235(0.765)}{1000}} = 0.209\)

The upper bound is of:

\(0.235 + 1.96\sqrt{\frac{0.235(0.765)}{1000}} = 0.261\)

This means that option A is the correct option.

More can be learned about the z-distribution at https://brainly.com/question/25890103

#SPJ1

The solution for definite integral ∫2^x dx from [−1,1] is

 3/ 2 ln2

Definite Integral:

A definite integral is defined as the exact limit and summation examined in the previous section to find the net area between the function and the x axis. Also note that the definite integral notation is very similar to the indefinite integral notation. The reason for this will become clear in due course.

There are a few terms that should be left out along the way. The number "a" below the integral sign is called the lower integral limit, and the number "b" above the integral sign is called the upper integral limit. Even if the intervals a and b are specified, the lower bound is not necessarily less than the upper bound. Collectively, a and b are often referred to as the interval of integration.

Given a function :

f(x) that is continuous function on the interval [a , b]

we divide the interval into n subintervals of equal breadth, Δx, and from each interval choose a point, x i. Then the definite integral of f(x) from a to b is

\(\int\limits^b_a f(x) \, dx = \lim_{n \to \infty} f(xi)\) Δx.

According to the question:

let's start by differentiating 2ˣ.

   y = 2ˣ

⇒ ln y = x ln 2

⇒ 1/y dy/dx = ln2

⇒ d /dx = y ln 2 = 2ˣ ln2

So remembering integration is the reverse of differentiating.

  y = 2ˣ

⇒ dy/dx = 2ˣ ln 2

⇒ ∫2ˣ dx = 2ˣ / ln 2 + C

2ˣ > 0, ∀ x ∈ R, there is no negative area so we can

Insert the limits and evaluate immediately.

= 1/ ln2 [2x]\(\left \{ {{y= -1} \atop {x=1}} \right.\)

= 1/ln 2  (2¹ - 2⁻¹)

= 3/ 2ln 2

Learn more about Definite Integral:

https://brainly.com/question/29685762

#SPJ4

There is a difference in the average salary among the three racial groups being studied.

A study was conducted comparing the average salary for Blacks, Whites, and Hispanics, using random samples of 10 people in each racial group. The standard deviations of the groups were quite different.

To determine if there is a significant difference in salaries among these racial groups, the following steps can be taken:

1. Calculate the mean salary for each racial group (Blacks, Whites, and Hispanics) using the data from the random samples.

2. Calculate the variance and standard deviation for each group's salary to understand the spread of data within each group.

3. Perform an analysis of variance (ANOVA) test, which helps in comparing the means of multiple groups (in this case, the three racial groups). This test will indicate whether there is a significant difference in the mean salaries of the groups.

If the results of the ANOVA test show a significant difference, it means there is a difference in the average salary among the three racial groups being studied.

Learn more about average here,

https://brainly.com/question/29509552

#SPJ11

Answer:

a)  y = 2 x - 1

b)  y = - x + 7

c)  y = 7 x + 2

Step-by-step explanation:

Use a pair of (x1, y1) and (x2, y2) points to find the equation of the line for each tables:

a) (5, 9) and (10, 19)

slope: (y2 - y1) / (x2 - x1) = (19 - 9) / (10 - 5) = 10 / 5 = 2

Then  y = 2 x + b

solve for b in:  9 = 2 (5) + b

b = 9 - 10 = -1

Then   y = 2 x - 1

b) (2, 5) and (5, 2)

slope: (y2 - y1) / (x2 - x1) = (2 - 5) / (5 - 2) = -3 / 3 = -1

Then  y = - x + b

solve for b in:  5 = - (2) + b

b = 5 + 2 = 7

Then   y = - x + 7

c) (3, 23) and (6, 44)

slope: (y2 - y1) / (x2 - x1) = (44 - 23) / (6 - 3) = 21 / 3 = 7

Then  y = 7 x + b

solve for b in:  23 = 7 (3) + b

b = 23 - 21 = 2

Then   y = 7 x + 2

Answer: C. 25.6

Step-by-step explanation:

Answer:

- 3x < 2

x < - 1

Explanation and Check part below

Explanation and Check part belowHope this helps :)

Step-by-step explanation:

1. To solve, first isolate the variable by doing the inverse operation which is in this case dividing by - 3 on both sides of the equation.

- 3x < 2

- 3 -3

x < - 1

2. Graph it if needed with an open dot because - 1 is not part of the set of solutions.

<--------○

<----l----l-----l----->

-2 -1 0

Check:

1. Substitute x with any number less than - 1 and solve.

- 3 (- 2) < 2

-6 < 2

True statement.

Hello!

surface area

= 2(6*2) + 2(4*2) + 4*6

= 2*12 + 2*8 + 24

= 24 + 16 + 24

= 64 square inches

The mean, median, and sample variance of the given dataset are:Mean = 23.17Median = 21Sample variance = 173.5592

(a) Mean The mean (or average) of a dataset is calculated by summing up all the values and dividing by the total number of values.

The formula for calculating the mean is: `mean = (sum of values) / (total number of values)`For the given dataset, we have:20, 50, 22, 14, 23, 10

Sum of values = 20 + 50 + 22 + 14 + 23 + 10 = 139

Total number of values = 6Therefore, the mean is given by: `mean = 139 / 6 = 23.17`Answer: 23.17 (rounded to two decimal places)

(b) Median To find the median, we need to arrange the dataset in increasing order:10, 14, 20, 22, 23, 50The median is the middle value of the dataset. If there are an odd number of values, the median is the middle value. If there are an even number of values, the median is the average of the two middle values. Here, we have 6 values, so the median is the average of the two middle values: `median = (20 + 22) / 2 = 21` Answer: 21(e)

Sample variance s²The sample variance is calculated by finding the mean of the squared differences between each value and the mean of the dataset.

The formula for calculating the sample variance is: `s² = ∑(x - mean)² / (n - 1)`where `∑` means "sum of", `x` is each individual value in the dataset, `mean` is the mean of the dataset, and `n` is the total number of values.For the given dataset, we have already calculated the mean to be 23.17.

Now, we need to calculate the squared differences between each value and the mean:

20 - 23.17 = -3.1722 - 23.17

= -1.170 - 23.17

= -13 - 23.17

= -9.1723 - 23.17

= -0.1710 - 23.17

= -13.17

The sum of the squared differences is given by:

∑(x - mean)² = (-3.17)² + (-1.17)² + (-13.17)² + (-9.17)² + (-0.17)² + (-13.17)²

= 867.7959

Therefore, the sample variance is given by: `s² = 867.7959 / (6 - 1) = 173.5592`Answer: 173.5592 (rounded to four decimal places)

The mean, median, and sample variance of the given dataset are:Mean = 23.17Median = 21Sample variance = 173.5592

To know more about sample variance visit:

brainly.com/question/30112124

#SPJ11

Answer:

decimeters to meters

Step-by-step explanation:

There are 10 decimeters in a meter.

Answer:

decimeters to meters

hectograms to kilograms

Step-by-step explanation:

Answer:

-17

Step-by-step explanation:

-12 + (-5) is basically subtracting 5 from -12.

Or you can think of it as adding 5 to 12. Then make the answer negative.

Hope I helped :)

Please consider Brainliest :)

Answer:

the answer is irrational

The total distance travelled by the particle is 24 units.

What is displacement?

Displacement is defined as a change in an object's position. An object's displacement is defined as how far it has moved from its starting point. The dependent variable in the displacement time graph is displacement, which is represented on the y-axis, and the independent variable is time, which is represented on the x-axis. Position-time graphs are another name for displacement time graphs.

The displacement curve is given for 0 ≤ t ≤ 18.

We need to find the total distance of the curve, it will be the positive sum of all displacements.

So, total distance = (7-0) + (3-0) + (2+3) + (2+4) + (7-4)

                             = 7 + 3 + 5 + 6 + 3

                             = 24

Hence, the total distance is 24 units.

To learn more about displacement

https://brainly.com/question/12329052

#SPJ1

The smallest sample size that will result in a margin of error of no more than 5% for a 95% confidence interval is given as follows:

385.

A confidence interval of proportions has the bounds given by the rule presented as follows:

\(\pi \pm z\sqrt{\frac{\pi(1-\pi)}{n}}\)

In which the variables used to calculated these bounds are listed as follows:

The margin of error is modeled as follows:

\(M = z\sqrt{\frac{\pi(1-\pi)}{n}}\)

The confidence level is of 95%, hence the critical value z is the value of Z that has a p-value of \(\frac{1+0.95}{2} = 0.975\), so the critical value is z = 1.96.

We have no estimate, hence we consider that:

\(\pi = 0.5\)

The minimum sample size is obtained as n when M = 0.05, hence:

\(M = z\sqrt{\frac{\pi(1-\pi)}{n}}\)

\(0.05 = 1.96\sqrt{\frac{0.5(0.5)}{n}}\)

\(0.05\sqrt{n} = 1.96 \times 0.5\)

\(\sqrt{n} = 1.96 \times 10\) (0.5/0.05 = 10).

\((\sqrt{n})^2 = (1.96 \times 10)^2\)

n = 384.16

Hence rounded to 385, as a sample size of 384 would have a margin of error slightly above 0.05.

More can be learned about the z-distribution at https://brainly.com/question/25890103

#SPJ1

hi

Slope is 2 .  

here is how you do the trick :  by reading on the graph

if you go forward of  1 on the X axis , how much did the line goes up or down ?  

Here at  0  on  X- axis, we are at 0 on the Y- axis.

If you go forward of  1 on X axis, you are at 2 on Y axis.  

So the slope is  + 2

Other technique by calculus  between two points.  

Take two diffents  point on the X axis.   Call them A and B  

Slope is  :   (  f(A) -f(B) )     /  A-B

exemple here :   we have two easy points . Take 0  as  A  and  1 as  B

We read on the graph :  

A = 0  and B = 1

f(A)= 0  and f(B) = 2

Now we apply  formula :    

(  f(A) -f(B) )     /  (A-B )

(0 -2 )  / (0-1)  =  -2/-1 =  2

Slope is 2

Easy, dont you think ?

Answer:

The fourth choice.

Step-by-step explanation:

An exponential function passes through (3, 5) and (4, 10).

And we want to determine which other point is also on the graph.

First, we can find the exponential function. A standard exponential function is in the form:

\(y=a(b)^x\)

The point (3, 5) tells us that y = 5 when x = 3. Thus:

\(5=a(b)^3\)

The point (4, 10) tells us that y = 10 when x = 4. Thus:

\(10=a(b)^4\)

In the first equation, we can divide both sides by a:

\(\displaystyle \frac{5}{a}=b^3\)

And we can rewrite the second equation:

\(\displaystyle 2(5)=a(b^4)\Rightarrow 2\Big(\frac{5}{a}\Big)=b^4\)

Substitute:

\(2b^3=b^4\)

Divide:

\(b=2\)

Using the first equation again, substitute:

\(5=a(2)^3\)

Simplify and solve:

\(5=a(8)\Rightarrow \displaystyle a=\frac{5}{8}\)

So, our exponential function is:

\(\displaystyle y=\frac{5}{8}(2)^x\)

Next, we can simply try each point and see which point is correct.

Testing the first point (remember that (2,0) means that y = 0 when x = 2):

\(\displaystyle 0\stackrel{?}{=}\frac{5}{8}(2)^0=\frac{5}{8}(1)=\frac{5}{8}\neq 0\)

Testing the second point:

\(\displaystyle 1\stackrel{?}{=}\frac{5}{8}(2)^2=\frac{5}{8}(4)=\frac{5}{2}\neq 1\)

The third point:

\(\displaystyle 15\stackrel{?}{=}\frac{5}{8}(2)^5=\frac{5}{8}(32)=5(4)=20\neq 15\)

And the fourth point:

\(\displaystyle 20\stackrel{?}{=}\frac{5}{8}(2)^5=\frac{5}{8}(32)=5(4)=20\stackrel{\checkmark}{=}20\)

Therefore, D is the correct choice.

Answer:

(2,0)

Step-by-step explanation:

The answer is (2,0) because the number line passes through that point