Suppose you make the following deposits into an account earning 2.1%: $12,000 today followed by $6,000 each year for the next 7 years (so the last cash flow is at year 7). How much will you have in the account after 10 years? Round to the nearest dollar.
Answers
By making an initial deposit of $12,000 followed by annual deposits of $6,000 for the next 7 years into an account earning 2.1% interest, you will have approximately $63,274 in the account after 10 years.
To calculate the total amount in the account after 10 years, we need to consider the initial deposit, the annual deposits, and the interest earned.
The initial deposit of $12,000 will contribute to the account's value immediately.
For the annual deposits of $6,000 for the next 7 years, we can calculate the future value using the future value of an ordinary annuity formula:
\(FV = P * [(1 + r)^{n - 1}] / r\)
where FV is the future value, P is the annual payment, r is the interest rate per period, and n is the number of periods.
Using the formula, we can calculate the future value of the annual deposits:
\(FV = $6,000 * [(1 + 2.1\% / 100)^{7 - 1}] / (2.1\% / 100) = $42,274\)
(rounded to the nearest dollar).
To calculate the total amount in the account after 10 years, we need to add the initial deposit, the future value of the annual deposits, and any interest earned on these amounts over the 10-year period.
The interest earned on the initial deposit can be calculated as:
Interest = $12,000 * (2.1% / 100) * 10 = $2,520.
Adding the initial deposit, the future value of the annual deposits, and the interest earned, we get:
Total amount = $12,000 + $42,274 + $2,520 = $56,794.
However, we need to consider the interest earned on the account value over the last 3 years. Using compound interest, the interest earned on the total amount can be calculated as:
Interest = $56,794 * (2.1% / 100) * 3 = $3,576.
Adding the interest earned on the total amount, the final balance after 10 years is:
Final balance = $56,794 + $3,576 = $60,370.
Rounding to the nearest dollar, the total amount in the account after 10 years is approximately $63,274.
Learn more about deposits here:
https://brainly.com/question/11853650
#SPJ11
Related Questions
Calculate the length labeled x
Image above! PLEASE HELP ME WITH THESE 2 QUESTIONS ILL GIVE YOU BRAINLIST ANSWER

Answers
Answer:
Top-
11.5 or the square root of 133
Bottom-
15.3 or the square root of 235
Answer:
c) \(x=\sqrt{133}\)
d)\(x=\sqrt{235}\)
Step-by-step explanation:
Pythagorean Theorem: \(a^2+b^2=c^2\)
c) Solve for that side length and then solve for x
\(4^2+y^2=10^2\\y=\sqrt{133} \\(\sqrt{84} )^2+7^2=x^2\\x=\sqrt{133}\)
d) Solve for that side length and then solve for x
\(5^2+y^2=10^2\\y=\sqrt{(16^2-5^2)} \\(\sqrt{231} )^2+7^2=x^2\\x=\sqrt{235}\)
5(x+1)=4x+21
solve please
Answers
You have to move the terms then solve the problem
help please (not hard but I dont understand lol)

Answers
Answer:
C
Step-by-step explanation:
Function
\(f(x) = 1.5x\)
(22, 33) is a valid point in this line, therefore it's (22, 33), aka option C
If y=1-x+6x^(2)+3e^(x) is a solution of a homogeneous linear fourth order differential equation with constant coefficients, then what are the roots of the auxiliary equation?
Answers
The roots of the auxiliary equation are 0 (repeated root) and -b, where b is a constant.
To find the roots of the auxiliary equation for a homogeneous linear fourth-order differential equation with constant coefficients, we need to substitute the given solution into the differential equation and solve for the roots.
The given solution is: \(y = 1 - x + 6x^2 + 3e^x.\)
The general form of a fourth-order homogeneous linear differential equation with constant coefficients is:
ay'''' + by''' + cy'' + dy' + ey = 0.
Let's differentiate y with respect to x to find the first and second derivatives:
\(y' = -1 + 12x + 3e^x,\)
\(y'' = 12 + 3e^x,\)
\(y''' = 3e^x,\)
\(y'''' = 3e^x.\)
Now, substitute these derivatives into the differential equation:
\(a(3e^x) + b(3e^x) + c(12 + 3e^x) + d(-1 + 12x + 3e^x) + e(1 - x + 6x^2 + 3e^x) = 0.\)
Simplifying the equation, we get:
\(3ae^x + 3be^x + 12c + 3ce^x - d + 12dx + 3de^x + e - ex + 6ex^2 + 3e^x = 0.\)
Rearranging the terms, we have:
\((6ex^2 + (12d - e)x + (3a + 3b + 12c + 3d + 3e))e^x + (12c - d + e) = 0.\)
For this equation to hold true for all x, the coefficients of each term must be zero. Therefore, we have the following equations:
6e = 0 ---> e = 0,
12d - e = 0 ---> d = 0,
3a + 3b + 12c + 3d + 3e = 0 ---> a + b + 4c = 0,
12c - d + e = 0 ---> c - e = 0.
From the equations e = 0 and d = 0, we can deduce that the differential equation has a repeated root of 0.
Substituting e = 0 into the equation c - e = 0, we get c = 0.
Finally, substituting d = 0 and c = 0 into the equation a + b + 4c = 0, we have a + b = 0, which implies a = -b.
Therefore, the roots of the auxiliary equation are 0 (repeated root) and -b, where b is a constant.
To know more about auxiliary equation refer here:
https://brainly.com/question/18521479
#SPJ11
At a baseball game, 212 cups of coffee were sold, and $489 was collected. If small coffees sold for $2 each and large coffees sold for $3 each, how many of each size was sold?
Answers
There are 147 small coffees and 65 large coffees were sold.
What is equation?
A mathematical definition of an equation is a claim that two expressions are equal when they are joined by the equals sign ("="). For illustration, 2x - 5 = 13. 2x - 5 and 13 are expressions in this case. These two expressions are joined together by the sign "=".
Let's call the number of small coffees sold "x" and the number of large coffees sold "y".
We know that a small coffee costs $2 and a large coffee costs $3, and that a total of 212 cups of coffee were sold, so we can set up two equations based on the number of cups sold and the amount of money collected:
x + y = 212 (equation 1: total number of cups sold)
2x + 3y = 489 (equation 2: total amount of money collected)
Now we can solve for x and y by using substitution or elimination:
Solve equation 1 for x in terms of y:
x = 212 - y
Substitute this expression for x into equation 2:
2(212 - y) + 3y = 489
Distribute the 2:
424 - 2y + 3y = 489
Simplify:
y = 65
Substitute this value for y into the expression we found for x:
x = 212 - y = 212 - 65 = 147
Therefore, 147 small coffees and 65 large coffees were sold.
Learn more about equations on:
https://brainly.com/question/27893282
#SPJ1
NUMBER 14 PLEASEE AHHH

Answers
the correct answer is false
what is equivalent to (10+7r-r^2)+(-6r^2-18+5r)
Answers
Answer:
- 7r² + 12r - 8
Step-by-step explanation:
Given
(10 + 7r - r² ) + (- 6r² - 18 + 5r) ← remove parenthesis
= 10 + 7r - r² - 6r² - 18 + 5r ← collect like terms
= - 7r² + 12r - 8
The expression (10 + 7r - r² ) + (- 6r² - 18 + 5r) is equivalent to - 7r² + 12r - 8 after applying the property.
What is an expression?It is defined as the combination of constants and variables with mathematical operators.
It is given that:
The expression is:
(10 + 7r - r² ) + (- 6r² - 18 + 5r)
Using distribution property:
After applying the above property the expression can be written as:
10 + 7r - r² - 6r² - 18 + 5r
Adding like terms:
- 7r² + 12r - 8
Thus, the expression (10 + 7r - r² ) + (- 6r² - 18 + 5r) is equivalent to - 7r² + 12r - 8 after applying the property.
Learn more about the expression here:
brainly.com/question/14083225
#SPJ6
Please Answer Quickly
A ladder that is 24 feet long reaches the window of a building 18 feet above ground. What is the distance from the base of the ladder to the building? Express your answer as a radical
Answers
i think it is six.
EXPLANATION
Hi can anyone help me with question 1 and 3

Answers
Answer:
292 and 2092
Step-by-step explanation:
Surface area:
Wall 1: 40 x 3 = 120m^2
Wall2: 40 x 3 = 120m^2
Wall 3: 15 x 3 = 45m^2
Wall 4: 15 x 3 = 45m^2
Ceiling: 15 x 40 = 600m^2
Total : 240 + 600 + 90 = 730
So, cost of painting = 730/25 x 10 = 29.2 x 10 = 292
Total: 292 + 1800 = 2092
The total cost of paint would be 2092 dollars.
The area of the cuboid is the sum of product of the length, breadth of the given prism.
Surface area:
Wall 1: 40 x 3 = 120m^2
Wall2: 40 x 3 = 120m^2
Wall 3: 15 x 3 = 45m^2
Wall 4: 15 x 3 = 45m^2
Ceiling: 15 x 40 = 600m^2
The Total area: 240 + 600 + 90 = 730
So, The cost of painting = 730/25 x 10
= 29.2 x 10
= 292
Total: 292 + 1800 = 2092
Learn more about cuboid;
https://brainly.com/question/15843335
#SPJ2
3) Hallar el conjunto solución de las siguientes ecuaciones y realizar la comprobación. a) 2x+7= -3 b) 7x-3= 9x+5 h) (2x-1)3= -125 c) 6x+7-2x = -x-8 i) 2 (x+7)2= 242 d) -9+6x= 15+2x j) 1 + √2x + 3 = 6 e) 4x-8= -4 (2x-3)+4 k) √3x + 22 = 4 f) 2x+17 = 5 (x+1) g) 7x-5= -3 (-x-9)
Answers
Step-by-step explanation:
a) 2x+7= -3
2x = -10
x = -5.
b) 7x-3= 9x+5
7x - 9x = 5 + 3
-2x = 8
x = -4
h) (2x-1)3= -125
6x - 3 = -125
6 x = -128
x = 21.33
c) 6x+7-2x = -x-8
6x -2x -x = -8 -7
3x = -15
x = -5
i) 2 (x+7)2= 242
2x + 14 * 2 = 242
2x + 28 = 242
2x = 242 + 28
2x = 270
x = 135.
d) -9+6x= 15+2x
6x -2x = 15 + 9
4x = 24
x = 6.
j) 1 + √2x + 3 = 6
√2x + 4 = 6
√2x = 6+4
√2x = 10
x = 7.1
e) 4x-8= -4 (2x-3)+4
4x - 8 = -8x +12 + 4
4x -8 = -8x + 16
4x - 8x = 16 - 8
-4x = 8
x = -2.
k) √3x + 22 = 4
√3x = 4 + 22
√3x = 26
x = 15
f) 2x+17 = 5 (x+1)
2x + 17 = 5x + 5
2x - 5x = 5 -17
-3x = -12
x = 4.
g) 7x-5= -3 (-x-9)
7x - 5 = 3x + 27
7x - 3x = 27 + 5
4x = 32
x = 8.
Wer +266. A bag contains $3$ balls labeled $2, 4$ and $8$. A ball is to be picked, the value on the label is to be recorded and then the ball is to be returned to the bag. This will be done three times and then the values will be added together. What is the sum of all of the possible distinct sums?
Answers
Answer:
The sum of all the possible distinct sum is 128
Step-by-step explanation:
The number of balls in the bag = 3
The ball labels, (the numbers written on the balls) = 2, 4, and 8
The number of balls selected with replacement = 1
Therefore, we have have, the number of ways of selecting 1 ball from 3 = 3 ways
The distinct combination of the selected balls are;
2, 2, 2 with sum 2 + 2 + 2 = 6
2, 2, 4 with sum 2 + 2 + 4 = 8
2, 2, 8 with sum 2 + 2 + 8 = 12
2, 4, 4 with sum 2 + 4 + 4 = 10
2, 4, 8 with sum 2 + 4 + 8 = 14
2, 8, 8 with sum 2 + 8 + 8 = 18
4, 4, 4 with sum 4 + 4 + 4 = 12
4, 4, 8 with sum 4 + 4 + 8 = 16
8, 8, 4 with sum 8 + 8 + 4 = 20
8, 8. 8 with sum 8 + 8 + 8 = 24
The distinct sums are therefore;
6, 8, 12, 10, 14, 18, 16, 20, 24
The sum of the distinct sum is 6 + 8 + 12 + 10 + 14 + 18 + 16 + 20 + 24 = 128.
Answer: 128
Step-by-step explanation:
Any sum formed by a combination of the numbers 2, 4, 8 and must be divisible by 2. The smallest possible value of such a sum is equal to 3*2=6 , and the largest possible value of such a sum is equal to 3*8=24 . After testing, we find that
6 = 2+2+2, 8=4+2+2, 10=4+4+2
12 = 4+4+4, 14 = 8+4+2, 16 = 8+4+4
18 = 8+8+2, 20 = 8+8+4, 24 = 8+8+8
However, we cannot find a combination that will add to be 22: if two of the numbers are not 8, then the maximum possible sum is 4+4+8 = 16. Thus, two of the numbers picked must be 8, but then the third ball must have the number 6, which is not possible. Thus, the answer is the sum of the even numbers from 6 to 24 excluding 22, which is 128 .
What are the solutions of this quadratic equation? X^2+10=0
Answers
Answer:
+3.16i, -3.16i
Step-by-step explanation:
This has complex roots.
ax² + bx + c = 0
a = 1
b = 0
c = 10
Roots: +3.16i, -3.16i
derive equation (25-11) for component a in terms of molar units, starting with the control-volume expression for the conservation of mass.
Answers
The equation states that the gradient of the natural logarithm of the density of component A, denoted as (∇ ln(ρA)), is equal to the negative divergence of the velocity vector (∇ · V).
To derive equation (25-11), we start with the general form of the conservation of mass equation:
∂(ρA) / ∂t + ∇ · (ρA V) = ṁA_gen Where:
ρA is the density of component A,
t is time,
V is the velocity vector,
∇ is the gradient operator,
ṁA_gen is the net rate of generation of component A within the control volume.
Assuming steady-state conditions (no change with time) and neglecting any mass generation or consumption, we can simplify the equation to:
∇ · (ρA V) = 0
This equation states that the divergence of the mass flux of component A is zero, indicating a steady-state condition.
By applying the divergence theorem, we can rewrite the equation as:
∫(∇ · (ρA V)) dV = 0
Using the product rule of divergence, we have:
∫(∇ρA · V + ρA ∇ · V) dV = 0
Since the control volume is arbitrary, the integral can be simplified to:
∇ρA · V + ρA ∇ · V = 0
Rearranging the equation, we obtain:
∇ρA · V = -ρA ∇ · V
Dividing both sides by ρA, we get:
V · (∇ρA / ρA) = -∇ · V
Finally, recognizing that the left side of the equation is the gradient of the natural logarithm of ρA (i.e., (∇ ln(ρA))), we have:
(∇ ln(ρA)) · V = -∇ · V
Learn more about divergence theorem here:
https://brainly.com/question/10773892
#SPJ11
Ruby bought a new car for £10625. She got a discount of 15% of the recommended retail
price of the car. How much money did Ruby save?
Answers
Answer:
15/100 x 10625
Saved: 1593.75 pounds
Which graph represents this table of an arithmetic sequence?

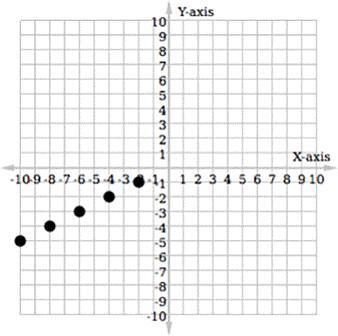



Answers
Answer:
the x axis contains the arithmetic sequence
I need help asap help me with all questions and please put. The number for the question so I know thank you

Answers
Answer:
1. 6
2. 8
3. 80
4. 11
5. 60
6. 3
7. 30
8. 0.8
9. 5
10. 600
11. 150
12. 110
13. 15
14. 1.1
15. 36
Hope it helps
A message digest is defined as him) - (m*7;2 MOD 7793. If the message m = 23, calculate the hash
Answers
The hash of the given message is 135.
In computing, a message digest is a fixed-sized string of bytes that represents the original data's cryptographic hash. This hash is used to authenticate a message, guaranteeing the integrity of the data in the message.
Here, it is given the message m = 23
The formula to calculate hash is him) - (m*7;2 MOD 7793.
So, let's calculate the hash : him) - (m*7;2 MOD 7793(him) - (23*7;2 MOD 7793
⇒ (8*23) - (49 MOD 7793)
⇒ 184 - 49= 135.
So, the hash of the given message is 135.
To know more about message digest, please click here:
https://brainly.com/question/6384456
#SPJ11
Kami was taking a quiz and she decided to simplify the following expression Her steps are shown below. Step 1: 1.3 (5+2.3m - 2.4) Step 2: 6.3+3.6m - 1.1 Step 3: 6.3-1.1 +3.6m Step 4: 5.2+3.6m Which statement identifies and explains Kami's first mistake?
a. Kami's first mistake was in Step 4. She should have reordered the terms by writing 3.6m first and then 5.2.
b. Kami's first mistake was in Step 2. of multiplying by 1.3. She added 1.3 to each term instead
c. Kami's first mistake was in Step 2. She should only add 1.3 to 5 and not to all 3 terms.
d. Kami's first mistake was in Step 3. She should have made 3.6m negative and the 1.1 should be positive.
e. Kami's first mistake was in Step 2. She should multiply 1.3 to 5 and to -2.4 only instead of adding by 1.3 to each term.
Answers
The statement that shows kemi first mistake is that
' Kemi first mistake was in step 2 , instead of multiplying 1.3 with all terms , she added 1.3 to each term instead'. (optionB)
What is simplification of expression?Simplification is the process of replacing a mathematical expression by an equivalent one, that is simpler. For example;
5( 2x+6) can be simplified by using distributive law. i.e 5× 2x + 5× 6
= 10x + 30
Similar simplifying 1.3 (5+2.3m - 2.4), we use distributive law to simplify.
1.3 × 5 + 1.3 × 2.3m -2.4 × 1.3
= 6.5 + 2.99m - 3.12
The next step is to collect like terms
= 6.5-3.12 + 2.99m
= 3.38 + 2.99m
Instead of using the distributive law, Kami added 1.3 to all the terms in parentheses.
learn more about simplification of expression from
https://brainly.com/question/723406
#SPJ1
What is the simplest fraction Kieran could be thinking of? My fraction is larger than 0.2 but smaller than 0.4 and when I convert my fraction to a decimal it has one decimal place.
Answers
Answer:
The fraction is 3/10 = .3
PLEEEEEEEASE HELLLP What is the midpoint of segment RT with endpoints at (-5,2) and (1, -3)?
Answers
Answer:
-2, -1/2
Step-by-step explanation:
Step-by-step explanation:
The midpoint of a line segment between two points is given by
\(M = ( \frac{x1 + x2}{2} , \frac{y1 + y2}{2} )\)
where ( x1 , y1) and ( x2 , y2) are the points
From the question
The midpoint of the line segment using points (-5,2) and (1, -3) is
\(M = ( \frac{ - 5 + 1}{2} , \frac{2 - 3}{2} )\)
\(M = ( - \frac{ 4}{2} , - \frac{1}{2} )\)
We have the final answer as
\(M = ( - 2, - \frac{1}{2} )\)
Hope this helps you
2.) Let's consider a Stackelberg version of monopolistic competition. Suppose market demand is given by P = 30 – Q and there are ""n"" firms in the market with the first firm denoted as the leader
Answers
The specific numerical solution will depend on the number of firms in the market and their behavior.
In a Stackelberg version of monopolistic competition, there is a leader firm that sets its output first, and all the other firms in the market act as followers and choose their outputs simultaneously.
Suppose there are "n" firms in the market, with the first firm denoted as the leader. Let's assume that each firm has a constant marginal cost of $10 per unit. Then, the leader firm's profit-maximizing output level can be found by solving the following problem:
max (30-Q1-Q2-...-Qn)Q1 - 10Q1
subject to Q1 >= 0
where Q1 is the output level of the leader firm, and Q2, Q3, ..., Qn are the output levels of the follower firms.
Taking the first-order condition by differentiating with respect to Q1 and setting it equal to zero, we get:
d/dQ1 [(30-Q1-Q2-...-Qn)Q1 - 10Q1] = 0
Simplifying this expression, we get:
30 - Q1 - Q2 - ... - Qn - 2Q1 = 0
Solving for Q1, we get:
Q1 = (30 - Q2 - ... - Qn)/3
This equation gives us the leader firm's profit-maximizing output level as a function of the follower firms' output levels.
Now, let's consider the follower firms' profit-maximizing output levels. Since each follower firm is a price-taker, its profit-maximizing output level can be found by equating marginal cost to market price, which is equal to the market demand curve divided by the total quantity produced by all firms in the market. Therefore, the profit-maximizing output level of the jth follower firm can be expressed as:
Qj* = (1/n) * (30 - Q1 - Q2 - ... - Qj-1 - Qj+1 - ... - Qn - 10)
where Qj* is the follower firm's profit-maximizing output level, and j = 2, 3, ..., n.
Using these expressions for the leader and follower firms' profit-maximizing output levels, we can solve for the equilibrium outputs and profits in the Stackelberg version of monopolistic competition. However, the specific numerical solution will depend on the number of firms in the market and their behavior.
To learn more about market visit:
https://brainly.com/question/13414268
#SPJ11
if rx y= 0.83, then we can conclude that x and y have a relatively
Answers
If rxy = 0.83, we can conclude that x and y have a relatively strong positive linear relationship or correlation.
The correlation coefficient (r) measures the strength and direction of the linear relationship between two variables, in this case, x and y. The value of r ranges between -1 and 1. A positive value indicates a positive relationship, meaning that as one variable increases, the other variable tends to increase as well.
In this case, with rxy = 0.83, the correlation coefficient is close to 1, suggesting a strong positive linear relationship. This means that when x increases, y also tends to increase, and vice versa. The closer the value of r is to 1, the stronger the linear relationship between x and y.
It is important to note that correlation does not imply causation. While a high correlation coefficient indicates a strong linear relationship, it does not provide information about the underlying cause or direction of the relationship between the variables. Other factors and variables may influence the relationship, and further analysis may be required to understand the nature of the relationship between x and y.
To learn more about correlation click here:
brainly.com/question/16169720
#SPJ11
Once we have categorized an object, our memory of the object increasingly resembles thecategoryA) algorithm.B) prototype.C) heuristic.D) mental set
Answers
Once we have categorized an object, our memory of the object increasingly resembles the category is B) prototype. This means that when we categorize an object, our memory of it begins to resemble the prototype or typical example of that category. For example, if we categorize a bird as a robin, our memory of the bird will increasingly resemble the characteristics of a typical robin.
This happens because our brain uses prototypes as a shortcut to process information and make sense of the world around us. We use prototypes to quickly identify objects and make assumptions about their characteristics based on their category.
our memory of an object after categorization is influenced by the prototype of the category. This helps us to quickly process and make sense of information, but it can also lead to errors and biases in our thinking.
A prototype is a mental image or best example of a category. When we categorize an object, our memory of the object increasingly resembles the prototype because we tend to recall the most representative or typical example of the category.
Once we categorize an object, our memory of the object becomes more like the prototype, which is the best example of the category. This is because we tend to remember the most representative or typical examples of a category.
To know more about prototype, visit:
https://brainly.com/question/28187820
#SPJ11
Which method for solving a system of equations starts by solving one equation for one variable?
Select one:
A. Substitution Method
B. Elimination Method
Answers
Answer: A: substitution method
Step-by-step explanation:
The substitution method is one way of solving systems of equations. To use the substitution method, use one equation to find an expression for one of the variables in terms of the other variable. Then substitute that expression in place of that variable in the second equation.
10^2 x 10^6 x 10 using single exponent
Answers
Step-by-step explanation:
10^2 + 10^6 + 10^1 = 10 ^(2+6+1) = 10 ^9
hello can you help me with this plane trigonometry question please and thank you for your time for doing this
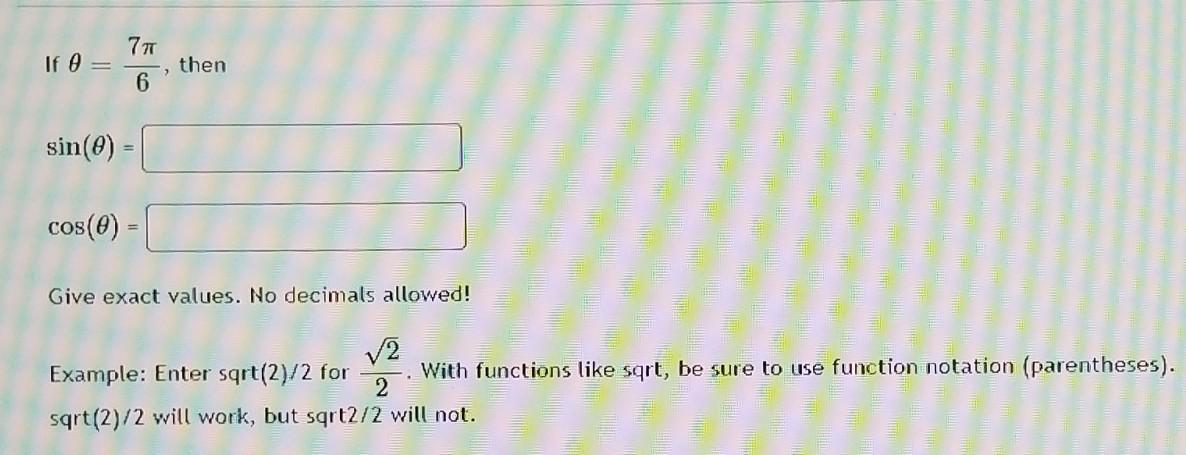
Answers
hello
\(\theta=\frac{7\pi}{6}\)\(\sin \theta=\frac{\sin 7\pi}{6}\)we need to first convert the values into degrees first
\(\begin{gathered} 2\pi=360 \\ \frac{7\pi}{6}=x \\ 2\pi x=\frac{7\pi}{6}\times360 \\ 2x\times6=7\times360 \\ 12x=2520 \\ x=\frac{2520}{12} \\ x=210 \\ \theta=210^0 \end{gathered}\)now we can convert the values in degrees to surds form
\(\begin{gathered} \sin 210=-\frac{1}{2} \\ \frac{\sin 7\pi}{6}=-\frac{1}{2} \end{gathered}\)now let's solve for cosine
\(\begin{gathered} \cos 210=-\frac{\sqrt[]{3}}{2} \\ \frac{\cos 7\pi}{6}=-\frac{\sqrt[]{3}}{2} \end{gathered}\)An arts academy requires there to be 4 teachers for every 100 students and 5 tutors for every 60 students. How many students does the academy have per teacher? Per tutor? How many tutors does the academy need if it has 144 standents?
Answers
Answer: 25 students per teacher and 12 students per tutor. They need 2.4 tutors for the school
Given: AB is parallel to DC and E is the midpoint of AC.
Prove: ▲ABE ≅ ▲CDE
Answers
Therefore, the AAA test of congruency demonstrated that ABE is Congruence to CDE.
Congruent Definition ExampleSomething that is "exactly equal" in terms of size and shape is referred to as congruent. No matter which way we spin, flip, or rotate the shapes, they remain accurate. For instance, you may draw two circles with the same radius, cut them out, and stack them.
In this case, we are aware that AB ll DC - GIVEN
In light of the characteristic of parallel lines,
Let's recall the property of parallel lines:-
"When the pair of alternating angles equals one another, two straight lines become parallel."
Alternate angles are angle ABE and angle CDB.
angle BAE ≅ angle DCE -{alternate angles}
E is a midway, given that.
According to the midpoint theorem, opposite angles are congruent when angle AES and angle CED are compared.
Here, we may see the congruency test from AAA.
Hence its proved that ∆ABE ≅ ∆CDE by AAA test of congruency.
To know more about Congruence visit:-
https://brainly.com/question/7888063
#SPJ1
The cost of renting a bicycle, y, for
x hours can be modeled by a linear
function. Renters pay a fixed insurance
fee of $12 plus an additional cost of $10
per hour, for a maximum of 6 hours.
What is the range of the function for this
situation?
Answers
Answer:
Step-by-step explanation:
9x - 1 / 2 + 9/2 x = 0.128886 (43)
2 / 5x - 1b = 4/3 - 1 - 1 = 2x (4)
= x1 + 24 - 3/4 + 8
9/3 - 1 x = /3
a blank CD can hold 80 minutes of music you have burned m minutes of music onto the cd which equation models the amount of time that is left on the cd
Answers
The equation that models the amount of time that is left on the cd is 80 - m.
What is an equation?An equation is the statement that illustrates that the variables given. In this case, two or more components are taken into consideration to describe the scenario.
It is vital to note that an equation is a mathematical statement which is made up of two expressions that are connected by an equal sign.
In this case, the blank CD can hold 80 minutes of music you have burned m minutes of music onto the cd. The time left will be:
= Total minutes - Time used
= 80 - m
This illustrates the equation.
Learn more about equations on:
brainly.com/question/2972832
#SPJ1