Answers
Related Questions
4a) Why are the two triangles congruent? *
H
M
K
J
L

Answers
Answer:
SAS Congruency (see below)
Step-by-step explanation:
First, prove the two triangles congruent:
You should know that ∠HKL ≅ ∠MKL because of Vertical Angles Congruence Theorem because they're vertical angles.
If it's already given that HK ≅ MK and JK ≅ LK, then you can clearly see that you can use the SAS Postulate to prove ΔHKL ≅ ΔMKL.
For a flow chart proof for this, refer to the image:

find the value of the variable in the picture below. round to the nearest tenth if necessary

Answers
To find the angles shown we need to remember the definition of the tangent function:
\(\tan \theta=\frac{\text{opp}}{\text{adj}}\)In the case for angle x we notice that the opposite leg is 1 and the adjacent leg is 3, plugging this values in the equation above and solving for x we have:
\(\begin{gathered} \tan x=\frac{1}{3} \\ x=\tan ^{-1}(\frac{1}{3}) \\ x=18.4 \end{gathered}\)In the case for angle y we notice that the opposite leg is 3 and the adjacent leg is 1, plugging this values in the equation above and solving for y we have:
\(\begin{gathered} \tan y=\frac{3}{1} \\ y=\tan ^{-1}(\frac{3}{1}) \\ y=71.6 \end{gathered}\)Therefore, x=18.4° and y=71.6°
Which part of this graph shows a nonlinear relationship?
О A. 1
О B. 3
О C. 4
О D. 2

Answers
Answer:
it is 4 feet deep
Step-by-step explanation:
What are the best
Descriptions for the data sets? Explain why.
Mean 79 median 84 mode 83
Best description of the data set?
Why?
Mean 10 median 8 mode 3
Best description of the data set?
Why?
Mean 46 median 52 mode 80
Best description of the data set?
Why?
Answers
1. For the data set with Mean 79, Median 84, and Mode 83:
The best description for this data set would be moderately positively skewed because the mean (79) is lower than the median (84), indicating the presence of some lower values that pull the mean down.
2. For the data set with Mean 10, Median 8, and Mode 3:
The best description for this data set would be highly positively skewed because the mean (10) is higher than the median (8), suggesting the presence of a few higher values that pull the mean up.
3. For the data set with Mean 46, Median 52, and Mode 80:
The best description for this data set would be slightly negatively skewed because the mean (46) is lower than the median (52), indicating the presence of some higher values that pull the mean down.
For the data set with Mean 79, Median 84, and Mode 83:
The best description for this data set would be that it is moderately positively skewed.
This is because the mean (79) is lower than the median (84), indicating that there are some lower values that pull the mean down.
The mode (83) being close to the median suggests that it is a relatively common value in the data set.
Overall, this data set is slightly skewed to the left, but not excessively so.
For the data set with Mean 10, Median 8, and Mode 3:
The best description for this data set would be that it is highly positively skewed.
The mean (10) is higher than the median (8), which suggests the presence of a few higher values that pull the mean up.
The mode (3) being significantly lower than the median indicates that 3 is the most frequently occurring value in the data set.
The skewness towards the right indicates that there are some extreme values that are significantly higher than the rest of the data.
For the data set with Mean 46, Median 52, and Mode 80:
The best description for this data set would be that it is moderately negatively skewed.
The mean (46) is lower than the median (52), implying the presence of some higher values that pull the mean down.
The mode (80) being higher than both the mean and median indicates that 80 is the most common value in the data set.
This data set shows a slight skewness to the left, but not as pronounced as the first example.
There may be a few outliers on the lower end, but the majority of the data is centered around the higher values.
In summary, the best descriptions for the data sets are based on the relationship between the mean, median, and mode.
Analyzing these measures helps us understand the central tendency and the shape of the distribution, whether it is symmetric or skewed.
For similar question on data set.
https://brainly.com/question/30154121
#SPJ8
Find the length of side x in simplest radical form with a rational denominator.

Answers
Answer:
x = 1.58Step-by-step explanation:
base = X
hypotenuse = √5
cos 45° = base / hypotenuse
1/√2 = X / √5
X = √5 / √2
X = 1.58
8.71 ... 2 significant figure
Answers
Answer:
8.71
Sig Figs
3
8.71
Decimals
2
8.71
Scientific Notation
8.71 × 100
E-Notation
8.71e+0
Words
eight point seven one
Step-by-step explanation:
Angle EFG is 80
Find side of angle x
Answers
80+2x=180
2x=180-80
2x=100
x=100/2
x=50
9(x - 2) = 7x + 5 a) true for all values of x b) true for one value of x c) true for no values of x
Answers
Answer:
b) true for one value
Explanation:
If you solve for x, there is only one value of x
Answer:
(b)
Step-by-step explanation:
9(x - 2) = 7x + 5 ← distribute left side
9x - 18 = 7x + 5 ( subtract 7x from both sides )
2x - 18 = 5 ( add 18 to both sides )
2x = 23 ( divide both sides by 2 )
x = \(\frac{23}{2}\)
Solve the following Linear Programming Problem by Graphical Method:
Max z = 15x1 + 20 xz x₁ + 4x₂ ≥ 12 x₁ + x₂ ≤ 6 s.t., and x₁, x₂ ≥ 0
Answers
The solution to the linear programming problem is:
Maximum value of z = 120
x₁ = 0, x₂ = 6
To solve the given linear programming problem using the graphical method, we first need to plot the feasible region determined by the constraints and then identify the optimal solution.
The constraints are:
x₁ + x₂ ≥ 12
x₁ + x₂ ≤ 6
x₁, x₂ ≥ 0
Let's plot these constraints on a graph:
The line x₁ + x₂ = 12:
Plotting this line on the graph, we find that it passes through the points (12, 0) and (0, 12). Shade the region above this line.
The line x₁ + x₂ = 6:
Plotting this line on the graph, we find that it passes through the points (6, 0) and (0, 6). Shade the region below this line.
The x-axis (x₁ ≥ 0) and y-axis (x₂ ≥ 0):
Shade the region in the first quadrant of the graph.
The feasible region is the overlapping shaded region determined by all the constraints.
Next, we need to find the corner points of the feasible region by finding the intersection points of the lines. In this case, the corner points are (6, 0), (4, 2), (0, 6), and (0, 0).
Now, we evaluate the objective function z = 15x₁ + 20x₂ at each corner point:
For (6, 0): z = 15(6) + 20(0) = 90
For (4, 2): z = 15(4) + 20(2) = 100
For (0, 6): z = 15(0) + 20(6) = 120
For (0, 0): z = 15(0) + 20(0) = 0
From the evaluations, we can see that the maximum value of z is 120, which occurs at the corner point (0, 6).
Know more about linear programming problem here:
https://brainly.com/question/29405467
#SPJ11
Which statements are true? Select three options. ∠F corresponds to ∠F'. Segment EE' is parallel to segment FF'. The distance from point D' to the origin is the distance of point D to the origin. The measure of ∠E' is the measure of ∠E. △DEF △
Answers
Answer:
Triangle DEF was dilated according to the rule DO,One-third(x,y)(one-third x, one-third y) to create similar triangle D'E'F'.
On a coordinate plane, (0, 0) is the center of dilation. Triangle F D E is dilated to create smaller triangle F prime D prime E prime. Triangle F D E has points (negative 9, 3), (negative 9, 9), (negative 6, 6). Triangle F prime D prime E prime has points (negative 3, 1), (negative 3, 3), and (negative 2, 2).
Which statements are true? Select three options.
right answer ∠F corresponds to ∠F'.
wrong answer Segment EE' is parallel to segment FF'.
right answer The distance from point D' to the origin is One-third the distance of point D to the origin.
wrong answer The measure of ∠E' is One-third the measure of ∠E.
right answer△DEF △D'E'F'
Step-by-step explanation:
Answer:
A, C, E
Step-by-step explanation:
EDU
y =7 -3x is it linear or non linear?
Answers
Answer:
y = 7 - 3x is linear.
10 cards are numbered from 1 through 10. The cards are drawn at random. If two cards are drawn with replacement, find the probability of choosing a prime number in both the first and the second draw.
Answers
Answer:
4/25 or 16%
Step-by-step explanation:
There are 4 prime numbers between 1 and 10 (2, 3, 5, 7)
There are 10 cards to choose from.
The formula for probability is (success)/(total)
4/10 x 4/10 = 16/100 or 4/25
The probability of choosing a prime number in both the first and the second draw is 16%
What is probability?Probability is a measure of the likelihood of an event to occur. Many events cannot be predicted with total certainty. We can predict only the chance of an event to occur i.e., how likely they are going to happen, using it.
Given that, 10 cards are numbered from 1 through 10, two cards are drawn with replacement,
We need to find the probability of choosing a prime number in both the first and the second draw.
There are 4 prime numbers between 1 and 10 = 2, 3, 5, 7
There are 10 cards to choose from.
Probability = favorable outcomes / total outcomes
= 4/10 x 4/10
= 16/100
= 16%
Hence, the probability of choosing a prime number in both the first and the second draw is 16%
Learn more about probability, click;
https://brainly.com/question/30034780
#SPJ2
How many different ways are there to get 10 heads in 20 throws of a true coin?
Answers
Now how many ways can we get 10 heads? This is 20 choose 10, or (20/10)
This means our probability is (20/10)2(20)=184756/1048576≈.1762
How many and what type of solutions does 5x2−2x+6 have?
1 rational solution
2 rational solutions
2 irrational solutions
2 nonreal solutions
Answers
Answer:
2 nonreal solutions
Step-by-step explanation:
given a quadratic equation in standard form
ax² + bx + c = 0 (a ≠ 0 )
then the nature of the roots are determined by the discriminant
b² - 4ac
• if b² - 4ac > 0 then 2 real and irrational solutions
• if b² - 4ac > 0 and a perfect square then 2 real and rational solutions
• if b² - 4ac = 0 then 2 real and equal solutions
• if b² - 4ac < 0 then no real solutions
5x² - 2x + 6 = 0 ← in standard form
with a = 5 , b = - 2 , c = 6
b² - 4ac
= (- 2)² - (4 × 5 × 6)
= 4 - 120
= - 116
since b² - 4ac < 0
then there are 2 nonreal solutions to the equation
Select “spin” to start the spinner. Perform the experiment with 50 trials. What’s the experimental probability of the spinner landing on red?
Answers
Answer:
1/10
Step-by-step explanation:
Answer:
5/50 or 1/10
Step-by-step explanation:
Both are correct, but the best answer is 1/10 because it is the simplest, and its reduced form.
) calculate a 95 percent confidence interval for the difference between the mean rates for fixed- and variable-rate 48-month auto loans. can we be 95 percent confident that the difference between these means exceeds .4 percent? (round your answers to 4 decimal places.)
Answers
The 95% confidence interval for the difference between the mean rates for fixed- and variable-rate 48-month auto loans is (0.2877%, 0.5123%). Yes, we can be 95% confident that the difference between these means exceeds 0.4%.
To calculate a 95% confidence interval for the difference between the mean rates for fixed- and variable-rate 48-month auto loans, we need to know the sample means, standard deviations, and sample sizes for each group. Let's assume that these values are as follows:
Sample mean for fixed-rate 48-month auto loans = 4.5%
Sample standard deviation for fixed-rate 48-month auto loans = 1.2%
Sample size for fixed-rate 48-month auto loans = 100
Sample mean for variable-rate 48-month auto loans = 4.1%
Sample standard deviation for variable-rate 48-month auto loans = 1.3%
Sample size for variable-rate 48-month auto loans = 150
The formula for the 95% confidence interval for the difference between two means is:
CI = (X1 - X2) ± t(α/2, df) × √[(s1²/n1) + (s2²/n2)]
where:
X1 and X2 are the sample means for the two groups
s1 and s2 are the sample standard deviations for the two groups
n1 and n2 are the sample sizes for the two groups
t(α/2, df) is the t-value for the desired level of confidence (α) and degrees of freedom (df), which is calculated as (n1 + n2 - 2).
Plugging in the values, we get:
CI = (4.5% - 4.1%) ± t(0.025, 248) × √[(1.2%²/100) + (1.3%²/150)]
CI = 0.4% ± 1.9719 × 0.0551
CI = (0.2877%, 0.5123%)
Therefore, the 95% confidence interval for the difference between the mean rates for fixed- and variable-rate 48-month auto loans is (0.2877%, 0.5123%).
To determine if we can be 95% confident that the difference between these means exceeds 0.4%, we need to check if 0.4% falls outside the confidence interval. Since 0.4% is outside the interval, we can be 95% confident that the difference between these means exceeds 0.4%.
Learn more about confidence interval :
https://brainly.com/question/30215881
#SPJ4
calculate the height of an equilateral triangle with side is 8 cm long
Answers
Step-by-step explanation:
use pythagoras theorem for finding the height
hypotenuse is 8cm
base is 4 cm
height?
a^2+b^2=c^2
64-16=a^2
a=7.07cm
Height of equilateral triangle is 7.07cm
help pls i need this done like right now plss

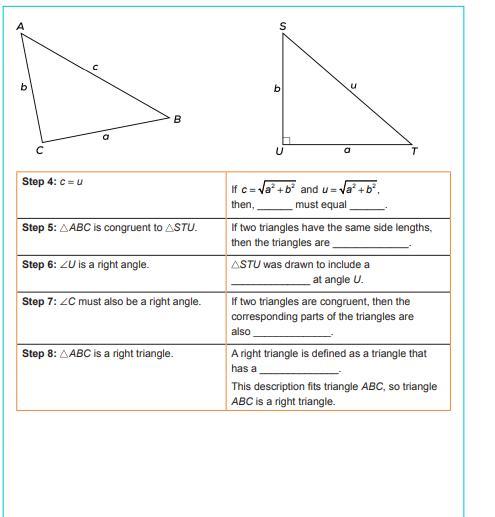

Answers
The options that complete the statements are:
Pythagoras' theoremc must equal u.the triangles are congruent.right anglecongruent.right triangle.What is the converse Pythagoras theorem?The converse Pythagoras theorem states that If the square of a side is equal to the sum of the square of the other two sides, then the triangle must be a right-angled triangle.
Thus, given ABC with side lengths a, b, c.
where a² + b² = c²
Then c must be the side opposite a right angle and ABC must be a right triangle.
Learn more about right angles at: https://brainly.com/question/1248322
#SPJ1
What is the value of expression below?

Answers
\(\huge\text{Hey there!}\)
\(\huge\boxed{\mathsf{\sqrt{49} + 2\times6}}\\\\\huge\boxed{= \mathsf{\bold{7}+ 2\times6}}\\\\\huge\boxed{= \mathsf{7 + \bf 12}}\\\\\huge\boxed{\mathsf{= \bf 19}}\\\\\\\\\huge\text{Therefore, your answer is: \boxed{\mathsf{19}}}\huge\checkmark\)
\(\huge\text{Good luck on your assignment \& enjoy your day!}\)
~\(\frak{Amphitrite1040:)}\)
5. (20 points) Solve the initial value problem y" – 2y′ + 10y = 0, y(0) = 0, y′(0) = 6
Answers
The solution to the initial value problem y" - 2y' + 10y = 0, y(0) = 0, y'(0) = 6 is y(t) = 6\(e^t\) × sin(3t).
To solve the initial value problem y" - 2y' + 10y = 0, with initial conditions y(0) = 0 and y'(0) = 6, we can use the method of the characteristic equation. Let's solve it step by step:
Step 1: Characteristic equation
We assume the solution has the form y = \(e^{(rt)\), where r is a constant. Substituting this into the differential equation, we get:
r² - 2r + 10 = 0
Step 2: Solve the characteristic equation
Solving the quadratic equation, we find the roots:
r = (2 ± sqrt(2² - 4(1)(10))) / 2
r = (2 ± sqrt(-36)) / 2
r = 1 ± 3i
Step 3: General solution
Since the roots are complex, the general solution of the differential equation can be written as:
y(t) = \(e^{(1t)\) (A × cos(3t) + B × sin(3t))
Step 4: Apply initial conditions
Using the initial condition y(0) = 0, we substitute t = 0 into the general solution:
0 = A × cos(0) + B × sin(0)
0 = A
Using the initial condition y'(0) = 6, we substitute t = 0 into the derivative of the general solution:
6 = (A × 1 × cos(0) - 3B × sin(0))
6 = A
Step 5: Final solution
Now we have A = 0 and B = 6. Substituting these values into the general solution, we obtain the particular solution:
y(t) = \(e^t\) × (0 × cos(3t) + 6 × sin(3t))
y(t) = 6\(e^t\) × sin(3t)
Learn more about the initial value problem at
https://brainly.com/question/30466257
#SPJ4
what is the domain of the function y=2squareroot x-5
Answers
Answer:
10
Step-by-step explanation:
your is muplitying it 20
Use a ruler and protractor to measure the sides and angles of each shape. Then use what
you learned about indicating congruence to show which shapes are congruent and which are not.

Answers
It is very difficult to measure the sides and angles of each shape on laptop or mobile.
Define the congruent triangle?Two triangles are said to be congruent if their corresponding sides and angles are equal in measure. In other words, if you can superimpose one triangle on top of the other so that all corresponding sides and angles match, then they are congruent.
There are several ways to prove that two triangles are congruent, including:
Side-Side-Side (SSS): If the three sides of one triangle are equal in measure to the three sides of another triangle, then the triangles are congruent.Side-Angle-Side (SAS): If two sides and the included angle of one triangle are equal in measure to two sides and the included angle of another triangle, then the triangles are congruent.Angle-Side-Angle (ASA): If two angles and the included side of one triangle are equal in measure to two angles and the included side of another triangle, then the triangles are congruent.Angle-Angle-Side (AAS): If two angles and a non-included side of one triangle are equal in measure to two angles and the corresponding non-included side of another triangle, then the triangles are congruent.Hypotenuse-Leg (HL): If the hypotenuse and one leg of a right triangle are equal in measure to the hypotenuse and one leg of another right triangle, then the triangles are congruent.To know more about triangle, visit:
https://brainly.com/question/17815056
#SPJ1
Please help me I need to turn this in soon

Answers
The range of 8,7,12,7,11,10,7,12
Answers
Answer:
a) Median: 9
b) Range: 5
c) Mode: 7
Explanation:
The median is the number in the middle.
First, you put the numbers in order: 7, 7, 7, 8, 10, 11, 12, 12
The middle of this is 8 and 10, so you plus them and divide by to 2, then it gives 9, so the median is 9.
To find the range, you minus the highest number and the lowest number, 12-7=5.
Mode is the most occurring and repetitive number, in this case, 7, because it is written 3 times.
Read more about this on brainly: https://brainly.com/question/17122811
1. Differentiate the function f(x) = ln (81 sin^2 (x)) f’(x) 2. Differentiate the function P(t) = in ( √t2 + 9) p' (t) 3. if x2 + y2 + z2 = 9, dx/dt = B, and dy/dt = 4, find dz/dt when (x,y,z) = (2,2,1)
dz/dt =
Answers
First you will get 4dz
I have to solve these using area models:
1. 30 x 24
2. 40 x 43
3. 30 x 37
4. 20 x 26
5. 50 x 35
Answers
if the odds in favor of a horse winning a race is 4:7 , what is the probability of the horse winning the race?
Answers
The probability of the horse winning the race is 4/11 or approximately 0.36 or 36%.
The odds in favor of a horse winning a race is expressed as a ratio of the number of favorable outcomes to the number of unfavorable outcomes. In this case, the odds in favor of the horse winning the race is 4:7.
This means that for every 4 favorable outcomes, there are 7 unfavorable outcomes.
To determine the probability of the horse winning the race, we can use the formula:
Probability = favorable outcomes / total outcomes
In this case, the total number of outcomes is the sum of the favorable and unfavorable outcomes, which is 4 + 7 = 11.
Therefore, the probability of the horse winning the race is:
Probability = 4 / 11
This means that the horse has a probability of approximately 0.36 or 36% of winning the race.
In summary, the odds in favor of a horse winning a race of 4:7 means that there are 4 favorable outcomes for every 7 unfavorable outcomes.
know more about the probability
https://brainly.com/question/23417919
#SPJ11
At the volunteer fire departments carnival the probability of winning a door prize is 3/4. If 800 people attend the carnival, about how many could be expected to win a door prize?
Show work please
Answers
800/4
=200
200 x 3
answer 600 people could be expected to win a door prize
The probability of getting a soda at the movie theater is 70%. What is the probability that the next three guests in the movie theater will get a soda
Answers
The probability that the next three guests in the movie theater will get a soda, when the probability of getting a soda at the movie theater is 70% is 0.343 or 34.3%.
What is probability?Probability of an event is the ratio of number of favorable outcome to the total number of outcome of that event.
The probability of getting a soda at the movie theater is 70%.
\(P=70\%\\P=\dfrac{70}{100}\\P=0.7\)
By the chain rule, the probability of getting the soda to the next 3 guest is equal to the cube of the probability of getting a soda at the movie theater.
Thus, the probability that the next three guests in the movie theater will get a soda is,
\(P^3=0.7^3\\P^3=0.343\)
Thus,the probability that the next three guests in the movie theater will get a soda, when the probability of getting a soda at the movie theater is 70% is 0.343 or 34.3%.
Learn more about the probability here;
https://brainly.com/question/24756209
#SPJ1
A class consisting of 3 undergraduate students and 9 graduate students is randomly divided into three groups of 4. What is the probability that each group includes an undergraduate student
Answers
The probability that each group includes an undergraduate student is approximately 0.565 or 56.5%. This can be determined by calculating the favorable outcomes and dividing it by possible outcomes.
First, let's consider the number of ways to select one undergraduate student from the three available. This can be done in 3 ways.
Next, for each selected undergraduate student, we need to select three more students from the remaining 11 students (9 graduate students and 2 remaining undergraduate students). This can be done in (11 choose 3) ways.
To calculate the total number of possible outcomes, we need to select three groups of 4 from the 12 students, which can be done in (12 choose 4) * (8 choose 4) * (4 choose 4) ways.
Therefore, the probability can be calculated as (3 * (11 choose 3)) / ((12 choose 4) * (8 choose 4) * (4 choose 4)).
Performing the calculations, the probability that each group includes an undergraduate student is approximately 0.565 or 56.5%.
Learn more about probability here:
brainly.com/question/32117953
#SPJ11
