Show that a discrete metric on a vector space X≠{0} cannot be obtained from a norm (the pair (X,d) is called the discrete metric space if the metric is d(x,x)=0, d(x,y)=1 for x≠y).

Answers
You know that the discrete metric only takes values of 1 and 0. Now suppose it comes from some norm ||.||. Then for any α in the underlying field of your vector space and x,y∈X, you must have that
∥α(x−y)∥=|α|∥x−y∥.
But now ||x−y|| is a fixed number and I can make α arbitrarily large and consequently the discrete metric does not come from any norm on X.
Step-by-step explanation:
hope this helps
Related Questions
I have not done domain or range in so long so pls help 60 points!!

Answers
Answer:
5. domain: -1 ≤ x ≤ 1
range: 0 ≤ y ≤ 1
6. domain: x < 1 or -1 < x < 1 or x > 1
range: y ≤ 1 or y > 1
i graphed the functions, hope this helps! <3

what is the domain of y=log5x?
A. all real numbers less than 0
B. all real numbers greater than 0
C. all real numbers not equal to 0
D. all real numbers
I'm sorry to rush but I need the answer kinda quickly
Answers
So the answer is B
two angles are complementary.the measure of the larger angle is two times the measure of the smaller angle .What is the measure of each angle ?
Answers
We know that :
The sum of two angles that are complementary = 90°
Smaller angle = x
Larger angle = 2x
We can make the formula as
= 2x + x = 90°
= 3x = 90°
= x = 90 ÷ 3
= x = 30
The smaller angle = x = 30°
The larger angle = 2x = 30 × 2 = 60°
Therefore , the measure of each of the angles are 30° and 60° .
The base of the mountain is 6,500 feet above sea level and AB measures 230 feet across. Given that the measurements for QAP is 20° and QBP is 35°, how far above sea level is peak P ? Express your answer to the nearest foot.
Height above sea level:

Answers
Answer:
6610
Step-by-step explanation:
We have tan(X) = opposite/ adjacent
tan(QBP) = PQ/BQ
tan(35) = PQ/BQ ---eq(1)
tan(QAP) = PQ/AQ
tan(20) = \(\frac{PQ}{AB +BQ}\)
\(=\frac{1}{\frac{AB+BQ}{PQ} } \\\\=\frac{1}{\frac{AB}{PQ} +\frac{BQ}{PQ} } \\\\= \frac{1}{\frac{230}{PQ} + tan(35)} \;\;\;(from\;eq(1))\\\\= \frac{1}{\frac{230 + PQ tan(35)}{PQ} } \\\\= \frac{PQ}{230+PQ tan(35)}\)
230*tan(20) + PQ*tan(20)*tan(35) = PQ
⇒ 230 tan(20) = PQ - PQ*tan(20)*tan(35)
⇒ 230 tan(20) = PQ[1 - tan(20)*tan(35)]
\(PQ = \frac{230 tan(20)}{1 - tan(20)tan(35)}\)
\(= \frac{230*0.36}{1 - 0.36*0.7}\\\\= \frac{82.8}{1-0.25} \\\\=\frac{82.8}{0.75} \\\\= 110.4\)
PQ = 110.4
≈110
Height above sea level = 6500 + PQ
6500 + 110
= 6610
I would greatly appreciate if anyone could show me how to complete this problem.

Answers
Answer:
the best way to solve this is to have graph paper, so your numbers and points align. I have a model attached. the first point is hours slept 8. so on your graph go to 8 then across to 83, which is the test score. make a mark to represent this point. do this for all and it will create a line

PLEASE HELP ME IM SO DESPERATE

Answers
Two linear functions with point (1,4) are given by:
y = 2x + 2.y = -2x + 6.The graph with these two functions is given at the end of the answer.
What is a linear function?A linear function, in slope-intercept format, is modeled according to the rule presented below:
y = mx + b
In which the coefficients are described as follows:
The coefficient m is the slope of the function, which is the rate of change of the function, that is, the change in y divided by the change in x.The coefficient b is the y-intercept of the function, which is the the value of y when the function crosses the y-axis(x = 0).A line with slope of 2 is given by:
y = 2x + b.
Supposing that it goes through point (1,4), when x = 1, y = 4, hence we can find the intercept as follows:
4 = 2 + b
b = 2.
Hence the first equation is:
y = 2x + 2.
A line with slope of -2 is given by:
y = -2x + b.
Then the intercept is found as follows, supposing that it also goes through the point (1,4):
4 = -2 + b
b = 6.
Then the equation is:
y = -2x + 6.
More can be learned about linear functions at https://brainly.com/question/24808124
#SPJ1

Solve the following inequality: |2x+5|<2−x
Answers
Step-by-step explanation:
To solve the inequality |2x + 5| < 2 - x, we need to split it into two cases:
When 2x + 5 > 0:
In this case, the absolute value is positive, so we can simplify the inequality as follows:
2x + 5 < 2 - x
Expanding the left side:
3x + 5 < 2
Subtracting 5 from both sides:
3x < -3
Dividing both sides by 3:
x < -1
So the solution for this case is x < -1.
When 2x + 5 < 0:
In this case, the absolute value is negative, so we can simplify the inequality as follows:
(2x + 5) < 2 - x
Expanding the left side:
-2x - 5 < 2 - x
Adding x to both sides:
-2x - 5 + x < 2
Combining like terms:
-x - 5 < 2
Adding 5 to both sides:
-x < 7
Dividing both sides by -1:
x > -7
So the solution for this case is x > -7.
Taking the union of the two solutions, we get:
-7 < x < -1.
This means that x is between -7 and -1, excluding -7 and -1.
Turn 6/8 into a whole number and fraction
Answers
Simplest form-3/4
The nearest whole number is one because 0.75 rounds to one
6/8 can not be turned into a bigger number unless you are trying to reduce it which would be 3/4
A complex shape combined frim a square and rectangle b=6, c=8, and d=12 what is the area of this complex figure?
Answers
The area of the complex figure is 132 square units
How to determine the area
From the information given, we have that the composite shape is a combination of a:
SquareRectangleThe formula for area of a square is given as:
Area = a ²
Where 'a' is the length of it side
Area = 6²
Area of square = 36 square units
The formula for area of a rectangle is given as:
Area = width × length
Area = 8 × 12
Area of rectangle = 96 square units
Area of the complex figure = Area of square + area of rectangle
= 36 + 96
= 132 square units
Thus, the area of the complex figure is 132 square units
Learn more about area here:
https://brainly.com/question/25292087
#SPJ1
Square root of 202!!!!!!!!!!!!!! I need help
Answers
Answer:
approximately 14.2127 but it could also be simplified to the square root of 2 times the square root of 101
Two pieces of equipment were purchased for a total of $2000. If one piece cost $810 more than the other, find the price of the less expensive piece of equipment.
Answers
Answer:
595.
Step-by-step explanation:
Take $2000 and subtract $810 from it. You now have the total of the two pieces of equipment without the extra pricing. You should get $1,190. Then divide that number by 2. You will get $595.
1st Piece of Equipment = $595
2nd Piece of Equipment = $1,405
2nd Piece is $810 more expensive and the two pieces combined is $2,000.
Given the following, determine the set (B n C)'.
U= {x|x EN and x < 10}
B = {x|x EN and x is even and x< 10}
C = {x|x E N and x < 10}

Answers
Answer:
Step-by-step explanation:
U={x|x∈N and x<10}
or U={1,2,3,4,5,6,7,8,9}
B={x|x∈N and x is even and x<10}
or
B={2,4,6,8}
C={x|x∈N and x<10}
or
C={1,2,3,4,5,6,7,8,9}
(B∩C)={2,4,6,8}
(B∩C)'={1,3,5,7,9}
Solve the inequality and graph the solution on the line provided. 6x-6<-30
Answers
The solution to the inequality 6x - 6 < -30 is x < -4, and it is graphically represented as a closed circle at -4 and shading to the left of -4 on the number line.
To solve the inequality 6x - 6 < -30, we can follow these steps:
Step 1: Add 6 to both sides of the inequality to isolate the variable:
6x - 6 + 6 < -30 + 6
6x < -24
Step 2: Divide both sides of the inequality by 6 to solve for x:
(6x)/6 < (-24)/6
x < -4
The solution to the inequality is x < -4. This means that any value of x less than -4 will satisfy the inequality.
To graph the solution on the number line, we represent -4 as a closed circle (since it is not included in the solution) and shade the region to the left of -4 to indicate all values less than -4.
On the number line, mark a point at -4 with a closed circle:
<--------●-----------------
Then, shade the region to the left of -4:
<--------●================
The shaded region represents the solution to the inequality x < -4.
For more such question on inequality visit:
https://brainly.com/question/30238989
#SPJ8
Leslie leaves her house and drives to another city for her
sister's wedding. She drives 77 miles north and then 420
miles east. How far is Leslie from her house? HELP PLEASE
Answers
Answer:
Step-by-step explanation: You have to add the number
420
+77
497
Question 1 Part A (01.02, 01.03 MC): A boutique owner needs to sell $1,200 in clothing each week. This week, she is within $125 of
her goal.
Write an absolute value equation to represent the scenario. (2 points)
A. Ix+1,200 125
B. x 1,200|= 125
C. x + 125|= 1,200
D. |x-125 = 1,200

Answers
|x- 1,200|= 125 is an absolute value equation to represent the boutique owner goal.
What is meant by absolute value?Without taking into account direction, absolute value describes how far a number is from zero on the number line. A number's absolute value can never be negative.
Write an example of absolute value.The example for absolute value is |-9|=9.
|x- 1,200|= 125 is the correct representation to achieve her goal because it will result in rise of goal. Other options will give negative sign and that is impossible.
To learn more about absolute value visit the link:
https://brainly.com/question/1301718
#SPJ1
|x- 1,200|= 125 is an absolute value equation to represent the boutique owner goal.
What is meant by absolute value?
Absolute value is a term used in mathematics to refer to the magnitude or distance of a number from zero regardless of its sign. In other words, it is the numerical distance of a number from zero on the number line, regardless of whether it is a positive or negative number. For example, the absolute value of -3 is 3, and the absolute value of 3 is also 3. The symbol for absolute value is two vertical lines surrounding the number or expression, for example |-3| = 3.
Write an example of absolute value.
The example for absolute value is |-9|=9.
|x- 1,200|= 125 is the correct representation to achieve her goal because it will result in rise of goal. Other options will give negative sign and that is imposible.
To learn more about absolute value visit the link:
https://brainly.com/question/24368848
#SPJ1
9. Joe goes to the store with $15 to buy salad. Bags
of pre-washed salad are on sale for $2 each. What
are the possible numbers of bags Joe can buy?
Answers
Answer:
7
Step-by-step explanation:
$15 to buy salad and $2 for each bag. You need to find how many times 2 goes into 15, and evenly it goes 7 times so, therefore, 7 x 2 = 14 with $1 remainder.
Question regarding a Normal Distribution chart: Ten thousand adults were given a literacy test. The results were nearly normally distributed (μ = 75, σ = 15).
A. About how many scored between 60 and 80?
B. Approximately what score did only the top 15% exceed?
C. Find the percentile rank of the person who scored 88.
Answers
Answer:
a) 4,706 scored between 60 and 80
b) A score of 90.6.
c) 81th percentile.
Step-by-step explanation:
When the distribution is normal, we use the z-score formula.
In a set with mean \(\mu\) and standard deviation \(\sigma\), the zscore of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.
In this question, we have that:
\(\mu = 75, \sigma = 15\)
A. About how many scored between 60 and 80?
Proportion:
This is the pvalue of Z when X = 80 subtracted by the pvalue of Z when X = 60. So
X = 80
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{80 - 75}{15}\)
\(Z = 0.33\)
\(Z = 0.33\) has a pvalue of 0.6293
X = 60
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{60 - 75}{15}\)
\(Z = -1\)
\(Z = -1\) has a pvalue of 0.1587
0.6293 - 0.1587 = 0.4706
How many?
0.4706 out of 10,000, so
0.4706*10000 = 4706
4,706 scored between 60 and 80.
B. Approximately what score did only the top 15% exceed?
The 85th percentile, which is X when Z has a pvalue of 0.85. So X when Z = 1.037.
\(Z = \frac{X - \mu}{\sigma}\)
\(1.037 = \frac{X - 75}{15}\)
\(X - 75 = 1.037*15\)
\(X = 90.6\)
A score of 90.6.
C. Find the percentile rank of the person who scored 88.
This is the pvalue of Z when X = 88. So
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{88 - 75}{15}\)
\(Z = 0.87\)
\(Z = 0.87\) has a pvalue of 0.8078
So, rounding up, the 81th percentile.
It takes a bear 9 more days to eat a barrel of honey alone than it takes the bear and a second bear to eat the barrel of honey together. It takes the first bear 7 fewer days to eat a barrel of honey alone than it takes the second bear to eat a barrel of honey alone. In how many days can the two bears eat a barrel of honey together?
Answers
Answer:
it takes the bears 12 days to eat honey together.
Step-by-step explanation:
1/x+9+1/x+16=1/x
(solve this using cross multiplication)
= x^2 = 144
x=12
The number of days that it takes for both of the considered bears to eat a barrel of honey together is 12 days.
How are number of people to time needed to complete a task related?More people to do a task means less time it will take.
Less people to do a task means more time it will take.
Thus, they are inversely related.
We can define a constant as "Manpower" needed for doing that specific work.
Each worker has his/her own strength. That strength is applied per unit of time.
Let we define:
Manpower needed for a work = time taken for that work × strength used for that work per unit of time
(we used multiplication as it represents linear use of strength to finish the task, so using that strength for a finite units of time means you stacked up your strengths that many times to finish that work)
Here, we have:
Instead of people, here we consider bears.Work in consideration = eating honey out of a considered barrel (assuming all barrels considered will have same amount of honey)Assume that:
Strength of first bear (call it bear A) for eating honey = S per day (as unit of time is a day here).Strength of second bear (call it bear B) for eating honey = T per dayManpower the work needs be M (assume)Time needed by bear A to complete the work = 'x' daysTime needed by bear B to complete the work = 'y' days.Then, we get:
Manpower needed for a work = time taken for that work × strength used for that work per unit of time
\(M = x \times S\\M = y \times T\)
If bear A and bear B both do the work together, then their strenghts will add up.
That means, the combined strength would be S + T per day
The manpower the work needs is still M, therefore, the time needed is:
\(M = \text{Time needed} \times (S + T)\\\\\text{Time needed} = \dfrac{M}{S+T}\)
This is the time needed for both bears to do the work together.
Also, it is given that:
It takes a bear 9 more days to eat a barrel of honey alone than it takes the bear and a second bear to eat the barrel of honey together.
or
Time needed by bear A to do work = 9 + time needed by both bears to do the work
or
\(x = 9 + \dfrac{M}{S + T}\)
Also, it is given that:
It takes the first bear 7 fewer days to eat a barrel of honey alone than it takes the second bear to eat a barrel of honey alone.
or
Time taken by bear A to do the work = Time taken by bear B for that work - 7 days
or
\(x = y - 7\)
Thus, we got these equations:
\(M = S \times x\\M = T \times y\\\\x = \dfrac{M}{S + T} +9\\\\x = y - 7\)
call them first, second, third and fourth equation as per their ordering.
From the first and second equation, we get:
\(S = \dfrac{M}{x}\\\\T = \dfrac{M}{y}\)
Putting these values in the third equation, we get:
\(x = \dfrac{M}{M/x + M/y} + 9\\\\\\x = \dfrac{xy}{x+y} + 9\\\\(x-9)(x+y) = xy\\x^2 -9x -9y + xy = xy\\x^2 -9x = 9y\)
From the fourth equation, we know that:
\(x = y - 7\)
or \(y = x +7\)
Putting this value of y in the equation \(x^2 -9x = 9y\), we get:
\(x^2 -9x = 9(x+7)\\x^2 -9x = 9x + 63\\x^2 -18x -63 =0\\\\x^2 -21x + 3x -63 = 0\\x(x-21) + 3(x - 21) = 0\\(x+3)(x-21) = 0\\(x+3) = 0, (x-21) = 0\\x = -3, x = 21\)
'x' represents time in number of days. So it cannot be negative.
Thus, the value of x obtained is 21.
Also, we need: Time needed for both the bears to do the work together.
This time is the value of \(\dfrac{M}{S+T}\), which is equal to \(x - 9\) days (from the third equation)
Thus, the time needed for both the bears to do the work together = \(x-9 = 21-9 = 12 \; \rm days\)
Thus, the number of days that it takes for both of the considered bears to eat a barrel of honey together is 12 days.
Learn more about work and time here:
https://brainly.com/question/1063042
#SPJ2
What is the factor to dilate triangle ABC to get A'B'C'? (Write your answer below. Show your work for partial credit.)

Answers
Answer:
The Factor is 1.5
Step-by-step explanation:
5.1(which is B'C') divided by 3.4(which is BC) = 1.5
3.6(A'C') divided by divided by 2.4(AC) = 1.5
2.7(A'B') divided by 1.8(AB) = 1.5
Henceforth the factor is 1.5
Write the following numbers in order, putting the largest
first:
a)
31 009
31.53
31. 099
b)
0.007
0.03
0.009
Answers
Answer:
a)
31.53
31.099
31.009 ⬅ (I'm assuming this one is a decimal too)
b)
0.03
0.009
0.007
Explanation:
Here ya go.
Hope this helps :)
5.5 + n = 10 please help
Answers
Answer:
n = 4.5
Step-by-step explanation:
10 - 5.5 = n
Answer:
I feel like its 4.5
Step-by-step explanation:
Find an equation of the line in slope-intercept form that passes through the point (5, -3) and is perpendicular to the line that passes through the points (1,5) and (2, 3).
Answers
Answer: y = 2x + 7
Step-by-step explanation:
Given line = -1/2
given line = y = -1/2x + 7
Perp line = y = 2x + 7
PLEASE HELP
WILL MARK BRAINLY
1. The population in Small town in 2010 was 45,230 people and is growing exponentially at a rate of 2 percent. Which of the following equations
defines the population t years after 2010?
O P= 45, 2302
OP=45, 230(1+0-2)*
OP=45, 2300
OP=45, 230(1 +0.02)
Answers
Answer:
C
Step-by-step explanation:
The following equation defines the population t years after 2010,
P = 45230(1 + 0.02\()^{t}\).
What is Exponential Growth?An exponential function's curve is created by a pattern of data called exponential growth, which exhibits higher increases over time.
y = a(1+r)ˣ,
where a is the initial population and r is the rate in decimals and sx is the time period.
Given:
The population in Small town in 2010 was 45,230 people and is growing exponentially at a rate of 2 percent.
The formula;
y = a(1+r)ˣ,
where a is the initial population and r is the rate in decimals and x is the time period.
That means,
P = 45230(1 + 0.02\()^{t}\)
Therefore, the equation is P = 45230(1 + 0.02\()^{t}\)
To learn more about the exponential growth;
https://brainly.com/question/12490064
#SPJ2
What is the solution to the equation 1 over 5 multiplied by n equals 3? n = 8 n = 15 n = 25 n = 125
70 PTS!
Answers
Solution:
Note that:
Given equation: 1/5 x n = 3Simplify the LHS, then use cross multiplication to solve for n.
1/5 x n = 3=> n/5 = 3=> n = 3 x 5=> n = 15Answer:
n = 15
Step-by-step explanation:
\(\dfrac{1}{5}n=3\)
Multiply both sides by 5:
\(\implies n = 3 \times 5 = 15\)
answer these 2 qns if possible or atleast one

Answers
1. The percentage of maize flour is given as 16.7 %
2. The common ratio of the GP is r = 1.5.
How to solve for the common ratio1. We have to set up equation
60x + 90(1 - x) = 85
60x + 90 - 90x = 85
now we have to solve for the value of x
-30x = -5
x = 5 / 30
x = 0.167
Hence percentage of maize flour is given as 16.7 %
2. a + ar = 20 (1)
ar + ar^2 = 30 (2)
We can rearrange equation (1) to get an expression for a:
a = 20 / (1 + r)
Now, we can substitute a into equation (2) to get an equation only in terms of r:
\(20r / (1 + r) + 20r^2 / (1 + r) = 30\\20r + 20r^2 = 30(1 + r)\\20r + 20r^2 = 30 + 30r\\20r^2 - 10r - 30 = 0\\2r^2 - r - 3 = 0\)
Solving this quadratic equation, we get:
We have two solutions, r = 1.5 and r = -1. But since the GP is increasing, we discard the negative solution. Therefore, the common ratio of the GP is r = 1.5.
Read more on common ratio here:https://brainly.com/question/1509142
#SPJ1
PLEASE HELP I'M DESPERATE
Answer the question below.
Solve the equation -22 = q + 7(2q - 1)
and verify the solution. Show all work.
Answers
The solution of the equation -22 = q + 7(2q - 1) is as follows:
q = -1How to solve equation?Equations are mathematical statements containing two algebraic expressions on both sides of an 'equal to (=)' sign.
Let's solve the equations as follows:
-22 = q + 7(2q - 1)
The variable in the equation is q. A variable is an letter use to represent number in an equation.
-22 = q + 7(2q - 1)
-22 = q + 14q - 7
-22 = 15q - 7
add 7 to both sides of the equation
-22 + 7 = 15q - 7 + 7
-15 = 15q
divide both sides by 15
q = -15 / 15
q = -1
Therefore,
q = -1
learn more on equation here: https://brainly.com/question/13591596
#SPJ1
Answer:
-22 = q + 7(2q - 1)
-22 = q + 14q - 7
-22 = 15q - 7
add 7 to both sides of the equation
-22 + 7 = 15q - 7 + 7
-15 = 15q
divide both sides by 15
q = -15 / 15
q = -1
Step-by-step explanation:
The graphs below have the same shape. What is the equation of the graph of g(x)? g(x) V -5 5 O g( A. g(x) = x2 - 2 B. g(x) = (x - 2)2 -(y22

Answers
Answer:
I believe it’s b
Step-by-step explanation:
Answer:
g(x)=(x+2)^2
Step-by-step explanation:
can someone help me with this ?

Answers
Step-by-step explanation:
-coefficent=1
-collect like terms /variables
-add numbbers (1+2)=3p

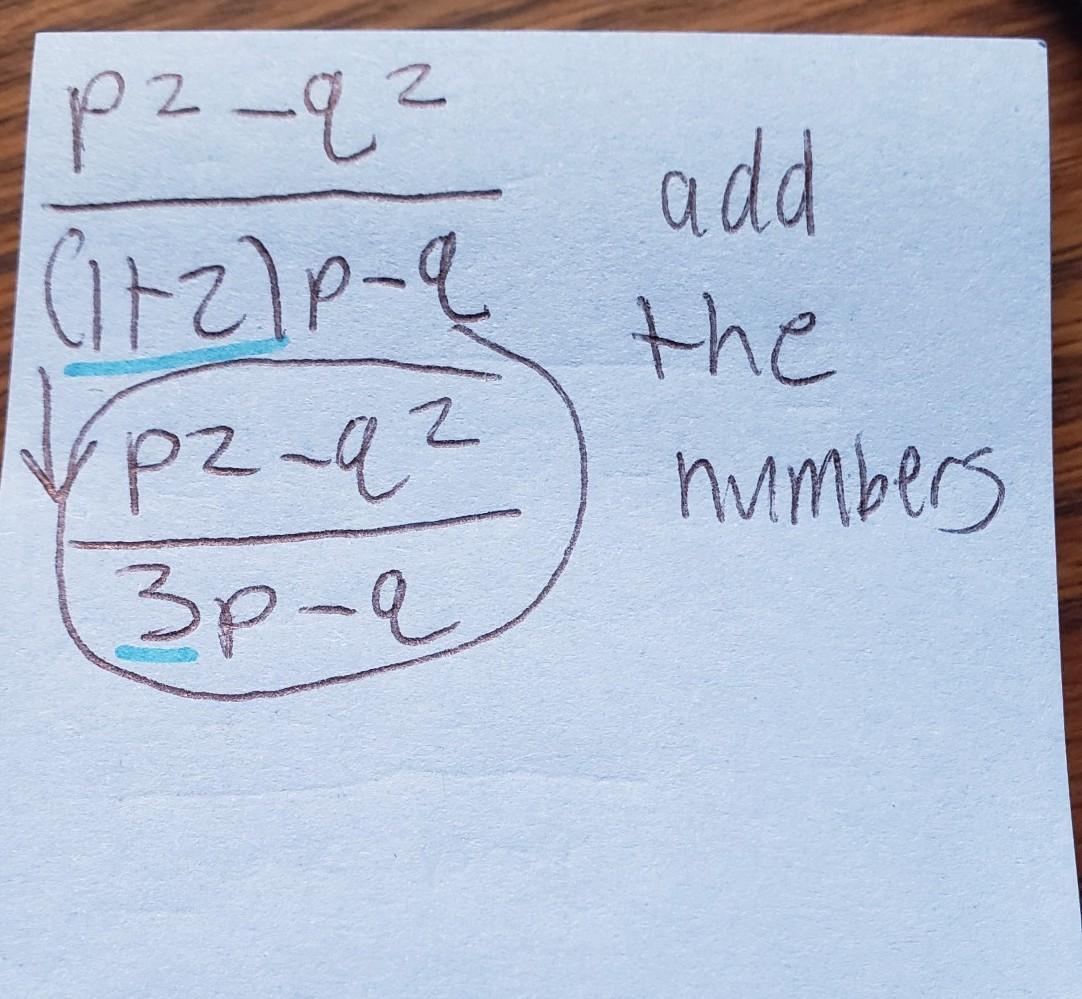
Answer:
1
Step-by-step explanation:
(p+q)(p-q)/(p+q)(p-q)=1
[It is an identity : a²-b²= (a+b)(a-b)]
Hope this will help:)
20. Find the unknown angle measures.
51°
53°

Answers
The unknown angle measures are d = 76, c = 53, a = 51 and b = 76
Finding the unknown angle measures.From the question, we have the following parameters that can be used in our computation:
The lines
The sum of angles on a line is 180
So, we have
b = 180 - 51 - 53
b = 76
By vertical angle theorems, we have
d = 76
c = 53
a = 51
Read more about angles at
https://brainly.com/question/31898235
#SPJ1
How many solutions does this system have? no solutions one unique solution O O two solutions O or an infinite number of solutions

Answers
Answer:
no solutions
Step-by-step explanation:
the equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
equation of blue line is y = x + 2 , in slope- intercept form
with slope m = 1
equation of red line is y = x - 3 , in slope- intercept form
with slope m = 1
• Parallel lines have equal slopes
then the blue and red lines are parallel.
the solution to the system is at the point of intersection of the 2 lines
since the lines are parallel then they do not intersect each other.
thus the system shown has no solution.