Rewrite the inequality with zero on one side and one rational expression on teh other. 3/x+2+4>3 what is the first step
Answers
Answer:
Add –3 to both sides
Step-by-step explanation:
edge 2021
Related Questions
Find the equation of the curve that satisfies dy/dx=4x3yand whose y-intercept is 7.
Answers
The equation of the curve that satisfies $dy/dx=4x^3y$ and whose y-intercept is 7 is $y=7e^{x^4}$.Answer: $y=7e^{x^4}$.
The given differential equation is: $dy/dx=4x^3y$.We are to find the equation of the curve that satisfies the given differential equation, and whose y-intercept is 7. We can use separation of variables to solve the differential equation as follows:\[\frac{dy}{dx}=4x^3y\]Dividing both sides by y gives:\[\frac{1}{y} \frac{dy}{dx}=4x^3\]Integrating both sides with respect to x gives:\[\int \frac{1}{y} \frac{dy}{dx} dx=\int 4x^3 dx\]\[\int \frac{1}{y} dy=\int 4x^3 dx\]Evaluating the integrals gives:\[\ln |y|=\frac{x^4}{1}+c_1\]Where $c_1$ is the constant of integration.
To solve for y, we exponentiate both sides as follows:\[|y|=e^{\frac{x^4}{1}+c_1}\]The y-intercept is given as 7, and we know that the y-intercept occurs at the point (0,7). We can use this fact to solve for the constant of integration $c_1$ as follows:\[|7|=e^{\frac{0^4}{1}+c_1}\]Taking the natural logarithm of both sides gives:\[\ln 7=\frac{0^4}{1}+c_1\]Therefore, $c_1=\ln 7$, and the equation of the curve that satisfies the given differential equation, and whose y-intercept is 7 is:\[y=e^{\frac{x^4}{1}+\ln 7}=7e^{x^4}\]
Hence, the equation of the curve that satisfies $dy/dx=4x^3y$ and whose y-intercept is 7 is $y=7e^{x^4}$.Answer: $y=7e^{x^4}$.
Learn more about Equation
brainly.com/question/29538993
#SPJ4
f(x) = ln(2 + sin(x)), 0 ≤ x ≤ 2. Find the interval(s) on which f is concave up. (Enter your answer using interval notation.).
Answers
To determine where the function f(x) = ln(2 + sin(x)) is concave up, we need to find the second derivative of the function and then determine where the second derivative is positive.
First, we find the first derivative of f(x):
f'(x) = cos(x) / (2 + sin(x))
Then, we find the second derivative of f(x):
f''(x) = [(-sin(x))(2 + sin(x)) - (cos(x))^2] / (2 + sin(x))^2
Simplifying this expression, we get:
f''(x) = [-sin(x)^2 - cos(x)^2 - 2sin(x)cos(x)] / (2 + sin(x))^2
f''(x) = [-1 - sin(2x)] / (2 + sin(x))^2
Now, to find where f''(x) is positive, we need to solve the inequality:
f''(x) > 0
[-1 - sin(2x)] / (2 + sin(x))^2 > 0
The denominator is always positive, so we only need to consider the numerator. We can solve the inequality by considering two cases:
Case 1: -1 < sin(2x) < 0
In this case, the numerator is negative, so the inequality cannot hold. Therefore, there are no solutions in this case.
Case 2: sin(2x) < -1
In this case, sin(2x) is negative and less than -1, which means that 2x is in the third or fourth quadrant. The solutions are given by:
π/2 < x < 3π/4
5π/2 < x < 11π/4
Note that these intervals are within the given domain of the function, 0 ≤ x ≤ 2.
Therefore, the interval on which f(x) = ln(2 + sin(x)) is concave up is:
(π/2, 3π/4) U (5π/2, 11π/4)
To know more about interval notation, visit the link given below:
https://brainly.com/question/29531272
#SPJ4
APH4: Find the 1 st and 2 nd derivatives of \( Q=x^{4} e^{3 x} \)
Answers
the first derivative of\(\( Q=x^{4} e^{3x} \) is \( Q' = 4x^{3}e^{3x} + 3x^{4}e^{3x} \)\), and the second derivative is \(\( Q'' = 12x^{2}e^{3x} + 24x^{3}e^{3x} + 9x^{4}e^{3x} \)\).
To find the first and second derivatives of \(\( Q=x^{4} e^{3x} \),\) we will use the product rule and chain rule. Let's start with the first derivative. 1. Use the product rule: \(\[ Q' = (x^{4})' \cdot e^{3x} + x^{4} \cdot (e^{3x})' \]\)
Simplify: \(\[ Q' = 4x^{3} \cdot e^{3x} + x^{4} \cdot 3e^{3x} \] [Q' = 4x^{3}e^{3x} + 3x^{4}e^{3x} \]\)
Now, let's find the second derivative. 2. Use the product rule again:
\(\[ Q'' = (4x^{3}e^{3x})' + (3x^{4}e^{3x})' \]\)
Simplify: \(\[ Q'' = (12x^{2}e^{3x} + 4x^{3} \cdot 3e^{3x}) + (12x^{3}e^{3x} + 3x^{4} \cdot 3e^{3x}) \] \\ Q'' = 12x^{2}e^{3x} + 12x^{3}e^{3x} + 12x^{3}e^{3x} + 9x^{4}e^{3x} \] \\\ Q'' = 12x^{2}e^{3x} + 24x^{3}e^{3x} + 9x^{4}e^{3x} \]\)
To know more about derivative visit:
brainly.com/question/29144258
#SPJ11
A pair of jeans at a local retail store has a cost of $32.00. If the sales tax is 5.3%, what is the total amount paid for the jeans?
Answers
The total amount that a customer will pay for the jeans is $33.696.
How to calculate the value?From the information given, pair of jeans at a local retail store has a cost of $32.00. If the sales tax is 5.3%,
The value of the sales tax will be:
= Sales percentage × Amount
= 5.3% × $32
= $1.696
The total amount paid will be:
= Cost + Tax
= $32 + $1.696
= $33.696
The amount is $33.696.
Learn more about percentages on;
brainly.com/question/24877689
#SPJ1
2.6 + 0.9 show work
Answers
Answer:
3.5 is your answer.
Step-by-step explanation:
2.6
0.9+
------
3.5
Answer
3.5
Explanation
2.6 + 0.9
3.5
Good Luck ^-^

Tony is solving the equation 4x = 12x + 20 for x. Tony uses the multiplicative property of equality to rewrite the equation as x = 3x + 20. Which statement correctly explains whether
A Tony used the property correctly? Tony used the property correctly because he multiplied one term on each side of the equals sign by 1/4
B Tony did not use the property correctly because he should have multiplied both sides of the equals sign by 1/12 not 1/4
C Tony did not use the property correctly because he did not multiply every term on both sides of the equals sign by 1/4
D Tony used the property correctly because he multiplied every term containing x by 1/4
Answers
Answer:
C)
Step-by-step explanation:
The correct answer is C: Tony did not use the property correctly because he did not multiply every term on both sides of the equals sign by 1/4.
To solve the equation 4x = 12x + 20, Tony used the multiplicative property of equality but made an error in the application. The correct approach would be to multiply every term on both sides of the equals sign by the reciprocal of the coefficient of x, which is 1/4 in this case.
However, Tony only multiplied one term on each side by 1/4, resulting in equation x = 3x + 20. This action is incorrect because it does not apply the property to every term containing x. To solve the equation correctly, Tony should have multiplied both sides by 1/4, resulting in x/4 = (3x + 20)/4.
Which statement is correct?
N
H
#
w
T
А.
ATNW - AHCL
В.
ANWT ACHL
C
ANWT 2 ALCH
D
AWTNAHCL

Answers
Answer:
b
Step-by-step explanation:
n hc l
a n w t
yan lng po Ang Alam k
How many lines of symmetry does the figure have?
A. 0
B. 1
C. 2

Answers
Answer:
The answer is A, so zero.
Please help. This is geometry.

Answers
Answer:
ASA
Step-by-step explanation:
We know angle KLM=angle KLNWe know KL=KLWe know angle MKL=angle NKLHELP NEEDED !!!
find the unknown angles

Answers
Answer:
x = 90
y = 45
Since it's a square the corners are = 90.
To find y the 90 is halved therefore it is = 45.
Please solve these questions




Answers
Answer:
1.
1. Same side exterior angles
2. They are supplementary angles
3. VUW = 80
2. x = 40
3. x = 6
4. x = 8
Step-by-step explanation:
1.
1.
Same side exterior angles
2.
They are supplementary angles
3.
100 + 100 = 200
360 - 200 = 160
160 / 2 = 80
2.
110 = 3x - 10
110 + 10 = 3x
120 = 3x
120 / 3 = x
x = 40
3.
115 + 115 = 230
360 - 230 = 130
130 / 2 = 65
65 = 8x + 17
65 - 17 = 8x
48 = 8x
48 / 8 = x
x = 6
4.
102 + 102 = 204
360 - 204 = 156
156 / 2 = 78
78 = 10x - 2
78 + 2 = 10x
80 = 10x
80 / 10 = x
x = 8
√126 rational or irrational
Answers
Answer:
Rational
Step-by-step explanation:
meredith is a general surgeon who performs surgeries such as appendectomies and laparoscopic cholecystectomies. the average number of sutures that meredith uses to close a patient is 37, and the standard deviation is 8. the distribution of number of sutures is right skewed. random samples of 32 are drawn from meredith's patient population, and the number of sutures used to close each patient is noted. use the central limit theorem to find the mean and standard error of the sampling distribution. select the statement that describes the shape of the sampling distribution. group of answer choices unknown the sampling distribution is normally distributed with a mean of 37 and standard deviation 1.41. the sampling distribution is right skewed with a mean of 37 and standard deviation 8. the sampling distribution is normally distributed with a mean of 37 and standard deviation 8. the sampling distribution is right skewed with a mean of 37 and standard deviation 1.41.
Answers
The statement that accurately describes the form of the sampling distribution is:The inspecting dissemination is regularly circulated with a mean of 37 and standard deviation 1.41.
According to the central limit theorem, regardless of how the population distribution is shaped, the sampling distribution of the sample mean will be approximately normally distributed for a sufficiently large sample size.
For this situation, irregular examples of 32 are drawn from Meredith's patient populace, which fulfills the state of a sufficiently huge example size. The central limit theorem can be used to determine the sampling distribution's mean and standard error.
In this instance, the population mean, which is 37, is equal to the mean of the sampling distribution.
The population standard deviation divided by the square root of the sample size is the sampling distribution's standard error. For this situation, the standard mistake is 8 partitioned by the square foundation of 32, which is around 1.41.
Therefore, the statement that accurately describes the form of the sampling distribution is:
The inspecting dissemination is regularly circulated with a mean of 37 and standard deviation 1.41.
To know more about central limit theorem refer to
https://brainly.com/question/898534
#SPJ11
BEDMAS QUESTIONS
Solve the following.
Show your work.
Round you asnwers to the nearest hundredth

Answers
Using BODMAS, the solutions of the expressions are: (1) 9, (2)-12, (3) 0.27, and (4) 0.24
What is BODMAS?We should know that BODMAS is a mathematical approach which means
B=BracketO=OffD=DivisionM=MultiplicationA=Addition S=SubtractionIn each of the expressions, we must follow the approach
1) \(\frac{(6+3)^{2} }{3*9}\)
Simplifying this we have 9²/9=81/9
=9
2) \(\frac{3^{2} -6*2}{(12-8)/2^{2} *10}\)
Using BODMAS we have
(9-12)/4/40
-3÷1/9
⇒-3*4
=-12
(3) \(\frac{4.5+2*2.5^{2}-6 }{8*4+9}\)
Using BODMAS we have
(4.5+2*6.25-6)/32+9
⇒(4.5+12.5-6)/41
=11/41=0.27
4 (\(\frac{3.5+16-2.5^{2} }{10.5-6*11}\))
Using BODMAS we have
(3.5+16-6.25)/10.5-66
Simplifying
(13.25)/-55.5
=0.24
Therefore, the values when simplified are (1) 9, (2)-12, (3) 0.27, and (4) 0.24
Learn more about BODMAS on https://brainly.com/question/29626866
#SPJ1
In 2019, the average full-time worker in the U.S. spent `8.5` hours working each workday. This is an increase on the average of `8.1` hours per workday in 2014. What is the percent change from 2014 to 2019? Round to the nearest tenth of a percent.
Answers
Answer 2019 /0.0047 /2014/ 0.493
Step-by-step explanation:
The percent change in average full-time worker in the U.S from 2014 to 2019 is 4.93%.
What is Percent Change?A percentage is a portion of a whole that is stated as a number between 0 and 100 as opposed to being expressed as a fraction.
The percentages of everything are 100%, nothing is 0%, 50% of something is 50%, and nothing is 0%.
Given:
In 2019, the average full-time worker in the U.S. spent `8.5` hours working each workday.
This is an increase on the average of `8.1` hours per workday in 2014.
So, Percent change = (Final value - initial value) / initial value x 100
Percent change = 8.5 - 8.1 / 8.1 x 100
Percent change = 4.93%
Thus, the percent change in average full-time worker in the U.S from 2014 to 2019 is 4.93%.
Learn more about the percent change here:
brainly.com/question/809966
#SPJ2
The radii of the two spheres are 2cm and 5cm.
The ratio of their volumes and surface areas are?
Answers
Answer:
1
Step-by-step explanation:
You have two groups of 5 adults. In group 1, their ages are 35, 55, 75, 95, and 105. In group 2, their ages are 60, 61, 62, 63, and 64. Which group has a larger standard deviation
Answers
Group 1 has a larger standard deviation of 25.6 than Group 2 which has a standard deviation of 1.4.
Standard deviation is a statistical measure that calculates how far away each number in a set is from the mean and thus from every other number in the set.
Standard deviation is calculated as the square root of variance, where variance is the average of squared differences from the mean.
Let's compute the standard deviation of the two groups.
Group 1 has a mean of (35+55+75+95+105)/5=73
Age deviations from the mean (in years) are: -38, -18, +2, +22, +32
Squared deviations from the mean are: 1444, 324, 4, 484, 1024
Variance = (1444+324+4+484+1024)/5 = 654
Standard deviation = √(654) = 25.6
Group 2 has a mean of (60+61+62+63+64)/5=62.
Age deviations from the mean are: -2, -1, 0, +1, +2
Squared deviations from the mean are: 4, 1, 0, 1, 4
Variance = (4+1+0+1+4)/5 = 2
Standard deviation = √(2) = 1.4
Therefore, Group 1 has a larger standard deviation of 25.6 than Group 2 which has a standard deviation of 1.4.
To learn about standard deviation here:
https://brainly.com/question/24298037
#SPJ11
Find the slope of the line.
1/3
-1/3
3
-3
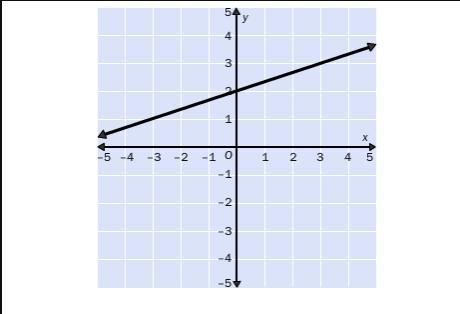
Answers
Answer:
if a line is like so it has a positive slope: / . IF a line is like this it has a negative: \
Therefore that leaves out -1/3 and -3
Either A or C is correct
From the y intercept (where the line intercepts the y axis), in this case 2, to the next point on the line (3, 3) it goes up 1 and over 3 to get to that point from the intercept. Therefore the slope is 1/3. (Rise/run)
1/3
Step-by-step explanation:
Of the children in Russell's class, 10 have a blue biock and 7 have a green block:4 children have both a blue block and a green block. How many children have a blue black but not a green block
Answers
Answer:
10 have blue 7 have green blocks and 4 have a blue and green block. but only 10 have just a blue block
evaluate the integral. 4) ∫ -8x cos 6x dx
Answers
The solution of the integral ∫ -8x cos 6x dx is (-4/3)xsin(6x) - (2/9)cos(6x) + C
To evaluate the integral ∫ -8x cos 6x dx, we will use integration by parts, which involves the formula
∫u dv = uv - ∫v du, where u and dv are functions of x.
We need to follow this steps-
Step 1: Choose u and dv
Let u = -8x and dv = cos(6x) dx.
Step 2: Differentiate u and integrate dv
Differentiate u with respect to x to get du: du = -8 dx.
Integrate dv with respect to x to get v:
v = ∫cos(6x) dx = (1/6)sin(6x).
Step 3: Apply the integration by parts formula
∫ -8x cos 6x dx = uv - ∫v du = (-8x)(1/6)sin(6x) - ∫(1/6)sin(6x)(-8) dx
Step 4: Simplify the expression and integrate
= (-4/3)xsin(6x) + (4/3)∫sin(6x) dx
Now,we integrate sin(6x) with respect to x:
∫sin(6x) dx = (-1/6)cos(6x)
Step 5: Substitute the integral back into the expression
= (-4/3)xsin(6x) + (4/3)(-1/6)cos(6x) + C
Step 6: Simplify the expression and include the constant of integration
= (-4/3)xsin(6x) - (2/9)cos(6x) + C
So, the evaluated integral is ∫ -8x cos 6x dx = (-4/3)xsin(6x) - (2/9)cos(6x) + C.
Learn more about trigonometric ratios : https://brainly.com/question/12172664
#SPJ11
A rental car company charges $45 plus $0.20 per mile to rent a car. Mr. Lawrence does not want to spend more than $100 for his rental car. Write and solve an inequality to find how many miles he can drive and not spend more than $100. Interpret the solution.
STEP ONE: write the correct inequality (1 point)
HINT: The charge from the car company and the cost per mile must be no more than $100.
STEP TWO: solve the inequality to find the correct solution set (2 points)
HINT: Remember to undo addition or subtraction first.
STEP THREE: interpreting the solution (in other words, tell what it means/represents/stands for)
FOR EXAMPLE: "The solution means that Jennifer must work 50 or more hours to make the money she needs to buy a new dress.
Answers
Answer: 45+.20m≤ 100
Step-by-step explanation:100-45=55/.20=275
So he should drive no more than 275 miles.
equivalent ratio to 2:5
Answers
Answer:
4:10
8:20
16:40
1:2.5
Step-by-step explanation:
Use the slope formula to find the missing coordinate.
7
m = T(13, 2), W_,-5)
a) -11
b) 11
c) 15
Answers
Answer:
C
Step-by-step explanation:
Given the equations of two lines, describe how to determine if the lines are parallel.
Answers
Answer:
For two linear equations : a₁x + b₁y + c₁ = 0 and a₂x + b₂y + c₂ = 0;
To determine if two such straight lines are parallel, then you should check for the following conditions :
a₁/a₂ = b₁/b₂ ≠ c₁/c₂ , where a,b are coefficients of x and y for each line.
if this condition is true for the coefficients of x,y, and the intercept of lines (c₁ and c₂) then they are parallel.
for instance,
let's say we have two lines :
x + 2y - 4 = 0,
2x + 4y - 12 = 0;
Now to check if they are parallel you simply check if the condition for parallel lines is met or not.
for these two lines :
a₁ = 1 , b₁ = 2, and c₁ = -4;
a₂ = 2, b₂ = 4, and c₂ = -12;
evaluating the values in the condition we have :
\(\frac{1}{2}\) = \(\frac{2}{4}\) ≠\(\frac{-4}{-12}\) ;
since the condition for parallel is true for these two lines, we can conclude they are parallel.
Find two numbers whose sum is 49. If the greater is 4 more than 8 times the smaller. Solve
Answers
I got
44
and
5
Explanation:
Call the numbers
x
and
y
, you get:
x
+
y
=
49
x
=
8
y
+
4
substitute the second equation into the first:
8
y
+
4
+
y
=
49
9
y
=
45
y
=
45
9
=
5
so that:
x
=
8
⋅
5
+
4
=
44
Answer:
5 and 44
Step-by-step explanation:
smaller number: x
greater number: 8x + 4
x + 8x + 4 = 49
9x + 4 = 49
9x = 45
x = 5
smaller number: 5
greater number: ?
8(5) + 4 = 40 + 4 = 44
5 + 44 = 49
Hope that helps.
You opened a bank account at the beginning of the month and have added the same amount of money each day. On the 5th day, you have $80. On the 21st day, you have $160. How much did you originally open the account with? How much are you putting in the account each day? How much will you have on the 30th day of the month?
Answers
We are given:
Money on the fifth day (a₅) = $80
Money on day 21 (a₂₁) = $160
To Find:
Money in the bank on the first day (a₁) = ?
Money being added to the account per day (d) = ?
Money in the bank on day 30 (a₃₀) = ?
__________________________________________________________
Finding the money on day one and the money added:
Solving for equations
Since we are adding a constant amount to our account every day, the amount of money in the bank can be represented by an AP
We know that the formula for the nth term of an AP is:
aₙ = a₁ + (n-1)d
So, we can write the amount of money on day 5 as:
a₅ = a₁ + (5-1)d
a₅ = a₁ + 4d
80 = a₁+ 4d [We are given that a₅ = 80]
We are also given the amount of money on day 21:
a₂₁ = $160
Money on day 21 can be rewritten as:
a₂₁ = a₁ + (21-1)d
160 = a₁ + 20d [Since a₂₁ = 160]
Solving the equations:
Now we have 2 equations. We will now solve them to get the values of a₁ and d
160 = a₁ + 20d ----------------------(1)
80 = a₁ + 4d--------------------------(2)
From equation (2), we can say that:
a₁ = 80 - 4d [subtracting 4d from both sides]
Now, we will use this value of a₁ in the equation (1):
160 = a₁ + 20d
160 = (80-4d) + 20d
160 = 80 + 16d
80 = 16d [subtracting 80 from both sides]
d = 5 [dividing both sides by 16]
Now that we know the value of d, we can use it in any of the 2 equations:
80 = a₁ + 4d
80 = a₁ + 4(5) [since d=5]
80 = a₁ + 20
a₁ = 60 [subtracting 20 from both sides]
Now, we know the values of the initial amount of money and the money added to the account everyday
Initial money in the bank = $60
Money added everyday = $5
__________________________________________________________
Money in day 30:
We will use the AP formula to find the value of money on day 30
a₃₀ = a₁ + (30-1)d
We know that a₁ = $60 , d = $5
a₃₀ = 60 + 29(5)
a₃₀ = 60 + 145
a₃₀ = $205
Money on day 30 = $205
Answer:
started with $55
adds $5 each day
$205 on the 30th day
Step-by-step explanation:
21-5 = 16
160-80 = 80
80 divided by 16 = 5
5 * 5 = 25
80 - 25 = 55
9 * 5 = 45
160 + 45 = 205
what is the decimal value of the following binary number? 10011101
Answers
The following binary number has the value of 157 in decimal form.
How can a binary number be converted to a decimal?Step 1: Compose the binary number in writing. 10011101
Multiplying each binary digit by the corresponding power of two in step two:
1x27 + 0x26 + 0x25 + 1x24 + 1x23 + 1x22 + 0x21 + 1x20
Solve the powers in Step 3:
1x128+0x64+0x32+1x16+8+4+0x2+1x1=128+0 + 0 + 0 + 16+8+4+0 + 0 + 1
Step 4: Add the figures listed above:
128 + 0 + 0 + 16 + 8 + 4 + 0 + 1 = 157.
What does the binary number system mean?A binary number is a number that is expressed in the binary system or base 2 numeral system, according to digital technology and mathematics. It talks about numerical values.by the two distinct digits 1 (one) and 0 (zero). The base-2 system is the positional notation that uses 2 as the radix.
Define decimal.A number that has been divided into a whole and a fraction is called a decimal. To express the numerical value of entirely and partially entire quantities between integers, decimal numbers are used.
To know more about binary number visit:
https://brainly.com/question/8649831
#SPJ4
i need help asap this is urgent

Answers
Answer:
I
Step-by-step explanation:
I don't know love you tho
Question 85
The distance between the end of the water supply pipe and the sink should be how many times the diameter of the supply pipe?
a. 1-1/2
b. 2 c. 3
d. 4
Answers
The distance between the end of the water supply pipe and the sink should be 2 times the diameter of the supply pipe. Option B is the correct answer.
The distance between the end of the water supply pipe and the sink is determined by plumbing codes and standards to ensure safe and efficient water delivery.
The National Standard Plumbing Code requires a minimum distance of 2 times the diameter of the supply pipe between the end of the pipe and the nearest point of use, such as a faucet or sink.
This helps prevent any potential contamination from the supply pipe and ensures adequate flow and pressure for efficient operation.
Learn more about the diameter at
https://brainly.com/question/16938080
#SPJ4
Marco states that both expressions are equal to x. Do you agree or disagree with Marco? Explain your thinking. 8x-7x -5x+5x

Answers
We diagree with Marco because
\(8x-7x=x\)and
\(-5x+5x=0\)where, in the first expression, we factorized x and got
\(8x-7x=(8-7)x=1x=x\)and in the second one, we got
\((-5+5)x=0\cdot x=0\)In summary, the first expression is equal to x and the second one is equal to zero, so they are different expressions.