Answers
Answer:
(x-2y){5-(x-2y)}
=(x-2y)(5-x+2y)
Related Questions
F(x) = 3* + 10xand g(x) = 5x - 3, find (f - g) (x)
Answers
Answer:
(f-g)(x)= (3x + 10) - (5x -3)
f-g = 3x + 10 - 5x + 3
f-g = -2x + 13
In the equation 2y=18, determine which property of equality will be used to isolate the variable. Choose the best answer. A. Multiplication Property of Equality B, Addition Property of Equality C. Division Property of Equality D. Subtraction Property of Equality
Answers
Answer:
(C) Division Property of Equality
Step-by-step explanation:
Looking at the equation \(2y=18\), we see that if we want to isolate y, we need to divide it by 2 - because we're multiplying it by 2, and 2 times a number divided by 2 is just the number.
The Division Property of Equality states that if we have an equation, \(a=b\), then \(a\div c = b\div c\).
So, that means \(2y\div 2\) will be equal to \(18 \div 2\), which is 9.
Hope this helped!
Answer:
C. Division Property of Equality
Step-by-step explanation:
The equation is
2y = 18
The answer is of the form
y = a number
Now, we have 2y. We want y alone. 2y means 2 multiplied by y. Since we want y alone, to undo the multiplication of 2 and y, we need to use division. We need to divide 2y by 2. Then we also need to divide the right side by 2. Since we are using division, the answer is:
C. Division Property of Equality
plz help and no spam plz. A large university accepts 60% of the students who apply. Of the students the
university accepts, 35% actually enroll. If 10,000 students apply, how many actually enroll?
Answers
Answer:
2100
Step-by-step explanation:
60%=0.6
35%=0.35
0.6*0.35=0.21
0.21*10000=2100
find x=, if x-5=10 HURRY!!!!
Answers
You have a stack of cards. You have 15 yellow, 6 green, 2 red, and 2 orange cards. Once you select one card…. You put the card back in the deck (this makes it an independent event). Answer the following questions:
The probability of selecting 2 yellow cards
Answer:
You select a green card and then a red card
Answer:
You select a green card OR an orange card
Answer:
You don’t select a yellow card
Answer:
You do not select an orange card
Answer:
You select a green card and a red card
Answer;
Answers
Answer:
1. 15/25 or 3/5
2. 2/25
3. 8/25
4. 10/25
5. 23/25
6. 8/25
Step-by-step explanation: sorry if something is wrong i truly am just delete if something is wrong
The formula P=2(l + w)relates the perimeter P, in decimeters, with the length and width of a rectangle.
Solve the formula for w.
1.w=2P-L
2.w=P/2+L
3.w=P/2-L
4.w=2P+L
Answers
Answer:
3. w=P/2-L
Step-by-step explanation:
Given formula:
P=2(l + w)Solving for w:
P=2(l + w)P/2 = 2(l + w)/2P/2 = l + wP/2 - l = l + w - lP/2 - l = ww = P/2 - lCorrect answer choice is:
3. w=P/2-LA company pays sales staff a monthly commission of:4% on the first $15,000 of sales6% on the next $20,000 of sales7.5% on all sales above $35,000What amount would Tony earn if his sales for a month were $41,000?
Answers
Answer
Tony's Commission for the month where his sales was $41,000 is
= $2,250
Explanation
4% on the first $15,000 of sales
6% on the next $20,000 of sales
7.5% on all sales above $35,000
So, if the sales in a month is $41,000, there will be
4% on the first $15,000 of sales
6% on the next $20,000 of sales
7.5% of the remaining $6,000
Tony's Commission = (4% × 15,000) + (6% × 20,000) + (7.5% × 6,000)
= (0.04 × 15,000) + (0.06 × 20,000) + (0.075 × 6,000)
= 600 + 1,200 + 450
= $2,250
Hope this Helps!!!
How
do you solve this for coefficients?
g(x) = { 1₁ -1 - T≤x≤0 осхь п 1 f(x+2TT) = g(x)
Answers
The coefficient for the interval -T ≤ x ≤ 0 in the function g(x) is 1. However, the coefficient for the interval 0 ≤ x ≤ 2π depends on the specific form of the function f(x). Without additional information about f(x), we cannot determine its coefficient for that interval.
To solve for the coefficients in the function g(x), we need to consider the conditions given:
g(x) = { 1, -1, -T ≤ x ≤ 0
{ 1, f(x + 2π) = g(x)
We have two pieces to the function g(x), one for the interval -T ≤ x ≤ 0 and another for the interval 0 ≤ x ≤ 2π.
For the interval -T ≤ x ≤ 0, we are given that g(x) = 1, so the coefficient for this interval is 1.
For the interval 0 ≤ x ≤ 2π, we are given that f(x + 2π) = g(x). This means that the function g(x) is equal to the function f(x) shifted by 2π. Since f(x) is not specified, we cannot determine the coefficient for this interval without additional information about f(x).
The coefficient for the interval -T ≤ x ≤ 0 is 1, but the coefficient for the interval 0 ≤ x ≤ 2π depends on the specific form of the function f(x).
Learn more about coefficients from the given link:
https://brainly.com/question/13431100
#SPJ11
14 less than 8 times a number is 3 more than 4 times the number. What is the number?
Answers
Answer:
x = 17/4
Step-by-step explanation:
Let x = the number
8x-14 = 4x+3
Subtract 4x from each side
8x -14-4x = 4x+3-4x
4x-14 = 3
Add 14 to each side
4x-14+14 = 3+14
4x = 17
Divide by 4
4x/4 = 17/4
x = 17/4
Select the most precise and correct definition of parallel lines from among those that are given.
A.Two distinct lines are parallel if they are in the same plane and never intersect each other.
b.Two distinct lines are parallel if they point in the same direction.
c.Two distinct lines are parallel if one can be rotated to sit on top of the other.
Answers
HELP!! Find the value of… (picture)

Answers
Answer:
C. 94
Step-by-step explanation:
|A| = \(\left[\begin{array}{ccc}a&b&c\\d&e&f\\g&h&i\end{array}\right]\)
det(A) = a * \(\left[\begin{array}{ccc}e&f\\h&i\\\end{array}\right]\) - b * \(\left[\begin{array}{ccc}d&f\\g&i\\\end{array}\right]\) + c * \(\left[\begin{array}{ccc}d&e\\g&h\\\end{array}\right]\)
OR
det(A) = aei - afh - bdi + bfg + cdh - ceg
|A| = \(\left[\begin{array}{ccc}-2&-2&-5\\2&7&-3\\8&9&9\end{array}\right]\)
det(A) = -2 * \(\left[\begin{array}{ccc}7&-3\\9&9\\\end{array}\right]\) - (-2) * \(\left[\begin{array}{ccc}2&-3\\8&9\\\end{array}\right]\) + (-5) * \(\left[\begin{array}{ccc}2&7\\8&9\\\end{array}\right]\)
OR
det(A) = (-2)(7)(9) - (-2)(-3)(9) - (-2)(2)(9) + (-2)(-3)(8) + (-5)(2)(9) - (-5)(7)(8)
det(A) = 94
PLEASE HELP ME I AM GROUNDED AND NEED THIS FINISHED NOWWW
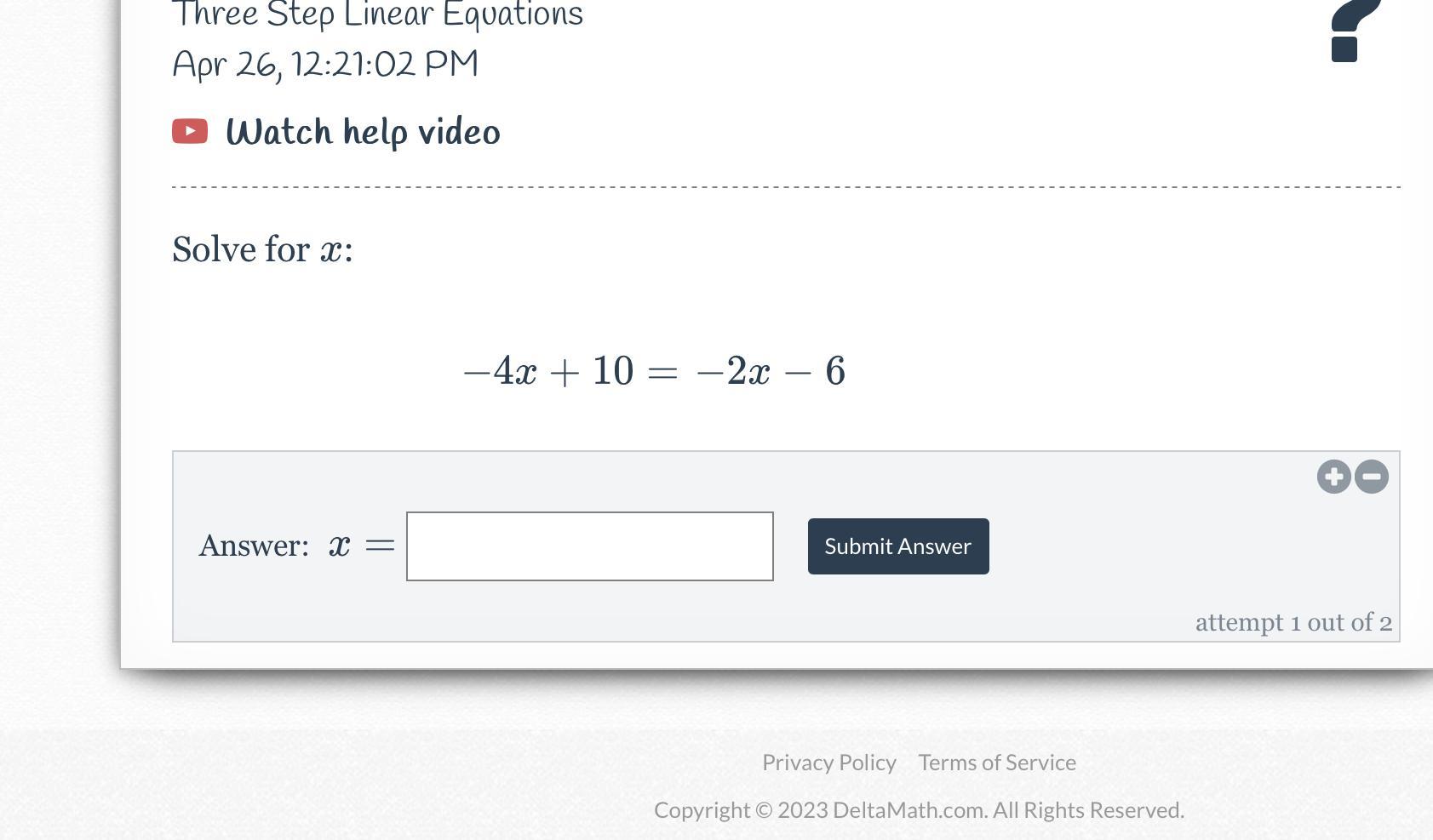
Answers
Answer:
x = 8
Step-by-step explanation:
-4x + 10 = -2x - 6
-4x + 2x = -6 - 10 (Calculate)
-2x = -16 (Divide both sides by -2)
x = 8
Have a nice day! :D
Answer:
x=8
Step-by-step explanation:
-4x+10=-2x-6 original equation
-4x+16=-2x add 6 both sides
16=2x add 4 both sides
x=8 divide 2 both sides
right rectangular prism calc: find w, l=n/a, h=n/a, v=n/a
Answers
The value of width 'w' of rectangular prism with l = n/a, h = n/a, v = n/a is given by, w = a/n.
We know that the volume of rectangular prism with length L and width W and Height H is given by,
V = L*W*H
Given that the Height of the rectangular prism, h = n/a
Length of the rectangular prism, l = n/a
Volume of the rectangular prism, v = n/a
let the width of the rectangular prism be 'w'.
So from the volume formula we get,
v = lwh
n/a = (n/a)*w*(n/a)
n/a = (n/a)²*w
w = (n/a)/(n/a)² = (n/a)*(a/n)² = a/n
Hence the value of w is a/n.
To know more about rectangular prism here
https://brainly.com/question/30095130
#SPJ4
Jahier's Bikes rents bikes for $20.00 plus $5.00 per hour. Daniel paid $35.00 to rent a bike. For how
many hours did she rent the bike?
Answers
Answer: 3 Hours
Step-by-step explanation:
Daniel first paid for her bike, which was 20 dollars. Then she used it for 3 hours, because 20 dollars+5 dollars+5 dollars+5 dollars equals 35 dollars.
Hope this helps!
82.1 in expanded form
Answers
Answer:
82.1 = 80 + 2 + 0.1
Step-by-step explanation:
Step-by-step explanation:
8 tens 2 ones and 1 tenth
Exercise 1. Two servers (S. and Ss) with exponential service time and same service rate are busy completing service of two jobs at time t = 0. The server that completes service first is referred to as the winning server (Sw), the other is referred to as the losing server (St). Jobs must complete their service before departing from the queue. A) Compute the probability of S to be the winning server, i.e., P(S = S1) = P(S = S2). Compute the probability of S, to be the winning server, i.e., P(S = Sx) = P(S = Si) (pt. 10). B) Compute the expected departure time of the winning server, defined as ty > 0 [pt. 10). C) Compute the expected departure time of the losing server, defined as t > t pt. 10).
Answers
A) Here, we have two servers: Server 1 (S1) and Server 2 (S2). And we need to compute the probability of S to be the winning server, i.e., P(S = S1) = P(S = S2).Since we have two servers with the same service rate, the jobs have equal chances of being assigned to either server.
Therefore, P(S = S1) = P(S = S2)
= 1/2.
(Both servers have equal probabilities of winning).
B) Expected departure time of the winning server, defined as ty > 0. It is also called the mean service time (MST) or the expected value of the service time. The expected value of an exponential distribution is equal to the reciprocal of the service rate. Thus, if the service rate of both servers is μ, then the expected departure time of the winning server will be 1/μ.
C) Expected departure time of the losing server, defined as t > t0. Since the two jobs can't leave until their services are complete, the service time of the winning server will be the total time taken by both jobs. Thus, the expected departure time of the losing server can be calculated by taking the expected departure time of the winning server (which is 1/μ) and subtracting the mean service time (MST) of a single job, which is 1/2μ. Therefore, the expected departure time of the losing server will be 1/μ - 1/2μ = 1/2μ.
To know more about probability visit:
https://brainly.com/question/31828911
#SPJ11
A
Find the slope.
y = 4x - 3
Remember: y = mx + b
m = −3
B
m = 4
C
m = 3
Answers
Answer:
4
Step-by-step explanation:
ik how to find slope and yeah
Point I is on line segment \overline{HJ}
HJ
. Given HI=2x,HI=2x, HJ=4x,HJ=4x, and IJ=4x-10,IJ=4x−10, determine the numerical length of \overline{IJ}.
IJ
.
Answers
Answer:
IJ = 20
Step-by-step explanation:
Point I is on the lines segment HJ. So,
HJ = HI + IJ
Putting all the given values, we get :
4x=2x+4x-10
Taking like terms together, we get :
-2x=-10
x=5
Put the value of x in IJ = 4x-10
IJ = 4(5)-10
IJ = 20
Hence, the value of IJ is 20.
Answer:
10
Step-by-step explanation:
The fruit fly decided to go on a all-vegetable diet. As a result, she now only weighs 90% of what she used to. If the fruit fly currently weighs 0.018 grams, how many grams did she weigh before she went on the diet?
Answers
9514 1404 393
Answer:
0.02 g
Step-by-step explanation:
Let w represent the original weight. The problem statement tells us ...
0.90w = 0.018 g
Dividing by the coefficient of w, we find ...
w = 0.02 g
She weighed 0.02 grams before the diet.
observe that the column is the sum of the and columns. find a nontrivial solution of without performing row operations
Answers
To find a nontrivial solution of a system of equations without performing row operations is to recognize that the column on the left side is the sum of the and columns.
To find a nontrivial solution of a system of equations, we can observe the relationship between the columns in the augmented matrix representing the system. If the column on the left side is the sum of the and columns, then there exists a nontrivial solution. Let's consider a system of equations with variables x, y, and z. The augmented matrix representing the system can be written as [A|B], where A represents the coefficients of the variables and B represents the constant terms.
If we notice that the column on the left side is the sum of the and columns, i.e., the sum of the first and second columns equals the third column, then we can conclude that the system of equations has a nontrivial solution. This means that there are infinitely many solutions to the system, rather than a unique solution. By recognizing this relationship, we can determine that the system is dependent, and we can find a nontrivial solution by setting one of the variables as a free variable and expressing the other variables in terms of it. This allows us to generate a solution set that satisfies the system of equations without performing row operations.
Learn more about coefficients here: brainly.com/question/13431100
#SPJ11
3. Find the general solution y(x of the following second order linear ODEs: ay+2y-8y=0 by"+2y+y=0 cy+2y+10y=0 (dy"+25y'=0 ey"+25y=0
Answers
(a) The general solution for the ODE ay + 2y - 8y = 0 is\(y(x) = C_{1} e^{4x/a} + C_{2}e^{-2x/a}\)
(b) The general solution for the ODE y" + 2y + y = 0 is \(y(x) = (C_{1} + C_{2} x)e^{-x}\)
(c) The general solution for the ODE cy + 2y + 10y = 0 is\(y(x) = C_{1}e^{-3x/cos(\sqrt{39x} /c)} + C_{2}e^{3x/cos(\sqrt{39x}/c)}\)
(d) The general solution for the ODE dy" + 25y' = 0 is\(y(x) = C_1+ C_{2}e^{-25x/d}\)
(e) The general solution for the ODE ey" + 25y = 0 is \(y(x) = C_1sin(5\sqrt{e})x + C_2cos(5\sqrt{e})x\)
To find the general solution of a second-order linear ODE, we need to solve the characteristic equation and use the roots to construct the general solution.
(a) For the ODE ay + 2y - 8y = 0, the characteristic equation is \(ar^2 + 2r - 8 = 0\). Solving this quadratic equation, we find the roots r₁ = 2/a and r₂ = -4/a. The general solution is \(y(x) = C_{1} e^{4x/a} + C_{2}e^{-2x/a}\), where C₁ and C₂ are arbitrary constants.
(b) For the ODE y" + 2y + y = 0, the characteristic equation is r^2 + 2r + 1 = 0. The roots are r₁ = r₂ = -1. The general solution is \(y(x) = (C_{1} + C_{2} x)e^{-x}\) , where C₁ and C₂ are arbitrary constants.
(c) For the ODE cy + 2y + 10y = 0, the characteristic equation is cr^2 + 2r + 10 = 0. Solving this quadratic equation, we find the roots r₁ = (-1 + √39i)/c and r₂ = (-1 - √39i)/c. The general solution is y(x) = \(y(x) = C_{1}e^{-3x/cos(\sqrt{39x} /c)} + C_{2}e^{3x/cos(\sqrt{39x}/c)}\), where C₁ and C₂ are arbitrary constants.
(d) For the ODE dy" + 25y' = 0, we can rewrite it as r^2 + 25r = 0. The roots are r₁ = 0 and r₂ = -25/d. The general solution is\(y(x) = C_1+ C_{2}e^{-25x/d}\), where C₁ and C₂ are arbitrary constants.
(e) For the ODE ey" + 25y = 0, the characteristic equation is er^2 + 25 = 0. Solving this quadratic equation, we find the roots r₁ = 5i√e and r₂ = -5i√e. The general solution is y(x) = C₁
Learn more about arbitrary constants here:
https://brainly.com/question/17225511
#SPJ11
I need help with number 8

Answers
Answer:
Ten thousands place, and its value is fifty thousand (50,000).
Step-by-step explanation:
3,152,308 in written form is:
"Three million, one hundred and fifty two thousand, three hundred and eight."
3,152,308
The underlined number is in the ten thousands place, and its value is 50,000. In the attached image, I've plugged in your given numbers into the place value chart to show the place value of each digit.

18 vocabulary words learned in 2 hours; 27 vocabulary words learned in 3 hours
Answers
Answer: They are equivalent
Step-by-step explanation:
Here is the complete question:
18 vocabulary words learbed in 2 hours 27 vocabulary words learned in 3 hours Are they equivalent? Why
From the question, we are informed that 18 vocabulary words are learned in 2 hours. The number of words learned per hour will be:
= 18/2
= 9 words per hour.
27 vocabulary words learned in 3 hours. The number of words learned per hour will be:
= 27/3
= 9 words per hour.
Since they both have identical number of words learned per hour, it means that they're equivalent.
Find the volume of a right rectangular prism if the dimensions of the base are 6.8 centimeters by 12.5 centimeters and the height is 13 centimeters.
A.
B.
C.
D.
Answers
Answer:
6.8 x 12.5 x 13 = 1105
Step-by-step explanation:
Answer:
the answer is 1105
Step-by-step explanation:
Brian buys a computer for £1600. It depreciates at a rate of 4% per year. How much will it be worth in 3 years? Give your answer to the nearest penny where appropriate.
Answers
Answer:
amount = £1415.57
Step-by-step explanation:
given data
computer buy = £1600
depreciates rate = 4% per year
time = 3 year
solution
we get here amount that is express here as
amount = P × \((1-r)^{t}\) ...................................1
here r is rate and t is time put here value
amount will be
amount = 1600 × \((1-0.04)^{3}\)
solve it we get
amount = £1415.57
What is the equation of this graphed line?
y=2x+2
y=-2x-2
y=6x+6
y=-6x-6

Answers
By calculating the given equations with points none of the equation represents equation of this graphed line.
What is the equation of the line?
The equation of a straight line is y=mx+c y = m x + c m is the gradient and c is the height at which the line crosses the y -axis, also known as the y-intercept.
We have given the,
y = 2x+2
y=-2x-2
y=6x+6
y=-6x-6
And, the graph.
From the line in the graph we can take the cordinate points which represents the equation of line.
point (1, 2)
By putting this point we can decide the given equation of line:
y=2(1)+2 = 4
y=-2(1)-2 = 0
y=6(1)+6 =12
y=-6(1) - 6 =-12
Hence, by calculating the given equations with points none of the equation represents equation of this graphed line.
To learn more about the equation of the line visit,
https://brainly.com/question/2787019
#SPJ1
What is the formula to find centre?
Answers
The general equation to find centre of a circle is ( x - h )^2 + ( y - k )^2 = r^2, where ( h, k ) is the center and r is the radius.
Centre of the CircleThe location of a moving point on a plane such that its separation from a stationary point on the plane remains constant or fixed is described as a circle. The centre of the circle is the name given to the fixed point
A circle's centre and radius make up its 2D shape. Knowing the circle's centre and radius allows us to draw any circle. The number of radii that a circle can have is unlimited. The intersection of all the radii constitutes the centre of a circle. It is also known as the centre of the circle's diameter.
Center of Circle FormulaThe general equation of a circle is another name for the centre of circle formula. If the radius is r, the center's coordinates are (h,k), and any point on the circle is (x,y), the centre of the circle formula is as follows:
(x - h)2 + (y - k)2 = r2
2 = r2
Also known as center of the circle equation. we'll use this formula to determine a circle's equation or centre.
To know more about Centre of the Circle, Refer to :
https://brainly.com/question/17104055
#SPJ4
Please help me!
If f(x) = 3x^2 -3 then what is f(4)?
Show work
Answers
Answer:
f(4)= 45
Step-by-step explanation:
f(x)=3x^2-3. (1)
f(4)=?
so we have to put x=4 in equation (1)
we get
f(4)=3[(4)^2]-3
f(4) =3(16)-3
f(4)= 48-3
f(4)= 45 Answer...
two angles that add up to 90 degrees are called ________ angles.
Answers
Answer: Right/complementary angles
Step-by-step explanation:
Two angles that add up to 90 degrees are called complementary angles.
Complementary angles are a pair of angles that, when added together, equal a right angle, which measures 90 degrees.
In other words, the sum of the measures of complementary angles is always 90 degrees.
Complementary angles often arise in geometry and trigonometry, and understanding their properties is important when working with angles and solving problems involving right triangles.
To learn more on Angles click:
https://brainly.com/question/28451077
#SPJ6
Evaluate. (3/5)3 enter your answer by filling in the boxes.
Answers
the final answer is 27/125.
To evaluate \((3/5)^3\), we simply need to multiply (3/5) by itself three times:
\((3/5)^3 = (3/5) * (3/5) * (3/5)\)
To simplify, we can first multiply the numerators together and the denominators together:
\((3/5)^3 = 27/125\)
To know more about multiply refer here
https://brainly.com/question/10883691#
#SPJ11
