Answers
Answer:
The first and third options are correct so choose both
Step-by-step explanation:
Related Questions
When the input is - 5 the Output is
Answers
Step-by-step explanation:
the output is 5 .........
4+3x<22 find the solution for the inequlity
Answers
Answer:x<6
Step-by-step explanation:
Step-by-step explanation:
4+3x<22
3x<22-4
3x<18
3x÷3<18÷3
x<6
Which of these statements is a valid perfect square trinomial?
Statement 1: x2 + 4x + 4 = (x + 2)2
Statement 2: a2 + 2ab + ab = (a + b)2
a
Statement 1 and statement 2 are valid.
b
Statement 1 and statement 2 are invalid.
c
Only statement 1 is valid.
d
Only statement 2 is valid.
Answers
Answer:
C
Step-by-step explanation:
consider the right side
(x + 2)²
= (x + 2)(x + 2) ← expand using FOIL
= x² + 2x + 2x + 4 ← collect like terms
= x² + 4x + 4 = left side
then x² + 4x + 4 is a valid perfect square binomial
x² + 4x + 4 = (x + 2)²
then
x² + 4x + 4
Statement 2
consider the right side
(a + b)²
= (a + b)(a + b) ← expand using FOIL
= a² + ab + ab + b² ≠ left side
then
a² + 2ab + ab is not a valid perfect square binomial
solve by factoring x^2+5-14=0
Answers
Answer:
Factored -> (x+7)(x-2)
Step-by-step explanation:
If you are solving for x then its x= -7, x=2
Check that the equation below is not exact but becomes exact when multiplied by the integrating factor. x^2y^3 + x(1+y 2)y' = 0
Integrating factor: µ(x,y)=1/(xy 3).
Solve the differential equation.
You can define the solution curve implicitly by a function in the form
F(x,y) = G(x) + H(y) = KF(x,y) = ?
Answers
The given equation is x2y3 + x(1+y 2)y' = 0. This is not an exact equation, as the derivative of F(x,y) is not equal to zero. To make this equation exact, we need to multiply it by an integrating factor.
The integrating factor (I.F.) of a differential equation is a function of the independent variable, which can be used to transform an equation into an exact equation. An exact equation is one in which the derivative of F(x,y) is equal to zero. To find the integrating factor, we need to multiply the given equation by I.F. and set the derivative of F(x,y) to zero.
To find the I.F., we need to divide both sides of the equation by x(1+y2):
x2y3 + x(1+y 2)y' / x(1+y2) = 0 / x(1+y2)
Rearranging this equation, we get:
y' + (x2y3 / x(1+y2)) = 0
Now, we can integrate both sides of the equation,
∫y' + (x2y3 / x(1+y2)) dx = 0
This gives us an expression for the I.F.,
I.F. = exp(∫(x2y3 / x(1+y2))dx)
Now, to make the equation exact, we need to multiply the given equation with the integrating factor. This gives us: I.F. x x2y3 + I.F. x x(1+y2)y' = 0
Simplifying this equation, we get:
F(x,y) = G(x) + H(y) = K
Where K is a constant. Thus, we have now converted the given equation into an exact equation.
Know more about exact equation here:
https://brainly.com/question/11640443
#SPJ11
Căn 84 bình phương trừ 37 bình phương chia 47
Answers
Answer:
\(\sqrt{84^{2} } -37^{2} /47\)=257 9/47
Step-by-step explanation:
Matthew bought a scale model toy version of the
space ship in his favorite science fiction television
show. If the tov is constructed with the scale
1 inch
= 12 feet and the toy is 1 foot 4 Inches
long, how long is the ship in the television show?
A. 192 feet
B.168 feet
C.60 feet
D. 49 feet
Answers
Answer: A. 192 feet
Step-by-step explanation:
1 foot 4 inches=
1 foot+4 inches=
12 inches+4 inches=
16 inches
Hence,
16*12=
16*(10+2)=
(16)(10)+(16)(2)=
160+32
192 feet
Which shows the factored form of x2 – 12x – 45? (x 3)(x – 15) (x – 3)(x – 15) (x 3)(x 15) (x – 3)(x 15).
Answers
The factors of the quadratic equation are (x+3) and (x-15).
Given to us\(x^2-12x-45\\\)To findThe factors of \(x^2-12x-45\\\).
Solution\(x^2-12x-45\\\)
As we can see in the quadratic equation the value of ac is -45x². therefore, we need to divide -12x into two parts such that their sum must give -12x and their product gives us -45x².
therefore, dividing -12x into -15x and 3x,
\(x^2-15x+3x-45\\\)
Taking common out,
\(x^2-15x+3x-45\\x(x-15)+3(x-15)\\(x+3)(x-15)\)
Hence, the factors of the quadratic equation are (x+3) and (x-15).
Learn more about quadratic equations:
https://brainly.com/question/2263981
Answer:
(x + 3)(x – 15)
Step-by-step explanation:
Red Valley Cabs charges $4.50 to initiate a cab ride, plus an additional $3.20 per mile driven. Write an equation showing how the cost of a cab ride, y, depends on the number of miles driven, x.
Answers
Answer: y=$4.50+$3.20x
Step-by-step explanation: You already have to pay $4.50 plus $3.20 every mile driven (x) equals your final cost (y)
What would be the value of the sum of squares due to regression (SSR) if the total sum of squares (SST) is 25.32 and the sum of squares due to error (SSE) is 6.89
Answers
The value of the sum of squares due to regression is 18.43.
Given the total sum of squares (SST) is 25.32 and the sum of squares due to error (SSE) is 6.89.
A common measure used in regression analysis is the sum of squares total (SST). The square of each difference is added to the sum of squares to obtain the squared disparities between individual data points and their mean. The variance is calculated using the sum of squares, which is also used to assess how well a regression curve fits the data.
We will use the total sum of squares (SST) is given as the summation of the sum of squares due to regression (SSR) and sum of squares error (SSE);
SST = SSR + SSE
Here, SST=25.32 and SSE=6.89
Substitute the values in the formula, we get
25.32=SSR+6.89
Now, we will subtract 6.89 from both sides, we get
25.32-6.89=SSR+6.89-6.89
18.43=SSR
Hence, the value of the sum of squares due to regression (SSR) when the total sum of squares (SST) is 25.32 and the sum of squares due to error (SSE) is 6.89 is 18.43.
Learn more about regression from here brainly.com/question/23438254
#SPJ4
Question 4(Multiple Choice Worth 6 points)
(03.02 MC)
How does the graph of g(x) = (x + 2)3 - 6 compare to the parent function of f(x) = x³?
Og(x) is shifted 2 units to the right and 6 units down.
Og(x) is shifted 6 units to the right and 2 units up.
g(x) is shifted 2 units to the left and 6 units down.
g(x) is shifted 6 units to the left and 2 units down.
Answers
The relationship of the graph g(x) = (x − 2)³ + 6 compare to the parent function of f(x) = x³ is that g(x) is shifted 2 units to the right and 6 units up.
What is translation of coordinates?Translations are a transformation technique that changes the position of an object from one point on the plane to another.
Given is a function, g(x) = (x − 2)³ + 6, we need to compare the graph of g(x) = (x + 2)³ - 6 to the parent function of f(x) = x³
The function compared to f(x) = x³, shows a translation of f(x) by 2 unit to the right along the horizontal and vertical translation of the function 6 units up
Hence, the relationship of the graph g(x) = (x − 2)³ + 6 compare to the parent function of f(x) = x³ is that g(x) is shifted 2 units to the right and 6 units up.
Learn more on translation here:
brainly.com/question/12861087
#SPJ1
Is W a subspace of the vector space? If not, state why. (Select all that apply.)
W is the set of all vectors in
R2
whose second component is the cube of the first.
W is a subspace of R2.W is not a subspace of R2 because it is not closed under addition.W is not a subspace of R2 because it is not closed under scalar multiplication.
Answers
We have found a vector in W and a scalar outside of W such that their product is not in W, which means that W is not closed under scalar multiplication. Hence, W cannot be a subspace of R2.
To show that a subset is a subspace of a vector space, we need to verify three conditions:
Closure under addition: For any vectors u and v in the subset, their sum u + v is also in the subset.
Closure under scalar multiplication: For any vector u in the subset and any scalar c, the product cu is also in the subset.
Contains the zero vector: The subset contains the zero vector.
In this case, W is defined as the set of all vectors in R2 whose second component is the cube of the first. We can write this as:
W = {(x, y) ∈ R2 | y = x^3}
Now, to show that W is not closed under scalar multiplication, we need to find a vector in W and a scalar outside of W such that their product is not in W.
Let's consider the vector (1, 1), which belongs to W since 1 = 1^3. If we multiply this vector by the scalar 2, we get:
(2, 2) = 2(1, 1)
However, (2, 2) does not belong to W because 2 is not equal to 1^3. Therefore, we have found a vector in W and a scalar outside of W such that their product is not in W, which means that W is not closed under scalar multiplication. Hence, W cannot be a subspace of R2.
Learn more about vector from
https://brainly.com/question/15519257
#SPJ11
HELP!!!!
(2^8 ⋅ 5^−5 ⋅ 19^0)^−2 ⋅ (5^-2 / 2^3)^4 ⋅ 2^28
Write in the simplified form, and show steps, please!!!!
Answers
Answer:
(2^8 ⋅ 5^−5 ⋅ 19^0)^−2 ⋅ (5^-2/2^3)^4 ⋅ 2^28
= (2^-16 ⋅ 5^10 ⋅ 1)⋅(5^-8 / 2^12) ⋅ 2^28
= 2^(-16-12+28) ⋅ 5^(10-8)
= 2^0 ⋅ 5^2
= 1 ⋅ 25
= 25
Step-by-step explanation:
M
135°
Angle JKL is a straight angle.
The measure of angle JKM is 135º.
The measure of angle MKL is xº.
What is the value of x

Answers
Answer: 45
Step-by-step explanation:
Hello there!
So this problem looks a lot more complex than it really is. First, we need to know that a straight line is 180 degrees. Since both of these angles must add up to 180, we just subtract 135 from 180. We then get 45.
Hope this helped! :D
Sorry if any information was incorrect or if i didn’t understand what you were asking. Just comment and I will reply back ready to help!
Which expression is equivalent to StartRoot 120 x EndRoot?
Answers
The answer choice which represents an expression which is equivalent to √120x as given in the task is; 2√30x.
Which expression is equivalent to the expression; √120x as given in the task content?It follows from simplification that the expression given in the task content can be rewritten as follows;
√120x
= √4 × √30x
= 2√30x.
Read more on equivalent expressions;
https://brainly.com/question/14507158
#SPJ1
how many 35 cent donuts can u buy with $1.00
Answers
Help me please I need this for my test tomorrow pleaseeeee!!!!!!

Answers
Answer:
st
Just substitute in the given values
Step-by-step explanation:
Hey, there!!
You can solve it simply,
1.
Given,
x= 1 , y=2 , z= 3
To solve:
\( = \frac{1}{ {x}^{2} } - \frac{x}{y + z} \)
\( = \frac{1}{ {1}^{2} } - \frac{1}{2 + 3} \)
\( = 1 - \frac{1}{5} \)
Taking LCM,
\( = \frac{5 - 1}{5} \)
Therefore, the final answer is 4/5.
2.
Given,
x= 1 , y= -2, z= -1.
To solve:
\( = \frac{1}{ {x}^{2} } - \frac{x}{y + z} \)
\( = \frac{1}{ {1}^{2} } - \frac{1}{ - 2 - 1} \)
Taking LCM,
\( = \frac{ - 3 - 1}{ - 3} \)
\( = \frac{ - 4}{ - 3} \)
Cancelling the minus sign,
\( \frac{4}{ 3} \)
is the final answer.
3.
Given,
x= -3, y= -1, z= 4
To solve:
\( = \frac{1}{ {x}^{2} } - \frac{x}{y + z} \)
\( = \frac{1}{ {( - 3)}^{2} } - \frac{ - 3}{ - 1 + 4} \)
\( = \frac{1}{9} - ( - 1)\)
\( = \frac{1 + 9}{9} \)
Therefore, the answer is 10/9.
4.
Given,
x= 1/2, y=1, z=1.
To solve:
\( = \frac{1}{ {x}^{2} } - \frac{x}{y + z} \)
\( = \frac{1}{ ( { \frac{1}{2}) }^{2} } - \frac{ \frac{1}{2} }{1 + 1} \)
Simplifying them ,
\(1 \times \frac{4}{1} - \frac{1}{2} \times 2\)
\( = 4 - 1\)
Therefore, the answer is 3.
Hope it helps...
what inferences about the relation between income and type of oven usage in population may be drawn from the data above?
Answers
No inferences can be made without performing a hypothesis test
A hypothesis test is a statistical test used to determine whether a specific hypothesis about a population parameter is supported by the data. In this test, a null hypothesis (H0) is stated, which is usually the assumption that the population parameter is equal to a specific value or falls within a certain range. An alternative hypothesis (Ha) is also stated, which is usually the opposite of the null hypothesis.
The next step is to collect data and use statistical techniques to calculate a test statistic, which measures how far the sample data deviates from the null hypothesis. The test statistic is compared to a critical value in a probability distribution, such as a t-distribution or z-distribution, which is determined based on the level of significance (alpha) and the degrees of freedom
Learn more about hypothesis test
https://brainly.com/question/30588452
#SPJ4
Full Question: what inferences about the relation between income and type of oven usage in population may be drawn from the data above?
Table attached

PLEASE HELP I WILL GIVE OUT BRAINLIST ONLY FOR THE RIGHT ANSWERS
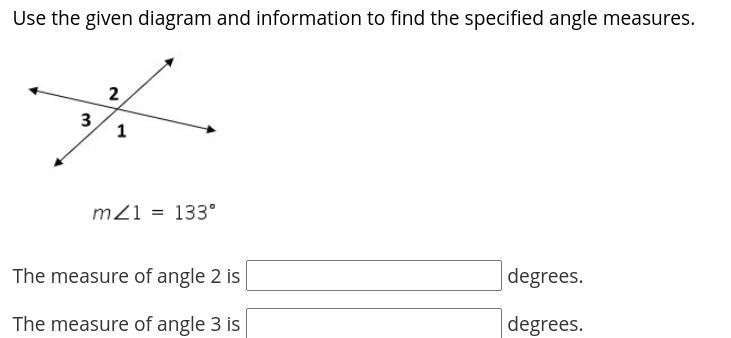
Answers
The measure of angle 2 and angle3 are 133 ad 47 degrees respectively
Vertical angles and angle on a straight lineFrom the given diagram, the measure of angle 1 and 2 are congruent. (vertical angles)
Given he following parameters
m<1 = 133 degrees
Since m<1 = m<2, hence m<2 = 133 degrees
Also;
m<2 + m<3 = 180
133 + m<3 = 180
m<3 = 180 - 133
m<3 = 47 degrees
Hence the measure of angle 2 and angle3 are 133 ad 47 degrees respectively
Learn more on vertical angles here: https://brainly.com/question/11553785
#SPJ1
solve the inequality -24< x - 3 - 8x
Answers
Answer:
x = 3
Step-by-step explanation:
-24 < x - 3 - 8x
-24 < -7x - 3
-24 + 3 = -21
-7x - 3 + 3 = -7x
-21 = -7x
-21 / -7 = 3
-7x / -7 = x
3 = x
What is 2^100 and how do you know?
Answers
Answer:
1.2676506 E30
Step-by-step explanation:
Calculator :)
Also E means to move the decimal places 30 times to the right
compute the odds in favor of obtaining a number divisible by 3 or 4 in a single roll of a die.
Answers
The odds in favor of obtaining a number divisible by 3 or 4 in a single roll of a die are 7:5 or 7/5.
The probability of obtaining a number divisible by 3 or 4 in a single roll of a die can be found by adding the probabilities of rolling 3, 4, 6, 8, 9, or 12, which are the numbers divisible by 3 or 4.
There are six equally likely outcomes when rolling a die, so the probability of obtaining a number divisible by 3 or 4 is:
P(divisible by 3 or 4) = P(3) + P(4) + P(6) + P(8) + P(9) + P(12)
P(divisible by 3 or 4) = 2/6 + 1/6 + 1/6 + 1/6 + 1/6 + 1/6
P(divisible by 3 or 4) = 7/12
The odds in favor of an event is the ratio of the probability of the event occurring to the probability of the event not occurring. Therefore, the odds in favor of obtaining a number divisible by 3 or 4 in a single roll of a die are:
Odds in favor = P(divisible by 3 or 4) / P(not divisible by 3 or 4)
Odds in favor = P(divisible by 3 or 4) / (1 - P(divisible by 3 or 4))
Odds in favor = 7/5
Know more about probability here;
https://brainly.com/question/30034780
#SPJ11
Choose the graph below that correctly represents the equation 3x + 9y = −18. (5 points)
the line through the points negative 6 comma 0 and 0 comma 2
the line through the points negative 6 comma 0 and 0 comma negative 2
the line through the points negative 2 comma 0 and 0 comma 6
the line through the points negative 2 comma 0 and 0 comma negative 6
Answers
Answer:
the line through the points negative 6 comma 0 and 0 comma negative 2
Step-by-step explanation:
Rewrite the equation to something you are familiar with such as the slope intercept form; y = mx + b. This means you need to solve for y.
3x + 9y = -18 --- divide everything by 3.
x + 3y = -6
3y = -6 - x
y = -2 - 1/3x
your slope is -1/3 and your y intercept is - 2.
remember, y-intercept is when x = 0. therefore, when x = 0, y = -2.
write as an ordered pair (0, -2). the only option that has (0, -2) is the second one.
Therefore, the line through the points negative 6 comma 0 and 0 comma negative 2.
A parking lot space is in shape of a rectangle. If the space has a length of 23 feet and a width of 12 feet, what is the area of the parking space?A.128ft2B.266ft2C.276ft2D.138ft2
Answers
area = length multiplied by width
Find A(x) the function representing the area of an equilateral triangle with sides of length six times the original length.
A(6x) = ?

Answers
AThe area of an equilateral triangle is given by the formula:
A = (sqrt(3)/4) * s^2
where A is the area and s is the length of one side.
If the original length of the side is x, then the new length is 6x. Therefore, we can write:
A(6x) = (sqrt(3)/4) * (6x)^2
Simplifying:
A(6x) = (sqrt(3)/4) * 36 * x^2
A(6x) = 9 * sqrt(3) * x^2
Therefore, the function representing the area of an equilateral triangle with sides of length six times the original length is:
A(6x) = 9 * sqrt(3) * x^2:
Bryan has $15 to spend on taxi fare. The ride costs $5 plus $2.50 per mile. How many mile can Bryan go without spending all $15
Answers
Answer:
3 miles.
Step-by-step explanation:
He has to pay 5 just to ride, and 2.50 per mile.
2.50(x)+5=15
2.50(4)+5=15
10+5=15
So he can riide 3 miles without spending all his money.
A kitchen supply outfit makes spoons and forks for sale. It costs the store $2 to buy the supplies needed to make a fork and $1 for the supplies to make a spoon. The store sells the forks for $4 and the spoons for $5. Last month they spent $39 on supplies and sold all the forks and spoons they made using those supplies for $93. How many forks and spoons did they make?
Answers
Given :
A kitchen supply outfit makes spoons and forks for sale. It costs the store $2 to buy the supplies needed to make a fork and $1 for the supplies to make a spoon. The store sells the forks for $4 and the spoons for $5.
Last month they spent $39 on supplies and sold all the forks and spoons they made using those supplies for $93.
To Find :
How many forks and spoons did the company make.
Solution :
Let, number of forks and spoons the company made are f and s.
For buying from the kitchen the equation is :
2f + s = 39 ....1)
For selling the equation will be :
4f + 5s = 93 ....2)
Multiplying equation 1) by 5 and subtracting equation 2) from it we get :
(10f + 5s) - ( 4f + 5s) = 195 - 93
6f = 102
f = 17 and s = 5
Therefore, the company make 17 forks and 5 spoons.
Which expression is equivalent to sine startfraction 7 pi over 6 endfraction?
Answers
The equivalent expression to the given one is -sin(pi/6).
Which expression is equivalent to the given one?The given expression is:
sin(7pi/6)
Where the argument is in radians.
Remember that:
sin(a + b) = sin(a)*cos(b) + sin(b)*cos(a)
Now we can rewrite:
sin(7pi/6) = sin(pi + pi/6)
Then we can use the above relation to rewrite:
sin(pi + pi/6) = sin(pi)*cos( pi/6) + sin(pi/6)*cos(pi)
And we know that:
sin(pi) = 0
cos(pi) = -1
Then we can write:
sin(7pi/6) = - sin(pi/6)
If you want to learn more about the sine function:
https://brainly.com/question/9565966
#SPJ4
what is the volume of a cylinder, in cubic m, with a height of 18m and a base diameter of 12m? round to the nearest tenths place.
Answers
The volume of the cylinder with a height of 18m and a base diameter of 12m is approximately 1940.4 cubic meters, rounded to the nearest tenths place. It is important to remember to use the correct formula and units when calculating the volume of a cylinder.
The volume of a cylinder can be calculated using the formula V=πr²h, where r is the radius of the base and h is the height of the cylinder.
The diameter of the base is given as 12m, which means the radius would be half of that, or 6m. Substituting these values in the formula, we get V=π(6)²(18), which simplifies to V=1940.4 cubic meters.
To find the volume of a cylinder, we need to know its height and the diameter of its base. In this case, the height is given as 18m and the base diameter as 12m.
We can calculate the radius of the base by dividing the diameter by 2, which gives us 6m.
Using the formula V=πr²h, we can substitute these values to get the volume of the cylinder. After simplification, we get a volume of 1940.4 cubic meters, rounded to the nearest tenths place. Therefore, the volume of the cylinder with a height of 18m and a base diameter of 12m is approximately 1940.4 cubic meters.
The volume of a cylinder can be calculated using the formula V=πr²h, where r is the radius of the base and h is the height of the cylinder. In this case, the volume of the cylinder with a height of 18m and a base diameter of 12m is approximately 1940.4 cubic meters, rounded to the nearest tenths place. It is important to remember to use the correct formula and units when calculating the volume of a cylinder.
To know more about volume visit:
brainly.com/question/28058531
#SPJ11
Find the equation for the graph in the interval -1 < x≤ 3 as displayed in the graph.

Answers
The equation for the graph in the interval is y = 3/2x - 1/2
Finding the equation for the graph in the intervalFrom the question, we have the following parameters that can be used in our computation:
The graph
Where, we have
(-1, -2) and (3, 4)
The equation of the line is calculated as
y = mx + c
Where
c = y when x = 0
Using the points, we have
-m + c = -2
3m + c = 4
Subtract the equations
-4m = -6
So, we have
m = 3/2
This means that
y = 3/2x +c
Next, we have
3/2 * 3 + c = 4
This gives
c = -1/2
Hence, the equation of the line is y = 3/2x - 1/2
Read more about linear function at
brainly.com/question/15602982
#SPJ1
