Please help me the picture is above. I’ll mark as brainliest please answer this.

Answers
Answer:
last option: 9200 + 80c ≤ 25,000
Step-by-step explanation:
the options showing ≥ 25,000 cannot be solutions because the weight must be less than 25,000
if there already is 9200 then that would be added to 80c and, when solved, would tell you how many 80kg containers can be loaded
80c ≤ 25,000 - 9,200
80c ≤ 15,800
c ≤ 15,800 ÷ 80
c ≤ 197.5
Related Questions
A square has n units for each side. Write 3 expression to represent the perimeter
of the square
Answers
Given a square with each side measuring n units, we need to write three expressions to represent the perimeter of the square. The perimeter of a square is the sum of the lengths of all its sides.
To calculate the perimeter of a square, we add up the lengths of all four sides. Since each side of the square measures n units, we can write the expressions for the perimeter as follows:
Perimeter = n + n + n + n
This expression represents the direct sum of all four sides of the square, where each side is represented by n units.
Perimeter = 4n
This expression simplifies the sum of all four sides into a single term, 4n, by multiplying the length of one side, n, by the number of sides in a square, which is 4.
Perimeter = 2(n + n)
This expression demonstrates an alternative way to calculate the perimeter by adding the lengths of two adjacent sides and then multiplying the sum by 2. In this case, we have (n + n) for the lengths of two adjacent sides and then multiply the sum by 2.
All three expressions accurately represent the perimeter of a square with each side measuring n units. The second expression, 4n, is the most simplified form of the perimeter expression, while the other two expressions provide alternative ways to understand the concept of the perimeter by breaking it down into component parts.
Learn more about perimeter here:- brainly.com/question/7486523
#SPJ11
What is the value of the expression below when x=8 and y=3? 8x-9y
Answers
Answer:
8(8)-9(3)
64-27= 37
Step-by-step explanation:
8(8)-9(3)
64-27=27
In a flower display, there are spaces for 3 plants. If there are 9 plant options, how many different ways can the plants be arranged? Hint: Are repeats allowed?
Answers
There are different consecutive ways that the flowers can be planted:
3,9,15,21 and so on.
Numbers that follow each other in a regular counting order or pattern are called consecutive numbers. They are written sequentially, where the difference between the numbers is fixed and no number in between is skipped.
Given that:
Roses plants in 1st, 2nd, row are:
3,9 ,.....
Since difference between consecutive terms is same:
It is an AP
We have to find number of row in the flower bed:
Lets assume there are n rows in the flowers bed.
We know that:
aₙ = a + (n-1)d
and d = 9-3 = 6
Therefore, the next numbers can be:
3,9,15 ,21 ......
Learn more about Consecutive :
https://brainly.com/question/30679467
#SPJ4
Can someone please help me with this

Answers
Answer:
I'm pretty sure the correct answer is
D. None of the choices are correct
Step-by-step explanation:
The most likely answer would be AHL ~ NKG, it all depends on the order you put it in. However that is not one of the options so the answer is D.
Hope this helps!
- Quinn <3
Use the given degree of confidence and sample data to construct a confidence interval for the population proportion p.
n=56, x=30; 95% confidence
A. 0.426
Answers
The 95% confidence interval for the population proportion is approximately 0.3573 to 0.7141. None of the given options (A, B, C, D) match the calculated interval.
To construct a confidence interval for the population proportion, we can use the formula:
Confidence Interval = sample proportion ± critical value * standard error
Where the sample proportion (p-hat) is calculated by dividing the number of successes (x) by the total sample size (n). In this case, n = 56 and x = 30, so the sample proportion is 30/56 = 0.5357.
The critical value is determined based on the desired degree of confidence. For a 95% confidence level, which is given in this case, the critical value can be obtained from a standard normal distribution. For a two-tailed test, the critical value is approximately 1.96.
The standard error is calculated by taking the square root of (p-hat * (1 - p-hat) / n). Plugging in the values, we get sqrt(0.5357 * (1 - 0.5357) / 56) = 0.0907.Now, we can construct the confidence interval:Confidence Interval = 0.5357 ± 1.96 * 0.0907Calculating the upper and lower bounds of the interval:
Lower bound = 0.5357 - (1.96 * 0.0907) ≈ 0.3573
Upper bound = 0.5357 + (1.96 * 0.0907) ≈ 0.7141\
for more search question interval
https://brainly.com/question/20309162
#SPJ8
In a survey of 60 first-year college students, 75% of the students said that they wished they had an academic advisor who knew them better. How many students in the survey expressed that wish?
Answers
45 students in the survey expressed the wish that they had an academic advisor who knew them better.
Out of the 60 first-year college students surveyed, 75% of them wished they had an academic advisor who knew them better.
To find out how many students expressed this wish, we can use the formula:number of students = percentage of students × total number of students surveyed
Plugging in the given values, we get:
number of students = 0.75 × 60 = 45
Therefore, 45 students in the survey expressed the wish that they had an academic advisor who knew them better.
To know more about percentage click here
brainly.com/question/16797504
#SPJ11
Michelle purchased a kids table with a square top. If the area of the square top is 400 square inches, what is the length of one of the sides?
Answers
Part A )a painter leans a ladder up against a building. The base of the ladder is placed 7 feet from the building to reach a height of 24 feet. How long is the ladder
Part B) The painter uses the same ladder from part A, but now needs to reach a height of 19 feet.How far away does the base of the ladder need to be placed from the building?

Answers
Answer:
Part A). 25 feet
Part B). 16.25 feet
Step-by-step explanation:
Part A). By applying Pythagoras theorem in ΔABC,
AC² = AB² + BC²
AC² = (24)² + 7²
AC² = 576 + 49
AC = √625
= 25 feet
Part B). By applying Pythagoras theorem in ΔABC,
AC² = AB² + BC²
(25)² = (19)² + x²
625 = 361 + x²
x² = 625 - 361
x = √264
x = 16.25 feet

brainliest for correct answer

Answers
Answer:
x = 21
Step-by-step explanation:
By the corresponding angle theorem, we can find that the measure of the smaller angle made by transversal and m is 54°.
Using the vertical angle theorem, we can find that the missing angle is the triangle, the two known angles are 4x and 2x, is also 54°.
Since the sum of the interior angles of a triangle is always equal to 180°, we can make the equation:
2x + 4x + 54 = 180
Now we solve for x. Subtract 54 from both sides and combine like terms.
6x = 126
Divide both sides by 6.
x = 21
and now we have found the value of x. x = 21.
I hope you find my answer and explanation to be helpful. Happy studying.
Answer:
x=21°
Step-by-step explanation:
Relations Between Angles
We need to recall two basic relations:
* Two angles opposite by crossing lines are always equal.
* The sum of angles in a triangle is 180°
We'll add some variables to the image, so x is easier found. Please check the image below.
The angles U and 54° are opposite and they must be equal, thus U=54°
Since m and n are parallel and they are crossed by a line, the angles V and U are equal, thus V=54°
Finally, The sum of the angles inside the triangle RST must be equal to 180°:
2x+4x+54=180
Joining like terms:
6x=180-54=126
Solving:
x=126/6
x=21°

a snack manufacturer finds that it must increase the salt content of its chips by 8 percent in order for a sample of consumers to notice that the chips are saltier than they were before (baseline). this example most nearly illustrates the concept of a( n ):
Answers
A threshold effect is when a small change in one variable results in a noticeable change in another variable. In this example, a small 8% increase in salt content of the chips is enough to produce a noticeable change in the saltiness of the chips to the sample of consumers.
The snack manufacturer can calculate the increase in salt content of their chips needed to produce the threshold effect by first determining the baseline salt content of the chips. Once this is known, they can then calculate what percentage increase to the salt content is needed by multiplying the baseline salt content by 8%. For example, if the baseline salt content of the chips is 10%, then the 8% increase in salt content would be calculated as 10% x 8% = 0.8%. This means that the snack manufacturer needs to increase the salt content of their chips by 0.8% to reach the threshold effect desired by the sample of consumers.
Learn more about variable here
https://brainly.com/question/29583350
#SPJ4
Thereisalinethatincludesthepoint(0, 19)andhasaslopeof 10/9 . What isitsequationin slope-intercept form?
Answers
Answer: y=(10/9)x+19
Step-by-step explanation:
The slope intercept form is y=mx+b, where m is the slope and b is the y-intercept (the value of y when x=0).
We are given the slope, m:
y=(10/9)x+b
To find b, enter the given point (0,19) and solve for b:
y=(10/9)x+b
19=(10/9)*(0)+b
b = 19
So we have y=(10/9)x+19
what is the are of this circle 8cm
Answers
Answer:
64π(cm)2
Step-by-step explanation:
Answer:
8cm
Step-by-step explanation:
It's 8×0. jbjbgubhibhyo
Find the value of x.

Answers
Answer:
Step-by-step explanation:
Use SOH CAH TOA to recall how the trig functions fit on a triangle
SOH: Sin(Ф)= Opp / Hyp
CAH: Cos(Ф)= Adj / Hyp
TOA: Tan(Ф) = Opp / Adj
The triangles are similar so
x + 46 + 78 = 180
x = 56°
ANSWERRRR QUICKKKK PLEASEEEE

Answers
Answer: sqrt(2)/2 which is choice D
======================================================
Explanation
(3pi/4) radians converts to 135 degrees after multiplying by the conversion factor (180/pi).
The angle 135 degrees is in quadrant 2. We subtract the angle 135 from 180 to find the reference angle
180-135 = 45
Then you can use a 45-45-90 triangle to determine that the ratio of opposite over hypotenuse is sqrt(2)/2
sine is positive in quadrant 2
------------
Alternatively, you can use a unit circle. Refer to the diagram below. In red, I've circled the angle 3pi/4 radians. The terminal point for this angle has a y coordinate of sqrt(2)/2
Recall that y = sin(theta).

Suppose α∈C is a root of the giver irreducible polynomial f(x)∈Q[x].Find the multiplicative inverse of β∈Q[α]
Answers
Suppose α∈C is a root of the giver irreducible polynomial f(x)∈Q[x]. To find the multiplicative inverse of β∈Q[α], we can use the following formula: β^(-1) = 1/β.
Let Q be the field of rational numbers. If α is a root of an irreducible polynomial f(x)∈Q[x], then α is an algebraic number. Therefore, the set Q[α] of numbers of the form r+sα (where r and s are rational numbers) is a field.
Thus, if β∈Q[α], then β^(-1) is also in Q[α].
Let's suppose that β = r+sα. In order to find β^(-1), we can use the following formula:
β^(-1) = (r+sα)^(-1) = 1/(r+sα) = (r-sα)/(r^2 - s^2 α^2).
Therefore, the multiplicative inverse of β∈Q[α] is (r-sα)/(r^2 - s^2 α^2).
Learn more about multiplicative inverse here:
https://brainly.com/question/17300806
#SPJ11
Mr. Baral has a stationery shop. His annual income is Rs 640000. If he is unmarried, how much income tax should he pay? find it
Answers
Mr. Baral has to pay Rs 64000 as an annual income tax at an interest of 10% for his stationary shop.
From the question, we have given that if he is unmarried and his income is between Rs 5,00,001 to Rs 7,00,000, he has to pay an annual interest of 10%.
Given annual income in Rs = 640000.
The annual income tax rate he has to pay at = 10%
So, to find out the income tax from the annual income we have to find out the 10% of 640000.
Income tax = 640000/100 * 10 = 64000
From the above analysis, we can conclude that Mr. Baral has to pay 64000 rs of income tax annually.
To know more about tax calculation,
https://brainly.com/question/31067537
#SPJ1
Given question is not having enough information, I am writing the complete question below:
Use it to calculate the income taxes. For an individual Income slab Up to Rs 5,00,000 0% Rs 5,00,001 to Rs 7,00,000 10% Rs 7,00,001 to Rs 10,00,000 20% Rs 10,00,001 to Rs 20,00,000 30% Tax rate For couple Tax rate 0% Income slab Up to Rs 6,00,000 Rs 6,00,001 to Rs 8,00,000 Rs 8,00,001 to Rs 11,00,000 20% Rs 11,00,001 to Rs 20,00,000 30%
a) Mr. Baral has a stationery shop. His annual income is Rs 6,40,000. If he is unmarried, how much income tax should he pay? 10%
Plot the following co-ordinates and find the fourth point D to make a square A (-1,3) B (1,-1) C(5,1).
Once you have found the missing coordinate use pythagoras to calculate the length of the sides.
Answers
Answer:
1) The fourth point 'D' to make a square is D(3, 5)
2) The length of the side of the square is 2·√5 units
Step-by-step explanation:
1) The coordinates to be plotted are;
A(-1, 3), B(1, -1), C(5, 1)
Using Microsoft Word, the given points are plotted and the point D is found by noting that the sides BC and AD of the square are parallel and equal in length and the point 'C' is 4 units to the right and 2 units upwards, from the point 'B', therefore, the point 'D' will be located at 4 units to the right and 2 units upwards, from the point 'A' which has coordinates of D(3, 5)
The fourth point 'D' to make a square is D(3, 5)
2) Using Pythagoras's theorem, the length of a side of the square (AB) is given as follows;
AB = √((3 - (-1))² + (1 - (-1))²) = √20 = 2·√5
The length of the side of the square, AB = BC = CD = AD = 2·√5


two cards are selected in a sequence from a standard deck. what is the probability that the second card is a jack given that the first card was a 2. (assume the 2 was not replaced.)
Answers
The probability that the second card is a jack given that the first card was a 2 is 52/51.
To calculate the probability that the second card is a jack given that the first card was a 2, we need to consider the remaining cards in the deck after the first card is drawn.
When the first card is drawn and it is a 2, there are 51 cards remaining in the deck, out of which there are 4 jacks.
The probability of drawing a jack as the second card, given that the first card was a 2, can be calculated using conditional probability:
P(Second card is a jack | First card is a 2) = P(Second card is a jack and First card is a 2) / P(First card is a 2)
Since the first card is already known to be a 2, the probability of the second card being a jack and the first card being a 2 is simply the probability of drawing a jack from the remaining 51 cards, which is 4/51.
The probability of the first card being a 2 is simply the probability of drawing a 2 from the initial deck, which is 4/52.
P(Second card is a jack | First card is a 2) = (4/51) / (4/52)
Simplifying the expression:
P(Second card is a jack | First card is a 2) = (4/51) * (52/4)
P(Second card is a jack | First card is a 2) = 52/51
To learn more about probability click here:
brainly.com/question/30736116
#SPJ11
pls help An equation is shown. What is the value of 13x that makes the equation true?
4x + 13x = 4x + 1/4
Answers
Answer:
78 is the answer
Step-by-step explanation:
because 1 =1
Dierections:Factor out the GCF from the following.Make sure your answer is factored completely by taking out any common numbers and or variables

Answers
Answer:
21
Step-by-step explanation:
your mom
help for 50 points!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!

Answers
The slope of the line given the information in the table is 1.
What is the slope?The slope is found in linear functions. The slope measures the rate of change of the dependent variable with respect to the independent variable. In this question, the independent variable is x and the dependent variable is y.
Slope = change in the value of y / change in the value of x
Slope = (0 - - 1) / (1 - 0) = 1
Slope = (1 - 0) / (2 - 1) = 1
Slope = (2 -1 ) / (3 - 2) = 1
Based on the value of the slope, it means that for every one percent change in the value of x, the value of y changes by 1%.
To learn more about slope, please check: https://brainly.com/question/3605446
#SPJ1
Answer:
Step-by-step explanation:
look at the x and axis and the irrational fractions and you should find your slope
Which point would be a solution to the system of linear inequalities shown below?
y>-4x+6 Y>1/3x -7
(9,-7)
(-12,-2)
(12, 1)
(-12,-7)

Answers
The point (9, -7) is the only solution to the system of linear inequalities given.
To determine which point would be a solution to the system of linear inequalities, let's substitute the given points into the inequalities and see which point satisfies both inequalities.
The system of linear inequalities is:
y > -4x + 6
y > (1/3)x - 7
Let's test each given point:
For the point (9, -7):
Substituting the values into the inequalities:
-7 > -4(9) + 6
-7 > -36 + 6
-7 > -30 (True)
-7 > (1/3)(9) - 7
-7 > 3 - 7
-7 > -4 (True)
Since both inequalities are true for the point (9, -7), it is a solution to the system of linear inequalities.
For the point (-12, -2):
Substituting the values into the inequalities:
-2 > -4(-12) + 6
-2 > 48 + 6
-2 > 54 (False)
-2 > (1/3)(-12) - 7
-2 > -4 - 7
-2 > -11 (False)
Since both inequalities are false for the point (-12, -2), it is not a solution to the system of linear inequalities.
For the point (12, 1):
Substituting the values into the inequalities:
1 > -4(12) + 6
1 > -48 + 6
1 > -42 (True)
1 > (1/3)(12) - 7
1 > 4 - 7
1 > -3 (True)
Since both inequalities are true for the point (12, 1), it is a solution to the system of linear inequalities.
For the point (-12, -7):
Substituting the values into the inequalities:
-7 > -4(-12) + 6
-7 > 48 + 6
-7 > 54 (False)
-7 > (1/3)(-12) - 7
-7 > -4 - 7
-7 > -11 (True)
Since one inequality is true and the other is false for the point (-12, -7), it is not a solution to the system of linear inequalities.
In conclusion, the point (9, -7) is the only solution to the system of linear inequalities given.
For similar question on inequalities.
https://brainly.com/question/28755685
#SPJ8
Based on data obtained from the Census Bureau, the number of Americans over age 100 is expected to beP(t) = 0.07e0.54t (0 ≤ t ≤ 4). Where P(t) is measured in millions and t is measured in decades, with t = 0, corresponding to the beginning of 2000.†How fast was the population of americans over age 100 changing at the beginning of 2000? million people/decade
Answers
The population of Americans over age 100 was changing at a rate of 0.0378 million people/decade at the beginning of 2000.
To find how fast the population of Americans over age 100 was changing at the beginning of 2000, we need to take the derivative of the function P(t) with respect to t. The derivative of P(t) with respect to t is given by:
P'(t) = 0.54*0.07e0.54t
At the beginning of 2000, t = 0. So, we need to plug in t = 0 into the derivative to find how fast the population was changing at that time:
P'(0) = 0.54*0.07e0.54*0 = 0.0378
Therefore, the answer will be 0.0378.
know more about population here
https://brainly.com/question/27991860#
#SPJ11
consider these functions:
f(x)=3x-7
g(x)=x+1/x-1
what is the value of f(g(3))
a. -1
b. 0
c. 3
d. 5
Answers
Answer:
Step-by-step explanation:
g(3)= (3+1)/(3-1)= 4/2= 2
f(2)= 3(2)-7 = 6 - 7 = -1
answer is a. -1
find the lateral area of the prism!!
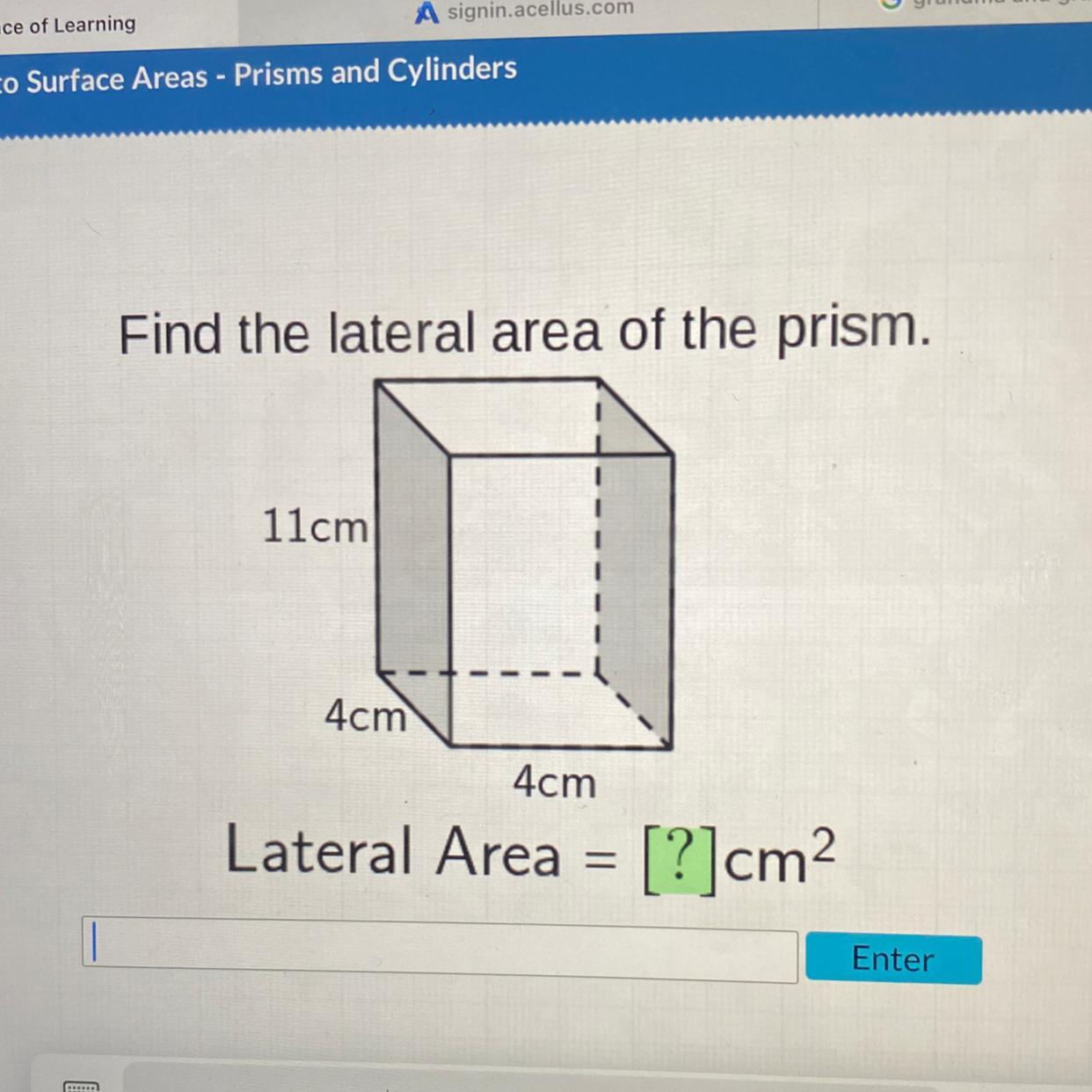
Answers
Check the picture below.
so we only want the area in pink.
\(\stackrel{\textit{left and right}}{2(4)(11)}~~ + ~~\stackrel{\textit{front and back}}{2(4)(11)}\implies \text{\LARGE 176}~cm^2\)

Please tell me the answer for
Factorise 15p square - 7q square
Answers
Answer:
I do not think you can simplify it any further
Step-by-step explanation:
If you mean 15p squared - 7 q squared
Because they have different variables; p and q cannot be combined unless it is multiplication or division.
for incompressible flow in a horizontal pipe of constant diameter and without fittings or valves show that the pressure is a linear function of pipe length.
Answers
For incompressible flow in a horizontal pipe of constant diameter and without fittings or valves, the pressure is a linear function of pipe length.
Linear function:A linear function can be defined as a function that shows a constant rate of change between input values (x) and output values (y). When we plot the graph of a linear function, it shows a straight line. The standard form of a linear function is y = mx + b, where m is the slope and b is the y-intercept.
Pressure:Pressure is defined as the force exerted per unit area. Pressure is a scalar quantity. It can be measured in units such as pascals, bars, pounds per square inch, etc.
Now let's consider the given statement for incompressible flow in a horizontal pipe of constant diameter and without fittings or valves to prove that pressure is a linear function of pipe length.
In a horizontal pipe of constant diameter, the following assumptions are made:No valves and fittings are presentFluid is incompressibleFriction is negligibleThe fluid flow is steady-state and laminarAt two points P1 and P2 along the horizontal pipe, let us assume that the fluid pressure and velocity are P1, V1 and P2, V2, respectively.
The pressure drop between the two points is given byΔP = P1 - P2The flow rate of the fluid through the pipe is given by the equation,Q = (A1V1) = (A2V2)
where, A is the cross-sectional area of the pipe.
Substituting V1 = Q/A1 and V2 = Q/A2 in ΔP = P1 - P2, we getΔP = P1 - P2 = 32μQL / πd4,
where μ is the viscosity of the fluid, L is the length of the pipe, d is the diameter of the pipe.
The above equation is called the Hagen-Poiseuille equation. It shows that pressure drop (ΔP) is proportional to the length of the pipe (L) when the other parameters such as flow rate (Q), viscosity (μ), and diameter (d) of the pipe are constant.
Thus, it can be concluded that the pressure is a linear function of pipe length.
To know more about linear function visit:
https://brainly.com/question/2248255
#SPJ11
In the table below, x represents the miles traveled and y represents the cost to travel by train.
Miles, x
Cost, y
2
8.50
5
15.25
8
22.00
12
31.00
What is the slope of this function?
Answers
Answer:
C
Step-by-step explanation:
The slope of this function would be: C) 2.25.
What is the slope of the function?The slope of the function is obtained with the formula; (y₂ - y₁)/(x₂ - x₁). It is the rate at which the independent variable changes with respect to the dependent variable.
Given the miles and cost of the distance traveled, the slope can be obtained in this way:
(15.25 - 8.50) / (5 - 2)
= 6.75/3
= 2.25
Learn more about the slope of a function here:
https://brainly.com/question/12339452
#SPJ2
Complete Question:
In the table below, x represents the miles traveled and y represents the cost to travel by train. Miles, x Cost, y 2 8.50 5 15.25 8 22.00 12 31.00 What is the slope of this function? A) 0.44
B) 0.63
C) 2.25
D) 22.50
Validation of the model and answering the question "what are my options" occur in the ___ phase of the IDC.
A. choice
B. design
C. intelligence
D. implantation
Answers
Validation of the model and answering the question "what are my options" occur in the design phase of the IDC (Intelligence, Design, and Choice) framework.
The IDC framework is a decision-making process that consists of three phases: Intelligence, Design, and Choice. Each phase corresponds to a specific set of activities and objectives.
In the intelligence phase, the focus is on gathering information, identifying the problem or decision to be made, and understanding the factors and variables involved. This phase involves data collection, analysis, and exploration to gain insights and knowledge about the problem domain.
In the design phase, the emphasis is on developing and evaluating potential options or solutions to address the problem or decision at hand. This phase involves creating models, prototypes, or simulations to represent the problem and exploring different alternatives.
Validation of the model is an important aspect of this phase to ensure that the proposed solutions align with the problem requirements and objectives.
The question "what are my options" is a fundamental question that arises during the design phase. It implies the exploration and generation of various possible choices or solutions that can be evaluated and compared.
Therefore, the design phase of the IDC framework encompasses the activities of validating the model and answering the question "what are my options." It involves refining and testing potential solutions to make informed decisions in the subsequent choice phase.
To know more about variable click here
brainly.com/question/2466865
#SPJ11
una familia ha consumido en un dia de verano: dos botellas de litro y medio de agua, 5 botellas de 1/4 de litro de jjugo de apple, y 4 de 1/4 de litro de limonada ¿cuantos litros de liquido han bebido ?podemos directamente sumar restar fracciones de distintos denominadores
Answers
Answer:
La familia ha bebido en un día de verano \(5\,\frac{1}{4}\) litros.
Step-by-step explanation:
Podemos evidenciar que es posible obtener el volumen total de los citados líquidos al sumar el volumen de cada uno. El volumen de cada bebida es igual al producto de su capacidad multiplicada por el número de botellas. Es decir:
\(V_{total} = V_{agua} + V_{jugo} + V_{limonada}\)
Donde todos los volúmenes se miden en litros.
\(V_{total} = (2\,botellas)\times \left(\frac{3}{2} \,\frac{L}{botella} \right)+(5\,botellas)\times \left(\frac{1}{4}\,\frac{L}{botella} \right) + (4\,botellas)\times \left(\frac{1}{4}\,\frac{L}{botella} \right)\)
\(V_{total} = (2\,botellas)\times \left(\frac{6}{4} \,\frac{L}{botella} \right)+(5\,botellas)\times \left(\frac{1}{4}\,\frac{L}{botella} \right) + (4\,botellas)\times \left(\frac{1}{4}\,\frac{L}{botella} \right)\)
\(V_{total} = \frac{12}{4}\,L + \frac{5}{4} \,L + \frac{4}{4} \,L\)
\(V_{total} = \frac{21}{4}\,L\)
\(V_{total} = \frac{20}{4}\,L + \frac{1}{4}\,L\)
\(V_{total} = 5\,\frac{1}{4}\,L\)
La familia ha bebido en un día de verano \(5\,\frac{1}{4}\) litros.