Answers
Answer:
24
Step-by-step explanation:
First, find the total volume of the shape by multiplying all side lengths:
1 * 2 * (3/2) = 3 cubic cm
A cube with side length of 1/2 has a volume of (1/2)(1/2)(1/2) = 1/8 cubic cm
If the total volume is 3 cubic cm, how many 1/8 cm cubes would fit?
3 / (1/8) = 24 cubes
Related Questions
If 4 tickets to a show cost $9.00, find the cost of 18 tickets.
Answers
If the price of 4 tickets to a show is $9.00 then the cost of 18 tickets is $40.5.
What is an arithmetic operation?The four fundamental operations of arithmetic are addition, subtraction, multiplication, and division of two or more numbers. Included in them is the study of integers, especially the order of operations, which is important for all other aspects of mathematics, notably algebra, information management, and geometry.
From the given data, provided in the question,
Price of 4 tickets = $9
Price of 18 tickets = (18 × $9)/4
Price = $40.5.
To know more about arithmetic operation:
https://brainly.com/question/13585407
#SPJ2
Convert 13/2 to a decimal using long division
Answers
Answer:
i need this awser hurry hp an do it
Step-by-step explanation:
hellppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppppp
can someone help me please???
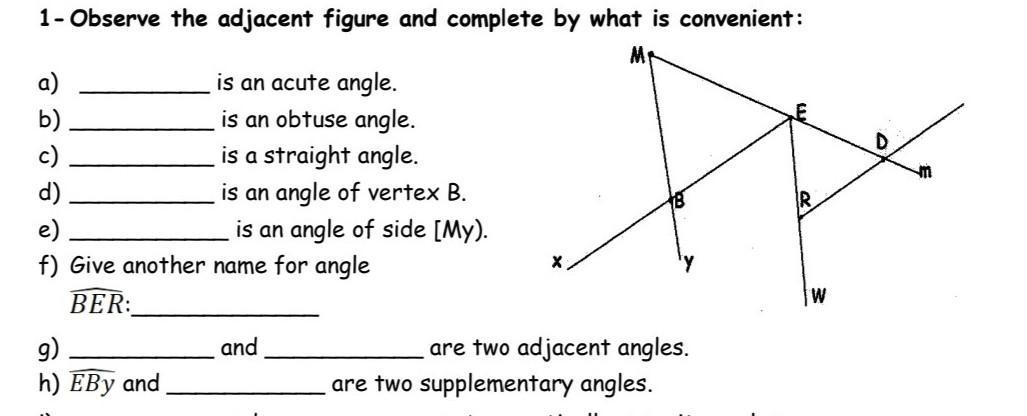
Answers
9514 1404 393
Answer:
a) MBE; b) EBY; c) MBY; d) MBE; e) YME; f) XEW; g) EBM, EBY; h) EBY, EBM
Step-by-step explanation:
There are many choices here. We'll use choices that make as much use as possible of the same points and segments.
a) ∠MBE is an acute angle
b) ∠EBY is an obtuse angle
c) ∠MBY is a straight angle
d) ∠MBE is an angle with vertex B
e) ∠YME is an angle with side MY
f) ∠XEW is another name for ∠BER
g) ∠EBM and ∠EBY are adjacent angles
h) ∠EBY and ∠EBM are supplementary angles
__
Additional comments
An acute angle measures less than 90°; an obtuse angle measures more than 90°. A straight angle measures exactly 180°. A linear pair is a pair of adjacent angles that together measure 180°. That is, they are supplementary and combine to make a straight angle. (Supplementary angles only need to have a sum that is 180°. They do not need to be a linear pair. However, in a figure like this, where angle measures are not marked, angles are guaranteed to be supplementary only if they are a linear pair.)
There are 20 students in Mrs. Tanya's class and 24 kids in Mrs. Olga's class. The students need to be placed on teams of equal size with students from only their own class. What's the largest size of a team?
Answers
The largest size of a team according to the task content is; 20 kids.
What is the largest size of a team?It follows from the task content that the population is; 20 students in Mrs. Tanya's class and 24 kids in Mrs. Olga's class.
However, since, the team forming condition states that students be placed on teams of equal size with students from only their own class.
It therefore follows that the largest size of a team is 20 as only 20 students are in Mrs. Tanya's class hence leaving 4 kids in Mrs. Olga's class without a team.
Read more on limiting factor;
https://brainly.com/question/16479356
#SPJ1
Goals Scored (per game)
There is a [DROP DOWN 1] association between the amount of goals scored and the number of wins a hockey team has. Most of the data points fall between [DROPDOWN 2] goals scored and [DROPDOWN
3] number of wins. Causation (DROPDOWN 4] be established because their relationship was not in a controlled setting.
![Goals Scored (per game)There is a [DROP DOWN 1] association between the amount of goals scored and the](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/Om1ZI94BXgac6eWEJSaoQSe02Jb6tiXS.png)
Answers
There is a Weak positive association between the amount of goals scored and the number of wins a hockey team has. Most of the data points fall between 4 goals scored and 5 number of wins. Causation cannot be established because their relationship was not in a controlled setting.
What is experiment about?When if a relationship between two variables is said to be negative, it is one that does not just necessarily mean that the association is weak. Although though both variables tend to increase in reaction to one another, a weak positive correlation depicts that the relationship is not very strong.
Therefore, even though the data tells us that there is a weak positive relationship that exist between the two variables, it is vital to know that that correlation does not equal causation.
Learn more about data points from
https://brainly.com/question/30588709
#SPJ1
Find the maximum/minimum value of the quadratic function q² + 22q = y - 85 by
completing the square method.
O-36
O-48
C
-24
-64
Answers
Step-by-step explanation:
To find the maximum/minimum value of the quadratic function q² + 22q = y - 85, we can complete the square as follows:
q² + 22q = y - 85
q² + 22q + 121 = y - 85 + 121 (adding (22/2)² = 121 to both sides)
(q + 11)² = y + 36
Now, we have a square of a binomial on the left side, which means the minimum value of the quadratic function is y + 36, and it occurs when (q + 11) = 0. Thus, the minimum value is:
y + 36 = 36 - 85 = -49
Similarly, the maximum value of the quadratic function occurs when (q + 11) = 0, but this time the value of y will be as large as possible. The largest possible value of y occurs when STU is a permutation of the digits 9, 8, and 7 (since PQR must be 4, 0, and 3 in some order). Thus, the maximum value is:
y + 36 = 9 + 8 + 7 - 85 + 36 = -25
Therefore, the options are incorrect and the correct answers are:
Minimum value: -49
Maximum value: -25
Hi please help on question! . If answer is correct I'll rate you five stars a thanks and maybe even brainliest! You will even get 39 pts!!
Here is a function machine.
Input : multiply by 6. Subtract 80: output
The input is the same as the output. Find the input.
Also can you please show me an easy to work out these type of questions
Answers
The input (x) that satisfies the condition of being multiplied by 6 and then Subtracted by 80 to give the same value as the output is 16.
The input as "x." According to the given information, the input (x) is multiplied by 6 and then subtracted by 80, resulting in the output. In mathematical terms, this can be expressed as:
Output = (6 * x) - 80
The problem states that the input and output are the same. Therefore, we can set up the equation:
x = (6 * x) - 80
To solve for x, we'll rearrange the equation and isolate the variable:
x - 6 * x = -80
Combine like terms:
-5 * x = -80
Divide both sides by -5:
x = (-80) / (-5)
Simplifying the division:
x = 16
Hence, the input (x) that satisfies the condition of being multiplied by 6 and then subtracted by 80 to give the same value as the output is 16.
For more questions on Subtracted .
https://brainly.com/question/31129970
#SPJ8
Una versión muy simplificada de una tabla de insumo-producto para la economía de Israel en 1958 divide dicha economía en tres sectores agricultura, manufactura y energía con los si-guientes resultados.
a) ¿Cuántas unidades de producción agrícola se requieren para obtener una unidad de produc-to agrícola?
b) ¿Cuántas unidades de producción agrícola se requieren para obtener 200 000 unidades de productos de esta naturaleza?
c) ¿Cuántas unidades de producción agrícola se requieren para obtener 50 000 unidades de energía?
d) ¿Cuántas unidades de energía se requieren para obtener 50 000 unidades de productos agrí-colas?

Answers
Note that:
a) 1 unit of agricultural production required to obtain 1 agricultural product.
b) 200,000 units of agricultural production are required to obtain 1 of agricultural product.
c) 22,727 units of agricultural production are required to obtain 50,000 units of energy.
d) we need 27,825 units of energy to produce 50,000 units of agricultural products.
How did we get the aabove answer?a) According to the table, the coefficient of production for agriculture is 0.293, that is
0.293 units of agricultural production = a unit of agricultural product.
b) To obtain 200,000 units of agricultural products we say
0.293 x 200,000,= 58,600 units of agricultural production.
c) the coefficient of production for energy with respect to agriculture is 0.044,
that is ,
0.044 units of agricultural production = a unit of energy.
So
50,000 units of energy,=
0.044 by 50,000,
= 2,200 units of agricultural production.
d) To obtain 50,000 units of agricultural products
use the Leontief inverse of the input-output table, which is simply the inverse of the coefficient matrix.
[ 3.4175 -0.2345 -0.5565 ]
[-0.1894 4.8264 -0.2114 ]
[-0.3074 0.1772 4.8324 ]
[ (3.4175 x0) + (-0.2345 x 0) + (-0.5565 x 50,000) ]
[ (-0.1894 x0) + (4.8264x 0) + (-0.2114 x50,000) ]
[ (-0.3074 x0) + (0.1772 x0) + (4.8324 x50,000) ]
[ -27,825 ]
[ -10,570 ]
[ 241,620 ]
Thus, we need 27,825 units of energy to produce 50,000 units of agricultural products.
Learn more about agricultural production
https://brainly.com/question/17711566
#SPJ1
Hi help pls no link ty!

Answers
Answer:
84.78
Step-by-step explanation:
The full area of the circle without the cutout is 113.04
The cutout sector is 28.26
So that would make our final answer 84.78
by subtracting 28.26(cutout) from 113.04(full circle)
(In case you are wondering) The way I found the cutout sector's area was this formula: π4²·(angle)/360
can some please help me id really appreciate it i’ll give you brainliest

Answers
Answer:
i cant really see the picture but i think its c
Step-by-step explanation:
if not c i think a
If A=(4,-5) and B=(7,-9), what is the length of AB
Answers
The length of line segment AB is 5 units.To find the length of line segment AB, we can use the distance formula, which is based on the Pythagorean theorem.
The distance formula calculates the distance between two points (x1, y1) and (x2, y2) in a coordinate plane.
Let's calculate the length of AB using the given coordinates for points A and B:
Coordinates of point A: A = (4, -5)
Coordinates of point B: B = (7, -9)
The distance formula is given by:
\(d = [(x2 - x1)^2 + (y2 - y1)^2]\)
Substituting the coordinates of A and B into the formula:
\(d = [(7 - 4)^2 + (-9 - (-5))^2]\\d =[(3)^2 + (-4)^2]\)
d = √[9 + 16]
d = √25
d = 5
Therefore, the length of line segment AB is 5 units.
The distance formula calculates the straight-line distance between two points in a two-dimensional space. In this case, it determines the distance between points A and B in the coordinate plane. By applying the formula and substituting the given coordinates, we find that the length of AB is 5 units.
For more such questions on distance formula.
https://brainly.com/question/661229
#SPJ8
A marketing research firm asked people how many times they visited the mall last month
Answers
Answer:
Can you add more context?
Step-by-step explanation:
sin^4x rewrite the power as a product of two squared terms
Answers
sin^4x as a product of two squared terms is sin^2(x) * sin^2(x)
How to rewrite the expression?The sine expression is given as
sin^4x
The above means
sin x raised to the power of 4
This in other words, the expression is represented as
(sin(x))^4
Express 4 as 2 + 2
(sin(x))^(2 + 2)
Apply the law of indices
(sin(x))^2 * (sin(x))^2
This gives
sin^2(x) * sin^2(x)
Hence, sin^4x as a product of two squared terms is sin^2(x) * sin^2(x)
Read more about products at:
https://brainly.com/question/10873737
#SPJ1
6. When 4 times the number x is added to 12, the result is 8. What number results when 2
times x is added to 7?
Answers
Answer: 5
Step-by-step:
1. set up equations : 4x+12=8 and 2x+7=?
2. solve for x in the first eq.
4x + 12 = 8
4x = -4
x = -1
3. plug x = -1 into 2x+7=?
2 ( -1 ) + 7 =
-2 + 7 =
5
What lis the length of bc?

Answers
Answer: C (23)
Step-by-step explanation:
Since the triangle is isosceles, BA = BC
x + 17 = 2x -6
x = 23
Answer:
C(23)
Step-by-step explanation:
Since line AB = line BC
x+17=2x-6, by collecting like terms x=23
Identify the unit rate in the graph.
A) 40 mph
B) 75 mph
C) 50 mph
D) 25 mph

Answers
C)50mph
speed= distance÷time
so ....
we find how much 25 miles took therefore we do
25÷0.5
which is
=50
Hope this helped you, have a good day bro cya)
Answer:
fast
Step-by-step explanation:
historical definition
Italian physicist Galileo Galilei is usually credited with being the first to measure speed by considering the distance covered and the time it takes. Galileo defined speed as the distance covered per unit of time.[3] In equation form, that is
{\displaystyle v={\frac {d}{t}},}v={\frac {d}{t}},
where {\displaystyle v}v is speed, {\displaystyle d}d is distance, and {\displaystyle t}t is time. A cyclist who covers 30 metres in a time of 2 seconds, for example, has a speed of 15 metres per second. Objects in motion often have variations in speed (a car might travel along a street at 50 km/h, slow to 0 km/h, and then reach 30 km/h).
Instantaneous speed
Speed at some instant, or assumed constant during a very short period of time, is called instantaneous speed. By looking at a speedometer, one can read the instantaneous speed of a car at any instant.[3] A car travelling at 50 km/h generally goes for less than one hour at a constant speed, but if it did go at that speed for a full hour, it would travel 50 km. If the vehicle continued at that speed for half an hour, it would cover half that distance (25 km). If it continued for only one minute, it would cover about 833 m.
In mathematical terms, the instantaneous speed {\displaystyle v}v is defined as the magnitude of the instantaneous velocity {\displaystyle {\boldsymbol {v}}}{\boldsymbol {v}}, that is, the derivative of the position {\displaystyle {\boldsymbol {r}}}{\boldsymbol {r}} with respect to time:[2][4]
{\displaystyle v=\left|{\boldsymbol {v}}\right|=\left|{\dot {\boldsymbol {r}}}\right|=\left|{\frac {d{\boldsymbol {r}}}{dt}}\right|\,.}v=\left|{\boldsymbol v}\right|=\left|{\dot {{\boldsymbol r}}}\right|=\left|{\frac {d{\boldsymbol r}}{dt}}\right|\,.
If {\displaystyle s}s is the length of the path (also known as the distance) travelled until time {\displaystyle t}t, the speed equals the time derivative of {\displaystyle s}s:[2]
{\displaystyle v={\frac {ds}{dt}}.}v={\frac {ds}{dt}}.
In the special case where the velocity is constant (that is, constant speed in a straight line), this can be simplified to {\displaystyle v=s/t}v=s/t. The average speed over a finite time interval is the total distance travelled divided by the time duration.
Average speed
Different from instantaneous speed, average speed is defined as the total distance covered divided by the time interval. For example, if a distance of 80 kilometres is driven in 1 hour, the average speed is 80 kilometres per hour. Likewise, if 320 kilometres are travelled in 4 hours, the average speed is also 80 kilometres per hour. When a distance in kilometres (km) is divided by a time in hours (h), the result is in kilometres per hour (km/h).
Average speed does not describe the speed variations that may have taken place during shorter time intervals (as it is the entire distance covered divided by the total time of travel), and so average speed is often quite different from a value of instantaneous speed.[3] If the average speed and the time of travel are known, the distance travelled can be calculated by rearranging the definition to
{\displaystyle d={\boldsymbol {\bar {v}}}t\,.}d={\boldsymbol {{\bar {v}}}}t\,.
Using this equation for an average speed of 80 kilometres per hour on a 4-hour trip, the distance covered is found to be 320 kilometres.
Expressed in graphical language, the slope of a tangent line at any point of a distance-time graph is the instantaneous speed at this point, while the slope of a chord line of the same graph is the average speed during the time interval covered by the chord. Average speed of an object is Vav = s÷t
Difference between speed and velocity
Speed denotes only how fast an object is moving, whereas velocity describes both how fast and in which direction the object is moving.[5] If a car is said to travel at 60 km/h, its speed has been specified. However, if the car is said to move at 60 km/h to the north, its velocity has now been specified.
The big difference can be discerned when considering movement around a circle. When something moves in a circular path and returns to its starting point, its average velocity is zero, but its average speed is found by dividing the circumference of the circle by the time taken to move around the circle. This is because the average velocity is calculated by considering only the displacement between the starting and end points, whereas the average speed considers only the total distance travelled.
A local hamburger shop sold a combined total of 688 hamburgers and cheeseburgers on Monday. There were 62 fewer cheeseburgers sold than hamburgers. How many hamburgers were sold on Monday? I hamburgers
Answers
Answer: 375 hamburgers
Step-by-step explanation:
Let's assume that x is the number of hamburgers sold on Monday.
According to the problem, the number of cheeseburgers sold is 62 fewer than the number of hamburgers sold. So the number of cheeseburgers sold is x-62.
The total number of hamburgers and cheeseburgers sold is 688. So we can set up an equation:
x + (x-62) = 688
Simplifying this equation, we get:
2x - 62 = 688
Adding 62 to both sides, we get:
2x = 750
Dividing both sides by 2, we get:
x = 375
Therefore, 375 hamburgers were sold on Monday.
Assume a bank loan requires an interest payment of $85 per year and a principal payment of $1,000 at the end of the loan's eight-year life. What would be the present value of this loan if it carried a 10% interest rate?
Answers
Answer:
The present value is \(PV = \$ 396,987\)
Step-by-step explanation:
From the question we are told that
The interest payment per year is \(C = \$ 85\)
The principal payment is \(P = \$ 1000\)
The duration is n = 8 years
The interest rate is \(r = 10\% = 0.10\)
The present value is mathematically represented as
\(PV = [ \frac{C}{r} * [1 - \frac{1 }{ (1 +r)^n} ] + \frac{P}{(1 + r)^n} ]\)
substituting values
\(PV = [ \frac{85}{0.10} * [1 - \frac{1 }{ (1 +0.10)^8} ] + \frac{1000}{(1 + 0.10)^ 8} ]\)
\(PV = \$ 396,987\)
WILL GIVE BRAINLIEST
Find the exact value of the indicated trigonometric functions using the given information.
Given: tan a=2.4, and π/2Find the exact value of the indicated trigonometric functions using the given information.
Given: tan a=2.4, and π/2find sin a and cot a
Answers
Answer:
Viewed as a right angled triangle
tan
(
x
)
=
5
12
can be thought of as the ratio of opposite to adjacent sides in a triangle with sides
5
,
12
and
13
(where
13
is derived from the Pythagorean Theorem)
So
sin
(
x
)
=
5
13
and
cos
(
x
)
=
12
13
Step-by-step explanation:
3 Jack walk from Santa Clara to Polo Allo. Il took I hour 25 min to walk from Santa Clot to Los Altos. Than it took 25 minute of wal from los altos to Palo buto. He arrived in Palo alto at 2:45 P.M. of what time die Santa Clara ? he leave Santa clara
Answers
The time Jack left Santa Clara is 1 : 55 pm
What is word problem?A word problem in math is a math question written as one sentence or more. These statements are interpreted into mathematical equation or expression.
The time for Jack to walk to lose Altos is 25 min and he uses another 25mins to work to Palo alto.
Therefore, the total time he spent is
25mins + 25 mins = 50 mins
He arrived Palo at 2 :45 pm, therefore the time he left Santa Clare will be ;
2:45 pm = 14 :45
= 14:45 - 50mins
= 13:55
= 1 : 55pm
Therefore he left at 1:55 pm
learn more about word problem from
https://brainly.com/question/21405634
#SPJ1
Find the volume of a sphere with a diameter of 6.2 in.
Answers
Answer: its 23 meters
Step-by-step explanation:
The volume of a sphere with a diameter of 6.2 in is 39.72π
What is the volume of the sphere?The volume of a sphere is defined as the total amount of capacity applied in a sphere that can be calculated using the volume formula for the sphere which is V = (4/3)πr3.
The radius of the sphere is;
\(\rm Radius =\dfrac{Diameter}{2}\\\\Radius=\dfrac{6.2}{2}\\\\Radius=3.1\)
The volume of the sphere is;
\(\rm Volume =\dfrac{4}{3}\pi r^3\\\\Volume =\dfrac{4}{3}\pi (3.1)^3\\\\Volume = 39.72\pi\)
Hence, the volume of a sphere with a diameter of 6.2 in is 39.72π.
Learn more about sphere here;
https://brainly.com/question/9994313
#SPJ2
Determine the value of the variables a, b, and when simplifying and evaluating the expression. 3/^8 3/^4 =a^b= c
a=
b=
c=
Answers
Answer:
A=3
B=4
C=81
Step-by-step explanation:
Just did it
Answer:
a =
✔ 3
b =
✔ 4
c =
✔ 81
blake bike east at 5m/s. five seconds later he speeds up to 9 m/s.
what is his change in velocity? what is his acceleration?
Answers
Answer:
The answer is down below
Step-by-step explanation:
v-u=◇velocity
\( = 9 - 5\)
◇velocity =4ms‐¹
acceleration =◇velocity/time
\(a = \frac{4}{5} \)
\(a = 0.8m {s}^{ - 2} \)
Change in velocity:
\( \sf \:final \: velocity - initial \: velocity = change \: in \: velocity\)
\( \therefore \tt \delta \: v = 9 - 5 \\ \tt = 4m {s}^{ - 1} \)
To find acceleration:
\( \rm \: a = \frac{ \triangle \: v}{\triangle \: t} \)
\( \rm \: a = \frac{ 4}{5 - 0} = \frac{4}{5} = 0.8m {s}^{ - 1} \)
I NEED THE ANSWER NOW PLS HELP
A tank full of water is leaking at a constant rate for 30 minutes.
The equation y=-9x + 500 gives the amount of water in the tank, where y is the number of liters and xis
the number of minutes since the tank began to leak.
Which statement is true?
A. There were 9 liters in the tank when the leak started, and it is
decreasing by 500 liters per minute.
B. There were 500 liters in the tank when the leak started, and it is
decreasing by 9 liters per minute.
C. There were 9 liters in the tank when the leak started, and it is
increasing by 500 liters per minute.
D. There were 500 liters in the tank after 30 minutes, and it is decreasing by 9 liters per minute.
Answers
Answer:
B
Step-by-step explanation:
have a good day man :D
Answer: what about if the numbers were y= -7x + 300?
Step-by-step explanation: That’s what it shows me on the test
The plumber charged Mrs. Evans $225.00 for materials and $35.00 an hour for labor to fix her leaky sink. The total bill came to $347.50. How many hours did it take the plumber to fix the sink?
Answers
Answer:
3.5 or 3\(\frac{1}{2}\)
Step-by-step explanation:
First, subtract. 347.50 - 225.00 = 122.50
Then, divide. 122.50 ÷ 35 = 3.5
Next, you can turn the decimal into a fraction 3\(\frac{1}{2}\)
Lastly, have a wonderful day! :)
What is the volume of the following rectangular prism? 3 1/3 1 2/5
Answers
Area of rectangular prism: Base x Height.
so 3x1/3x2/5=0.4 0r 2/5
The textbook for a course in basic mathematics costs $35. There are 34 students in the class. Find the total cost of the textbooks for this class.
Answers
Answer:
1190 notebooks
Step-by-step explanation:
Cost per notebook * number of students
35 * 34 = 1190
35*30=1050
35*4 = 140
35*34 = 1050 + 140 = 1190
So the answer is 1190 notebooks.
Can someone please solve this for me

Answers
Answer:
1.3136363636363637
Step-by-step explanation:
Bc u times 17 times 17 devide by 220
The perimeter of a triangle is 37 inches. The second side is 3 times as long as the first
side. The third side is 2 inches longer than the second side. Find the length of each
side. Show your work.
help asap
Answers
Answer:
5 in, 15 in, 17 in
Step-by-step explanation:
Let x = the first side
the second side = 3x
the third side = 3x + 2
The perimeter = x + 3x + (3x + 2) = 7x + 2 = 37
7x = 37 - 2 = 35
x = 35/7 = 5 inches
3x = 3*5 = 15 inches
3x + 2 = 17 inches
Here is a system of equations. y = - 3x - 4, y = - x - 2 Graph the system. Then write its solution Note that you can also answer No solutionor "Infinitely many" solutions
Answers
Answer;
\((-1,-1)\)Explanation;
Here, we want to get the solution a pair of linear equation which we are to solve simultaneously by the graphical method
We need to plot the graphs of these two lines; The point at which the lines meet will represent the solution to the system of linear equations
The general equation of a straight line is;
\(y=mx\text{ + b}\)where m is the slope and b is the y-intercept
To plot the lines, we need the x-intercepts and the y-intercepts
This refer to the point at which the line touches the x and y axes respectively
Let us take the lines one after the other;
\(y=-3x-4\)The y-intercept here is -4; so the point is (0,-4)
To get the x-intercept value, we simply set y to zero and get the value of x
\(\begin{gathered} 0=-3x-4 \\ -3x=4 \\ x\text{ = }\frac{-4}{3} \end{gathered}\)So the x-intercept is (-4/3,0)
To plot the line; we simply join (0,-4) and (-4/3,0)
For the second line;
We have the y-intercept as -2
So the point is (0,-2)
To get the x-intercept, we simply set y to 0 and solve for x
\(\begin{gathered} 0=-x-2 \\ x=-2 \end{gathered}\)So, the x-intercept point is (-2,0)
We can now join (0,-2) and (-2,0) to represent the second line
Proceeding, we get the lines on the cartesian grid
This is shown in the attachment below;
As we can see from the plot, the lines touch at the point (-1,-1) and that represents the solution to the equation

