p (x) = (x^2+6x+9) (x+3) how many distinct real number roots does p have? what is the smallest real root of p?
Answers
Related Questions
for the independent-measures t statistic, what is the effect of increasing the difference between sample means? group of answer choices increase the likelihood of rejecting the null and increase measures of effect size increase the likelihood of rejecting the null and decrease measures of effect size decrease the likelihood of rejecting the null and increase measures of effect size decrease the likelihood of rejecting the null and decrease measures of effect size
Answers
Choose:
Increase the likelihood of rejecting the null hypothesis and increase measures of effect size.
T STATISTICThe t statistic is the ratio of the calculated deviation of a parameter from its hypothesized value with its standard error.
In general, the likelihood of discovering a significant difference increases as the variance of the difference scores increases. A limited variation for the difference scores in a repeated-measures study suggests that the treatment has little or no effect.
Increasing the number of scores within each sample reduces the predicted standard error of the mean difference. This increases the size of the observed t, making it easier to reject the null hypothesis H0. In other words, when sample size increases, statistical power increases.
Higher t-value corresponds to a lower p-value, indicating that the difference between sample-mean (Χ) and population-mean (μ) is statistically significant (hence we reject the null hypothesis).
Learn more about t-statistic here:
https://brainly.com/question/15236063
#SPJ4
Write in equation in slope form of the line shown

Answers
Answer:
y=2x-5
Step-by-step explanation:
Slope intercept form is y=mx+b, with m being the slope and b being the y-intercept.
Slope is found through (y2-y1)/(x2-x1) and when the values are plugged in you get (-3-1)/(1-3) which equals -4/-2 or 2.
y=2x+b
Since b is the y-intercept, you plug in the value of the point where the line crosses over the y axis. In this case, that value is -5, so you get y=2x-5.
24 shirts cost $259.36. Find the unit rate.
Answers
The unit rate for each shirt if 24 shirts cost $259.36 as required to be determined is; $10.81.
What is the unit rate for each shirt?It follows from the task content that the unit rate is to be determined if 24 shirts cost $259.36.
On this note, it follows that; the unit rate is given by the quotient;
Unit rate = Total cost / No. of units
Unit rate = 259.36 / 24
Unit rate = 10.80667.
Ultimately, the unit rate of the shirts as required is; $10.81.
Read more on unit rate;
https://brainly.com/question/19493296
#SPJ1
\(9x^2-4\)
please simplify!
Answers
Answer:
(3x+2)(3x−2)
Step-by-step explanation:
3+5 is an example of?
Expression
Equation
Inequality
Answers
Answer:
Equation I believe is the answer
let me know if not
How many sides does a regular polygon have when each side is 30 degrees
Answers
The total number of sides of a regular polygon with each exterior angle of measure 30 degrees is equal to 12.
Let us consider 'n' be the number of sides of the regular polygon.
Let 'y' be the measure of each of the exterior angle of regular polygon.
y = 30 degrees
Measure of each of the exterior angle of regular polygon 'y'
= ( 360° ) / n
⇒ n = ( 360° / y )
Substitute the value we get,
⇒ n = ( 360° / 30° )
⇒ n = 12
Therefore, the number of sides of a regular polygon with each of the exterior angle 30 degrees is equal to 12.
Learn more about regular polygon here
brainly.com/question/12092158
#SPJ4
The above question is incomplete, the complete question is:
How many sides does a regular polygon have when each exterior angle measures 30 degrees?
A wild animal preserve can support no more than 200 elephants. 30 elephants were known to be in the preserve in 1980. Assume that the rate of growth of the population is proportional to how close the population is to this maximum, with a growth constant of 0.01 and time measured in years. (a) Set up a differential equation and solve it to show why the number of elephants can be modeled by the function y(t) = 200 - 170e-0.017. (b) Using the answer in (a), how long will it take for the elephant population to double from the number in 1980? Round your answer to 2 decimal places.
Answers
It will take approximately 32.11 years for the elephant population to double from the number in 1980.
Let's set up the differential equation to model the population growth. We assume that the rate of change of the population is proportional to the difference between the maximum capacity (200 elephants) and the current population (y elephants) with a growth constant of 0.01. This can be expressed as:
dy/dt = k(200 - y),
where dy/dt represents the rate of change of the population with respect to time, and k is the growth constant.
To solve this differential equation, we separate the variables and integrate:
∫(dy / (200 - y)) = ∫k dt.
Using partial fraction decomposition and integrating, we find
- ln|200 - y| = kt + C,
where C is the constant of integration.
Next, we can solve for y(t) by isolating y in the equation:
200 - y = Ce^(-kt).
Given that y(0) = 30 (number of elephants in 1980), we can substitute the initial condition into the equation:
200 - 30 = Ce^(-k * 0),
170 = C.
Plugging this value back into the equation, we have:
200 - y = 170e^(-kt).
Simplifying, we obtain the equation for the number of elephants as a function of time:
y(t) = 200 - 170e^(-0.017t).
To determine how long it will take for the population to double from the number in 1980 (30 elephants), we solve the equation y(t) = 2 * y(0):
200 - 170e^(-0.017t) = 2 * 30,
170e^(-0.017t) = 140,
e^(-0.017t) = 140/170,
e^(-0.017t) = 0.8235.
Taking the natural logarithm of both sides, we get:
-0.017t = ln(0.8235),
t ≈ -ln(0.8235)/0.017,
t ≈ 32.11.
Rounding to 2 decimal places, it will take approximately 32.11 years for the elephant population to double from the number in 1980.
Learn more about differential equation here:
https://brainly.com/question/31492438
#SPJ11
You have won a new PS 5 system in a rafflel With a bit of research, you have determined that the cost of a new PS 5 system is $712 with tax. If you know that the value of gaming consoles decreases each year by 12%, based on the previous year's value, what will your PS 5 system be worth after 6 years?
Answers
The salvage value of the PS 5 system after 6 years will be $640.
Explain the term depreciation?Depreciation is the estimated decrease in value of fixed assets over the course of a fiscal year. The amount of an asset's value has indeed been used is shown through depreciation. It enables businesses to purchase assets over a predetermined length of time and generate income from those assets.The formula for the depreciation;
Depreciation per year = (cost of asset - salvage value)/ (useful life of assets)
For the stated question-
Cost of new PS 5 system = $712.salvage value to be find.Depreciation per year = 12%useful life of assets = 6 years.12 = (712 - salvage value)/ (6)
salvage value = 712 - 12x6
salvage value = $640.
Thus, the salvage value of the PS 5 system after 6 years will be $640.
To know more about the depreciation, here
https://brainly.com/question/25806993
#SPJ1
12 Need help. I can’t fail this!!

Answers
reason:
the difference or the triangles is 1/3
Answer:it’s D
Step-by-step explanation:

help what’s the answer

Answers
asif has a bag of 58 sweets he gives 5 of his sweets to each of his 6 friends how many sweets does he have left
Answers
Answer:28 if I’m not mistaken
Step-by-step explanation:
=58 - 30
=28
explain if you can
PART A: What percent of the students who have a job, do not have a driver’s license?
A. 23.66%
B. 18.28%
C. 91.23%
D. 81.72%
PART B: Given that a student does not have a job, what is the relative frequency that he has a driver's license?
A. 87%
B. 91.2%
C. 40.6%
D. 34.7%

Answers
write 0.216 as a rational number in lowest terms
Answers
Answer:
\(=\frac{27}{125}\)
Step-by-step explanation:
\(0.216\)
\(\mathrm{Multiply\:and\:divide\:by\:10\:for\:every\:number\:after\:the\:decimal\:point.}\)
\(\mathrm{There\:are\:}3\mathrm{\:digits\:to\:the\:right\:of\:the\:decimal\:point,\:therefore\:multiply\:and\:divide\:by\:}1000\)
\(=\frac{0.216\cdot \:1000}{1000}\)
\(=\frac{216}{1000}\)
\(216,\:1000\)
\(\mathrm{The\:GCD\:of\:}a,\:b\mathrm{\:is\:the\:largest\:positive\:number\:that\:divides\:both\:}a\mathrm{\:and\:}b\mathrm{\:without\:a\:remainder}\)
\(2,\:3\mathrm{\:are\:all\:prime\:numbers,\:therefore\:no\:further\:factorization\:is\:possible}\)
\(=2\cdot \:2\cdot \:2\cdot \:3\cdot \:3\cdot \:3\)
\(2,\:5\mathrm{\:are\:all\:prime\:numbers,\:therefore\:no\:further\:factorization\:is\:possible}\)
\(=2\cdot \:2\cdot \:2\cdot \:5\cdot \:5\cdot \:5\)
\(\mathrm{The\:prime\:factors\:common\:to\:}216,\:1000\mathrm{\:are\:}\)
\(=2\cdot \:2\cdot \:2\)
\(\mathrm{Multiply\:the\:numbers:}\:2\cdot \:2\cdot \:2=8\)
\(=8\)
\(=\frac{8\cdot \:27}{8\cdot \:125}\)
\(\mathrm{Cancel\:the\:common\:factor:}\:8\)
\(=\frac{27}{125}\)
Hence, the correct answer is \(=\frac{27}{125}\)
Cuál es el menor número entero que, disminuido en 8, resulta menor que su triple
Answers
Answer:
-3
Step-by-step explanation:
Let x = the number
Then 3x = its triple, and the condition is:
x - 8 < 3x
Subtract x from each side
-8 < 2x
Divide each side by 2
-4 < x
Reverse the inequality
x > -4
The smallest integer greater than -4 is -3.
Check:
-3 - 8 < 3(-3)
-11 < -9
It checks.
The chef at LaBerge started the week with 50 pounds of cheese and used 15 4/5 pounds. How much cheese
remains in inventory?
Answers
Answer: 34.2 pounds
Step-by-step explanation:
First, convert 15 4/5 into an improper fraction. You should get 79/5. Then, convert 50 into a fraction that has a denominator of 5. Right now, 50= 50/1 so just multiply the top and bottom by 5 to make it 250/5. Then, subtract 79/5 from 250/5. You should get 171/5. Lastly, simplify. You should get 34.2 pounds as your final answer. Hope this helps!
.............................................

Answers
Answer:
The value would be 100
Step-by-step explanation:
true or false: the symbol in boolean algebra means regular addition as in linear regular algebra
Answers
Answer: False
Step-by-step explanation:
In Boolean algebra, the symbol "+" represents logical OR operation, not regular addition as in linear algebra. The logical OR operation is used to combine two or more logical statements into a single statement that is true if at least one of the statements is true.
For example, if A and B are two logical statements, then A+B is true if A is true, or B is true, or both A and B are true.
Therefore, it is important to understand the context in which the symbol "+" is being used, whether it is in the context of regular addition or logical OR operation.
please whats the answer to this question
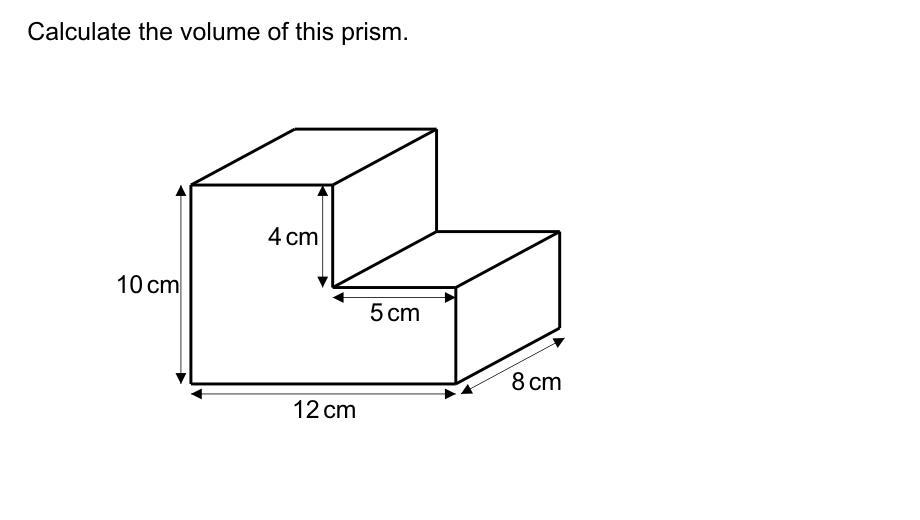
Answers
Answer:
\(800~cm^3\)
Step-by-step explanation:
\(Volume ~of A=(10*(12-5)*5=\)
\(10*7*8=\)\(560 ~cm^3\)\(Volume~ of ~B= 5*8(10-4)=\)
\(5*8*6=\)\(40*6=\)\(240~cm^3\)\(Total ~volume= A+B\)
\(560+240=\)
\(800~cm^3\)
-----------------------------
hope it helps...
have a great day!!
For the four points P(k, 1), Q(-2,-3), R(2, 3) and S(1,k), it is known that PQ is parallel to RS. Find
the possible values of k.
Answers
Answer:
Solution is in attached photo.
Step-by-step explanation:
Do take note for this question, since PQ and RS are parallel, they have the same slope.

Answer this question correctly for 10 pts and brainlesit! be first :)
Answers
What is the question?
The 3-sigma limits for a process whose distribution conforms to the normal distribution includes _______ of the observed values, in the long run.
Answers
The 3-sigma limits for a process whose distribution conforms to the normal distribution include 99.74% of the observed values, in the long run. This means that if you have a process that is normally distributed, then, in the long run, 99.74% of the values will fall within three standard deviations of the mean.
This is an important concept to understand because it can be used to help control a process. For example, if you know that 99.74% of the values will fall within three standard deviations of the mean, then you can set up control limits that correspond to those values. This can help you ensure that your process stays within the desired range.
Understanding the 3-sigma limits can help you better control your process and ensure that it produces the desired results.
For more information on 3-sigma limits click on the link below:
https://brainly.com/question/17063477
#SPJ4
which number represents the opposite of -25
Answers
Two factory plants are making TV panels. Yesterday, Plant A produced 3000 fewer panels than Plant B did. Two percent of the panels from Plant A and 5% of the panels from Plant B were defective. How many panels did Plant B produce, if the two plants together produced 570 defective panels?
Answers
Answer:
9000 panels
Step-by-step explanation:
See attached for detailed explanation.
Remember that percent means division by a 100.

Pls help will
Give brainlist

Answers
Answer:
hhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhhh
Step-by-step explanation:
Answer:
40%
Step-by-step explanation:
Total girls
= 12 + 18
= 30
Girls who prefer soccer =
12
Percentage =
12/30
= 0.4
= 40%
Question 9) Use the indicated steps to solve the heat equation: k ∂²u/∂x²=∂u/∂t 0 0 ax at subject to boundary conditions u(0,t) = 0, u(L,t) = 0, u(x,0) = x, 0
Answers
The final solution is: u(x,t) = Σ (-1)^n (2L)/(nπ)^2 sin(nπx/L) exp(-k n^2 π^2 t/L^2).
To solve the heat equation:
k ∂²u/∂x² = ∂u/∂t
subject to boundary conditions u(0,t) = 0, u(L,t) = 0, and initial condition u(x,0) = x,
we can use separation of variables method as follows:
Assume a solution of the form: u(x,t) = X(x)T(t)
Substitute the above expression into the heat equation:
k X''(x)T(t) = X(x)T'(t)
Divide both sides by X(x)T(t):
k X''(x)/X(x) = T'(t)/T(t) = λ (some constant)
Solve for X(x) by assuming that k λ is a positive constant:
X''(x) + λ X(x) = 0
Applying the boundary conditions u(0,t) = 0, u(L,t) = 0 leads to the following solutions:
X(x) = sin(nπx/L) with n = 1, 2, 3, ...
Solve for T(t):
T'(t)/T(t) = k λ, which gives T(t) = c exp(k λ t).
Using the initial condition u(x,0) = x, we get:
u(x,0) = Σ cn sin(nπx/L) = x.
Then, using standard methods, we obtain the final solution:
u(x,t) = Σ cn sin(nπx/L) exp(-k n^2 π^2 t/L^2),
where cn can be determined from the initial condition u(x,0) = x.
For this problem, since the initial condition is u(x,0) = x, we have:
cn = 2/L ∫0^L x sin(nπx/L) dx = (-1)^n (2L)/(nπ)^2.
Know more about heat equation here;
https://brainly.com/question/28205183
#SPJ11
Find the probability that you roll the same number twice in a row when you toss two fair 6-sided dice.
Answers
Answer: 6/36
Step-by-step explanation:
When tossing two number cubes, you can get such sample space
(1,1) (1,2) (1,3) (1,4) (1,5) (1,6)
(2,1) (2,2) (2,3) (2,4) (2,5) (2,6)
(3,1) (3,2) (3,3) (3,4) (3,5) (3,6)
(4,1) (4,2) (4,3) (4,4) (4,5) (4,6)
(5,1) (5,2) (5,3) (5,4) (5,5) (5,6)
(6,1) (6,2) (6,3) (6,4) (6,5) (6,6)
There are 36 possible outcomes. Only (1,1), (2,2), (3,3), (4,4), (5,5), and (6,6) use the same number twice.
Hence, the probability that you roll two numbers that are the same in a row is 6/36, or in simplified form, 1/6.
Hope that helps! :)
Please Help! Will give Brainliest!
Assume the statement is true for n = k. Prove that it must be true for n = k + 1, therefore proving it true for all natural numbers, n.
Hint: Since the total number of dots increases by n each time, prove that d(k) + (k + 1) = d (k + 1).

Answers
The proof of mathematical induction is; d( k+1) is only true when d(k) is true , also d(1) should also be true.
How to Solve Mathematical Induction?
Mathematical induction is a method that is used to prove that a statement P(n) holds true for every natural number n.
For each and every natural number n, mathematical induction is a method of demonstrating a statement, theorem, or formula that is presumed to be true.
It is given that the statement is true for n = k. Thus we can say that from the principle of Mathematical Induction;
Let d(n) be a statement involving Natural Number n such that;
d(1) is true
d(m) is true
d(m + 1) is true
So, the statement d(n) is true for all the n natural numbers.
According to the second principle of Mathematical Induction, let d(n) be a statement involving Natural number n such that;
d(1) is true
d(m) is true when d(n) is true for all n where 1 ≤ n ≤m .
Then the statement d(n) is true for all n (natural numbers)
Read more about Mathematical Induction at; https://brainly.com/question/24672369
#SPJ1
An arts academy requires there to be 6 teachers for every 108 students and 5 tutors for every 45 students. How many students does the academy have per teacher? Per tutor? How many tutors does the academy need if it has 63 students?
Answers
Answer:
a) 18 students per teacher
b) 9 students per tutor
c) 7 tutors
Step-by-step explanation:
An arts academy requires there to be 6 teachers for every 108 students and 5 tutors for every 45 students.
a) How many students does the academy have per teacher?
6 teachers = 108 students
1 teacher = x
Cross Multiply.
6x = 108
x = 108/6
x = 18 students per teacher
b) Per tutor?
5 tutor = 45 students
1 tutor = x
Cross Multiply
5x = 45
x = 45/5
x = 9 students
= 9 students per tutor
c) How many tutors does the academy need if it has 63 students?
45 students = 5 tutors
63 students = x
Cross Multiply
45x = 63 × 5
x = 63 × 5/45
x = 7 tutors
Find the measure of x. 8 62° X x = [?]
![Find the measure of x. 8 62 X x = [?]](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/CSB37U3alY2uHcR7n1WyvMvBHnrfo0gm.jpeg)
Answers
Answer:
x ≈ 17
Step-by-step explanation:
using the cosine ratio in the right triangle
cos62° = \(\frac{adjacent}{hypotenuse}\) = \(\frac{8}{x}\) ( multiply both sides by x )
x × cos62° = 8 ( divide both sides by cos62° )
x = \(\frac{8}{cos62}\) ≈ 17 ( to the nearest whole number )
adding rational numbers:
after swimming 60 feet below the surface of the water, a whale swims up 30 feet. use a number line to help you create an equation that shows the new location of the whale in relation to the water's surface.
ANSWER:
A. The equation is -60 + 30 = 30. The whale is 30 feet below the waters surface.
B. The equation is -60 + (-30) = -90. The whale is 90 feet below the waters surface.
C. The equation is -60 + 30 = -90. The whale is 90 feet below the waters surface
D. The equation is -60 + (-30) = -30. The whale is 30 feet below the waters surface.
Answers
Answer:
First, in standard notation, we must define our zero.
In this case, would make sense to define the zero in the surface of the water.
(below is a number line so you can see the process to think this)
Then if the whale is swimming 60ft bellow the surface of the water, then the first position of the whale is:
-60
Now the whale goes up 30ft, then the new position of the whale is:
-60ft + 30ft = -30ft.
The correct option is A:
The equation is -60 + 30 = -30. The whale is 30 feet below the water's surface.
(you wrote that the equation was equal to +30 instead of -30 in the option A, i supposed that it was a typo)

Answer:
I belive the answer is A.
Step-by-step explanation: