Mr. Johnston supports a young tree by using a stake and a string forming a right angle with the ground.
60 in.
33 in.
What is the length of the string? Round to the nearest tenth.
Answers
Answer:68.48
Step-by-step explanation:a²+b²=c² a=60 b=33 so 60²+33²=4689 and take the square root of that which equals 68.48
Related Questions
Find the volume of this solid
A. 27,600
B. 20,400
C. 21,000
D. 1,700

Answers
i did my math i hope it helps good luck
How much money is shown? i know the $25 i just dk the change

Answers
Answer:
$25.20
Step-by-step explanation:
This is the answer because:
1) 10 + 10 + 5 = 25
2) Next, dimes are equal to 0.10
25 + 0.10 + 0.10 = 25.20
Hope this helps! :D
Please help!!!!! Find the perimeter of the figure. Round to the nearest hundredth if needed

Answers
The perimeter of the figure is 48 meters
How to determine the perimeter of the figureFrom the question, we have the following parameters that can be used in our computation:
Shape = rectangle
length = 10 meters
width = 14 meters
The perimeter of the figure is then calculated as
Perimeter = 2 * (length + width)
Substitute the known values in the above equation, so, we have the following representation
Perimeter = 2 * (10 + 14)
Evaluate
Perimeter = 48
Hence, the perimeter is 48 meters
Read more about perimeter at
https://brainly.com/question/19819849
#SPJ1
im cant figure out how to do this one ((-3)^2)^-3
Answers
Answer:
\(\dfrac{1}{729}\)
Step-by-step explanation:
\(\left(\dfrac{}{}(-3)^2\dfrac{}{}\right)^{-3}\)
First, we should evaluate inside the large parentheses:
\((-3)^2 = (-3)\cdot (-3) = 9\)
We know that a number to a positive exponent is equal to the base number multiplied by itself as many times as the exponent. For example,
\(4^3 = 4 \, \cdot\, 4\, \cdot \,4\)
↑1 ↑2 ↑3 times because the exponent is 3
Next, we can put the value 9 into where \((-3)^2\) was originally:
\((9)^{-3}\)
We know that a number to a negative power is equal to 1 divided by that number to the absolute value of that negative power. For example,
\(3^{-2} = \dfrac{1}{3^2} = \dfrac{1}{3\cdot 3} = \dfrac{1}{9}\)
Finally, we can apply this principle to the \(9^{-3}\):
\(9^{-3} = \dfrac{1}{9^3} = \boxed{\dfrac{1}{729}}\)
The "to prove" part of the information is the _____ of the "if-then" statement.
if
then
none of the above
Answers
Answer:
The correct answer option is 'then'.
Step-by-step explanation:
Does anyone know how to solve this?

Answers
Answer:
a) the average of change for the number of bacteria from 0 to 6.8 hours is
\(the \: average \: of \: change \: = \frac{2292 - 1000}{6.8 - 0} = 190 \: bacteria \: per \: hour\)
b)
\(the \: average \: rate\: of \: change \: from \: 10.2 \: to \: 13.6 \: hours \: = \frac{5080 - 3856}{13.6 - 10.2} = 360\)
When a chocolate bar is cut in half its density is: a) doubled b) unchanged c) halved
Answers
The correct answer is b) unchanged. Cutting a chocolate bar in half does not affect its density.
When a chocolate bar is cut in half, its density remains unchanged. Density is a physical property of a substance that is determined by its mass per unit volume. When the chocolate bar is cut in half, the mass is also divided into two equal parts, resulting in a reduction of the volume by half as well. Since both the numerator and denominator in the density formula change proportionally, the density remains the same.
For example, if the density of a chocolate bar is 1 gram per cubic centimeter, cutting it in half would result in two smaller bars, each with half the volume and half the mass. The density of each of the new bars would still be 1 gram per cubic centimeter, even though they are smaller in size.
Therefore, the correct answer is b) unchanged. Cutting a chocolate bar in half does not affect its density.
Learn more about density here:
https://brainly.com/question/6107689
#SPJ11
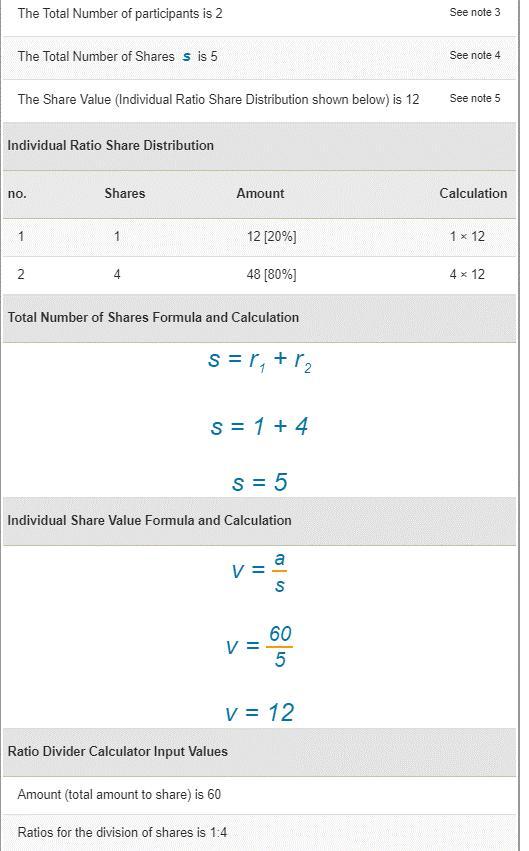
Share £60 in the ratio 1:4
Answers
Answer:
s=5
v=2
Step-by-step explanation:
I'm a little confused so I have no need for an explanation just answer honestly

Answers
Hope this helps
find the sum of fourteen and eight tenths and 276.93
Answers
Answer:
299.03
Step-by-step explanation:
5.7 gal/h = c/min? What is the answer
Answers
Answer:
5.7 gal / hour
5.7 gal / 60 min
5.7(16) c / 60 min
A rectangular prism that has a length of 6 units, width of 4 units, and height of 5 units.
Look at the three-dimensional figure.
The volume of the prism is the amount of space
the figure.
120 cubes fit inside the prism, so the figure has a volume of
square units.
Answers
Answer:
inside
120
Step-by-step explanation:
E D G E N U I T Y
Stay safe and have a wonderful day/afternoon/night!!!!!
Brainliest?!?!
Answer:
1.) Inside
2.) 120
Step-by-step explanation:
Give Him/Her Brainliest! ↑ ( ˙ ˘ ˙)
ʕ •ᴥ•ʔ εїз

Sam is thinking of retiring from his job after many years of faithful service. during the last 35 years at his job, his annual salary averages to $37,991. every month, his bills total $1,755. how much money would sam need each month to supplement his monthly social security benefit (assuming his annual benefit is 42% of his salary average) and still be able to pay his bills? a. at least $81.23 b. at least $425.32 c. at least $1,410.92 d. at least $3,019.67
Answers
Using proportions, the amount of money that Sam would need each month to pay his bills is of:
b. at least $425.32.
What is a proportion?A proportion is a fraction of a total amount, and the measures are related using a rule of three.
In this problem, his annual social security benefit is of 42% of $37,991, hence:
A = 0.42 x 37991 = $15,956.22.
Hence the monthly amount is given by:
M = 15956.22/12 = $1,329.68
He needs $1,755 monthly, hence the amount he needs is of at least:
An = 1755 - 1329.68 = $425.32.
Which means that option b is correct.
More can be learned about proportions at https://brainly.com/question/24372153
#SPJ4
Janice wants to paint this figure on her wall in her bedroom. If paint cost $3.89 per square unit, how much will it cost to paint this figure on her wall?
Answers
Answer:we need to know the size of the wall
Step-by-step explanation:
find the number of matchings of five men with five women given the constraints in the figure above on the right, where the rows represent the men and the columns represent the women.
Answers
Based on the figure provided, it is clear that each man has a preference for a certain woman. Specifically, man 1 prefers woman 3, man 2 prefers woman 5, man 3 prefers woman 2, man 4 prefers woman 4, and man 5 prefers woman 1.
Using this information, we can determine the number of matchings by considering each man and his preferred woman.
Starting with man 1, he must be matched with woman 3. There is only one possible match for him.
Moving on to man 2, he must be matched with woman 5. Again, there is only one possible match for him.
For man 3, his preferred woman is already matched with man 1. Therefore, he must be matched with woman 4.
For man 4, his preferred woman is already matched with man 2. Therefore, he must be matched with woman 2.
Finally, for man 5, his preferred woman is already matched with man 3. Therefore, he must be matched with woman 1.
Putting all of these matches together, we have:
Man 1 -> Woman 3
Man 2 -> Woman 5
Man 3 -> Woman 4
Man 4 -> Woman 2
Man 5 -> Woman 1
Therefore, there is only one possible matching of the five men with the five women given the constraints in the figure above.
Know more about rows and columns, here:
https://brainly.com/app/ask?q=32095167
#SPJ11
You poured 3/4 cup of water in a measuring cup recipe indicates that you only need 2/3 cup how much water should you pour out
Answers
Answer:
you need to pour out 1/12 of the water.
Step-by-step explanation:
\(\frac{3}{4} -\frac{2}{3}\)
you need to find like denominators
\(\frac{9}{12} -\frac{8}{12 }\)
Now subtract
\(\frac{1}{12}\)
68
Ч1
find c in the upside down triangle

Answers
Answer:
71°
Step-by-step explanation:
What is an angle?An angle is a measure of a turn, measured in degrees or °. Angles are made up of two rays, forming the sides of the angle.
If we know that all 3 of a triangle's angles must add up to 180°, we can use this expression to solve for the missing angle:
angle 1 + angle 2 + angle 3 = 180°Inserting our 2 angles into the expression gives:
68° + 41° + angle 3 = 180°To solve for angle 3, we can rearrange the equation above:
180 - (68 + 41) = angle 3Solving this equation gives us:
71° = angle 3Therefore, the measure of the missing angle is 71°
Answer:
71°
Step-by-step explanation:
What is an angle?
An angle is a measure of a turn, measured in degrees or °. Angles are made up of two rays, forming the sides of the angle.
If we know that all 3 of a triangle's angles must add up to 180°, we can use this expression to solve for the missing angle:
angle 1 + angle 2 + angle 3 = 180°
Inserting our 2 angles into the expression gives:
68° + 41° + angle 3 = 180°
To solve for angle 3, we can rearrange the equation above:
180 - (68 + 41) = angle 3
Solving this equation gives us:
71° = angle 3
Therefore, the measure of the missing angle is 71°
What is the change in elevation from -87.2 Feet to 33.6 Feet?
Answers
Answer:
120.8 feet
Step-by-step explanation:
a marketing research consultant hired by coca-cola is interested in determining the proportion of customers who favor coke over other soft drinks. a random sample of 400 consumers was selected from the market under investigation and showed that 53% favored coca-cola over other brands. compute a 95% confidence interval for the true proportion of people who favor coke. place your lower limit, rounded to 3 decimal places, in the first blank . for example, 0.345 would be a legitimate entry.
Answers
The lower limit is 0.481 and upper limit is 0.579 .
Given
a=0.05,
Z(0.025)=1.96 (from standard normal table)
So
lower limit is
p= -Z* √(p*(1-p)/n)
=0.53-1.96* √(0.53*(1-0.53)/400)
=0.481
So upper limit is
p = +Z*√(p*(1-p)/n)
=0.53+1.96*√(0.53*(1-0.53)/400)
=0.579
Know more about confidence interval,
https://brainly.com/question/32546207
#SPJ4
Simplify.If x=2, then what is 3x^2 - 2x + 1?

Answers
Step-by-step explanation:
3x^2-2x+1
3(2)^2-2(2)+1
3(4)-4+1
12-4+1
8+1=9 Answer
Step-by-step explanation:
3(2)^2 - 2x + 1
=3x4 - 2x + 1
=12 - 2x + 1
= 13 - 2x
Answer: 13 - 2x
I NEED HELP ON THIS ASAP!

Answers
The exponential function that models the situation in this problem is defined as follows:
y = 3(4)^x.
How to define an exponential function?The standard definition of an exponential function is given as follows:
y = ab^x.
In which:
a is the initial value.b is the rate of change.Three friends start the project, hence the initial value represented by the parameter a is given as follows:
a = 3.
Each day, the number of people in the project is multiplied by four, hence the parameter b is given as follows:
b = 4.
Hence the function is:
y = 3(4)^x.
More can be learned about exponential functions at https://brainly.com/question/2456547
#SPJ1
Find formulas for A (x) on [0, 2) and (2,4]. (Express numbers in exact form. Use symbolic notation and fractions where needed.) on [0, 2], A(x) = on (2,4], A(x) =
Answers
On [0, 2], A(x) can be represented by the linear equation A(x) = (1/2)x.
This means that for every x in the interval [0, 2], the value of A(x) is equal to one-half times x. For example, when x = 1, A(x) = (1/2)x = (1/2) * 1 = 1/2.
On [0, 2], A(x) can be represented by the linear equation A(x) = (1/2)x.
On (2,4], A(x) can be represented by the linear equation A(x) = ((-1/2)x + 2). This means that for every x in the interval (2,4], the value of A(x) is equal to one-half times x minus 2. For example, when x = 3, A(x) = ((-1/2)x + 2) = (-1/2) * 3 + 2 = 2 - 1.5 = 0.5.
Learn more about linear equation here:
https://brainly.com/question/29739212
#SPJ4
I need help!! 13 points

Answers
Answer: 8/15
Step-by-step explanation:
Vertical: there are 4 shaded squares out of 5 total squares → 4/5
Horizontal: there are 2 shaded squares out of 3 total squares → 2/3
\(\dfrac{4}{5}\times \dfrac{2}{3}\quad =\large\boxed{\dfrac{8}{15}}\)
Determine if the two triangles are congruent. If they are, state how you know.
Can someone help me?

Answers
Answer:
C
Step-by-step explanation:
the hypotenuse and the leg of the first right angle are congruent to the other triangle's.
ill give brainest i need help please

Answers
Answer:
\(5(x - 2) = \frac{15x + 6}{3} - 12 \\ 5(x - 2) = \frac{15x + 6 - 36}{3} \\ 5(x - 2) = \frac{15x - 30}{3} \\ 5(x - 2) = \frac{15(x - 2)}{3} \\ 15(x - 2) = 15(x - 2)\)
No solution, as value of x doesn't exist.Answer:
All real numbers
Step-by-step explanation:
Given
5(x - 2) = \(\frac{15x+6}{3}\) - 12
Multiply through by 3 to clear the fraction
15(x - 2) = 15x + 6 - 36 ← distribute left side and simplify
15x - 30 = 15x - 30
Since both sides are equal then any real value of x will be a solution.
Thus the solution is All real numbers
37 points! The square root of 83 can be plotted on the number line below.

Answers
Answer:
9.1 is the nearest tenth
9.11 to the nearest hundredth
just slightly to the right of 9.1
Step-by-step explanation:
sqrt(83)
sqrt(81) = 9
So we know that it is slightly greater than 9
9.1*9.1 =81.81
9.2*9.2=84.64
9.1 is closer
9.11 * 9.11 =82.9921
9.12 * 9.12 =83.1744
9.11 is closer
Answer:
9.1 is the nearest tenth
9.11 to the nearest hundred
to the right of 9.1
Step-by-step explanation:yi did it on i ready6
find the value of "y" log(4y+1)=log(y-7)-2log2
Answers
Answer:
y = -8/15
Step-by-step explanation:
log (4y + 1) = log (y - 7) - 2log2
log (4y + 1) = log (y - 7) - log2² (power law)
log (4y + 1) = log [(y - 7)/4]
4y + 1 = (y - 7)/4 (remove log)
4(4y + 1) = y - 7
16y - y = -8
15y = -8
y = -8/15
according to a recent study from the centers for disease control on american adults, the proportion that have a mobile phone is 89%, the proportion that have a landline is 57%, and 2% have neither a landline nor a mobile phone. what proportion of american adults have a mobile phone, and not a landline?
Answers
Approximately 34% of American adults have a mobile phone but not a landline, based on the given proportions from the study conducted by the Centers for Disease Control.
The proportion of American adults who have a mobile phone but not a landline, we need to subtract the proportion of those who have both a mobile phone and a landline from the proportion of those who have a mobile phone.
Let's denote the proportion of American adults who have a mobile phone as P(M), the proportion who have a landline as P(L), and the proportion who have neither as P(N).
Given information:
P(M) = 89% (proportion with a mobile phone)
P(L) = 57% (proportion with a landline)
P(N) = 2% (proportion with neither)
We can now calculate the proportion of adults who have a mobile phone but not a landline using the following equation:
P(M and not L) = P(M) - P(M and L)
To find P(M and L), we can subtract P(N) from P(L) since those who have neither are not included in the group with a landline:
P(M and L) = P(L) - P(N)
P(M and L) = 57% - 2%
P(M and L) = 55%
Now we can substitute the values back into the equation to find P(M and not L):
P(M and not L) = P(M) - P(M and L)
P(M and not L) = 89% - 55%
P(M and not L) = 34%
For similar questions on American adults
https://brainly.com/question/16346008
#SPJ8
or
Find g(x), where g(x) is the translation 5 units up of f(x)=x2.
Write your answer in the form a(x–h)2+k, where a, h, and k are integers.
Answers
Integers g(x) = \((x - 0)^2\) + 5 the desired form with a = 1, h = 0, and k = 5.
The function f(x) = \(x^2\), a translation 5 units up gives us the function:
g(x) = f(x) + 5
g(x) = \(x^2 + 5\)
The form \(a(x - h)^2\) + k, we need to complete the square.
We can do this by adding and subtracting \((h^2)\) inside the parentheses:
g(x) = \(x^2 + 5 + h^2 - h^2\)
The terms that involve x and those that do not:
g(x) = \((x^2 - h^2) + 5 - h^2\)
The expression \((x^2 - h^2)\) using the difference of squares formula:
g(x) = \((x - h)(x + h) + 5 - h^2\)
The coefficient of \((x - h)^2\) to be 1, so we can divide both sides by (x + h) and then multiply by a constant factor a:
g(x) = \(a(x - h)^2 + 5 - h^2\)
g(x) = \(a(x^2 - 2hx + h^2) + 5 - h^2\)
g(x) = \(ax^2 - 2ahx + ah^2 + 5 - h^2\)
To get the correct coefficients, we need to choose a and h such that:
2ah = 0 (so that the x term disappears)
\(ah^2 + 5 - h^2\) = 5 (so that the constant term is 5)
The first condition is satisfied if we choose h = 0.
The second condition is satisfied if we choose a = 1.
Then we have:
g(x) = \(1(x - 0)^2 + 5 - 0^2\)
g(x) = \((x - 0)^2\) + 5
For similar questions on Integers
https://brainly.com/question/14967701
#SPJ11
solve sinx = 2x-3 using false position method
Answers
The root of the equation sinx = 2x-3 is 0.8401 (approx).
Given equation is sinx = 2x-3
We need to solve this equation using false position method.
False position method is also known as the regula falsi method.
It is an iterative method used to solve nonlinear equations.
The method is based on the intermediate value theorem.
False position method is a modified version of the bisection method.
The following steps are followed to solve the given equation using the false position method:
1. We will take the end points of the interval a and b in such a way that f(a) and f(b) have opposite signs.
Here, f(x) = sinx - 2x + 3.
2. Calculate the value of c using the following formula: c = [(a*f(b)) - (b*f(a))] / (f(b) - f(a))
3. Evaluate the function at point c and find the sign of f(c).
4. If f(c) is positive, then the root lies between a and c. So, we replace b with c. If f(c) is negative, then the root lies between c and b. So, we replace a with c.
5. Repeat the steps 2 to 4 until we obtain the required accuracy.
Let's solve the given equation using the false position method.
We will take a = 0 and b = 1 because f(0) = 3 and f(1) = -0.1585 have opposite signs.
So, the root lies between 0 and 1.
The calculation is shown in the attached image below.
Therefore, the root of the equation sinx = 2x-3 is 0.8401 (approx).
Learn more about equation
brainly.com/question/29657983
#SPJ11