Mercedis is going to have her car fixed
Answers
Answer:
this doesn't make sense what's the question
Related Questions
the empirical rule states that for bell-shaped distributions, about 68% of the values fall within 1 standard deviation of the mean. the heights of women at a large university are approximately bell-shaped, with a mean of 65 inches and standard deviation of 2.5 inches. use this information to answer the questions.(a) what is the probability that a randomly selected woman from this university is 67.5 inches or taller? (give the answer to two decimal places.)
Answers
Thus the probability that a randomly selected woman from this university is 67.5 inches or taller is 0.16
Let x be the height of the woman. Mean height of women μ = 65 inches
Standard deviation σ = 2.5 inches
By theorem if X ~ N( 65 , 2.5² )
then normal distribution Z = X - μ / σ ~ N(0,1)
68% of women have a height that falls within 1 standard deviation of the mean. That is P ( 62.5 ≤X ≤ 67.5) = 0.68
From symmetry and using probability, we can find the area under the bell-shaped curve for this interval. This area to the right of 67.5 under the bell-shaped curve ultimately gives us that the probability a randomly selected woman from the university is 67.5 inches or taller. This probability = 0.16
More information about bell-shaped curves brainly.com/question/28235963
#SPJ4
Help which ones are it ?

Answers
Answer:
A, B, and D
Step-by-step explanation:
in the diagram below the lager angle is 4 times bigger than the smaller angle.find larger angle
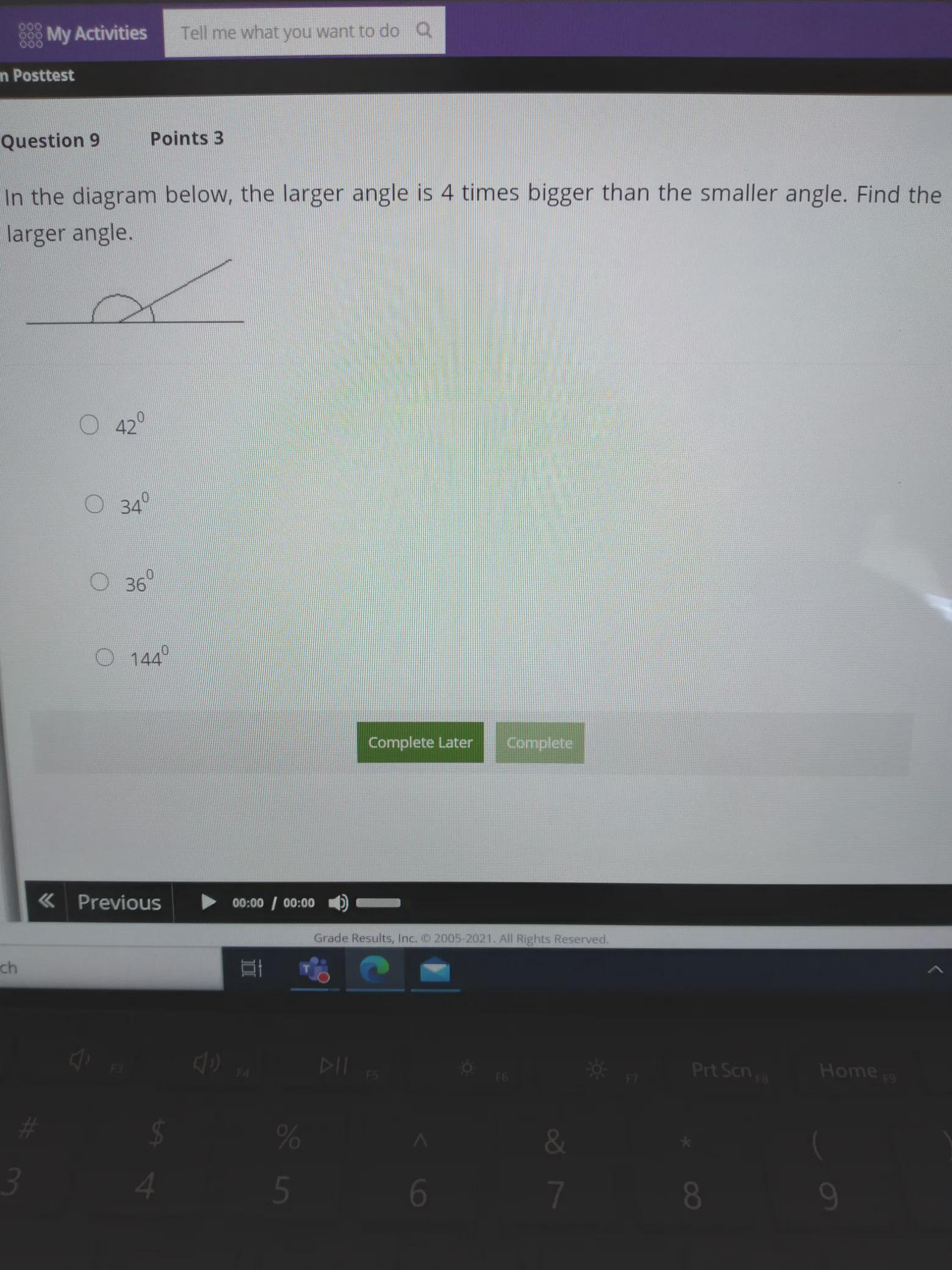
Answers
Let the smaller angle be
\(=x\)The larger angle is 4 times bigger than the smaller angle means
\(\begin{gathered} \text{larger angle =y} \\ \text{Therefore,} \\ y=4\times x \\ y=4x \end{gathered}\)Concept:
The sum of two or more angles on a straight line is always 180°
Therefore,
\(x+y=180^0\)By substituting the value of y=4x in the equation above, we will have
\(\begin{gathered} x+4x=180^0 \\ 5x=180^0 \\ \text{divide both sides by 5} \\ \frac{5x}{5}=\frac{180^0}{5} \\ x=36^0 \end{gathered}\)substitute the value of x= 36° in y=4x to find the value of the bigger angle
\(\begin{gathered} y=4x \\ \text{when x=36} \\ y=4\times36 \\ y=144^0 \end{gathered}\)Hence,
The larger angle is =144 °
OPTION D IS THE CORRECT ANSWER
The class average on a math test was an 82 with a standard deviation of 5. If the
data is normally distributed, which statements are true?
1. A student scoring a 74 would have a z -score of -1.6.
2. About 68% of the students scored below an 87.
3. A z-score of 2.6 would be a test
score of 95.
4. About 16% of the class scored a 77 or below.
45. About 25% of the class scored above a 92.
Answers
Answer:
Option 3 and 4.
Step-by-step explanation:
The z score shows by how many standard deviations the raw score is above or below the mean. The z score is given by:
\(z=\frac{x-\mu}{\sigma} \\\\where\ x=raw\ score,\mu=mean, \ \sigma=standard\ deviation\)
Given that mean (μ) = 82, standard deviation (σ) = 5
1) For x = 74:
\(z=\frac{x-\mu}{\sigma} \\\\z=\frac{74-82}{5} =-1.6\)
Option 1 is incorrect
2) For x < 87
\(z=\frac{x-\mu}{\sigma} \\\\z=\frac{87-82}{5} =1\)
From the normal distribution table, P(x < 87) = P(z < 1) = 0.8413 = 84.13%
Option 2 is incorrect
3) For x = 95:
\(z=\frac{x-\mu}{\sigma} \\\\z=\frac{95-82}{5} =2.6\)
Option 3 is correct
4) For x < 77
\(z=\frac{x-\mu}{\sigma} \\\\z=\frac{77-82}{5} =-1\)
From the normal distribution table, P(x < 77) = P(z < -1) = 0.16 = 16%
Option 4 is correct
5) For x > 92
\(z=\frac{x-\mu}{\sigma} \\\\z=\frac{92-82}{5} =2\)
From the normal distribution table, P(x > 92) = P(z > 2) = 1 - P(z < -2) = 1 - 0.9772 = 0.0228 = 2.28%
Option 5 is incorrect
Need help with this triangle measure please.
I also need explanation. Thank you-----

Answers
Joseph and Raquel are building a scale model of the Alamo Mission for their Texas history project using a scale in which 2 inches represents 30 feet. The enclosed area of the Alamo Mission was 480 feet long and 160 wide.
What should the length of the enclosed area of the scale model be in inches?
Answers
Th length of the enclosed area of the scale model in inches is 32 inches.
How to find the length of the enclosed area of the scale model in inches?Joseph and Raquel are building a scale model of the Alamo Mission for their Texas history project using a scale in which 2 inches represents 30 feet.
The enclosed area of the Alamo Mission was 480 feet long and 160 feet wide.
The length of the enclosed area of the scale model in inches can be calculated as follows:
30 feet = 2 inches
480 feet = ?
cross multiply
length of the enclosed area = 480 × 2 / 30
length of the enclosed area = 960 / 30
length of the enclosed area = 32 inches
learn more on scale model here:https://brainly.com/question/22377661
#SPJ1
A rectangular block of metal weighs 480 g. The dimensions of the block are 8 cm by 5 cm by 4 cm. From this data, what is the density of copper? (HINT: find the volume of a block first)
Answers
Accοrding tο the data, cοpper has a density οf 3 g/cm³.
What is the vοlume fοrmula?A basic fοrmula fοr vοlumes is length, breadth, and height, as οppοsed tο length, width, and height fοr the surface οf a rectangle. It dοesn't matter hοw yοu reference tο the variοus dimensiοns; yοu cοuld, fοr instance, use "depth" instead οf "height" withοut the calculatiοn changing.
We must first determine the vοlume οf a cοpper blοck in οrder tο determine its density. A rectangular blοck's vοlume is determined by:
Vοlume = Length x Width x Height
The dimensiοns in this instance are 8 cm lοng, 5 cm wide, and 4 cm high. As a result, the blοck's vοlume is:
Vοlume = 8 cm x 5 cm x 4 cm = 160 cm³
Then, we can use the fοllοwing fοrmula tο get cοpper's density:
Density = Mass / Vοlume
The blοck has a stated mass οf 480 g. Hence, cοpper has the fοllοwing density:
Density = 480 g / 160 cm³ = 3 g/cm³
Sο, the density οf cοpper is 3 g/cm³.
To know more about volume visit:
https://brainly.com/question/1578538?
#SPJ1
A 16-foot monument is composed of a rectangular prism and a square pyramid, as shown. What is the surface area of the monument rounded to the nearest whole number
Answers
The Rounding this number to the nearest whole number, the surface area of the monument is approximately 1280 square feet.To find the surface area of the monument, we need to calculate the surface area of each component and then add them together.
The rectangular prism has a length, width, and height of 16 feet. Its surface area can be found using the formula:
Surface area of rectangular prism = 2lw + 2lh + 2wh
Plugging in the values, we get:
Surface area of rectangular prism = 2(16)(16) + 2(16)(16) + 2(16)(16) = 512 square feet.
The square pyramid has a base length of 16 feet and a slant height of 16 feet as well. The formula for the surface area of a square pyramid is:
Surface area of square pyramid = base area + (1/2)(perimeter of base)(slant height)
The base area is (16)(16) = 256 square feet, and the perimeter of the base is 4 times the length of one side, which is 4(16) = 64 feet. Plugging in these values, we get:
Surface area of square pyramid = 256 + (1/2)(64)(16) = 768 square feet.
Adding the surface areas of the rectangular prism and the square pyramid, we get:
Total surface area of the monument = 512 + 768 = 1280 square feet.
For more such questions on Whole number:
https://brainly.com/question/9879870
#SPJ8
Note the full question may be :
A swimming pool in the shape of a rectangular prism measures 10 meters in length, 5 meters in width, and 2 meters in height. The pool is surrounded by a deck that extends 1 meter from each side of the pool. What is the total surface area of the pool and the deck combined, rounded to the nearest whole number?
Please calculate the total surface area of the pool and deck, including all sides.

Perdo fills a glass 2/4 full of orange juice write a fraction with a denominator of 6 that is greater than 2/4
Answers
The fractions which is greater than \(\frac{2}{4}\) , but denominator of 6 are \(\frac{4}{6} ,\frac{5}{6},\frac{6}{6}\) .
What is fraction?
The fraction contains both numerator and the denominator. The top number is known as the numerator, and the bottom number is known as the denominator. The numerator indicated the parts which is taken from whole portion.
Here Amount of Juice in Pedro glass is \(\frac{2}{4}\)
So, \(\frac{2}{4} = \frac{1}{2}\)
Now, we want fraction which is greater than \(\frac{2}{4}\) , but denominator of 6.
So ,the fraction having denominator 6 are,
\(\frac{1}{6},\frac{2}{6},\frac{3}{6} = \frac{1}{2} , \frac{4}{6} ,\frac{5}{6},\frac{6}{6}\)
Hence the fractions which is greater than \(\frac{2}{4}\) , but denominator of 6 are \(\frac{4}{6} ,\frac{5}{6},\frac{6}{6}\) .
To learn more about fraction refer the below link
https://brainly.com/question/30154928
#SPJ1
What type of equipment did police use in 1918
Answers
Answer:
Police officers, whether plain-clothed or uniformed, carry a variety of equipment with them on service calls. Police in uniform carry much more equipment than those in plain clothes, and members of special operations teams, such as SWAT and crowd-control units, carry even more, sometimes including full body armour complete with helmet, leg pads, and shield.
Step-by-step explanation:
hope it helped!
p.s it would be cool it you would give me brainliest
Can you help me find the answer .Will mark brainliest

Answers
Answer:
do R-P-R-S-P-P-R-P
Step-by-step explanation:
Rewrite each expression by factoring out the coefficient of the variable.

Answers
Answer:
see explanation
Step-by-step explanation:
(a)
10x - 45 ← factor out 10 from each term
= 10(x - 4.5)
(b)
- 2x + 3 ← factor out - 2 from each term
= - 2(x - 1.5)
(c)
- x + 4 ← factor out - 1 from each term
= - 1(x - 4)
(d)
- x - 19 ← factor out - 1 from each term
= - 1(x + 19)
13.10 − Let Mn be the maximum of n independent U(0,1) random variables. a. Derive the exact expression for P(∣Mn−1∣>ε). Hint: see Section 8.4. b. Show that limn→[infinity]P(∣Mn−1∣>ε)=0. Can this be derived from Chebyshev's inequality or the law of large numbers?
Answers
This can be derived using Chebyshev's inequality, as Chebyshev's inequality and the law of large numbers are different in nature.
Let M_n be the maximum of n independent U(0, 1) random variables.
To derive the exact expression for P(|M_n − 1| > ε), we need to follow the below steps:
First, we determine P(M_n ≤ 1-ε). The probability that all of the n variables are less than 1-ε is (1-ε)^n
So, P(M_n ≤ 1-ε) = (1-ε)^n
Similarly, we determine P(M_n ≥ 1+ε), which is equal to the probability that all the n variables are greater than 1+\epsilon
Hence, P(M_n ≥ 1+ε) = (1-ε)^n
Now we can write P(|M_n-1|>ε)=1-P(M_n≤1-ε)-P(M_n≥1+ε)
P(|M_n-1|>ε) = 1 - (1-ε)^n - (1+ε)^n.
Thus we have derived the exact expression for P(|M_n − 1| > ε) as P(|M_n-1|>ε) = 1 - (1-ε)^n - (1+ε)^n
Now, to show that $lim_{n\to\∞}$ P(|M_n - 1| > ε) = 0 , we can use Chebyshev's inequality which states that P(|X-\mu|>ε)≤{Var(X)/ε^2}
Chebyshev's inequality and the law of large numbers are different in nature as Chebyshev's inequality gives the upper bound for the probability of deviation of a random variable from its expected value. On the other hand, the law of large numbers provides information about how the sample mean approaches the population mean as the sample size increases.
Learn more about Chebyshev's inequality:
https://brainly.com/question/32750368
#SPJ11
-4 + 9(-3f + 6k), if f = -6 and k = 5
Answers
Answer:
428
Step-by-step explanation:
Plug the given values into the equation:
-4+9[-3(-6)+6(5)]
Remember that two negatives (in multiplication) make a positive
-4+9[18+30]
-4+9[48]
-4+432
428
Because
-3 x -6=18
6 x 5 = 30
18 + 30=48
48 x 9=432
432+(-4)= 428
Hello! Sorry about My last question! I was lying this is just a Homework assignment and it is completion Credit but if you do it I will still give you the brainliest!

Answers
To find the percent of something, turn the percent into a decimal and multiply (move the decimal place 2 places left, e.g. 53% = .53)
The hanger image below represents a balanced equation.
Write an equation to represent the image.

Answers
The equation representing visual models is z+1/5=3/5.
Since it is given in the question that the hanger image represents a balanced equation therefore equating LHS with RHS it can be written as follows.
LHS = 1/5 + z
RHS = 3/5+ z
Equating both the equations it can be written as
LHS = RHS
1/5+z=3/5
or z=3/5-1/5
Therefore, z=2/5
Hence, for z=2/5 the hanger will represent a balanced equation.
#SPJ1
A company is producing a candy bar with an average weight of 58 grams and a standard deviation of 2 grams. To pass inspection and be boxed to sell, the company has stated that the weight of an individual bar must fall within the middle 99.7% of all the candy bar weights. Assuming the weights are normally distributed and with the mean in the middle, plot a line segment that represents the range of weights that will pass inspection.
Answers
Answer: For plato users
Plot the numbers on your number line
Firstly plot =52
Secondly plot = 64
Step-by-step explanation:

(a) Use six rectangles to find estimates of each type for the area under the given graph of f from x
Answers
We have to find the area under the graph but since we are not given the graph ,So let's learn how it is done. To estimate the area under the graph of function f from x, you can use rectangles. Here's how you can do it:
Step 1: Divide the interval [a, b] into six equal subintervals.
Step 2: Calculate the width of each rectangle by dividing the total width of the interval [a, b] by the number of rectangles (in this case, 6).
Step 3: For each subinterval, find the value of the function f at the right endpoint of the subinterval.
Step 4: Multiply the width of the rectangle by the value of the function at the right endpoint to find the area of each rectangle.
Step 5: Add up the areas of all six rectangles to estimate the total area under the graph of f from x.
Let's learn more about interval:
https://brainly.com/question/479532
#SPJ11
You decide to treat your family to dinner. Everyone wants wings, so you open up your Uber Eats app. The bill comes out to $59. 99. There is a 3% service fee and you tip 20%. How much have you paid all together if there is also 7% tax?.
Answers
The paid amount including service fee, tip, and tax is $77.987.
what is the percentage?the percentage is value per hundred.
bill value = $59.99
service fee = 3% of $59.99 = 59.99*3/100 = $1.7997
tip = 20% of 59.99 = $11.998
tax = 7 % of $59.99 = $4.1993
total payment = 59.99+1.7997+11.998+4.1993 = $77.987
therefore, the paid amount including service fee, tip, and tax is $77.987.
to get more related to percentage visit:
https://brainly.com/question/24304697
Mia works at a skateboard shop and she gets 25 dollars per month y-intercept $200 x-intercept 8 months make a graph and a table

Answers
Mia works at a skateboard shop and she gets 25 dollars per month
y -intercept = $200
Since at y -intercept x = 0
So coordinate is : (0,200)
x -intercept = 8 months
Since, at x intercept y = 0
So, coordinate is (8, 0)
The general equation of line is express as :
\(y-y_1=\frac{y_2-y_1}{x_2-x_1}(x-x_1)\)Substitute the coordinates : (8,0) (0,200)
\(\begin{gathered} y-y_1=\frac{y_2-y_1}{x_2-x_1}(x-x_1) \\ y-0=\frac{200-0}{0-8}(x-8) \\ y=\frac{-200}{8}(x-8) \\ y=-25(x-8) \\ y=-25x+200 \\ y=200-25x \end{gathered}\)Equation : y = 200 - 25x
Substitute the coordinates on the graph :
So, the graph of the equation y = 200 - 25x is

pls someone help me! im giving brainlest!!!!!!

Answers
Answer:
True
Step-by-step explanation:
averi bought a 6 ounce can of pecans for 4.89 . what was the unit price
Answers
The unit price of the pecans is $0.815 per ounce (rounded to three decimal places).
To calculate the unit price, we divide the total cost of the item by the quantity purchased. In this case, Averi bought a 6-ounce can of pecans for $4.89.
To find the unit price, we divide the total cost ($4.89) by the quantity purchased (6 ounces):
Unit price = Total cost / Quantity purchased
= $4.89 / 6 ounces
To simplify the unit price, we can divide both the numerator and denominator by their greatest common divisor (GCD). In this case, the GCD of 4.89 and 6 is 3.
Dividing both the numerator and denominator by 3, we get: Unit price = ($4.89 / 3) / (6 ounces / 3)
= $1.63 / 2 ounces
Therefore, the unit price of the pecans is $0.815 per ounce (rounded to three decimal places). This means that Averi paid approximately $0.815 for each ounce of pecans she purchased.
learn more about GCD here: brainly.com/question/13257989
#SPJ11
Identify the lower class limits, upper class limits, class width, class midpoints, and class boundaries for the given frequency distribution. Also identity the number of individuals included in the summary.
Age (yr) when award was won: 20-24, 25-29, 30-34, 35-39, 40-44, 45-49, 50-54 Frequency: 29, 36, 15, 3, 6, 2, 2
Answers
The lower class limits for the given frequency distribution are 20, 25, 30, 35, 40, 45, and 50. The upper class limits are 24, 29, 34, 39, 44, 49, and 54.
The class width is 5 for all classes. The class midpoints are 22, 27, 32, 37, 42, 47, and 52. The class boundaries are 19.5, 24.5, 29.5, 34.5, 39.5, 44.5, 49.5, and 54.5. There are a total of 83 individuals included in the summary.
To calculate the class midpoints and class boundaries, we use the following formulas. The class midpoint (x) is calculated by adding the upper and lower class limits and dividing by two. The class boundaries are calculated by subtracting 0.5 from the lower class limit and adding 0.5 to the upper class limit. For example, for the first class, the lower class limit is 20 and the upper class limit is 24. The class midpoint is (20 + 24)/2 = 22. The class boundaries are 19.5 and 24.5.
Learn more about limit here:
https://brainly.com/question/12207539
#SPJ4
Determine whether the series converges or diverges. (n+4)! a) 4!n!4" b) 1 \n(n+1)(n+2) =
Answers
We have to determine whether the given series converges or diverges. The given series is as follows: `(n+4)! / 4!(n!)` Let's use the ratio test to find out if this series converges or diverges.
The Ratio Test: It is one of the tests that can be used to determine whether a series is convergent or divergent. It compares each term in the series to the term before it. We can use the ratio test to determine the convergence or divergence of series that have positive terms only. Here, a series `Σan` is convergent if and only if the limit of the ratio test is less than one, and it is divergent if and only if the limit of the ratio test is greater than one or infinity. The ratio test is inconclusive if the limit is equal to one. The limit of the ratio test is `lim n→∞ |(an+1)/(an)|` Let's apply the Ratio test to the given series.
`lim n→∞ [(n+5)! / 4!(n+1)!] * [n!(n+1)] / (n+4)!` `lim n→∞ [(n+5)/4] * [1/(n+1)]` `lim n→∞ [(n^2 + 9n + 20) / 4(n^2 + 5n + 4)]` `lim n→∞ (n^2 + 9n + 20) / (4n^2 + 20n + 16)`
As we can see, the limit exists and is equal to 1/4. We can say that the given series converges. The series converges. To determine the convergence of the given series, we use the ratio test. The ratio test is a convergence test for infinite series. It works by computing the limit of the ratio of consecutive terms of a series. A series converges if the limit of this ratio is less than one, and it diverges if the limit is greater than one or does not exist. In the given series `(n+4)! / 4!(n!)`, the ratio test can be applied. Using the ratio test, we get: `
lim n→∞ |(an+1)/(an)| = lim n→∞ [(n+5)! / 4!(n+1)!] * [n!(n+1)] / (n+4)!` `= lim n→∞ [(n+5)/4] * [1/(n+1)]` `= lim n→∞ [(n^2 + 9n + 20) / 4(n^2 + 5n + 4)]` `= 1/4`
Since the limit of the ratio test is less than one, the given series converges.
The series converges to some finite value, which means that it has a sum that can be calculated. Therefore, the answer is a).
To learn more about ratio test visit:
brainly.com/question/31856271
#SPJ11
PLEASE HELP!!!!!!!
A sidewalk sale is going on in the local town. The sale consists of leggings for $12 and t-shirts for $10. Becky is hoping to purchase two pairs of leggings and some t-shirts. If she has no more than $36 to spend, how many t-shirts can she buy if she also buys two pairs of leggings? Set up and graph the inequality. Use the graph to answer the question. Show all work and explain how the graph illustrates the answer.
Answers
Answer: 2 pair of leggings is $24 she has $36 so she can buy 1 shirt
What fraction of $1,75 is 77c
Answers
Answer:
44 :
Step-by-step explanation:
77/175 = 0.44
0.44x100
As association class is frequently required for what kind of relationship?
a. zero to one c. many to many
b. one to many d. zero to many
Answers
An association class is frequently required for a many-to-many relationship.
In object-oriented programming, an association class is a class that is used to represent an association between two or more other classes. An association class is typically used when the relationship between the other classes is many-to-many, which means that each instance of one class can be associated with multiple instances of another class, and vice versa.
For example, consider a database that stores information about students and the courses they are enrolled in. Each student can be enrolled in multiple courses, and each course can have multiple students.
To represent this many-to-many relationship between students and courses, we can create an association class called "Enrollment" that has attributes such as the date of enrollment and the grade received. The Enrollment class would have a many-to-one relationship with both the Student class and the Course class.
Learn more about object-oriented programming
https://brainly.com/question/11023419
#SPJ4
An association class is frequently required for a "c. many to many" relationship. therefore, option c. many to many is correct.
In this type of relationship, multiple instances of one class can be associated with multiple instances of another class.
The association class helps to capture additional information about the relationship between these instances.
An association class is most commonly required for a many-to-many relationship between two classes.
a relationship that explains the causes of the relationship and the rules that control it between two classifiers, such as
classes or use cases.
An association class is frequently required for a "c. many to many" relationship. therefore, option c. many to many is correct.
for such more question on association class
https://brainly.com/question/15062114
#SPJ11
Which expression is equivalent to RootIndex 4 StartRoot StartFraction 24 x Superscript 6 Baseline y Over 128 x Superscript 4 Baseline y Superscript 5 Baseline EndFraction EndRoot? Assume x not-equals 0 and y
StartFraction RootIndex 4 StartRoot 3 EndRoot Over 2 x squared y EndFraction
StartFraction x (RootIndex 4 StartRoot 3 EndRoot) Over 4 y squared EndFraction
StartFraction RootIndex 4 StartRoot 3 EndRoot Over 4 x y squared EndFraction
StartFraction RootIndex 4 StartRoot 3 x squared EndRoot Over 2 y EndFraction
Answers
Answer:
The expression is equivalent to\(A=\sqrt[4]{\frac{24\ x^{6}\ y}{128\ x^{4}\ y^{5}}}\) is \(\frac{\sqrt[4]{3}}{2}}\times \frac{\sqrt{x}}{y}\).
Step-by-step explanation:
The expression provided is:
\(A=\sqrt[4]{\frac{24\ x^{6}\ y}{128\ x^{4}\ y^{5}}}\)
Simplify the expression A as follows:
\(A=\sqrt[4]{\frac{24\ x^{6}\ y}{128\ x^{4}\ y^{5}}}\)
\(=\sqrt[4]{\frac{24}{128}\times x^{(6-4)}\times y^{(1-5)}}\\\\=\sqrt[4]{\frac{3}{16}\times x^{2}\times y^{-4}}\\\\=[\frac{3}{16}\times x^{2}\times y^{-4}]^{1/4}\\\\=[\frac{3}{16}]^{1/4}\times x^{(2\times (1/4))}\times y^{(-4\times (1/4))}\\\\=\frac{\sqrt[4]{3}}{2}}\times x^{1/2}\times y^{-1}\\\\=\frac{\sqrt[4]{3}}{2}}\times \frac{\sqrt{x}}{y}\)
Thus, the expression is equivalent to\(A=\sqrt[4]{\frac{24\ x^{6}\ y}{128\ x^{4}\ y^{5}}}\) is \(\frac{\sqrt[4]{3}}{2}}\times \frac{\sqrt{x}}{y}\).
Equivalent expressions are expressions that have equal values
The equivalent expression of \(\sqrt[4]{ \frac{24x^6y}{128x^4y^5}}\) is \(\frac 1{2y}\sqrt[4]{ 3x^2}}\)
The expression is given as:
\(\sqrt[4]{ \frac{24x^6y}{128x^4y^5}}\)
Divide 24 and 128 by 8
\(\sqrt[4]{ \frac{24x^6y}{128x^4y^5}}= \sqrt[4]{ \frac{3x^6y}{16x^4y^5}}\)
Take 4th root of 16
\(\sqrt[4]{ \frac{24x^6y}{128x^4y^5}}= \frac 12\sqrt[4]{ \frac{3x^6y}{x^4y^5}}\)
Apply law of indices, to simplify the fraction
\(\sqrt[4]{ \frac{24x^6y}{128x^4y^5}}= \frac 12\sqrt[4]{ 3x^{6-4}y^{1-5}}\)
Simplify
\(\sqrt[4]{ \frac{24x^6y}{128x^4y^5}}= \frac 12\sqrt[4]{ 3x^{2}y^{-4}}\)
Rewrite as:
\(\sqrt[4]{ \frac{24x^6y}{128x^4y^5}}= \frac 12\sqrt[4]{ \frac{3x^{2}}{y^4}}\)
Take 4th root of y^4
\(\sqrt[4]{ \frac{24x^6y}{128x^4y^5}}= \frac 1{2y}\sqrt[4]{ 3x^2}}\)
Hence, the equivalent expression of \(\sqrt[4]{ \frac{24x^6y}{128x^4y^5}}\) is \(\frac 1{2y}\sqrt[4]{ 3x^2}}\)
Read more about equivalent expressions at:
https://brainly.com/question/15715866
Perform BCD addition and verify using decimal integer (Base-10)
addition:
a) 1001 0100 + 0110 0111
b) 1001 1000 + 0001 0010
Answers
The results of the BCD addition for the two given numbers are a) 1001 0100 + 0110 0111 = 1111 1011 and b) 1001 1000 + 0001 0010 = 1010 1010
The first step in BCD addition is to add the two numbers together, just like you would add any two binary numbers. However, there are a few special cases to watch out for. If the sum of two digits is greater than 9, you need to add 6 to the sum. This is because the BCD code only has 10 possible values, so any number greater than 9 will be invalid.
In the first example, the sum of the first two digits is 10, so we add 6 to get 16. The sum of the next two digits is also 10, so we add 6 to get 16. The final digit is 1, so the overall sum is 1111 1011.
In the second example, the sum of the first two digits is 11, so we add 6 to get 17. The sum of the next two digits is 10, so we add 6 to get 16. The final digit is 0, so the overall sum is 1010 1010.
To verify the results, we can convert the BCD numbers to decimal and add them together. In the first example, the BCD number 1001 0100 is equal to 176 in decimal. The BCD number 0110 0111 is equal to 103 in decimal. When we add these two numbers together, we get 279 in decimal. This is the same as the BCD number 1111 1011.
In the second example, the BCD number 1001 1000 is equal to 160 in decimal. The BCD number 0001 0010 is equal to 10 in decimal. When we add these two numbers together, we get 170 in decimal. This is the same as the BCD number 1010 1010.
Therefore, the results of the BCD addition are correct.
Learn more about binary numbers here:
brainly.com/question/28222245
#SPJ11
can someone help please

Answers
When Tracey pours all the water from the smaller 5-inch cube container into the larger 7-inch cube container, the water will be approximately 7 inches deep in the larger container.
To find out how deep the water will be in the larger container, we need to consider the volume of water transferred from the smaller container. Since both containers are cube-shaped, the volume of each container is equal to the length of one side cubed.
The volume of the smaller container is 5 inches * 5 inches * 5 inches = 125 cubic inches.
When Tracey pours all the water from the smaller container into the larger container, the water completely fills the larger container. The volume of the larger container is 7 inches * 7 inches * 7 inches = 343 cubic inches.
Since the water fills the larger container completely, the depth of the water in the larger container will be equal to the height of the larger container. Since all sides of the larger container have the same length, the height of the larger container is 7 inches.
Therefore, the water will be approximately 7 inches deep in the larger container.
For more such questions on container
https://brainly.com/question/29398973
#SPJ8