Megan has two books that each have dimensions of 12 inches x 6 inches x 2 inches. What is the volume, in cubic inches, of Megan's two books?

6/15

you add everything she sells together. ( 3+1+6+5=15) Then you put the amount of ovens she sells above taht number, making it a fraction.

The answer of the given question based on the percent is , 20.49 trillion is about 52.84% larger than 13.4 trillion.

Percentage is a way of expressing a quantity or value as a fraction of 100. It is a widely used method for describing proportions, rates, changes, and comparisons in various fields such as mathematics, economics, statistics, science, and everyday life. The term "percent" literally means "per hundred", and it is represented by the symbol "%". To convert a number to a percentage, we multiply it by 100 and add the symbol "%".

To find the percent larger that 20.49 trillion is to 13.4 trillion, we can use the following formula:

Percent change = (New value -Old value) /Old value * 100%

Plugging in the values we have:

Percent change = (20.49 trillion - 13.4 trillion) / 13.4 trillion * 100%

= 7.09 trillion / 13.4 trillion * 100%

= 52.84%

Therefore, 20.49 trillion is about 52.84% larger than 13.4 trillion.

To know more about Fraction visit:

https://brainly.com/question/11947917

#SPJ1

Therefore , the solution of the given problem of slope comes out to be  y = 7x - 17 is the equation in slope-intercept form.

A line's steepness is determined by its slope. In gradient-based equations, a situation known as gradient overflow can happen. One can determine the slope by dividing the sum of a run (width differential) and rise (climbing distinction) between two places. The equation with the hill variation, y = mx + b, is used to model the fixed path issue. where grade is mm, a = bc, and the line's y-intercept is situated; (0, b).

Here,

Given :

=> -7x + y = -17

7x both faces together

=>  -7x + 7x + y = 7x - 17

Condense: y = 7x - 17

So,

=>  y = 7x - 17 is the equation in slope-intercept form.

To know more about slope visit:

https://brainly.com/question/3605446

#SPJ1

Answer:

\(option \: b) \: \: \: 5 \sqrt{5} cm\)

Step-by-step explanation:

Given,

Base of the triangle = 10

Hypotenuse of the triangle = 15

So, according to Pythagoras Theorem,

\( {c}^{2} - {a}^{2} = {b}^{2} \)

\( {15}^{2} - {10}^{2} = {b}^{2} \)

\(225 - 100 = {b}^{2} \)

\(125 = {b}^{2} \)

\(b = \sqrt{125} \)

\(b = \sqrt{5 \times 5 \times 5} \)

\(b = 5 \sqrt{5} cm \: (ans)\)

9514 1404 393

Answer:

  3x +1 = 16

Step-by-step explanation:

"Simplified" usually means parentheses are eliminated and like terms are combined.

  3(x -2) +7 = 16 . . . . . given

  3x -6 +7 = 16 . . . . . . parentheses eliminated using the distributive property

  3x +1 = 16 . . . . . . . . . constants are combined (simplified equation)

__

You can solve this in 2 steps:

  3x = 15 . . . . subtract 1

  x = 5 . . . . . . divide by 3

b = √c2 - a2

= √122 - 10.5953711143072

= √31.738110950106

= 5.63366

Answer:

Adult ticket = $4

Student ticket = $7

Step-by-step explanation:

• On first day:

\({ \rm{13x + 11y = 129 - - - \{eqn(a) \}}}\)

• On second day:

\({ \rm{13x + 2y = 66 - - - \{eqn(b) \}}}\)

• Solving simultaneously;

Equation (a) - Equation (b):

\( - { \underline{ \rm{ \binom{13x + 11y = 129}{13x + 2y = 66} }}} \\ { \rm{00x + 9y = 63}} \\ \\ { \rm{y = \frac{63}{9} }} \\ \\ { \boxed{ \rm{ \: y = 7 \: }}} \\ \\ { \rm{13x + 2(7) = 66}} \\ \\ { \rm{13x + 14 = 66}} \\ \\ { \rm{13x = 52}} \\ \\ { \boxed{ \rm{x = 4}}}\)

•

Answer:

Step-by-step explanation:

To get the y values all you need to do is substitute the x value in the equation y=-2/3x+7.

For example:

y=-2/3(-6)=7

-2/3x6=-4

-4+7=3

(-6,3)

You can double check your work by filling the x and y coordinates in the equation and when solved if it it true you know you were correct.

To get the x value, you need to fill in the y in the equation y=-2/3x+7

for example:

5=-2/3x+7

-2=-2/3x

3=x

(3,5)

y=-2/3x+7

y=-2/3(15)+7

y=-10+7

y=-3

(15,-3)

y=-2/3x+7

15=-2/3x+7

8=-2/3x

-12=x

(-12,15)

Answer:

Either 180 feet, or 90 feet

Step-by-step explanation:

This is because she could just throw across the field rather than throwing it base to base.

I hope you understand!

"The correct answer is option A." The missing coordinates of point Q are (a, c/2).We are given the coordinates of points P and N as (0, c) and (2a, 0), respectively. We are also given that point Q lies on the same line as points P, Q, and N. We need to find the missing coordinates of point Q.

Since point Q lies on the same line as P, Q, and N, its x-coordinate must be halfway between the x-coordinates of points P and N. That is, the x-coordinate of Q is:

x-coordinate of Q = (x-coordinate of P + x-coordinate of N) / 2

x-coordinate of Q = (0 + 2a) / 2 = a

So, we know that the x-coordinate of point Q is a.

To find the y-coordinate of point Q, we can use the fact that point Q lies on the same line as point P, which has coordinates (0, c). The equation of the line passing through P and N is:

y - c = (0 - c) / (2a - 0) * (x - 0)

Simplifying this equation gives:

y - c = -c/2a * xy = -c/2a * x + c

Substituting x = a in this equation gives:

y = -c/2a * a + cy = -c/2 + cy = c/2

So, we know that the y-coordinate of point Q is c/2.

For such more questions on missing coordinates:

https://brainly.com/question/27481419

#SPJ11

Answer:

i believe it is a

Step-by-step explanation:

i think this because the 2 choice would not work because it does not have x in it

the third one would not work because it is the same as the 2 one

the fourth one would not work because it would =1x

so it is a

Given the function:

a) the inverse function is:

So, we have two linear functions, which are one-to-one (every element of the function's codomain is the image of at most one element of its domain).

b) In order to graph both functions, keep in mind that f is a line with slope 3 and y-intercept at y = -8. As for f^{-1} it is a line with slope 1/3 and y-intercept at y = 8/3. You can simply graph both function on the same axes by calculating the values of f and f^{-1} given some values of x, for instance:

x = ..., -2 , -1, 0, 1, 2,...

f(x) =

f^{-1} =

As can be seen in the following graph: purple line represents f and pink line represents f^{-1}:

c) The domain and range of f(x) and f^{-1} is the same:

Answer:

u=4

v=2√3

Step-by-step explanation:

The 30-60-90 triangle theorem states that the shortest side of a triangle is half of the hypotenuse, so 2*2=4. So, the hypotenuse, u, is 4.

The longest leg is √3 times greater than the shortest leg, so 2*√3=2√3. So, the longest leg, v, is 2√3.

Answer:

hehaw

Step-by-step explanation:

0.19, 91 hundredths, then the equation

Answer: 99.06cm

Step-by-step explanation:

Note that 1 inch = 2.54cm

Therefore, we'll convert the sides of the frame in inches to cm. This will be:

8 1/2 inch = 8.5 × 2.54cm = 21.59cm

11inches = 11 × 2.54cm = 27.94cm

Therefore, the perimeter of a rectangle will be:

= 2(length + width)

= 2(27.94 + 21.59)

= 2(49.53)

= 99.06cm

The probability that exactly one of the next three vehicles fail is 0.737 .

Given :

64% of all vehicles examined at a certain emissions inspection station pass the inspection. assuming that successive vehicles pass or fail independently of one another .

probability that exactly one of the next three vehicles fail is :

P = 1 -  probability that all of the next three vehicles passes .

= 1 - 64 % * 64 % * 64 %

= 1 - 64/100 - 64/100 - 64/100

= 1 - 0.64 * 0.64 * 0.64

= 1 - 0.4096 * 0.64

= 1 - 0.262

= 0.737

Learn more about the probability here:

https://brainly.com/question/11234923

#SPJ4

Answer:

y = -3x +15

Step-by-step explanation:

(2,9) (4,3)

slope is -6/2 or -3

use slope intercept form to solve for y-intercept

y = mx + b

9 = (-3)(2) + b

9 = -6 + b

15 = b

y = -3x + 15

Answer:

112°

Step-by-step explanation:

Angles on a line add to 180°.

Using the Central Limit Theorem, it is found that the measures are given by:

a) 2,500,000.

b) 88,388.35.

c) 2,500,000.

By the Central Limit Theorem, the sampling distribution of sample means of size n for a population of mean \(\mu\) and standard deviation \(\sigma\) has the same mean as the population, but with standard deviation \(s = \frac{\sigma}{\sqrt{n}}\)

Hence, we have that for options a and c, the mean is of 2,500,000 users, while for option b, the standard deviation is given by:

\(s = \frac{\sigma}{\sqrt{n}} = \frac{625000}{\sqrt{50}} = 88,388.35.\)

More can be learned about the Central Limit Theorem at https://brainly.com/question/24663213

The graph of the function \(f(x) = (9/4)x^2\) is a symmetric upward-opening parabola.

Overall, the graph represents a quadratic function with a positive leading coefficient, resulting in an upward-opening parabolic curve. The graph has been attached.

For more questions on graph:

https://brainly.com/question/19040584

#SPJ8

Given the radical:

Let's reduce the radical.

Factor out 16 from 224

Rewrite 16 as 4²:

Thererfore, the simplified radical is:

Answer:

6 feet :/

Step-by-step explanation:

Answer:

52 in.

Step-by-step explanation:

Answer:

X=-2

Hope this helps! :]

The probability of choosing a red marble is 6/17.

The probability of an event occurring is the number of favorable outcomes divided by the total number of possible outcomes.

To calculate this probability, we can use the formula:

P(red marble) = number of red marbles / total number of marbles

In this case, we substitute the numbers we know:

P(red marble) = 6 / 17

So the probability of choosing a red marble is 6/17 or approximately 0.35.

Read more about probability at

https://brainly.com/question/251701

#SPJ1

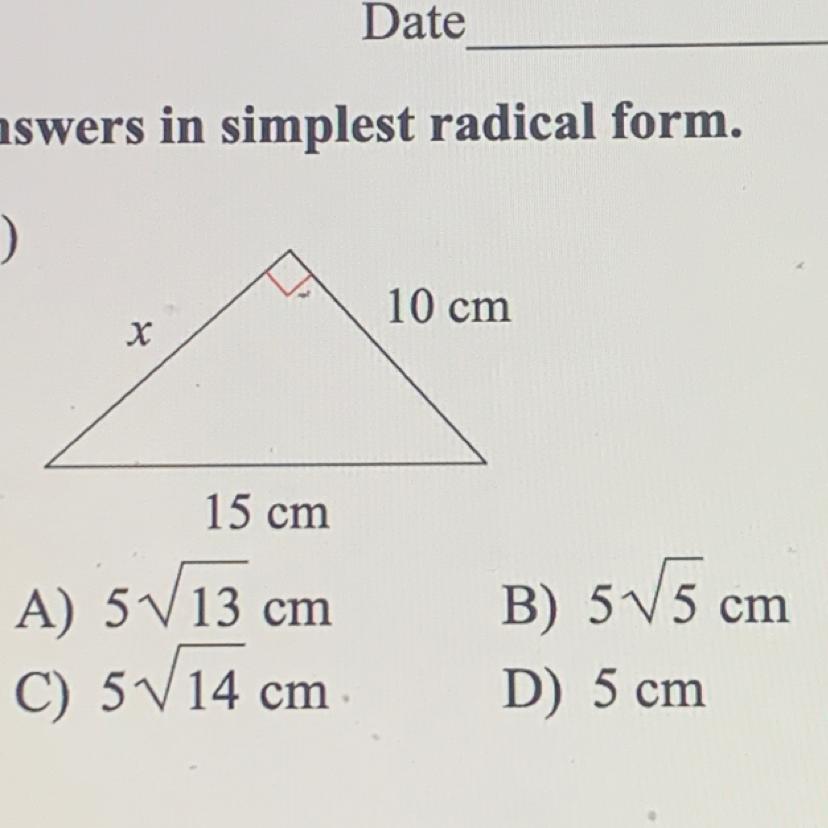
Answer:

Step-by-step explanation:

(y/10)^2+(x/30)^2=1

y^2/100+x^2/900=1

9y^2+x^2=900

9y^2=900-x^2

y^2=(900-x^2)/9, when x=8 we have

y^2=(900-8^2)/9

y^2=(900-64)/9

y^2=836/9

y=9.6 meters

The arch is 9.6 meters above the river at a distance of 8 meters from the center.

Answer:

339.29

Step-by-step explanation:

total circle area = 452.39

why?

because area of circle equation = pi * r^2

pi is the weird symbol. pi = 3.14

r = radius

r = 12

3.14 * 12^2 = 452.389342

simplified to 452.39.

but that is the whole thing.

we want only the shaded area.

first we will find the area the not shaded bit. then we will minus the total area, 452.39, by the not shaded bit so we only have the shaded bit.

again, area of circle equation = pi * r^2

r = 6.

3.14 * 6^2 = 113.097336

this is the not shaded bit. it can be simplified to 113.1

total area - not shaded bit = shaded bit

452.39 - 113.1 = 339.29

Step 1: Write the dividend and divisor:

\(\sf\:\frac{{x^3 - 8x^2 + 6x + 41}}{{x - 4}} \\ \)

Step 2: Divide the first term of the dividend by the first term of the divisor:

\(\sf\:\frac{{x^3}}{{x}} = x^2 \\ \)

Step 3: Multiply the divisor (x - 4) by the result (x^2):

\(\sf\:(x - 4) \cdot (x^2) = x^3 - 4x^2 \\ \)

Step 4: Subtract the result from the original dividend:

\(\sf\:(x^3 - 8x^2 + 6x + 41) - (x^3 - 4x^2) = -4x^2 + 6x + 41 \\ \)

Step 5: Bring down the next term from the dividend:

\(\sf\:\frac{{-4x^2 + 6x + 41}}{{x - 4}} \\ \)

Step 6: Repeat steps 2-5 with the new dividend:

\(\sf\:\frac{{-4x^2}}{{x}} = -4x \\ \)

\(\sf\:(x - 4) \cdot (-4x) = -4x^2 + 16x \\ \)

\(\sf\:(-4x^2 + 6x + 41) - (-4x^2 + 16x) = -10x + 41 \\ \)

Step 7: Bring down the next term from the dividend:

\(\sf\:\frac{{-10x + 41}}{{x - 4}} \\ \)

Step 8: Repeat steps 2-5 with the new dividend:

\(\sf\:\frac{{-10x}}{{x}} = -10 \\ \)

\(\sf\:(x - 4) \cdot (-10) = -10x + 40 \\ \)

\(\sf\:(-10x + 41) - (-10x + 40) = 1 \\ \)

Step 9: There are no more terms to bring down, so the division is complete.

Step 10: Write the final result:

The quotient is \(\sf\:x^2 - 4x - 10\\\) and the remainder is 1.

Therefore, the division of \(\sf\:(x^3 - 8x^2 + 6x + 41) by (x - 4) \\\) is:

\(\sf\:(x^3 - 8x^2 + 6x + 41) ÷ (x - 4) \\ \) \(\sf\:= x^2 - 4x - 10 + \frac{{1}}{{x - 4}} \\ \)