Martin is selling chocolate bars for a fundraiser. His goal is to sell 500 chocolate bars to win the top prize. He can sell 11 per hour by standing in front of the local grocery store. He already sold 258, and he uses the equation 11x+258=500 to find out how many more hours he needs to sell for.
Solve for x.

Answers
Answer:
X=22
Step-by-step explanation:
258 ÷ 11 = 23.454 repeating. 22 = X is the closest answer to 23. High chance it is 22.
Related Questions
Jane has a pile of 6 books. Together, the books weigh 4 pounds. If the books are
similar in weight, about how much does each book weigh?
Btw the answers not 2 lbs bc 2lb x 6 = 10 lbs and it needs to equal 4 lbs.
Answers
Answer:
\(\frac{2}{3}\) lbs per book
Step-by-step explanation:
We can divide 4 pounds by the total number of books, 6, to find out how much each book weighs.
4 lbs / 6 books = \(\frac{4/2}{6/2}=\frac{2}{3}\) lbs
Each book weighs \(\frac{2}{3}\) lbs
2/3 is equal to about 0.667
Another thing I wanted to note, 2 lbs * 6 = 12 lbs, not 10 lbs.
Help !! Will mark brainlest !!
A flowering plant stands 6.5 inches tall when its placed under a growing light. Its growth is 0.25 inches per day. Which of the following equations represents this situation?
A- y=0.25x+6.5
B- y=6.5x+0.25
C- y= -0.25x+6.5
D- y= - 6.5x+0.25
Answers
Answer: the answer would be a-y=0.25x+6.5
\(3/5 of 5/9\\\)
Answers
Answer:
⅓
Step-by-step explanation:\( \frac{5}{9} \times \frac{3}{5} \)
\( = \frac{1}{3} \times \frac{1}{1} \)
\( = \frac{1}{3} \)
Checking:\( \frac{1}{3} \div \frac{3}{5} \)
\( = \frac{1}{3} \times \frac{5}{3} \)
\( = \frac{5}{9} \)
#CarryOnLearning
Hey there!
When you see the word “of” in mathematical statement, it usually means “multiply” (aka Multiplication)
“3/5 of 5/9”
3/5 • 5/9
MULTIPLY the NUMERATORS (TOP NUMBERS) from each other & also MULTIPLY the DENOMINATORS (BOTTOM NUMBERS) from each other!
3(5) / 5(9)
3(5) = 15 ⬅️ NUMERATOR
5(9) = 45 ⬅️ DENOMINATOR
15/45
The GCF (Greatest Common Factor) for both of your numbers is: 15.. so DIVIDE both the NUMERATOR and DENOMINATOR from 15
15 ÷ 15 / 45 ÷ 15
15 ÷ 15 = 1 ⬅️ NUMERATOR
45 ÷ 15 = 3 ⬅️ DENOMINATOR
Answer: 1/3 ☑️
Good luck on your assignment and enjoy your day!
~LoveYourselfFirst:)
need help i dont understand

Answers
Answer:
Step-by-step explanation:
it would be "c" because the question asks for the number that makes the inequality true
4x≤ x+3
the graph in c is saying that every number that is 1 or less than 1 makes the inequality true. so lets take -7 as an example
4 x -7=-28
-28+3=-25
-25 is greater than -28 so the inequality is true. the reason why graph d doesnt work is because if we plug in 2 into the equation, then 4x =8 and x+3=5
5 is not greater than 8 so it doesnt work
City Park was receiving lots of rain. It rained 11 inches of rain in five and a half hours. What was the average rainfall for one hour?
Answers
Answer:
2
Step-by-step explanation:
11/5.5=2
5.5=5:30
Answer:
2.3
Step-by-step explanation:
PLEASE HELP NEEDS TO BE DONE IN 10 MINUTES!!!

Answers
Answer:
QR = 15 cm
PQ = 20 cm
Step-by-step explanation:
Use the Pythagorean theorem. \(a^{2} +b^{2} =c^{2}\)
Find QR first since you know QP are SR.
\(a^{2} +b^{2} =c^{2}\\12^{2} +9^{2} =c^{2}\\144+81=c^2 \\225=c^2\\\sqrt{225} = \sqrt{c^2} \\c = 15\)
QR = 15 cm
Now you can find PQ. First find the length of PR by adding PS and SR together.
16 + 9 = 25
\(a^{2} +b^{2} =c^{2}\\a^{2} +15^{2} =25^{2}\\a^{2} +225 =625\\a^{2} +225-225 =625-225\\\a^{2} = 400\\\sqrt{a^2} =\sqrt{400} \\a = 20\)
PQ = 20 cm
inverse the multiplier into its reciprocal fraction and proceed into multiplication of fractions. 1 1 1 4 8 Soluuon: 1 3 1 1 3 1 9 Solution
Answers
Answer:
Step-by-step explanation:
To complete the steps to demonstrate why you multiply by the reciprocal when dividing fractions.
To find
Step 1:
Rewrite it as
.
Step 2:
Multiply the numerator and the denominator by the reciprocal of
.
A passenger train traveled 180 miles in the same amount of time it took a freight train to travel 120 miles. The rate of the freight train was 15 miles per hour slower than the rate of the passenger train. Find the rate of the passenger train.
Answers
Answer:
The passenger train is moving at 45 miles per hour
Step-by-step explanation:
Let the amount of time it took the two trains to travel the distance = t.
Since the two trains traveled the distance at the same time,
Rate of the passenger train =\(\frac{180}{t}\)
Rate of the freight train = \(\frac{120}{t}\)
Where t is in hours.
From the problem, we can see that the rate of the freight train was 15 miles per hour slower than the rate of the passenger train. Mathematically, we can represent this as
\(\frac{120}{t}= \frac{180}{t}-15\)
from the above equation, we can now get our value for t as
\(\frac{120-180}{t}=-15\\\frac{-60}{t}=-15\\t=4 hours\)
We have our time of travel for the two trains as 4 hours.
The rate of the passenger train can now be calculated by 180/4 = 45 miles per hour
A school has 726 students. There are 340 boys. About what percent of the students are girls?
Answers
Answer: 348 girls so somewhere around 80 percent
Step-by-step explanation:
write -4i+(1/4-5i)-(-3/4+8i)+17i as a complex number in the standard form
Answers
Answer:
1
Step-by-step explanation:
Eliminate parentheses using the distributive property.
= -4i +1/4 -5i +3/4 -8i +17i
= (1/4 +3/4) +i(-4 -5 -8 +17)
= 1 + 0i
= 1
Solve the system graphically and check the solution. 2x+y=10. Y = -2
Answers
we have the system
2x+y=10
y=-2
step 1
Find out the intercepts
Line 1
2x+y=10
y-intercept ----> (0,10)
x-intercept ----> (5,0)
Line 2
y=-2
Is a horizontal line that passes through the point (0,-2)
step 2
Graph the given lines
see the attached figure
the intersection point is (6,-2)
that means
the solution is
x=6
y=-2
check the solution
substitute the value of x and y in equation 1
2x+y=10
2(6)+(-2)=10
12-2=10
10=10 ----> is true
that means ----> the solution is correct

Solve for the exact solutions in the interval [0,2]
List your answers separated by a comma, if it has no real solutions, enter DNE.
![Solve for the exact solutions in the interval [0,2] List your answers separated by a comma, if it has](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/T4DZjcEt3TRBDPvOs0HF3QykR4WfDi8B.png)
Answers
The solutions to the trigonometric equation in this problem are given as follows:
θ = π/6.θ = 5π/6.θ = 7π/6.θ = 11π/6.What are complementary angles?Two angles are said to be complementary angles when the sum of their measures is of 90º.
For complementary angles, we have that the sine of one of the angles is the cosine of the other angle, hence in this problem we have that 2θ and θ are complementary angles.
Then the value of θ is obtained as follows:
2θ + θ = 90
3θ = 90
θ = 30º.
θ = π/6.
The equivalent angles, which are also solutions to the equation, are given as follows:
θ = 5π/6. -> second quadrant.θ = 7π/6. -> third quadrant.θ = 11π/6. -> fourth quadrant.More can be learned about complementary angles at brainly.com/question/98924
#SPJ1
Please help!!
Thankyou!!

Answers
The scale factor is: 1.8
because of :
\(\frac{36}{20}=1.8\\ \\\\\frac{25.2}{14}=1.8\)
for each of the following, determine the constant c so that f(x) satisfies the conditions of being a pmf for a random variable x, and then depict each pmf as a line graph: (a) f(x) = x/c(b) f(x) = cx(c) f(x) = c(1/4)^x(d) f(x) = c(x + 1)^2(e) f(x) = x/c(f) f(x) = c/(x+1)(x+2)Hint: In part (f), write f(x) = 1/(x+1) - 1/(x+2)
Answers
A probability mass function (pmf) for a random variable X must satisfy the following two conditions:
1. f(x) ≥ 0 for all x
2. Σf(x) = 1
(a) f(x) = x/c
To find the constant c, we can use the second condition and sum over all possible values of x:
Σf(x) = Σ(x/c) = 1/c Σx = 1
Since we don't have a specified range for x, we cannot solve for c.
(b) f(x) = cx
Again, we can use the second condition to find the constant c:
Σf(x) = Σ(cx) = cΣx = 1
Without a specified range for x, we cannot solve for c.
(c) f(x) = c(1/4)^x
Using the second condition:
Σf(x) = Σ(c(1/4)^x) = cΣ(1/4)^x = 1
We can use the formula for an infinite geometric series to find the sum:
Σ(1/4)^x = 1/(1 - 1/4) = 4/3
So, c = 3/4
(d) f(x) = c(x + 1)^2
Using the second condition:
Σf(x) = Σ(c(x + 1)^2) = cΣ(x + 1)^2 = 1
Without a specified range for x, we cannot solve for c.
(e) f(x) = x/c
This is the same as part (a), so we cannot solve for c without a specified range for x.
(f) f(x) = c/(x+1)(x+2)
Using the hint, we can rewrite f(x) as:
f(x) = c(1/(x+1) - 1/(x+2))
Using the second condition:
Σf(x) = Σ(c(1/(x+1) - 1/(x+2))) = cΣ(1/(x+1) - 1/(x+2)) = 1
We can use the formula for a telescoping series to find the sum:
Σ(1/(x+1) - 1/(x+2)) = 1 - 1/(x+2)
As x approaches infinity, the sum approaches 1.
So, c = 1
To depict each pmf as a line graph, we would plot the values of f(x) on the y-axis and the values of x on the x-axis. However, without a specified range for x, we cannot create accurate graphs for parts (a), (b), (d), and (e). For part (c), the graph would be a decreasing exponential curve, with f(x) approaching 0 as x increases. For part (f), the graph would be a decreasing curve, with f(x) approaching 0 as x increases.
Learn more about probability mass function:
brainly.com/question/14932242
#SPJ11
Find all solutions to the equation x3 + 16x – 3x2 = 48.
–4, –3, 4
–4, 3, 4
3, –4i, 4i
–3, –4i, 4i
Answers
The solutions of the quadratic equation x³+ 16x -3x² = 48 are 3, –4i, 4i
What is a quadratic equation?A quadratic equation is the second-order degree algebraic expression in a variable. the standard form of this expression is ax² + bx + c = 0 where a. b are coefficients and x is the variable and c is a constant.
A mathematical statement with two expressions that have the same value and the sign "equal to" between them is called an equation.
We are given the quadratic equation as;
x³+ 16x -3x² = 48
Subtract 48 from both sides;
x³+ 16x -3x² - 48= 48 -48
x³+ 16x -3x² - 48= 0
Factor left side of equation.
(x - 3)( x² + 16) = 0
Therefore, the roots are 3, –4i, 4i
Learn more about quadratic equations;
brainly.com/question/17177510
#SPJ1
Jack decides to estimate the volume of a coffee cup by modeling it as a right cylinder. Jack measures its radius as 2.2 cm and its volume as 117 cubic centimeters. Find the height of the cup in centimeters. Round your answer to the nearest tenth if necessary.
Answers
If you find the area of the base circle, and you know the volume, you can find the height.
To find the area of the base circle, we follow the formula a = pi x radius^. 2.2^2 is 4.84, and pi x 4.84 is approximately 15.2 cm^2.
Now we divide the volume by the area, so 117 / 15.2 to get 7.69736842, which we round to the nearest tenth to get 7.7 centimeters.
Greg deposits $500 into an account that pays simple interest at a rate of % 3 per year. How much interest will he be paid in the first 6 years?
Answers
Answer: 90 dollars
Work Shown:
i = p*r*t
i = 500*0.03*6
i = 90
Leach Inc. experienced the following events for the first two years of its operations:
Year 1:
Issued $10,000 of common stock for cash.
Provided $100,000 of services on account.
Provided $27,000 of services and received cash.
Collected $73,000 cash from accounts receivable.
Paid $16,000 of salaries expense for the year.
Adjusted the accounting records to reflect uncollectible accounts expense for the year. Leach estimates that 8 percent of the ending accounts receivable balance will be uncollectible.
Year 2:
Wrote off an uncollectible account for $680.
Provided $120,000 of services on account.
Provided $20,000 of services and collected cash.
Collected $102,000 cash from accounts receivable.
Paid $26,000 of salaries expense for the year.
Adjusted the accounts to reflect uncollectible accounts expense for the year. Leach estimates that 8 percent of the ending accounts receivable balance will be uncollectible.
Exercise 5-9A (Algo) Parts a to c
Required
Organize the transaction data in accounts under an accounting equation.
Prepare the income statement, statement of changes in stockholders’ equity, balance sheet, and statement of cash flows for Year 1.
What is the net realizable value of the accounts receivable at December 31, Year 1?
Answers
The net realizable value of accounts receivable at December 31, Year 1, is the expected amount the company will collect from its accounts receivable. Since 8 percent of the ending accounts receivable balance is estimated to be uncollectible, we need to calculate it:
Ending accounts receivable balance: $27,000
Uncollectible accounts: $27,000 * 8% = $2,160
Net realizable value: $27,000 - $2,160 = $24,840.
To organize the transaction data in accounts under an accounting equation, we need to identify the accounts involved in each transaction and classify them into their respective categories: assets, liabilities, and equity.
Year 1:
1. Issued $10,000 of common stock for cash:
- Increase in cash (asset) by $10,000
- Increase in common stock (equity) by $10,000
2. Provided $100,000 of services on account:
- Increase in accounts receivable (asset) by $100,000
- Increase in service revenue (equity) by $100,000
3. Provided $27,000 of services and received cash:
- Increase in cash (asset) by $27,000
- Increase in service revenue (equity) by $27,000
4. Collected $73,000 cash from accounts receivable:
- Decrease in accounts receivable (asset) by $73,000
- Increase in cash (asset) by $73,000
5. Paid $16,000 of salaries expense for the year:
- Decrease in cash (asset) by $16,000
- Decrease in retained earnings (equity) by $16,000
6. Adjusted the accounting records to reflect uncollectible accounts expense for the year:
- Increase in uncollectible accounts expense (expense) by an amount based on the estimate
To prepare the financial statements for Year 1, we need to compile the information from the transactions:
Income Statement:
- Service revenue: $100,000 + $27,000 = $127,000
- Salaries expense: $16,000
- Uncollectible accounts expense: based on the estimate
Statement of Changes in Stockholders' Equity:
- Common stock: $10,000
Balance Sheet:
- Assets:
- Cash: $10,000 + $27,000 - $73,000 = $-36,000
- Accounts receivable: $100,000 - $73,000 = $27,000
- Liabilities: None
- Equity:
- Common stock: $10,000
- Retained earnings: based on the income statement
Statement of Cash Flows:
- Cash flows from operating activities: $27,000 - $73,000 - $16,000 = $-62,000
- Cash flows from financing activities: $10,000
- Cash flows from investing activities: None
The net realizable value of accounts receivable at December 31, Year 1, is the expected amount the company will collect from its accounts receivable. Since 8 percent of the ending accounts receivable balance is estimated to be uncollectible, we need to calculate it:
Ending accounts receivable balance: $27,000
Uncollectible accounts: $27,000 * 8% = $2,160
Net realizable value: $27,000 - $2,160 = $24,840.
For more such questions on receivable balance, click on:
https://brainly.com/question/24230946
#SPJ8
Determine which set of side measurements could be used to form a right triangle.
4, 11, 20
16, 21, 25
5, 13, 25
3, 4, 5
Answers
The set of side measurements that could be used to form a right triangle is 3, 4 and 5 option (D) is correct.
What is a right-angle triangle?
It is a triangle in which one of the angles is 90 degrees and the other two are sharp angles. The sides of a right-angled triangle are known as the hypotenuse, perpendicular, and base.
It is given that:
The side length of the triangle is shown in the option:
As we know,
Pythagoras' theorem is the square of the hypotenuse in a right-angled triangle is equal to the sum of the squares of the other two sides.
Using Pythagoras' theorem:
From option(D):
3, 4 and 5
(3)² + (4)² = 5²
9 + 16 = 25 (True)
Thus, the set of side measurements that could be used to form a right triangle is 3, 4 and 5 option (D) is correct.
Learn more about the right-angle triangle here:
brainly.com/question/3770177
#SPJ1
what is the quotient of 1/6 and 2/9
Answers
Answer:
3/4
Step-by-step explanation:
What is the equation of the line that passes through (4, 3) and (-4, -1)?
Choices:
2x+1
y=2x+7
y=x/2 +1
y= -x/2 +7
Answers
The equation of the line that passes through (4, 3) and (-4, -1) is y = -x/2 + 5. So, correct option is A.
To find the equation of the line that passes through two given points, we use the slope-intercept form of a linear equation, which is:
y = mx + b
where m is the slope of the line and b is the y-intercept.
First, we need to find the slope of the line using the two given points. The formula for finding slope between two points is:
m = (y₂ - y₁) / (x₂ - x₁)
where (x₁, y₁) and (x₂, y₂) are the coordinates of the two points.
Substituting the given points into the formula, we get:
m = (-1 - 3) / (-4 - 4) = -4/8 = -1/2
Now we know the slope, we can use one of the given points (4, 3) and substitute the slope into the slope-intercept form of the equation and solve for b.
y = mx + b
3 = (-1/2)(4) + b
3 = -2 + b
b = 5
Therefore, the equation of the line that passes through (4, 3) and (-4, -1) is:
y = -x/2 + 5
So, correct option is A.
To learn more about equation click on,
https://brainly.com/question/29255626
#SPJ1
Complete question is:
What is the equation of the line that passes through (4, 3) and (-4, -1)?
Choices:
y = -x/2 + 5
y=2x+7
y=x/2 +1
y= -x/2 +7
An educational researcher devised a wooden toy assembly project to test learning in 6-year-olds. The time in seconds to assemble the project was noted, and the toy was disassembled out of the child's sight. Then the child was given the task to repeat. The researcher would conclude that learning occurred if the mean of the second assembly times was less than the mean of the first assembly times.
Find the 99% confidence interval for the difference in means.
Child
2
3
4
Trial 1
108 140
154
115
Trial 2
99
118 154
96
5
130
108
107
102
110
0 0.7
0-07<4-4 < 23.5
0-29
O 29
Answers
The differences are: -9, 22, 0, -19, -105, 24, -46, -8, -5, -8, -8
The mean of the differences is: -9.5
The standard deviation of the differences is: 38.3
To calculate the 99% confidence interval, we use the formula:
(mean difference) +/- (t-value) * (standard deviation of differences / square root of sample size)
The sample size is 11, so the degrees of freedom are 10. Using a t-table with 10 degrees of freedom and a 99% confidence level, we find the t-value to be 3.169.
Plugging in the values, we get:
-9.5 +/- 3.169 * (38.3 / sqrt(11))
Which simplifies to:
-9.5 +/- 23.5
Therefore, the 99% confidence interval for the difference in means is (-33, 14).
find the range of this equation

Answers
The range of the given equation is [-1, infinity).
We are given that;
Equation y= underroot(x+5)
Now,
The domain of this equation is the set of x values that make the expression under the square root non-negative.
That is, x+5 >= 0, or x >= -5. So the domain is [-5, infinity).
The range of this equation is the set of y values that are obtained by plugging in the domain values into the equation. Since the square root function is always non-negative, and we are subtracting 1 from it, the smallest possible value of y is -1, when x = -5. As x increases, y also increases, and there is no upper bound for y.
Therefore, by the range the answer will be [-1, infinity).
Learn more about the domain and range of the function:
brainly.com/question/2264373
#SPJ1
The random variable x is the number of occurrences of an event over an interval of ten minutes. It can be assumed that the probability of an occurrence is the same in any two time periods of an equal length. It is known that the mean number of occurrences in ten minutes is 5.3. (please show work)
1. Refer to Exhibit 2. The random variable x satisfies which of the following probability distributions?
a. normal b. Poisson c. binomial d. Not enough information is given to answer this question.
2. Refer to Exhibit 2. The probability that there are 8 occurrences in ten minutes is
a. .0241 b. .0771 c. .1126 d. .9107
3. Refer to Exhibit 2. The probability that there are less than 3 occurrences is
a. .0659 b. .0948 c. .1016 d. .1239
Answers
The random number x satisfies Poisson distribution of probability and the probability that there are 8 occurrences in ten minutes is 0.0241 and The probability that there are less than 3 occurrences is 0.0659.
Given,
The random variable x is the number of occurrences of an event over an interval of ten minutes. It can be assumed that the probability of an occurrence is the same in any two time periods of an equal length. It is known that the mean number of occurrences in ten minutes is 5.3.
1) A Poisson distribution characterizes the random variable x that is provided.
2) Having 8 occurrences in 10 minutes is likely based on the following formula:
P(X = 8) = (e^(-5.3) * 5.3^8) / 8! = 0.0241 (rounded to four decimal places) (rounded to four decimal places)
Consequently,
(a) 0.0241 is the correct response.
3) The likelihood that there are fewer than 3 occurrences is as follows:
P(X 3) = P(X = 0), P(X = 1), and P(X = 2) = (e(-5.3) * 5.30), (e(-5.3) * 5.31), and (e(-5.3) * 5.32), respectively, / 0!, / 1/1, and / 2/ respectively, / 2! = 0.0659 (rounded to four decimal places)
Accordingly,
(a) 0.0659 is the correct response.
The random number x satisfies Poisson distribution of probability and the probability that there are 8 occurrences in ten minutes is 0.0241 and The probability that there are less than 3 occurrences is 0.0659.
For similar questions on probability distributions,
https://brainly.com/question/29204567
#SPJ4
The random number x satisfies Poisson distribution of probability and the probability that there are 8 occurrences in ten minutes is 0.0241 and The probability that there are less than 3 occurrences is 0.0659.
Given,
The random variable x is the number of occurrences of an event over an interval of ten minutes. It can be assumed that the probability of an occurrence is the same in any two time periods of equal length. It is known that the mean number of occurrences in ten minutes is 5.3.
1) A Poisson distribution characterizes the random variable x that is provided.
2) Having 8 occurrences in 10 minutes is likely based on the following formula:
P(X = 8) = (\(e^(-5.3)\) * \(5.3^8\)) / 8! = 0.0241 (rounded to four decimal places) (rounded to four decimal places)
Consequently,
(a) 0.0241 is the correct response.
3) The likelihood that there are fewer than 3 occurrences is as follows:
P(X 3) = P(X = 0), P(X = 1), and
P(X = 2) = (\(e(-5.3)\)* 5.30), (\(e(-5.3)\)* 5.31), and (\(e(-5.3)\) * 5.32),
respectively, / 0!, / 1/1, and / 2/ respectively, / 2! = 0.0659 (rounded to four decimal places)
Accordingly,
(a) 0.0659 is the correct response.
The random number x satisfies Poisson distribution of probability and the probability that there are 8 occurrences in ten minutes is 0.0241 and The probability that there are less than 3 occurrences is 0.0659.
For similar questions on probability distributions,
brainly.com/question/29204567
#SPJ4
6 Farah is buying clothes from a website.
The website shows this information about a jacket Farah wants to buy.
Jacket
original price £30.99
sale price £16
The website claims this is a saving of 46%.
Is the sale price a saving of 46% on the original price?
Show why you think this.
they
Answers
Answer:
No ;
Step-by-step explanation:
Given that :
original price = £30.99
sale price = £16
The percentage saving :
(Original price - sale price) / original price * 100%
(£30.99 - £16) / £30.99 * 100%
£14.99 / £30.99 * 100%
= 0.4837 * 100%
= 48.37%
According to the calculation above, the sale price isn't a saving of 46% on the original price, rather it is a saving of 48.37% on the original price.
Mrs Brown and Mrs Jones make 4 dozen sandwiches in half an hour in Mrs Jones’ small kitchen. If they had 30 friends in to help, how many sandwiches could be made in the same time?
Answers
Answer:
1488 sandwiches in thirty minutes
Step-by-step explanation:
Solve sin(x) = 0.23 on 0 ≤ x < 2T.There are two solutions, A and B, with A
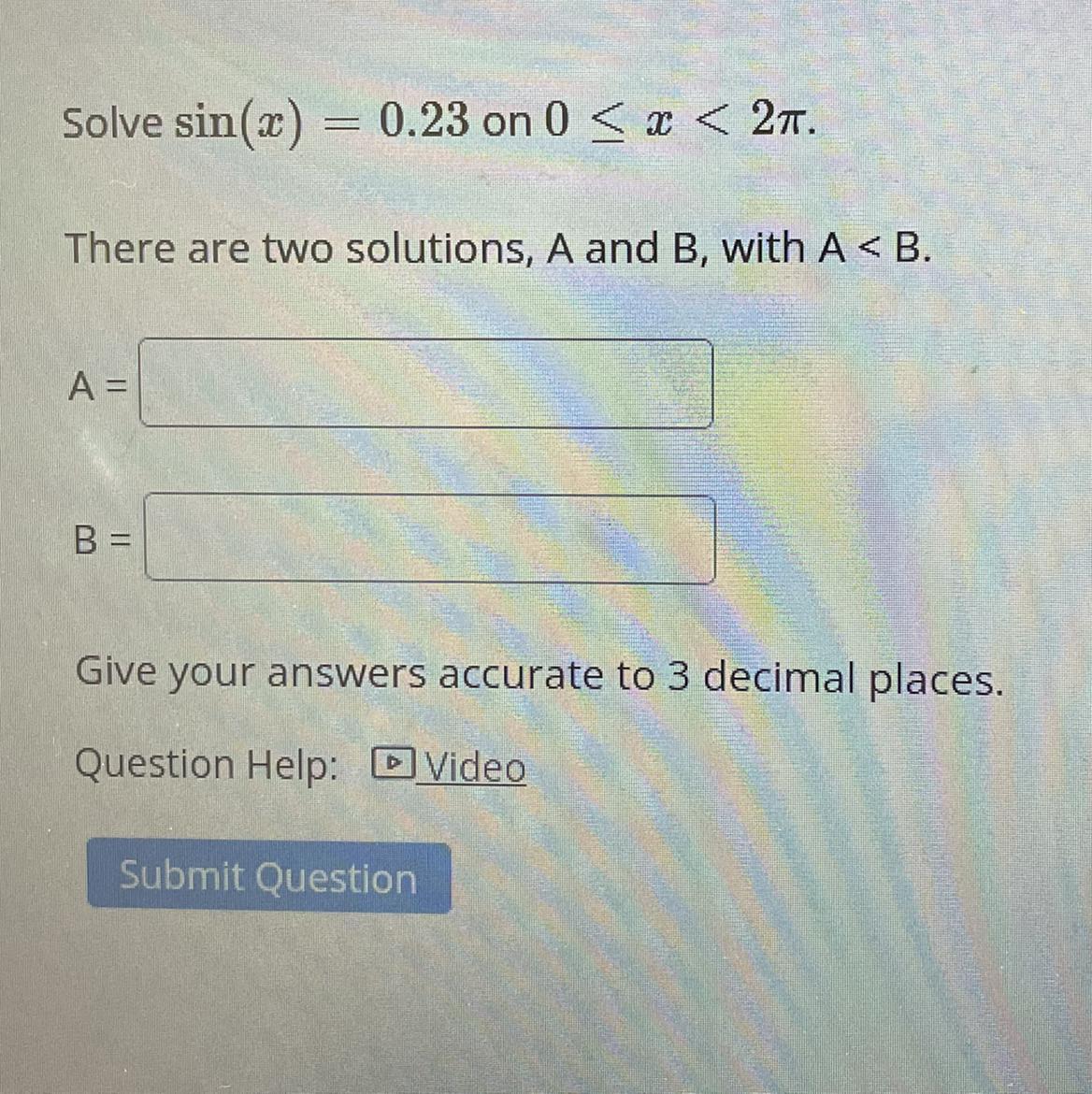
Answers
Given -
sin(x) = 0.23 on 0 ≤ x < 2π
To Find -
Two solutions, A and B =?
Step-by-Step Explanation -
\(0.23\text{ = }\frac{23}{100}\)So, the two solutions will be -
\(\begin{gathered} \sin^{-1}(\frac{23}{100})\text{ and }\pi\text{ - }\sin^{-1}(\frac{23}{100}) \\ \\ So,\text{ }\sin^{-1}(\frac{23}{100})\text{ = 0.232} \\ \\ Also,\text{ }\pi\text{ - }\sin^{-1}(\frac{23}{100})\text{ = 3.141 - 0.232 = 2.909} \end{gathered}\)Now, Since A < B
So,
A = 0.232
B = 2.909
Final Answer -
A = 0.232
B = 2.909
Avery's recipe ask for 100ml of milk. She only has a 10ml measuring cup. How many times will she need to fill her measuring cup to get the right amount of milk for her recipe
Answers
Ten times to get the required amount of 100ml of milk for her recipe.
Avery needs to measure 100ml of milk for her recipe but she only has a 10ml measuring cup.
Therefore, she needs to determine how many times she will need to fill her 10ml measuring cup to get the required amount of milk.
To calculate this, we can divide the required amount of milk by the capacity of the measuring cup. In this case, we divide 100ml by 10ml to get:
100ml ÷ 10ml = 10
This means that A very will need to fill her 10ml measuring cup 10 times to get the required amount of milk for her recipe.
Another way to think about it is to consider that 100ml is ten times the capacity of the 10ml measuring cup. Therefore, Avery will need to fill the measuring cup ten times to get the required amount of milk.
In summary, Avery will need to fill her 10ml measuring cup ten times to get the required amount of 100ml of milk for her recipe. t
for similar questions on recipe
https://brainly.com/question/25936585
#SPJ11
Question 8 of 10
If an 11-day single payment loan has a periodic interest rate of 9.3%, what is
the approximate effective interest rate of the loan?
A. 1812.0%
B. 19,120.0%
C. 18,120.0%
D. 1912.0%
Answers
The approximate effective interest rate of the loan is 19.12%, which corresponds to answer (B).
How to find interest rate?The formula for converting periodic interest rate to effective interest rate is
\($$(1+i_{eff})=(1+\frac{r}{m})^m$$\)
where \($i_{eff}$\)is the effective interest rate, r is the periodic interest rate, and m is the number of compounding periods in a year.
In this case, the loan has a single payment period of 11 days. To find the effective interest rate, we need to convert the periodic interest rate to an annual rate. There are different ways to do this, but one common method is to assume that a year has 365 days and use the following formula:
\($$i_{eff}=(1+\frac{r}{365})^{365/t}-1$$\)
where t is the time period in days.
Using this formula, we have:
\($$i_{eff}=(1+\frac{0.093}{365})^{365/11}-1\approx 0.1912$$\)
Therefore, the approximate effective interest rate of the loan is 19.12%, which corresponds to answer (B).
Top know more about Interest visit:
brainly.com/question/28792777
#SPJ1
Which statements are true about a decagonal (10-sided) pyramid? Select 3 options.
It has one face that is a decagon.
It has two faces that are decagons.
It has 11 faces.
It has 20 edges.
It has 20 vertices.
Answers
The correct statements are,
B)It has two faces that are decagons.
C)It has 11 faces.
D)It has 20 edges.
What is decagonal pyramid?
A decagonal pyramid is a solid whose base is a decagon or a ten sided figure, regular or irregular. The surfaces are ten isosceles triangles, in case of a regular decagonal pyramid with all the ten vertices meeting at a point.
Here The decagonal prism is formed by two regular decagons as its bases and 10 squares as its other faces. the top of the decagonal prism is a regular decagon, so is the bottom face.
We know that decagonal pyramid has 11 faces and 20 edges and 11 vertices.
Hence the correct statements are,
B)It has two faces that are decagons.
C)It has 11 faces.
D)It has 20 edges.
To learn more about decagonal pyramid refer the below link
https://brainly.com/question/22585969
#SPJ1