Let f(x,y)={ cx 2
y,
0,
if 0
elsewhere,
be the joint pdf of (X,Y). (a) Sketch the region for which f(x,y)>0. (b) Derive the value of c. (c) Find the marginal pdfs of X and Y (d) Determine the conditional pdf of X given Y=y. (e) Are X and Y independent? Explain. (f) Celculate P(X≤Y). (g) Calculate P(X≤0.4∣Y≥0.5).
Answers
(a) The region for which f(x,y) > 0 is the region where the joint probability density function (pdf) is positive. In this case, f(x,y) = cx^2y. Since the pdf is only positive when cx^2y is positive, it means that the region is defined by the inequality cx^2y > 0. This implies that both x and y must be non-zero for f(x,y) to be positive. Therefore, the region where f(x,y) > 0 is the entire xy-plane excluding the x and y axes.
(b) To determine the value of c, we need to integrate the joint pdf over its entire support and set it equal to 1, since the joint pdf must integrate to 1 over its support to be a valid probability density function.
∫∫f(x,y) dxdy = ∫∫cx^2y dxdy
Since the region of integration is the entire xy-plane, we can integrate with respect to x first:
∫(∫cx^2y dx)dy = ∫(c/3 * x^3y)dy
Integrating with respect to y now, over the entire range of y:
∫(c/3 * x^3y)dy = c/3 * x^3 * [y^2/2] = c/6 * x^3 * y^2
To find the value of c, we set this expression equal to 1 and solve for c:
c/6 * x^3 * y^2 = 1
c = 6 / (x^3 * y^2)
(c) The marginal pdf of X can be obtained by integrating the joint pdf over the range of y:
fX(x) = ∫f(x,y) dy
∫cx^2y dy = c/2 * x^2 * y^2
To find the value of c, we integrate fX(x) over its entire support, which is the range of x:
∫fX(x) dx = ∫c/2 * x^2 * y^2 dx = 1
Integrating, we get:
c/2 * y^2 * [x^3/3] = 1
c * y^2 / 6 = 1
c = 6 / y^2
Therefore, the marginal pdf of X is fX(x) = (6 / y^2) * x^2.
Similarly, we can find the marginal pdf of Y by integrating the joint pdf over the range of x:
fY(y) = ∫f(x,y) dx
∫cx^2y dx = c/3 * x^3 * y
Integrating fY(y) over its entire support, which is the range of y, we get:
∫fY(y) dy = ∫c/3 * x^3 * y dy = 1
Integrating, we have:
c/3 * x^3 * [y^2/2] = 1
c * x^3 / 6 = 1
c = 6 / x^3
Therefore, the marginal pdf of Y is fY(y) = (6 / x^3) * y.
(d) The conditional pdf of X given Y=y can be obtained using the formula:
fX|Y(x|y) = f(x,y) / fY(y)
Substituting the values of f(x,y), fY(y), and c derived earlier:
fX|Y(x|y) = (cx^2y) / [(6 / x^3) * y] = cx^5 / 6
Therefore, the conditional pdf of X given Y=y is fX|Y(x|y) = cx^5 / 6.
(e) To determine if X and Y are independent, we need to check if the joint pdf f(x,y) can be expressed as the product of the marginal pdfs fX(x) and fY(y). However, in this case, the joint pdf f(x,y) = cx^2y cannot be factorized into the product of the marginal pdfs (6 / y^2) * x^2 and (6 / x^3) * y. Therefore, X and Y are not independent.
(f) To calculate P(X ≤ Y), we integrate the joint pdf f(x,y) over the region where X ≤ Y:
P(X ≤ Y) = ∫∫f(x,y) dxdy
Since f(x,y) = cx^2y, the integral becomes:
∫∫cx^2y dxdy
Integrating over the appropriate region, we get:
∫(∫cx^2y dx)dy
The limits of integration will depend on the specific range of x and y.
(g) To calculate P(X ≤ 0.4 | Y ≥ 0.5), we need to find the conditional probability of X being less than or equal to 0.4 given that Y is greater than or equal to 0.5. This can be computed by integrating the conditional pdf fX|Y(x|y) over the range where X ≤ 0.4 and Y ≥ 0.5, and dividing it by the probability of Y being greater than or equal to 0.5:
P(X ≤ 0.4 | Y ≥ 0.5) = ∫(∫fX|Y(x|y) dx) dy / P(Y ≥ 0.5)
The limits of integration will depend on the specific range of x and y in the given conditions.
Learn more about joint probability density function here:
brainly.com/question/31129873
#SPJ11
Related Questions
Write the equation of the line shown on the coordinate plane below?
4
3
لا
2
1
-8-7 -6 -5 -4 -3 -2 -1
-1
-2
-3
-4
2 3 4 5 6 7 8
Answers
The equation of line shown on the coordinate plane is,
⇒ y = - 1/4x
What is Equation of line?The equation of line in point-slope form passing through the points
(x₁ , y₁) and (x₂, y₂) with slope m is defined as;
⇒ y - y₁ = m (x - x₁)
Where, m = (y₂ - y₁) / (x₂ - x₁)
Given that;
Two points on the line are (0, 0) and (4, -1).
Now,
Since, The equation of line passes through the points (0, 0) and (4, -1).
So, We need to find the slope of the line.
Hence, Slope of the line is,
m = (y₂ - y₁) / (x₂ - x₁)
m = (- 1 - 0) / (4 - 0)
m = - 1 / 4
Thus, The equation of line with slope - 1/4 is,
⇒ y - 0 = - 1/4 (x - 0)
⇒ y = - 1/4x
Therefore, The equation of line passes through the points (0, 0) and
(4, -1) will be;
⇒ y = - 1/4x
Learn more about the equation of line visit:
https://brainly.com/question/18831322
#SPJ9

A number is greater than 16 and less than 29. This number has exactly 4 factors.
The sum of its factors is 42.
What is the number
Answers
Answer:
6+15+21=42.
Step-by-step explanation:
21 is greater than 16
15 is less than 29
1*21=21
3*7=21
7*3=21
factors of 21 are 1, 3, 7 and 21
1*15=15
3*5=15
5*3=15
factors of 15 are 1, 3, 5 and 15
6+15+21=42
1*6=6
2*3=6
3*2=6
factors of 6 are 1, 2, 3 and 6
Plssssss help me quickly

Answers
The given system of equations have infinitely many solutions and lines of both will overlap. (graph is attached)
What is a system of equations?A "system" of equations is a set or collection of equations that you deal with all together at once.
Given is a system of equations
y = 6+x
y = x+6
Here, the slope and y-intercept is same for both the lines,
We know that, If the two lines have the same y-intercept and the slope, they are actually overlapping lines.
Hence, the given system of equations have infinitely many solutions and lines of both will overlap.
Learn more about system of equations, click;
https://brainly.com/question/90105
#SPJ1

Andrew collects coins, He collected a total of 150 coins. If 72% of the coins he collected were foreign,
how many other coins did he collect?
Answers
He would of collected 42 coins. (108 foreign coins) and he would of collected 42 that weren't foreign.
Step-by-step explanation:
A table of values of a linear function is shown below.
Find the slope and y-intercept of the function's graph, and find the equation for the function.

Answers
y-intercept: (0,-7)
15. Describe the three general steps
for producing a recombinant DNA (rDNA) vector, state how rDNA can
be introduced into cells, and discuss the clinical applications of
rDNA.
Answers
Producing rDNA involves isolating and cleaving DNA, inserting fragments into a vector, and transforming host cells. rDNA can be introduced via transformation, transfection, or viral vectors. Clinical applications include protein production, gene therapy, vaccines, and diagnostics.
Producing a recombinant DNA (rDNA) vector involves several general steps. Here are the three main steps involved in the process:
Isolation and Cleavage of DNA:
The first step is to isolate the desired DNA fragments from the source organism. This can be done using various techniques such as PCR (Polymerase Chain Reaction) or restriction enzyme digestion. Restriction enzymes are enzymes that cut DNA at specific recognition sites. By using the appropriate restriction enzymes, the desired DNA fragment and a vector DNA can be cut at specific sites. The vector is usually a plasmid, which is a small circular DNA molecule.
Insertion of DNA Fragments into the Vector:
Once the DNA fragments and vector have been cut, they are mixed together and joined through a process called ligation. DNA ligase is used to catalyze the formation of covalent bonds between the ends of the DNA fragments and the vector. This creates a recombinant DNA molecule containing the desired DNA fragment within the vector. The recombinant DNA molecule is then introduced into host cells for replication.
Transformation of Host Cells:
The recombinant DNA molecules need to be introduced into host cells to produce multiple copies of the recombinant DNA. This is typically done using a process called transformation. Host cells, such as bacteria or yeast, are treated in a way that makes them more receptive to taking up the recombinant DNA. Methods for transformation include heat shock, electroporation, or using chemical agents. Once the host cells have taken up the recombinant DNA, they can be grown in culture to produce large quantities of the desired DNA fragment.
Introduction of rDNA into Cells:
Recombinant DNA can be introduced into cells using various methods, depending on the type of cells being targeted. Some common techniques include:
Transformation: As mentioned earlier, host cells, such as bacteria or yeast, can be treated to make them receptive to taking up the recombinant DNA. This can be achieved by exposing the cells to heat shock, electroporation, or using chemical agents.
Transfection: This method is used for introducing rDNA into eukaryotic cells, including animal cells. It involves the use of techniques such as calcium phosphate precipitation, liposome-mediated transfection, or electroporation.
Viral Vectors: Certain viruses, such as retroviruses, adenoviruses, or lentiviruses, can be modified to carry the recombinant DNA. These viral vectors can then infect target cells and deliver the rDNA into the host genome.
Clinical Applications of rDNA:
Recombinant DNA technology has revolutionized biomedical research and has led to numerous clinical applications. Some important applications include:
Production of Therapeutic Proteins: rDNA technology allows for the production of large quantities of therapeutic proteins, such as insulin, growth factors, clotting factors, and monoclonal antibodies. These proteins can be used to treat various diseases, including diabetes, cancer, and genetic disorders.
Gene Therapy: rDNA vectors can be used to deliver functional copies of genes into target cells to correct genetic defects. This holds promise for the treatment of inherited diseases caused by single gene mutations, such as cystic fibrosis and muscular dystrophy.
Vaccine Development: Recombinant DNA technology has been instrumental in the development of vaccines. By expressing specific antigens from pathogens, recombinant vaccines can be created to stimulate an immune response without causing disease.
Diagnostic Tools: Recombinant DNA techniques are used to produce specific DNA or RNA probes for diagnostic purposes. These probes can detect the presence of specific genes or mutations associated with diseases, aiding in early detection and personalized medicine.
for such more question on isolating
https://brainly.com/question/27746495
#SPJ8
{4z=8}
{3x-2z=11}
{x-4y+z=11}
Answers
Step-by-step explanation:
4z = 8
z = 8/4
z = 2
3x - 2z = 11
3x - 2.2 = 11
3x - 4 = 11
3x = 11 + 4
3x = 15
x = 15/3
x = 5
x - 4y + z = 11
5 - 4y + 2 = 11
-4y = 11 - 5 - 2
-4y = 4
y = 4/-4
y = -1
Solution → {x,y,z} = {5,-1,2}
Given the graph below, the equation in slope-intercept form is

Answers
Answer:
F(x)=-1x-5
Step-by-step explanation:
The line goes one down for every one to the right. and the line intersects at -5 on the y-axis.
Greyson and Preston have a piece of paper that is 10 inches by 9 inches. What is the area of the paper?
Answers
Answer:
90
Step-by-step explanation:
Need help real quick,I have been on this question for so long

Answers
Given the expression that models the tip amount for a total bill of $102:
\(0.25\mleft(102-x\mright)\)You know that "x" represents the dollar amount of the tax.
You also know that Mr. Jaxon tips $23.50.
Therefore, knowing all that information, you can set up the following equation to represent that situation:
\(23.50=0.25\mleft(102-x\mright)\)Now you have to solve for "x" in order to find its value:
\(\begin{gathered} 23.50=(0.25)(102)-(0.25)(x) \\ \\ 23.50=25.5-0.25x \end{gathered}\)\(\begin{gathered} 23.50-25.5=-0.25x \\ \\ -2=-0.25x \end{gathered}\)\(\begin{gathered} \frac{-2}{-0.25}=x \\ \\ x=8 \end{gathered}\)Hence, the answer is:
\(\text{ \$}8\)Find the equation of the parabola with its focus at (6, 2) and its directrix y = 0
Answers
Answer:
y= 1/4 x-62+1
Step-by-step explanation:
in the 2018 Drake Relays, 20 high-school girls and 23 college women competed in the high jump finals. Megan's Mnning jump in the high-school competition had a z-score of 2.51. Janae's winning jump in the college competition had a z-score of 2.00. Which statement correctly compares the Z-scores? O Megan's jump is more impressive because a positive z-score means that the jump was longer than the competitions' jumps, and her z-score is greater than Janae's. O Megan's jump is more impressive because a positive Z-score means that the jump was shorter than the competitions' jumps, and her Z-score is greater than Janae's. O Janae's jump is more impressive because her z-score is closer to the mean than Megan's. Janae's jump is more impressive because her z-score is smaller than Megan's.
Answers
Answer:
The person above me is right, and deserves brainliest.
(A) Megan’s jump is more impressive because a positive z-score means that the jump was longer than the competitions’ jumps, and her z-score is greater than Janae’s.
ED2021
Answer:
A) Megan’s jump is more impressive because a positive z-score means that the jump was longer than the competitions’ jumps, and her z-score is greater than Janae’s.
Step-by-step explanation:
edge 2023
Just say "Yes" or "No"
Answers
Answer:
No
Step-by-step explanation:
Answer:
yes
Step-by-step explanation:
Rose bought 60 feet of wire for $16.80 she needs 40 more feet. if the unit price is the same, how much will she pay for the extra 40 feet for wire?
Answers
Answer:
she will pay $11.20
Step-by-step explanation:
16.80÷60=0.28
0.28x40=11.20
hope this helps
Which of the following will form the composite function G(F(x)) shown
below?
G(F(x)) = 3√x +2
A. F(x)=√√x + 2 and G(x) = 3
OB. F(x)=√x and G(x) = 3x + 2
C. F(x) = 3x + 2 and G(x) = √√x
D. F(x) = 3√√x and G(x) = 2

Answers
F(x) = \(\sqrt x\) and G(x) \(= 3x + 2\) forms the composite function G(F(x)).
When F(x) = \(\sqrt x\) and G(x) \(= 3x + 2\) , the composite function G(F(x)) is given as follows:
G(F(x)) = G(\(\sqrt x\))
= \(3(\sqrt x) + 2\)
= \(3\sqrt x + 2\)
\(\therefore\) B is the correct Option.
Learn more about composition functions here,
brainly.com/question/30660139
brainly.com/question/30143914
Help please I really don’t understand this

Answers
Answer:
yea i agree with scorpiowo the asnwer is B
Step-by-step explanation:
If DAB = CBA,
DAB = 35° and CBA = 8x + 3
X= ?

Answers
Answer:
35= 8x + 3
35 - 3 = 8x
32 = 8x
32/8= 8x/8
x = 4
32=8x divid both sides by 8
X=4 degrees
A group of students in college of engineering studied the following subjects: 25% studied mathematics subject 20% studied electronics subject 55% studied Communications subject 10% studied both electronics and communications subjects 1- Draw Venn diagram 2- If a student is randomly selected what is the probability that he studied Communications or electronics or both subjects? 3- If a student is randomly selected what is the probability that he studied mathematics and Communications subjects?
Answers
Venn diagram:
The percentage of students that studied mathematics = 25%
The percentage of students that studied electronics = 20%
The percentage of students that studied Communications = 55%
The percentage of students that studied both electronics and Communications subjects = 10%
P(studied Communications or electronics or both subjects)
= P(studied Communications) + P(studied electronics) - P(studied both electronics and Communications subjects)
= 55% + 20% - 10%
= 65%
Therefore, the probability that a student studied Communications or electronics or both subjects is 65%.
P(studied mathematics and Communications subjects)
= P(studied mathematics) × P(studied Communications)
= 25% × 55%
= 13.75%
Therefore, the probability that a student studied mathematics and Communications subjects is 13.75%.
Drawing a Venn diagram, we have 25% studying Mathematics (M), 20% studying Electronics (E), and 55% studying Communications (C).10% studied both Electronics and Communications.
Therefore, the percentages become as follows: M = 25% - 10% = 15% E = 20% - 10% = 10% C = 55%.
Part 2 - To obtain the probability that a student studied Communications or Electronics or both subjects
P(Communication or Electronics) = P(Communication) + P(Electronics) - P(Communication and Electronics) = 55% + 20% - 10% = 65%.
The probability that a student studied Communications or Electronics or both subjects is 65%.
Part 3 -To obtain the probability that a student studied Mathematics and Communications subjects,
P(Mathematics and Communications) = P(Mathematics) * P(Communications) = 25% * 55% = 13.75%.
The probability that a student studied Mathematics and Communications subjects is 13.75%.
To know more about Venn diagram visit:
https://brainly.com/question/17041038
#SPJ11
Tasha has foam blocks stored in a box that measures 214 ft long by 2 ft wide by 2 ft tall.
Each foam block is a cube with 12ft edge length.
How many blocks can fit into the box?
Answers
any two planes intersect to form a_____
Answers
Answer:
line
Step-by-step explanation:
When two planes intersect, they form a line.
What are the coordinates of the center and the length of the radius of the circle
whose equation is-y-12y-20 25-07
a) center (0.5) and radius 7.5
c) center (0-6) and radius 75
b) center (0.12) and radius 45
d) center (-12) and radius 4,5
Answers
The equation of the circle, obtained by applying the completing the square method to the specified equation indicates that the center and radius of the circle are;
Center (0, 6) and radius 7.5
What is the general form of the equation of a circle?The general form of the equation of a circle is; (x - h)² + (y - k)² = r², where;
(h, k) = The coordinates of the center of the circle
r = The measure of the length of the radius
The possible equation obtained from a similar question on the internet can be presented as follows;
x² + y² - 12·y - 20.25 = 0
The above equation can be evaluated using the completing the square method and excluding the x² term as follows;
y² - 12·y - 20.25 = 0
y² - 12·y = 20.25
y² - 12·y + (-12/2)² = 20.25 + (-12/2)²
y² - 12·y + (-6)² = 20.25 + (-6)² = 56.25
(y - 6)² = 56.25
Therefore;
y - 6 = √(56.25) = ±7.5
The possible equation of the circle obtained by plugging in the x² term therefore is; (x - 0)² + (y - 6)² = 7.5²
The center of the circle is therefore;
(h, k) = (0, 6)
The radius of the circle, r = 7.5
The possible correction is therefore, option (a), where the 5 is a typing error
Learn more on the equation of a circle here: https://brainly.com/question/30307148
#SPJ1
4 Which statement about the expression -4/9 x - 3/8 is true? 9 A. The product is greater than 1. B. The product is less than both factors. C. The product is less than D. The product is a positive number.
Answers
Given data:
The given expression is -4/9 x=3/8
The given expression can be written as,
\(\begin{gathered} -\frac{4}{9}x=\frac{3}{8} \\ x=-\frac{27}{32} \end{gathered}\)The product is less than both the factors.
Thus, option C) is correct.
The digit 5 in which number represents a value of 0.5?
Answers
it's called 'tenths' and there's a thing that whatever goes on that side has no 'ones' it just starts with tens and it's ALWAYS followed by 'ths' after every digit
like hundredths or thousandths .
hope you understood it well
A school received 2 orders of new chairs. The first order contained 180 chairs. The second order contained 204 chairs. Each of the 17 classrooms received the same number of chairs. How many chairs will be put in storage as extra chairs?
A.
10
B.
17
C.
22
D.
44
Answers
Answer:its c
Step-by-step explanation:
HELPPP ASAP , Determine the slope of a line that is Parallel to the line that goes through the
points (9, 6) and (3, 10)

Answers
Answer:
slope = - \(\frac{2}{3}\)
Step-by-step explanation:
Parallel lines have equal slopes
Calculate the slope m using the slope formula
m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₁, y₁ ) = (9, 6) and (x₂, y₂ ) = (3, 10)
m = \(\frac{10-6}{3-9}\) = \(\frac{4}{-6}\) = - \(\frac{2}{3}\) ← slope of parallel line
Answer:
Step-by-step explanation:
Y2-Y1÷X2-X1=slope
10-6÷3-9=M
4÷-6=M
Slope =0.67
1. What is the circumference of the circle
below
(18 m
C 113 m
A 36 m
B 56.5 m
D 324 m
Answers
The formula for circumference is C = pi(d)
If the diameter was 18 all you would do is multiply 18x3.14 is would give you 56.5.
Hope it helped :)!
what is the aswer for −2x + 6x − 8 = 12
Answers
Answer:
x=5
Step-by-step explanation:
combine like terms: -2x + 6x = 4x
add eight to the other side 4x - 8 = 12 ----> 4x = 20
then divide 4x = 20 -----> x = 5
Answer:
x=5 or (5,0)
Step-by-step explanation:
7x - 5 = 2x - 15
5x + 2 = 4x - 1
3 - X = 4x + 13
16 - 2x = 5x + 9
3X + 5 = 2x + 7
Answers
Answer:
5x- 10
20x=5
3= 17x
25-10x
6x=12
Step-by-step explanation:
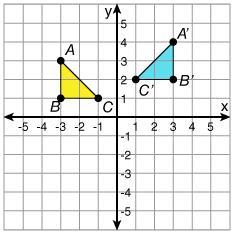
What transformations would result in the image shown?
Δ ABC is reflected over the x-axis and translated right 1 unit.
Δ ABC is reflected over the y-axis and translated up 1 unit.
Δ ABC is reflected over both axes.
Δ ABC is translated right 4 units and up 1 unit.
Answers
Simplify. (Integer Exponents)
\(\frac{a^4c^2e^0}{b^-^1d^-^3}\)
Answers
Explanation:
Firstly remove the “e” because e^0 is equal to 1 so it will remain the same
Move the b^-1 to the numerator and change the negative to a positive (just leave it as “b” in this case)
Move d^-3 to the numerator as well and change the -3 to positive 3 so you have a^4c^2bd^3
Finally reorder the b and c so it’s a^4bc^2d^3