jason bought a tv that costs 2,200 if the sales tax was 5.5 % how much did he pay for the tv
Answers
Answer: $2321
Step-by-step explanation:
Sales tax means that the total price is the original price, plus the tax.
The tax is 5.5%, so to get the tax we multiply the sales price by 5.5% and add that to the original price.
To turn a percent into a decimal that we can use on a calculator, simply move the percentage's decimal point two spaces to the left.
5.5 --> 0.55 --> 0.055.
Now multiply 2200 by 0.055
2200 * 0.055 = 121
2200 + 121 = 2321
Related Questions
How many solutions does 3 - 2x = 5-x+ 3 + 4x have?
Answers
Answer:
It should be one, right??
Step-by-step explanation:
Cause, if u follow the order of operations, you would follow the same steps. I’m not tryin to take points but don’t mark me brainliest.
Answer:
One solution
Step-by-step explanation:
If you put the two equations on a graphing calculator you would see that there are only 1 point that has intersected. For a more written answer, you would combine -x and 4x to get 3x and 5 and 3 to get 8.
From there on out, you would then have this equation 3 - 2x = 8 + 3x. Add 2x both sides to get 3 = 8 + 5x, then subtract 8 both sides to get -5 = 5x. Divide both sides to get x = -1. This is the only known solution that I know of.
How many liters of 15% acid and 33% acid should be mixed to make 40 liters of 21% acid solution?
Answers
Answer:
26 and 2/3 of 15% acid
13 and 1/3 of 33% acid
Step-by-step explanation:
Please let me know if you want me to add an explanation as to why this is the answer. I can definitely do that, I just wouldn’t want to write it if you don’t want me to :)
f(x) = -2x²+28x -99 in vertex form
Answers
The expression we have is:
\(f\mleft(x\mright)=-2x^2+28x-99\)Since this is a quadratic equation, is the equation of a parabola in standard form:
\(f(x)=ax^2+bx+c\)In this case, comparing the general form and the equation we have:
\(\begin{gathered} a=-2 \\ b=28 \\ c=-99 \end{gathered}\)We will define the vertex of the parabola as (h,k). And the general vertex form is:
\(f(x)=a(x-h)^2+k\)Where h is defined as follows:
\(h=\frac{-b}{2a}\)We already know b and a, so we substitute them to find h:
\(h=\frac{-28}{2(-2)}=\frac{-28}{-4}=7\)\(h=7\)Now we only need to find k, which is defined as the value of the function when x=h:
\(k=f(h)\)So we find f(h) by substituting h=7 into the original expression:
\(\begin{gathered} f(x)=-2x^2+28x-99 \\ k=f(h)=-2(7)^2+28(7)-99 \end{gathered}\)Solving the operations to find k:
\(\begin{gathered} k=-2(49)+196-99 \\ k=-98+196-99 \\ k=-1 \end{gathered}\)Now that we have h and k, we go back to the general vertex form:
\(f(x)=a(x-h)^2+k\)And substitute a=-2, h=7 and k=-1:
\(f(x)=-2(x-7)^2-1\)Answer:
\(f(x)=-2(x-7)^2-1\)What is the domain for the given function?
3 + 9x
4+5x
Answers
Answer:
12x plus 9x
Step-by-step explanation:
y = 7x-3
Rearrange the equation to make x the subject
Answers
Step-by-step explanation:
y = 7x - 3
To make x the subject, you need to isolate it. (Get x by itself)
y = 7x - 3
Add 3 on both sides...
y + 3 = 7x
Divide by 7 (Maybe your teacher wants 7x = y = 3 but if she doesn't and wants x completely by itself here's the answer)
x = y/7 + 3/6
When computing the degrees of freedom for ANOVA, how is the between-group estimate calculated?
a. (n - 1)/k
b. n - 1
c. k - 1
d. N - k
Answers
The option that follows is c .
The between-group estimate for calculating the degrees of freedom in ANOVA is calculated using the formula k - 1, where k represents the number of groups or treatments being compared.
This estimate represents the variability between the group means and is used in the F-ratio calculation to determine if the differences between the groups are significant.
When conducting ANOVA, the between-group estimate is an important factor in determining the degrees of freedom. This estimate represents the variability between the group means and is used to calculate the F-ratio, which determines if the differences between the groups are significant. The between-group estimate is calculated using the formula k - 1, where k represents the number of groups being compared. This formula is used because the estimate is based on the number of independent sources of variation between the groups. By accurately calculating the degrees of freedom, researchers can determine if the differences between the groups are statistically significant.
The between-group estimate for calculating the degrees of freedom in ANOVA is crucial for determining the statistical significance of differences between groups. It is calculated using the formula k - 1, where k represents the number of groups being compared. This estimate represents the variability between the group means and is used in the F-ratio calculation. Accurately calculating the degrees of freedom is essential in conducting valid ANOVA analyses.
To know more about F-ratio visit:
brainly.com/question/31827066
#SPJ11
To paint a wooden cube, Pinocchio used 4 gram of paint. When it is dry, he cut the cube into 8 equal cubes of smaller size. How much paint additional paint does Pinocchio need to paint the unpainted surfaces of smaller cubes?
Answers
Answer:
game1321.netlify.app
Step-by-step explanation:
This is a test sorry I'm trying to see if you can post links in comments
the citizens of a city were asked to choose their favorite type of pet. the circle graph shows how the citizens answered. if 130,000 citizens answered the question, how many chose cat?
Answers
Based on the information provided, the circle graph represents the preferences of the citizens for their favorite type of pet. we can calculate the number of citizens who chose cats by multiplying the total number of citizens (130,000) by that percentage. If the graph shows that 40% of citizens chose cats, then the calculation would be 130,000 * 0.40 = 52,000 citizens who chose cats as their favorite pets.
According to the circle graph, we can see that the blue section represents the percentage of citizens who chose cats as their favorite type of pet. To find out the actual number of citizens who chose cats, we need to use the information that 130,000 citizens answered the question.
First, we need to determine what percentage of citizens chose cats. From the graph, it looks like the blue section represents about 35% of the total.
To calculate this percentage as a decimal, we divide 35 by 100:
35/100 = 0.35
Now we can use this decimal to find out how many citizens chose cats:
0.35 x 130,000 = 45,500
Therefore, approximately 45,500 citizens chose cats as their favorite type of pet.
Learn more about Percentage:
brainly.com/question/28269290
#SPJ11
2
6
+
2
Area
HELP PLEASE

Answers
Answer:
X= 40
Step-by-step explanation:
if you compare the shapes, the X square is half of 6. so i added 6 and 3, then 2 and 3, then multiplied it, and the answer is 40.
2. (20 points) Assume the utility function of a representative consumer is U (X,Y)=min(X,Y). The consumer has $10 and Px =$1 and Py=$1. Find the optimal consumption bundle. Assume now price of X increases to $3. Fi,nd the new optimal consumption bundle. What is the total effect. Decompose the total effect into income effect and substitution effect. Draw a graph to accompany your answers and also quantify your answers.
Answers
The optimal consumption bundle with initial prices is (X,Y) = ($5,$5). The optimal consumption bundle changes when the price of good X increases.
The new optimal consumption bundle with the increased price of X is (X,Y) = ($3.33,$6.67).
The total effect is a decrease in the consumption of good X by approximately $1.67.
The income effect is zero, and the substitution effect is a decrease in the consumption of good X by approximately $1.67.
To find the optimal consumption bundle, we maximize the utility function subject to the budget constraint. The budget constraint is given by the equation Px*X + Py*Y = Income.
1. Initial prices:
Here, Px = $1, Py = $1, and the consumer has $10 as income. To find the optimal consumption bundle, we substitute the utility function into the budget constraint and differentiate it with respect to X. By setting the derivative equal to zero, we find the optimal consumption bundle:
Px = Py
1 = 1
X = Y
Substituting X = Y into the budget constraint:
1*X + 1*X = 10
2*X = 10
X = 5
Thus, the optimal consumption bundle with initial prices is (X,Y) = ($5,$5).
2. Increased price of X:
When the price of X increases to $3, we need to find the new optimal consumption bundle. By following the same steps as above, we differentiate the utility function with respect to X, taking into account the new price of X:
Px = Py
3 = 1
X = Y
Substituting X = Y into the budget constraint:
3*X + 1*X = 10
4*X = 10
X = 2.5
The new optimal consumption bundle is (X,Y) = ($2.5,$2.5).
To calculate the total effect, we compare the change in the consumption of good X between the initial and new optimal bundles. The total effect is the decrease in the consumption of good X, which is approximately $1.67.
To decompose the total effect into income and substitution effects, we need to hold the consumer's utility constant at the initial level. By adjusting the consumer's income, we find that the income effect is zero. Thus, the total effect can be attributed entirely to the substitution effect.
The optimal consumption bundle changes when the price of good X increases. The consumer reduces their consumption of good X and increases their consumption of good Y. The total effect is a decrease in the consumption of good X by approximately $1.67, which is solely attributed to the substitution effect.
To know more about prices follow the link:
https://brainly.com/question/29023044
#SPJ11
The three angles of an isosceles triangle are, 2x+2, X-12 and x-12. Find the
size of 2x+2
Answers
Explanation:
2x+2 + x-12 + x-12 = 180
4x -22 = 180
4x = 202
x = 50.5
Plug x = 50.5 in 2x + 2:
2(50.5) + 2 = 101 + 2 = 103 degree
two cards are drawn without replacement from a standard deck of 52 playing cards what is the probability of choosing a club and then without replacement a spade

Answers
occurringGiven a total of 52 playing cards, comprising of Club, Spade, Heart, and Spade.
\(\begin{gathered} n(\text{club) = 13} \\ n(\text{spade) =13} \\ n(\text{Heart) = 13} \\ n(Diamond)=\text{ 13} \\ \text{Total = 52} \end{gathered}\)Probability of an event is given as
\(Pr=\frac{Number\text{ of }desirable\text{ outcome}}{Number\text{ of total outcome}}\)Probability of choosing a club is evaluated as
\(\begin{gathered} Pr(\text{club) = }\frac{Number\text{ of club cards}}{Total\text{ number of playing cards}} \\ Pr(\text{club)}=\frac{13}{52}=\frac{1}{4} \\ \Rightarrow Pr(\text{club) = }\frac{1}{4} \end{gathered}\)Probability of choosing a spade, without replacement
\(\begin{gathered} Pr(\text{spade without replacement})\text{ = }\frac{Number\text{ of spade cards}}{Total\text{ number of playing cards - 1}} \\ =\frac{13}{51} \\ \Rightarrow Pr(\text{spade without replacement})=\frac{13}{51} \end{gathered}\)Thus, the probability of both events occuring (choosing a club, and then without replacement a spade) is given as
\(\begin{gathered} Pr(\text{club) }\times\text{ }Pr(\text{spade without replacement}) \\ =\frac{1}{4}\text{ }\times\text{ }\frac{13}{51} \\ =\frac{13}{204} \end{gathered}\)Hence, the probability of choosing a club, and then without replacement a spade is
\(\frac{13}{204}\)The number of pieces of cat food in a one-cup scoop is approximately Normally distributed with a mean of 344 pieces and a standard deviation of 16 pieces. If a random sample of 28 scoops of cat food is selected, what is the probability that the mean number of pieces will be more than 350 pieces? 0.0236 0.3538 0.6462 0.9764
Answers
Answer:
0.0236
Step-by-step explanation:
took the quiz
Place the numbers in the table to order them from least to greatest.
-14/3 -4 -3.95 -13/3 -4.25
Answers
Answer: i’m kinda late but -14/3 , -13/3 , 4.25 , -4 , -3.95
hope this helped! :)
Answer:
-14/3,-13/3,-4.25,-4,-3.95
Step-by-step explanation:
which constants can be multiplied by the equations so one variable will be eliminated when the systems are added together? 5x 13y
Answers
The constants that we have to multiply to eliminate one variable from the system are 12 and 5.
The equations are
5x+13y = 232
12x + 7y = 218
Here we can cancel both x term and y terms, lets choose x terms and apply the elimination method
The coefficient of x in first equation is 5 and 12 in second equation
We have to make it same
Prime factorization
5 = 5×1
12 = 2×2×3
LCM (5, 12 ) = 2×2×3×5 = 60
Multiply the first equation by 12 and second equation by 5
60x + 156y = 2784
60x + 35y = 1090
Subtract equation 2 from equation 1
121y = 1694
Here we have eliminated x term
Hence, the constants that we have to multiply to eliminate one variable from the system are 12 and 5.
The complete question is:
Which constants can be multiplied by the equations so one variable will be eliminated when the systems are added together? 5x + 13y = 232
12x + 7y = 218
Learn more about elimination method here
brainly.com/question/14619835
#SPJ4
to evaluate which of a set of curves fits the data best, we can use: a. APE b. MAPE c. R2 d. NPV
Answers
To evaluate which of a set of curves fits the data best, you can use the option "c. R2", also known as the coefficient of determination.
R2 is a statistical measure that helps determine the proportion of variance in the dependent variable explained by the independent variable(s) in the regression model. It ranges from 0 to 1, with higher values indicating a better fit of the curve to the data.
To evaluate which of a set of curves fits the data best, we can use the R2 (coefficient of determination) metric. R2 is a statistical measure that represents the proportion of the variance in the dependent variable that is explained by the independent variable(s) in a regression model.
A higher R2 value indicates a better fit of the curve to the data. APE (absolute percentage error), MAPE (mean absolute percentage error), and NPV (net present value) are not appropriate metrics for evaluating the fit of a curve to data. APE and MAPE are typically used to measure forecasting accuracy, while NPV is a financial metric used to determine the present value of future cash flows.
Learn more about coefficient of determination
brainly.com/question/28975079
#SPJ11
(Chapter 14) If f(x,y) has two local maximal, then f must have a local minimum.TrueFalse
Answers
It is true that the existence of two local maxima does not guarantee the presence of a local minimum. It is possible for a function to have multiple local maxima and no local minimum.
For example, consider the function f(x,y) = x^4 - 4x^2 + y^2. This function has two local maxima at (2,0) and (-2,0), but no local minimum. Therefore, the statement "if f(x,y) has two local maximal, then f must have a local minimum" is false. The presence or absence of local maxima and minima depends on the behavior of the function in the immediate vicinity of a point, and cannot be determined solely based on the number of local maxima. It is possible for a function to have an infinite number of local maxima and minima, or none at all. Therefore, it is important to carefully analyze the behavior of a function in order to determine the presence or absence of local extrema.
Know more about maxima here:
https://brainly.com/question/31387277
#SPJ11
Given a cylinder with the height of 10cm and the base radius of 9cm. Find the volume of this cylinder to the nearest tenth.
Answers
The volume of a cylinder is given by the formula
\(V=\pi r^2h\)where r is the radius of the base and h is the height of the cylinder.
Now, in our case r = 9 cm and h = 10 cm; thereofr
The walters backyard pool is rectangular in shape and similar to the rectangle formed by their fenced backyard. The pool measures 10 ft long and 8 ft wide .The length of the backyard is 35 ft long,what is the width of the backyard
Answers
Answer:
Step-by-step explanation:
55
Ted combines 7.28 ounces of strawberries with 8.06 ounces of blueberries to make fruit bowls. He pours the fruit equally into 4 bowls, and has 1.58 ounces of fruit left over. How many ounces of fruit are in each bowl?
Answers
Answer:
7.28 ounces + 8.06 ounces = 15.34 ounces combined
15.34 ounces - 1.58 ounces left over = 13.76 ounces
divide 13.76 ounces by 4 people = 3.44 ounces per bowl
Please give me the correct answer.Only answer if you're very good at math.
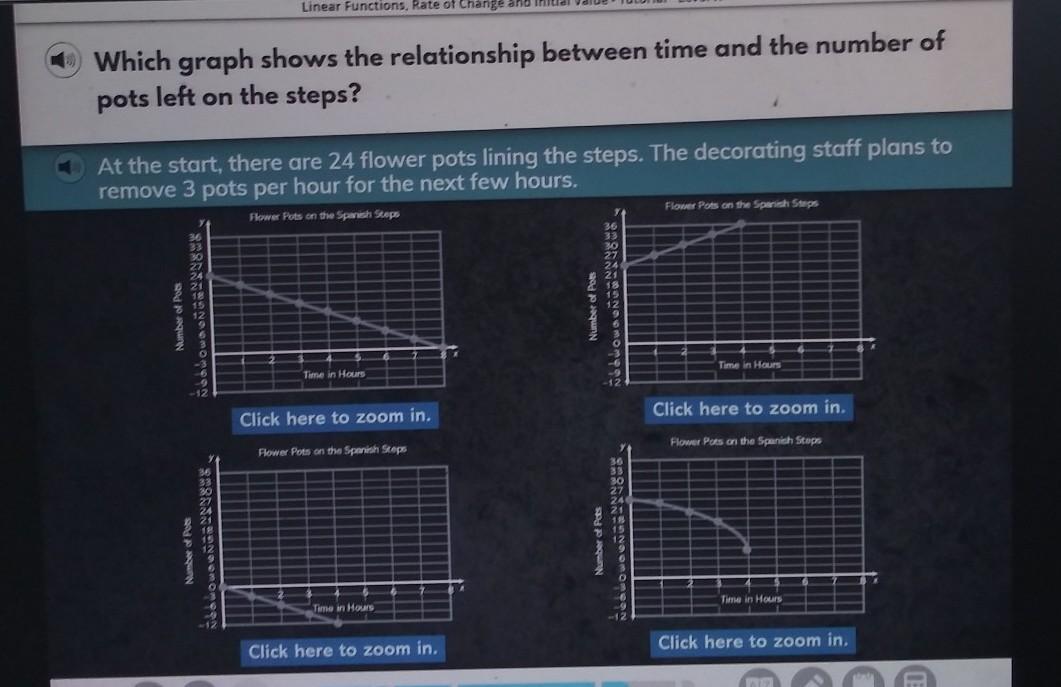
Answers
Answer:
1st graph
Step-by-step explanation:
this is the only graph that begins at 24, has a negative slope, is linear, and ends at (8,0) which, after 8 hours losing 3 pots per hour, makes sense
I can tell because at 0 hours, they started out with 24 plants, which means there should be a point at (0,24). I also know that free every hour, they remove 3 plants, so the line should be going down (as the number of pots keeps on getting lower).
I need help ASAP< I WILL GIVE BRAINLIEST TO THE FIRST ANSWER


Answers
Answer:
What do you want us to do
Step-by-step explanation:
.
If a car has tires with a diameter of 28 inches and is traveling at 55 mph, how fast are its tires spinning, in rpm^ prime s (revolutions per minute)? [There are 63,360 inches in 1 mile) Round your final answer to 2 decimal places .
Answers
Answer:
The tires are spinning at 660.49 revolutions per minute.
Step-by-step explanation:
The speed of the tires (v) is the same that the speed of the car, so to find the angular velocity of the tires we need to use the equation:
\( \omega = \frac{v}{r} \)
Where:
r: is the radius of the tires = d/2 = 28 inches/2 = 14 inches
\(\omega = \frac{55 mph}{14 inches*\frac{1 mile}{63360 inches}} = 2.49 \cdot 10^{5} \frac{rad}{h}*\frac{1 h}{60 min}*\frac{1 rev}{2\pi rad} = 660.49 rpm\)
Therefore, the tires are spinning at 660.49 revolutions per minute.
I hope it helps you!
The radius of a circle is 2 kilometers. What is the circle's area?
Use 3.14 for T.
Answers
Answer:
12.56 kilometers
Step-by-step explanation:
Formula for area of a circle A= πr^2
Insert the variables into the formula
A= (3.14) 2^2
A= (3.14) 4
A = 12.56
Due in 5 mins please help me!

Answers
Answer:
no
Step-by-step explanation:
solve this please show your work <3

Answers
Given:
The expression is
\(\sqrt[3]{48}=\sqrt[3]{8\cdot \_\_}=\)
To find:
The simplified form of the expression.
Solution:
We have,
\(\sqrt[3]{48}=\sqrt[3]{8\cdot \_\_}=\)
The expression \(\sqrt[3]{48}\) can be written as
\(\sqrt[3]{48}=\sqrt[3]{8\cdot 6}\)
\(\sqrt[3]{48}=\sqrt[3]{8}\cdot \sqrt[3]{6}\) \([\because \sqrt[3]{ab}=\sqrt[3]{a}\sqrt[3]{b}]\)
\(\sqrt[3]{48}=2\cdot \sqrt[3]{6}\)
\(\sqrt[3]{48}=2\sqrt[3]{6}\)
Therefore, \(\sqrt[3]{48}=\sqrt[3]{8\cdot 6}=2\sqrt[3]{6}\).
knlkn/l,kjn.kj.njkbjkb,.bgj,hjbhb b. ,.
Answers
Answer:
hmmmmmmmmmmmmmmmmm
Step-by-step explanation:
egfrggggggdfgd
djdgg
gfhdst
Select the correct answer. What is the fractional form of 0.8 ?
Answers
Answer:
4/5
Step-by-step explanation:
Answer: unless it is a recurring decimal the answer would be 8/10 as whenever a number is divided by a multiple of ten the point behind it moves to the left
\( 8 d \) transformation is be applied to Select one: a. disjoint b. overlap
Answers
Transformation doesn't depend on the shape of the figure if it has an overlap or not
The transformation \(8d\) can be applied to a figure with overlap or not with overlap.
Transformations are operations on a plane that change the position, shape, and size of geometric figures.
When a geometric figure is transformed,
its new image has the same shape as the original figure.
However,
it is in a new position and may have a different size.
Let's talk about different types of transformations.
Rotation:
It occurs when a shape is turned around a point, which is the rotation center.
Translation:
It moves the shape from one point to another on a plane.
Reflection:
It is an operation that results in the mirror image of the original shape.
Scaling:
The shape is transformed by changing the size without changing its orientation.
Transformation on \(8d\):
In the given problem, the transformation of \(8d\) can be applied to the figure with or without overlap.
This means that \(8d\) transformation doesn't depend on the shape of the figure if it has an overlap or not.
To Know more about rotation visit:
https://brainly.com/question/1571997
#SPJ11
Which of the following algebraic represents shows a dilation that is an enlargement ?
Answers
The algebraic representation that shows a dilation that is an enlargement is (5/2 x,5/2 y). (Option D)
A dilation is a type of transformation that changes the size of the shape or object. It refers to a process of changing an object’s size by decreasing or increasing its dimensions by a scaling factor. A dilation produces an image that has the same shape as the original image but is a different size.
A dilation that results in a larger image is called an enlargement while a dilation that generates a smaller image is called a reduction. A dilation is described using the scale factor and the center of the dilation (which is a fixed point in the plane).
For a scale factor > 1, the image is an enlargement; for a scale factor < 1 and > 0, the image is a reduction; and for a scale factor = 1, the figure and the image are congruent. Hence, for a point (x,y), algebraic representation that shows a dilation that is an enlargement is (5/2 x,5/2 y) as the scale factor is greater than 1. For the remaining options, the scale factor is between 0 and 1, hence they are reduction.
Note: The question is incomplete. The complete question probably is: Which of the following algebraic representation shows a dilation that is an enlargement? A) (1/3 x,1/3 y) B) (0.1x, 0.1y) C) (5/6 x,5/6 y) D) (5/2 x,5/2 y)
Learn more about Dilation:
https://brainly.com/question/29449871
#SPJ4