Is the line x=-3 parallel to the line y=1/3
Answers
Answer:
No, The line x = 3 is not parallel to the line y = 1/3
Step-by-step explanation:
Here's What the graph looks like on Demos graphing calculator.
High Hopes^^
Barry-

Related Questions
There were 23 girls and 21 boys trying out for the school's basketball team. If only 5 of them got called back, how many students didn't make the cut?
Answers
Step by step explanation:
23 + 21 = 44 total students
44-4= 39 students who didn’t make the cut
Durante una venta de liquidación, el Sr. Pablo, un profesor particular, compró un total de 12 libros de asesoría de Secundaria 4 y Secundaria 1 por S/82. El precio de un libro de asesoría de Secundaria 4 era de S/ 8 y el precio de un libro de asesoría de
Secundaria 1 era de S/ 6. Que el número de los libros de asesoría de Secundaria 1 comprados sea “k”.
a) Expresa el número de libros de asesoría de Secundaria 4 comprados en términos de “k”.
b) Forma una ecuación en “k' y resuélvela, calculando la cantidad de libros de cada tipo que compró el Sr.Pablo
Answers
Resolviendo un sistema de ecuaciones, vemos que se venden 6 libros de secundaria 4 y 6 libros de secundaria 1.
¿Como escribir un sistema de ecuaciones?
Primero definimos dos variables:
x = numero de libros de secundaria 4 vendidos.k = número de libros de secundaria 1 vendidos.Sabemos que se venden 12 libros en total, entonces:
x + k = 12
Tambien sabemos que se recauda un total de $82, entonces:
x*$8 + k*$6 = $82
Entonces tenemos dos ecuaciones.
a) Usando la primer ecuacion, podemos aislar x para tener:
x = 12 - k
b) Ahora podemos reemplazar eso en la otra ecuación para solucionar el sistema.
(12 - k)*$8 + k*$6 = $82
$96 - $2*x = $82
$2*x = $12
x = $12/$2 = 6
Es decir se venden 6 libros de secundaria 4, y como se venden 12 libros en total, los otros 6 libros vendidos son de secundaria 1.
Si quieres aprender más sobre sistemas de ecuaciones:
https://brainly.com/question/13729904
#SPJ1
A geologist gathered data about the total shoreline and maximum depth of several area lakes and organized the data into this table.
Total Shoreline (miles) 22 17 10 23 12 35 7
Maximum Depth (feet) 101 85 59 113 64 158 33
She then used a graphing tool to display the data in a scatter plot, with x representing the total miles of shoreline and y representing the maximum depth. She also used the graphing tool to find the equation of the line of best fit:
y = 4.26x + 10.908.
Based on the line of best fit, what is the approximate maximum depth of a lake that has 31 miles of shoreline?
Answers
Based on the line of best fit, the approximate maximum depth of a lake that has 31 miles of shoreline is; 142.968 ft
How to interpret a Line of best fit?The line of best fit is defined as a straight line which is drawn to pass through a set of plotted data points to give the best and most approximate relationship that exists between such data points.
Now, we are given a table of values that shows the total shoreline in miles which will be represented on the x-axis and then the maximum depth of several area lakes which will be represented on the y-axis.
However, when the geologist found the graph, she arrived at an equation of best fit as;
y = 4.26x + 10.908.
Thus, for 31 miles of shoreline, the approximate maximum depth is;
Approximate maximum depth = 4.26(31) + 10.908.
Approximate maximum depth = 142.968 ft
Read more about Line of best fit at; https://brainly.com/question/1441182
#SPJ1
Answer:
143 feet
Step-by-step explanation:
its technically 142 and change but edmentum rounds up
For which data set is a linear regression most reasonable?
a set of nine data pairs with a correlation coefficient r = –0.4
a set of five data pairs with a correlation coefficient r = 0.3
a set of four data pairs with a correlation coefficient r = –0.8
a set of six data pairs with a correlation coefficient r = 0.6
Answers
Answer:
A set of four data pairs with a correlation coefficient r = –0.8
Step-by-step explanation:
-0.8²=.64
It's the closest to 1. I also just took the quiz.
Answer:
c edge 2020
Step-by-step explanation:
By computing the first few derivatives and looking for a pattern, find 939 dx d939 d 939 (cos x)=
Answers
The value of 939 dx d939 d 939 (cos x) is cos x by computing first few derivatives and looking for a pattern.
To find 939 dx d939 d 939 (cos x), we need to compute the first few derivatives of cos x and look for a pattern.
The derivative is a key idea in calculus that gauges how quickly a function alters in relation to its input variable. In terms of geometry, the slope of the tangent line to the function graph at a particular location is represented by the derivative. The derivative has numerous crucial uses in mathematics, physics, engineering, and other disciplines, including optimisation, identifying extrema and inflection points, and simulating the rates of change of events that occur in the actual world. The derivative of various functions can be found using a variety of methods, including the power rule, product rule, chain rule, and quotient rule.
The first derivative of cos x is -sin x, the second derivative is -cos x, the third derivative is sin x, and the fourth derivative is cos x. We can notice that the pattern of the derivatives of cos x is that they cycle through the functions cos x, -sin x, -cos x, and sin x.
Since 939 is a multiple of 4 (939/4 = 234.75), we know that the 939th derivative of cos x will be the same as the fourth derivative of cos x, which is cos x.
Therefore, 939 dx d939 d 939 (cos x) = cos x.
Learn more about derivatives here:
https://brainly.com/question/31959163
#SPJ11
the radius of a sphere is increasing at a rate of 4 mm/s. how fast is the volume increasing (in mm3/s) when the diameter is 80 mm? (round your answer to two decimal places.)
Answers
Increase in volume is 802424.77 cubic mm/s when the diameter is 80 mm.
Let the radius of the sphere be R.
So, the volume of the sphere is given by,
V = \(\frac{4}{3}\pi r^3\)
Differentiating the above relation with respect to time 't' we get,
dV/dt = 4\(\pi r^2\). dr/dt
Given that, the change of radius (dr/dt) = 4 mm/s
When the diameter is 80 mm, then the radius = 80/2 = 40 mm
So the volume change when the diameter is 80 mm is given by,
dV/dt \(=4\pi\times40^2\times4\approx 802424.77\) cubic mm/s
Hence the volume increase in 802424.77 cubic mm/s.
To know more about Sphere refer to:
https://brainly.com/question/22807400
#SPJ4
What is 5 Left-bracket cosine (StartFraction pi Over 4 EndFraction) + I sine (StartFraction pi Over 4 EndFraction) right-bracket raised to the 3rd power?
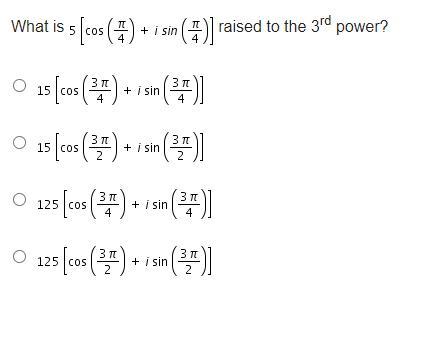
Answers
Answer:
C
Step-by-step explanation:
I took it on edg 2020
The Complex Alternative form to the expression (5[cos(π/4) + i sin(π/4)])^3 is given as: (Option C).
What is a complex Alternate form?A complex number of equations will take the form x + yi, where x and y are real numbers and i is an indeterminate that satisfies the rule i² = -1.
Hence, the solution to the above is given as follows:
Step 1 - Ludooph's number pi is given as:
π ≈ 3.14159265
Step 2 - Divide 3.14159265/4
= 0.78539816
Step 3
Cos (0.78539816)
= 0.70710678
It is assumed that the trigonometric angle argument is in radians.
Step 4
Divide: 3.14159265 / 4
= 0.78539816
Step 5 - find the Sin of the result of step 4
sin(0.78539816)
= 0.70710678
It is assumed that the trigonometric angle argument is in radians.
Step 6
Multiply i by the result of Step No 5
= i * 0.70710678
= 0.7071068i
Step 7 - Add the result of step no. 3 and the result of step number 6
= 0.70710678 + 0.7071068i
= 0.7071068+0.7071068i
Step 8 Multiply 5 by the result of step 7
= 5 * (0.7071068+0.7071068i)
= 5 * 0.70710678118655 + 5 * 0.70710678118655i
= 3.53553391+3.53553391i
= 3.53553391 +i(3.53553391)
= 3.5355339+3.5355339i
Step 9 - Exponentiate the results of Step 8 to the third power:
= (3.5355339+3.5355339i) ^ 3
= (5 × ei π/4)3 = 53 × ei 3 × π/4
= 125 × ei 3π/4
= -88.3883476+88.3883476i
If left in the Alternate Complex form, we have:
125[cos(3π/4) + i sin(3π/4)]
Learn more about Complex Forms at:
https://brainly.com/question/1466117?
#SPJ6
With reference to the various sampling methods, ________ is used when it is important to control where the sample is delivered and when the products are of a perishable nature.
Answers
Door to door sampling is used when it is important to control where the sample is delivered and when the products are of a perishable nature.
What is sampling?The term sampling selecting refers to the selection of a small proportion of the population extrapolating the results the results obtained from this small group to represent the characteristics of the entire population. This sample is chose in a manner as to reflect the properties the generality of the population.
Hence, door to door sampling is used when it is important to control where the sample is delivered and when the products are of a perishable nature.
Learn more about sampling:https://brainly.com/question/13287171
#SPJ4
find the area of the surface. the part of the plane 13x + 5y + z = 65 that lies in the first octant
Answers
the area of the surface that corresponds to the part of the plane 13x + 5y + z = 65 lying in the first octant is 32.5 square units.
To find the area of the surface that corresponds to the part of the plane 13x + 5y + z = 65 lying in the first octant, we need to find the equation of the portion of the plane that lies in the first octant and then calculate the surface area of that portion.
First, we need to find the intercepts of the plane with the x, y, and z-axes. Setting x = 0, we get:
5y + z = 65
Setting y = 0, we get:
13x + z = 65
Setting z = 0, we get:
13x + 5y = 65
Solving these three equations simultaneously, we get:
x = 5, y = 13, z = 0
So the plane intersects the x-axis at (5,0,0), the y-axis at (0,13,0), and the z-axis at (0,0,65).
The part of the plane that lies in the first octant is bounded by the x-axis, the y-axis, and the line connecting (5,0,0) and (0,13,0). This triangular region has a base of length 5 and a height of 13, so its area is (1/2)513 = 32.5.
To know more about area visit:
brainly.com/question/1631786
#SPJ11
Find the volume
of the figure below:

Answers
Step-by-step explanation:
Use Pythagorean theorem to find the base of the right triangle
221^2 = 195^2 + b^2
b = 104 km
triangle area = 1/2 base * height = 1/2 * 104 * 195 = 10140 km^2
Now multiply by the height to find volume
10140 km^2 * 15 km = 152100 km^3
4x^4 + 9x^3 - 3x^2 - 18x - 10
Answers
(x^2-2)(x+1)(4x+5)
if we allowed the number of edges between any two nodes to be more than two - i.e. there are 7 roads from city a to city b and 5 roads from city b back to city a and so on, like that for many of the other cities, then which of the two data structures we used for our graph problems, would be able to accurately store that graph information?
Answers
If we allow the number of edges between any two nodes to be more than two, we would need to use the adjacency matrix to accurately store that graph information.
The adjacency matrix is a matrix representation of a graph where the rows and columns represent the vertices and the values in the matrix represent the number of edges between two vertices.
In this case, we could have values greater than 1 in the matrix to represent multiple edges between two vertices.
On the other hand, the adjacency list data structure would not be able to accurately store this information, as it represents each vertex and its adjacent vertices in a linked list format.
It would be difficult to represent multiple edges between two vertices using this data structure.
To learn more about matrix refer below:
https://brainly.com/question/22799167
#SPJ11
Calculate the area of each circle.
grade V lvl
R-radius and D-diameter
1.R-8yd
2.D-18in
3.R-10yd
4.R-6in
5.R-4ft
6.D-10ft
7.R-1yd
8.D-14in
9.R-2yd
Answers
\({ \pmb{ \hookrightarrow}} \: \underline{\boxed{\pmb{\sf{Area_{(Circle)} \: = \: \pi \: {r}^{2} }}}} \: \pmb{\red{\bigstar}} \\ \)
_________________________________________________
1) Radius = 8 yd\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \pi \: {r}^{2} \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times {8}^{2} \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 8 \times 8 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 64 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{1408} {7} \: \: {yard}^{2} \: \\ \)
_________________________________________________
2) Diameter = 18 inch→ Radius = 18/2 = 9 inch
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \pi \: {r}^{2} \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times {9}^{2} \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 9 \times 9 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 81 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{1782} {7} \: \: {inch}^{2} \: \\ \)
_________________________________________________
3) Radius = 10 yd\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \pi \: {r}^{2} \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times {10}^{2} \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 10 \times 10 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 100 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{2200} {7} \: \: {yard}^{2} \: \\ \)
_________________________________________________
4) Radius = 6 inch\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \pi \: {r}^{2} \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times {6}^{2} \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 6 \times 6 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 36 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{792} {7} \: \: {inch}^{2} \: \\ \)
_________________________________________________
5) Radius = 4 ft\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \pi \: {r}^{2} \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times {4}^{2} \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 4 \times 4 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 16 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{352} {7} \: \: {ft}^{2} \: \\ \)
_________________________________________________
6) Diameter= 10 ft→ Radius = 10/2 = 5 ft
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \pi \: {r}^{2} \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times {5}^{2} \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 5 \times 5 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 25 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{550} {7} \: \: {ft}^{2} \: \\ \)
_________________________________________________
7) Radius = 1 yd\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \pi \: {r}^{2} \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times {1}^{2} \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 1 \times 1 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 1 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \: {yard}^{2} \: \\ \)
_________________________________________________
8) Diameter= 14 inch→ Radius = 14/2 = 7 inch
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \pi \: {r}^{2} \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times {7}^{2} \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 7 \times 7 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 49 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{1078} {7} \: \: \: \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: 154 \: \: {inch}^{2} \: \\ \)
_________________________________________________
9) Radius = 2 yd\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \pi \: {r}^{2} \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times {2}^{2} \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 2 \times 2 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{22} {7} \: \times 4 \\ \)
\(\longrightarrow \sf \: Area_{(Circle)} \: = \: \frac{88} {7} \: \: {yard}^{2} \: \\ \)
_________________________________________________
what is the length x of a side of the small inner square?
Answers
The length x of a side of the small inner square can be determined using the properties of similar triangles.
To find the length x, we can set up a proportion between the small inner square and the larger outer square.
Let's denote the side length of the small inner square as s and the side length of the larger outer square as S.
Since the small inner square is completely contained within the larger outer square, the ratio of their side lengths will be the same as the ratio of their corresponding sides.
Therefore, we can set up the following proportion:
s / S = x / (x + 10)
Here, the x + 10 represents the side length of the larger outer square, as it is 10 units longer than the side length of the small inner square.
To solve for x, we can cross-multiply the proportion:
s * (x + 10) = x * S
Expanding the equation:
sx + 10s = xS
Rearranging the equation to isolate x:
sx - xS = -10s
Factoring out the common term x:
x(s - S) = -10s
Dividing both sides by (s - S):
x = -10s / (s - S)
Now, we have an expression for x in terms of s and S.
It's important to note that the given information is insufficient to find the exact value of x without additional measurements or equations. The value of x will depend on the specific dimensions of the small inner square and the larger outer square.
to know more about small inner square here:
brainly.com/question/30517179
#SPJ11
An athlete throws a javelin, a long metal spear, at a track and field competition. The javelin's height in feet, h, as a function of the number of seconds since it was thrown, t, is modelled by the equation h (t)=-16t2 + 128t. What is the height of the javelin after 5 seconds? A) O feet B) 8 feet C) 240 feet D) 480 feet
Answers
Answer:
C) 240 feet
Step-by-step explanation:
't' represents seconds so we need to find:
h(5) = -16(5²) + 128(5)
h(5) = -16(25) + 640
h(5) = -400 + 640
h(5) = 240 feet
Check by Graphing
I need help!!! I am going to fail if I don't!!! I will mark you brainiest.
Room and board charges for on-campus students at the local college have increased 2.3% each year since 2000. In 2000, students paid $5,783 for room and board.
Write a function to model the cost C after t years since 2000.
If the trend continues, how much would a student expect to pay for room and board in 2017? Express your answer as a decimal rounded to the nearest hundredth.
Answers
Answer:
C = 5785.39
Step-by-step explanation:
C = 5,785 + .023t
.023 * 17 = .391
.391+ 5785 = 5785.391
hope this helps
a slope
5 and passes through the point (3, -7)
Answers
Answer:
y = 5x - 22 is the answery - intercept is -22.Step-by-step explanation:
Given:Slope: 5
Point: (3, -7)
Unknown:Equation = ? = y = mx + b
y - intercept = ?
Step 1. Input the coordinates into the equation of y = mx + b.
y = 5x + b
y-coordinate = -7
x-coordinate = 3
-7 = 5(3)+b
Step 2. Solve for b.
-7 = 15 + b
-15 -15
b = -33
Step 3. Make the y equation.
y = mx + b
mx = 5x
b = -22
y = 5x - 22
y = 5x - 22 is the answery - intercept is -22.Hope it helped,
Kavitha Banarjee
Find the area of the triangle. 7 in. 18 in. The area is square inches.
Answers
(7 x 18) / 2 = 63
If the table below represents a proportional relationship, what number will go in the empty box?

Answers
Answer:
The number will be 15
Hope you have a great day!
Answer:
15 is the correct answer
Lines r and a are intersected by a transversal. Which of the follow, could always be used to prove m and n are parallel?
Answers
The statement that could be used to prove that lines r and s are parallel is given as follows:
m < 8 = m < 6.
What are corresponding angles?When two parallel lines are cut by a transversal line, the corresponding angles are the angles that are in the equivalent position for each parallel line.
If the lines are in fact parallel, then the two angles are congruent.
From the image given at the end of the answer, the corresponding angles for this problem are given as follows:
Angle 8 and angle 6.
Hence the statement that proves by contradiction that the two lines are parallel is given as follows:
m < 8 = m < 6.
Meaning that the correct option is given by the third option.
Missing InformationThe problem is given by the image presented at the end of the answer.
More can be learned about corresponding angles at https://brainly.com/question/24607467
#SPJ1

What is the y-intercept of 2x y =- 3?
Answers
The y-intercept of the equation 2x - y = -3 is 3 and in ordered pair form is (0,3).
What is the y-intercept of the given equation?The slope-intercept formula is expressed as;
y = mx + b
Where m is slope and b is y-intercept.
Given the equation in the question
2x - y = -3
First, we solve form for y and reorder in slope intercept form.
2x - y = -3
Subtract 2x from both sides
2x - 2x- y = -3 - 2x
- y = -3 - 2x
Divide each term by -1
y = 3 + 2x
Reorder in slope intercept form
y = 2x + 3
To find the y-intercept, replace x with zero and solve for y
y = 2(0) + 3
y = 3
Therefore, the y-intercept is 3.
Learn more about equation of line here: brainly.com/question/2564656
#SPJ1
Diagnostic
Analytics
When completing an online shopping transaction, a typical shopper takes 7 seconds to
select each product and another 9 seconds to complete the check-out process. If it takes 37
seconds to complete a transaction, how many products are being purchased?
products
Submit
Answers
Answer:
In 26 seconds to complete a transaction, 2 products are being purchased.
Step-by-step explanation:
1 item = 9 seconds
Time taken in all to check out = 8 seconds
Time taken to shop = 26 seconds
Now as check out process takes 8 seconds, so the
Time left to ACTUALLY SHOP = Total Time - Time Used to check out
= 26 seconds = 8 seconds = 18 seconds
Shopping of 1 item = 8 seconds
Shopping of 2 items = 2 x ( Time taken in 1 item) = 2 x 9 = 18 seconds
So, in 18 seconds, 2 clothing item can be selected.
Hence,in 26 seconds to complete a transaction, 2 products are being purchased.
The area of a rectangle is 195 dm². The width is two less than the length. What is the length and the width of tge rectangle?
Answers
The length of the rectangle is 15 dm and the width is 13 dm.
Let's assume that the length of the rectangle is "L" and the width is "W".
From the problem statement, we have two pieces of information:
The area of the rectangle is 195 dm²:
Area = Length x Width
195 dm² = L x W
The width is two less than the length:
W = L - 2
Now, we can substitute the second equation into the first equation to eliminate W and get an equation with only one variable:
195 dm² = L x (L - 2)
Simplifying the equation:
195 dm² = L² - 2L
L² - 2L - 195 dm² = 0
To solve for L, we can use the quadratic formula:
L = (-b ± √(b² - 4ac)) / 2a
Where a = 1, b = -2, and c = -195.
L = (2 ± √(2² + 4 x 1 x 195)) / 2 x 1
L = (2 ± √4 + 780) / 2
L = (2 ± √784) / 2
L = (2 ± 28) / 2
L = 15 or L = -13
Since the length can't be negative, the length of the rectangle is L = 15 dm.
Now we can use the equation W = L - 2 to find the width:
W = 15 dm - 2 dm
W = 13 dm
Therefore, the length of the rectangle is 15 dm and the width is 13 dm.
To know more about width click here:
brainly.com/question/30173060
#SPJ4
in how many ways can a committee of three men and four women be formed from a group of 8 men and 8 women?
Answers
There are 3,920 ways to form such a committee from a group of 8 men and 8 women.
To form a committee of 3 men and 4 women, we need to select 3 men from a group of 8 men and 4 women from a group of 8 women.
The number of ways to select 3 men from a group of 8 men is:
8 choose 3 = (8!)/(3!5!) = 56
Similarly, the number of ways to select 4 women from a group of 8 women is:
8 choose 4 = (8!)/(4!4!) = 70
Therefore, the total number of ways to form a committee of 3 men and 4 women is:
56 \(\times\) 70 = 3,920
So, there are 3,920 ways to form such a committee from a group of 8 men and 8 women.
To learn more about number of ways visit: https://brainly.com/question/29110744
#SPJ11
Gasoline is pouring into a vertical cylindrical tank of radius 55 feet. When the depth of the gasoline is 66 feet, the depth is increasing at 0.30.3 ft/sec. How fast is the volume of gasoline changing at that instant
Answers
The volume of gasoline in the cylindrical tank is increasing at 23.56 ft.³/sec when the depth of the gasoline in the tank is 6 feet. Computed using differentiation.
Since the tank is cylindrical in shape, its volume can be written as:
V = πr²d,
where V is its volume, r is the radius, and d is the depth.
The radius is constant, given r = 5ft.
Thus the volume can be shown as:
V = π(5)²d,
or, V = 25πd.
Differentiating this with respect to time, we get:
δV/δt = 25πδd/δt ... (i),
where δV/δt, represents the rate of change of volume with respect to time, and δd/δt represents the rate of change of depth with respect to time.
Now, we are given that when the depth increases at 0.3 ft./sec when the depth of the gasoline is 6 feet.
Thus, we can take δd/δt = 0.3 ft./sec, in (i) to get:
δV/δt = 25πδd/δt = 25π(0.3) ft.³/sec = 23.56 ft.³/sec.
Thus, the volume of gasoline in the cylindrical tank is increasing at 23.56 ft.³/sec when the depth of the gasoline in the tank is 6 feet. Computed using differentiation.
The question written correctly is:
"Gasoline is pouring into a vertical cylindrical tank of radius 5 feet. When the depth of the gasoline is 6 feet, the depth is increasing at 0.3 ft./sec. How fast is the volume of gasoline changing at that instant?"
Learn more about differentiation at
https://brainly.com/question/15006940
#SPJ4
Answer ASAP please, Will give brainliest.

Answers
Answer:
see below
Step-by-step explanation:
The measure of a minor arc is the same as the angle that forms it.
1. Since ∠GBJ = 90°, the answer is 90°.
2. ∠HBI = 180° - 151° = 29° so the answer is 29°.
3. ∠HBJ = 180° so the answer is 180°.
4. The reflex angle ∠GBI = 90 + 151 = 241° so the answer is 241°/
5. Since ∠GBJ = 90°, the reflex angle ∠GBJ = 360 - 90 = 270° so the answer is 270°.
6. ∠GBH = 180 - 90 = 90° so the reflex angle ∠GBH = 360 - 90 = 270° so the answer is 270°.
lines that intersect and form four right angles are called?
Answers
Answer:
Perpendicular
Step-by-step explanation:
A sports club had 130 members last summer. This summer, due to some renovations, it has 182 members. What is the percent of increase in the number of members?HELP PLSSSSS
Answers
Answer:
Step-by-step explanation:
312
Answer:
36.92%
Step-by-step explanation:
180-132=48. Now we have to find 48 of 130 to find the percentage increase. 48 out of 130 = which equals 36.92.
PLEASE HELP ME I NEED THIS DONE TODAY!!!
Taylor swam 3 different heats in the 100m freestyle. The first heat was 29.1 seconds,the second heat was 28.7 seconds, and the third heat was 27.2 seconds. What was the difference between her best time and her worst time?
Answers
Answer:
16.5 you have to add the divde
What is half of a 3/4 cup?
Answers
Answer:0.375
Step-by-step explanation:
3/4=0.75
0.75/2=0.375
(3/4)/2=0.375