IQ scores are collected from a sample of 36 students
at a large university. The scores are summarized in
the histogram below.
Which of the following statements is true of the mean
and median?
O The mean is equal to the median IQ.
O The mean is less than the median IQ
O The mean is greater than the median IQ.
O It cannot be determined because we cannot tell the
exact values of the IQ scores in the sample.

Answers
Answer: The mean is less than the median IQ
Step-by-step explanation:
When a distribution is skewed left (there is an obvious tail going in the left direction, which is true in this case), then the mean is drawn down because it is not resistant to any outliers, or unusual data points.
The median, however, is resistant to outliers and will not move as much.
Therefore, the mean will be less than the median.
The relationship between the mean and median of a data set is determined by whether the data is symmetrically distributed, positively skewed, or negatively skewed. However, without a visual representation or specific values, we cannot definitively compare the mean and median of the IQ scores.
Explanation:In order to determine the relationship between the mean and the median of a data set, we need to know the nature of its distribution. If the data is symmetrically distributed, the mean and the median will be equal. If the data is positively skewed (has a long right tail), the mean will be larger than the median. If the data is negatively skewed (has a long left tail), the mean will be less than the median.
However, in the absence of the actual histogram in your question, the correct answer would be 'It cannot be determined because we cannot tell the exact values of the IQ scores in the sample.' This is because without a visual representation or specific values, we cannot determine the distribution's skewness, and by extension, cannot compare the mean and median.
Learn more about Mean and Median here:https://brainly.com/question/13006297
#SPJ2
Related Questions
A toy box is 5 feet long, 24 inches wide and 30 inches high. What is the volume of the toy box in cubic feet?
Answers
Answer:
25 cubic feet
Step-by-step explanation:
You need to convert all units to feet and then multiply the dimensions.
24 inches is 2 feet
30 inches is 2.5 feet
So, you would multiply 5X2X2.5= 25.
Write the slope-intercept equation of a line that passes through the points (-2, 5) and (6, -4)
Answers
The slope intercept form of a line which passes through the points (-2, 5) and (6, -4) is y = (-9/8)x + 11/4.
According to the question.
A line passes through the two points (-2, 5) and (6, -4).
As we know that, the slope intercept formula y = mx + b is used when you know the slope of the line to be examined and the point given is also the y intercept (0, b). In the formula, b represents the y value of the y intercept point.
So, the slope of the line = -4 -5/(6 + 2) = -9/8
And, the slope intercept form of a line which passes through the points (-2, 5) and (6, -4) is given by
y = (-9/8)x + b
Since, the line passes through (-2, 5) and (6, -4). So, both the points must statisfy the above equation.
⇒ 5 = (-9/8)(-2) + b
⇒ 5 = 9/4 + b
⇒ b = 5 - 9/4
⇒ b = (20 - 9)/4
⇒ b = 11/4
Therefore, the slope intercept form of a line which passes through the points (-2, 5) and (6, -4) is y = (-9/8)x + 11/4.
Find out more information about slope intercept form of a line here:
https://brainly.com/question/9682526
#SPJ4
Ut = 4uxx, 0 < x < 2,t > 0 u(0,t) = 1, u(2,t) = 2, u(x,0) = sin(17x) — 4 sin(Tt x/2) u = =
Answers
The solution of the given equation is\(u(x,t) = ∑(-1)n+1 4/(nπ) sin(nπ/4) sin(nπx / 2) exp(-n^2 π^2 t / 4)\)
The given equation is Ut = 4uxx, 0 < x < 2,t > 0u(0,t) = 1, u(2,t) = 2, u(x,0) = sin(17x) — 4 sin(Tt x/2)
The general form of the solution is given as:
\(u(x,t) = B0 + B1 x + ∑[Bn cos(nπx / L) + Cn sin(nπx / L)] exp(-n^2 π^2 t / L^2)\)
Where,\(Bn = (2/L) ∫f(x) cos(nπx / L) dx; from x = 0 to L . . . . . (1)\)
\(Cn = (2/L) ∫f(x) sin(nπx / L) dx; from x = 0 to L . . . . . (2)\)
\(L = 2Bn\)
First we need to find the values of B0 and B1.
Given initial conditions are\(u(x,0) = sin(17x) — 4 sin(Tt x/2)\)
We can write \(u(x,0) = B0 + B1 x + ∑[Bn cos(nπx / L) + Cn sin(nπx / L)]\)
From the given function, comparing the coefficients of the Fourier series, we have
\(B0 = 0, B1 = 0, Bn = (2/L) ∫f(x) cos(nπx / L) dx; from x = 0 to L = 0; for n = 1, 2, 3, .......\)
\(Cn = (2/L) ∫f(x) sin(nπx / L) dx; from x = 0 to L = (-1)n+1 4/(nπ)sin(nπ/4); for n = 1, 2, 3, .......L = 2.\)
Using the values of Bn and Cn, we can write the solution as \(u(x,t) = ∑(-1)n+1 4/(nπ) sin(nπ/4) sin(nπx / 2) exp(-n^2 π^2 t / 4)\)
Therefore, the solution of the given equation is\(u(x,t) = ∑(-1)n+1 4/(nπ) sin(nπ/4) sin(nπx / 2) exp(-n^2 π^2 t / 4)\)
Know more about equations here:
https://brainly.com/question/17145398
#SPJ11
Abi has 20 cents. Se bought x pencils for 3 cents each. If y equals the number of cents left, write an equation that shows the dependence of y on x. What is the domain of the function?
Answers
Answer:
The function would be:
y = 20 - 3xSince y should be positive or zero:
20 - 3x > 03x < 20x < 7So x can get values from 0 to 6.
The domain is:
x∈N, x = [0, 6]Answer:
function= y=20-3x
Domain= 1,2,3,4,5,6
Try It
Given: AD
Prove: DE
D
BC and BCD =
CE
Hint
B
Angles Segments Triangles Statements Reasons
AAS
CPCTC
Statements
✓ 1. AD = BC
✓2. ZBCD =
3. DC DC
4. AADC = ABCD
5. LEDC ZECD
SAS
converse of isosceles triangle thm
Reasons
1. given
2. given
3. reflexive property
4. SAS
5. CPCTC

Answers
The required statements and reasons to prove that DE is equal to CE is explained.
What is a triangle congruence theorem?The triangle congruence theorem is a theorem that can be used to prove that two or more triangles are the same, considering the corresponding properties of the triangles. The properties are length of the sides, and measure of internal angles.
The statements and reasons to prove that DE is equal to CE are explained below using the triangle congruence theorem.
STATEMENT REASON
1. AD = BC Given
2. <BCD = <ADC Given
3. DC = DC Reflexive property
4. ΔADC ≅ ΔBCD SAS
5. <EDC ≅ <ECD CPCTC
6. AC = BD Definition of diagonal
7. DE = CE Congruent sides of isosceles triangle
Learn more about the triangle congruence theorem at https://brainly.com/question/18922904
#SPJ1
Pleaseeeee HELP PlEASEEEeeEeEeE

Answers
5
Explanation:
Answer:
5.75
Step-by-step explanation:
a^2 + b^2 = c^2
4^2 + b^2 = 7^2
16 + b^2 = 49
49 - 16 = 33
√33 = 5.75
I NEED HELP BUT UTS NOT LIKE HELPPPPPP PLS SO YEAH

Answers
Answer: the answer is B
Step-by-step explanation:
Answer:
B. 16.2
Step-by-step explanation:
The angle is equal to a right angle so
90 - 73.8 = 16.2
x = 16.2
ayooooooooooo help me pls
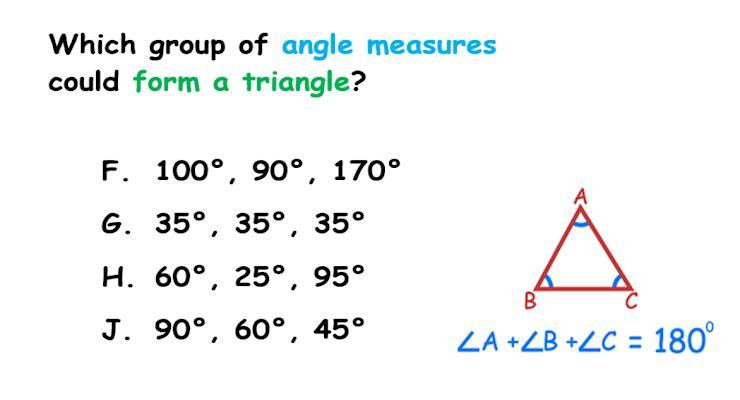
Answers
Answer:
The answer is H. I think :)
Please help me thank you

Answers
Answer:
For every one pound there are 16 ounces.
Step-by-step explanation:
We can simply see that by dividing the ounces by pounds, 32/2 = 16, and 48/3 = 16.
Number of ounces: 16, 32, 48, 64, 80, 96
Number of pounds: 1, 2, 3, 4, 5, 6
Assuming that the population is normally distributed, construct a 95% confidence interval for the population mean, based on the following sample size of n=8.
1,2,3,4,5,6,7 and 25
Change the number 25 to 8 and recalculate the confidence interval. Using these results, describe the effect of an outlier ( that is, an extreme value) on the confidence interval.
Find a 95% confidence interval for the population mean.
Answers
The 95% confidence interval for the population mean, based on a sample size of n=8 with the outlier 25 included, is [1.53, 8.47]. When the outlier is replaced with 8, the confidence interval becomes [2.04, 6.96]. The presence of the outlier significantly affects the width of the confidence interval, causing it to be wider and less precise.
A confidence interval is a range of values that is likely to contain the true population mean with a certain level of confidence. In this case, we are constructing a 95% confidence interval, which means that there is a 95% probability that the true population mean falls within the interval.
The formula for calculating the confidence interval for the population mean, assuming a normal distribution, is:
\(CI = x^-\)±\(t * (s / \sqrt{n})\)
Where:
CI represents the confidence interval
\(x^-\) is the sample mean
t is the critical value from the t-distribution table based on the desired confidence level and degrees of freedom
s is the sample standard deviation
n is the sample size
In the given scenario, the initial sample contains the outlier 25, resulting in a wider confidence interval. When the outlier is replaced with 8, the confidence interval becomes narrower.
The presence of an outlier can have a significant impact on the confidence interval. Outliers are extreme values that are far away from the rest of the data. In this case, the outlier value of 25 is much larger than the other observations. Including this outlier in the calculation increases the sample standard deviation, which leads to a wider confidence interval. Conversely, when the outlier is replaced with a value closer to the rest of the data (8), the standard deviation decreases, resulting in a narrower confidence interval.
In conclusion, outliers can distort the estimate of the population mean and increase the uncertainty in the estimate. They can cause the confidence interval to be wider and less precise, as observed in the comparison of the two confidence intervals calculated with and without the outlier.
Learn more about confidence intervals here:
https://brainly.com/question/31753211
#SPJ4
HELP QUICK!!!
The height of a triangle is 5 cm more than the base. If the area of the triangle 102 cm squared find
the height and length of the base
Height 17 Base 12
Height 18 Base 11
Height 12 Base 7
Height 16 Base 11
Answers
a tour bus normally leaves for its destination at 5 : 00 p.m. for a 350 mile trip. this week however, the bus leaves at 6 : 10 p.m. to arrive on time, the driver drives 10 miles per hour faster than usual. what is the bus` usual speed? the bus' usual speed is miles an hour.
Answers
The usual speed of the bus is 60 km/hr.
Define speed.Speed is the rate of change in location of an item, expressed in meters per second.
Given,
Let x be the usual speed.
Speed = Distance/ Time
Time = Distance/Speed
Bus usually travels 350 miles.
It takes time for it to get there = 350/x h
The bus departs this week at 6:10, though.
The 70-minute bus delay = 70/60 = 7/6 h
The bus's current speed is (x+10) miles per hour.
The new window of time for getting there is \(\frac{350}{x+10}\)
\(\frac{350}{x}\) + \(\frac{350}{x+10}\) = \(\frac{7}{6}\)
350(\(\frac{x+10-x}{x(x+10)}\) ) = \(\frac{7}{6}\)
350 ( \(\frac{10}{x^{2} +10x}\) )= \(\frac{7}{6}\)
7(x² +10x) = 6(350)(10)
x² + 10x = 3000
x² + 10x - 3000 = 0
x² -60x + 50x - 3000 = 0
x( x - 60) + 50(x - 60) = 0
(x + 50)(x - 60) = 0
x = -50 , x = 60
The usual speed of the bus is 60 km/hr.
To learn more about speed, visit:
https://brainly.com/question/15203844
#SPJ4
what set does the number 12 belong
Answers
Answer:
12 is a rational number because it can be expressed as the quotient of two integers: 12 ÷ 1.
12 belongs to the sets of natural numbers, integer numbers, rational numbers, and real numbers.
To what set does the number 12 belong?First, we can see that it is a whole number, so it is an integer number.
Also, all positive integers are natural numbers, so 12 is also a whole number.
Now, we also can rewrite 12 as:
12 = 12/1
So it is a quotient between two integer numbers, thus, 12 is also a rational number.
Finally, the trivial answer, 12 belongs to the set of the real numbers (the set that contains all the numbers).
Concluding:
12 belongs to the sets of whole numbers, integer numbers, rational numbers, and real numbers.
If you want to learn more about sets of numbers:
https://brainly.com/question/20118982
#SPJ1
6+2 (x+4) = 1/2 (3-x)

Answers
Answer:
x² - 8x + 12
Step-by-step explanation:
(x - 2) (x - 6)
x² -6x -2x + 12
x² - 8x + 12
The picture shows the equation like that, so I solve it that way!
Answer:
\(\tt x ^2-8x+12\)Step-by-step explanation:
\(\tt (x-2)(x-6)\)
Use the FOIL method:-
→ \(\boxed{\bf (a+b)(c+d)=ac+ad+bc+bd}\)
\(\tt x^2-6x-2x+12\)
Combine like terms:-
\(\tt x^2+(-6x-2x)+12\)
Simplify:-
\(\tt x ^2-8x+12\)
______________________
Hope this helps! :)
Which expression is equivalent to 7 k2, where k is an even number?
Answers
An equivalent expression to \(7k^2\), where k is an even number, is \(28n^2\), where n is an integer.
If k is an even number, then we can write k as 2n, where n is some integer. Substituting this into \(7k^2,\) we get:
\(7(2n)^2= 7(4n^2)\)
\(= 28n^2\)
Therefore, an equivalent expression to \(7k^2\), where k is an even number, is \(28n^2\), where n is an integer.
An integer is the number zero, a positive natural number or a negative integer with a minus sign. The negative numbers are the additive inverses of the corresponding positive numbers. In the language of mathematics, the set of integers is often denoted by the boldface Z.
Integers come in three types:
Zero (0)
Positive Integers (Natural numbers)
Negative Integers (Additive inverse of Natural Numbers)
To know more about integers visit:
https://brainly.com/question/15276410
#SPJ4
PLZZ HELPP IM FAILLING
What number could be a common denominator for these three fractions?
1/2 and 1/3 and 1/4
Answers
Answer:
12 would be a common denominator
Step-by-step explanation:
2 4 6 8 10 (12)
3 6 9 (12)
4 8 (12)
Container A and container B are being filled. Container A has 800 ml of water and is increasing by 6 ml per minute. Container B has 1000 ml and is increasing by 10 ml per minute. Write an equation using, m, to model how many minutes it will take for the two containers to have the same amount of water
Answers
The equation which shows the number of minutes it will take for the two containers to have same amount of water is 200+4t=0.
Given that container A has 800 ml and water is increasing by 6 ml per minute, container B has 1000 ml and water is increasing by 10 ml per minute.
We are required to find the equation that shows the minutes it will take for the two containers to have the same amount of water.
Water in container A=800 ml
Rate of increase of water in container A=6 ml per minute
Water in container B=1000 ml
Rate of increase of water in container B=10 ml per minute
According to the question the amount of water in both the containers is same.
let the number of minutes be t.
So,the equation will be:
800+6t=1000+10t
1000-800+10t-6t=0
200+4t=0
Hence the equation which shows the number of minutes it will take for the two containers to have same amount of water is 200+4t=0.
Learn more about equations at https://brainly.com/question/2972832
#SPJ9
The total length of these planks is 92 metres. Work out the number of planks of length 2 metres in Ben workshop.
Answers
Answer: 13
Step-by-step explanation:
How would three billion, nine hundred seventy-six thousand, twelve be written in standard form?
Answers
Step-by-step explanation:
3.000976×10⁹ would be how I will write in standard form
WILL GIVE BRAINLIEST!!
Which statement describes the transformation of M to circle N?
A. The circle was translated down 5 units and dilated by a factor of 1/2
B.The circle was translated down in 1 unit and dilated by a factor of 2
C. The circle was translated down 5 units in dilated by factor of 1/3
D. The circle was translated down in 1 unit and dilated by a factor of 3

Answers
Answer:
C. The circle was translated down 5 units in dilated by factor of 1/3
Step-by-step explanation:
The circle was translated down 5 units in dilated by factor of 1/3
Answer:
C
Step-by-step explanation:
The sum of two numbers is -18, if the first number is 10, which equation represents the situation and what is the second number .
Answers
Answer:
second number = - 28
Step-by-step explanation:
let the first number be x and the second number y , then
x + y = - 18 , that is
10 + y = - 18 ( subtract 10 from both sides )
y = - 28 , that is
the second number is - 28
Answer:
Step-by-step explanation:
The equation that represents the situation is -28 + 10 = -18
The second number is -28.
Using the same facts as #16, how long would it take to pay off 60% of the a. About 45 months b. About 50 months c. About 55 months d. About 37 months
Answers
To calculate how long it would take to pay off 60% of the debt,
we can use the same facts as in problem #16. Let's go through the steps:
1. Determine the total amount of debt: Find the original debt amount given in problem #16.
2. Calculate 60% of the debt: Multiply the total debt by 0.6 to find the amount that represents 60% of the debt.
3. Divide the amount obtained in step 2 by the monthly payment: This will give us the number of months it will take to pay off 60% of the debt.
Now, let's apply these steps to the options provided:
a. About 45 months: To determine if this is the correct answer, we need to perform the calculations outlined above using the original debt amount and the monthly payment given in problem #16.
b. About 50 months: Same as option a, perform the calculations using the original debt amount and the monthly payment.
c. About 55 months: Perform the calculations outlined above using the original debt amount and the monthly payment.
d. About 37 months: Perform the calculations outlined above using the original debt amount and the monthly payment.
After performing the calculations for each option, compare the results with the options provided to find the correct answer.
To Learn more about amount of debt:
https://brainly.com/question/28929846
#SPJ11
Look at the picture and tell me

Answers
Poppy's sister used approximately 3.14 inches more wire per wall hanging than Poppy.
How to find the amount of wire used ?To find the amount of wire used by both Poppy and her sister, we'll calculate the circumference of their respective wall hangings using the formula:
C = 2πr
Since the diameter is twice the radius, we can find her sister's radius as follows:
Poppy's radius = 6.25 inches
Poppy's diameter = 2 * 6.25 = 12.5 inches
Sister's diameter = Poppy's diameter + 1 = 12.5 + 1 = 13.5 inches
Sister's radius = 13.5 / 2 = 6.75 inches
Now, we'll calculate the circumferences using the given value for π:
Poppy's circumference = 2 x 3.14 x 6.25 ≈ 39.25 inches
Sister's circumference = 2 x 3.14 x 6.75 ≈ 42.39 inches
Difference = Sister's circumference - Poppy's circumference
Difference = 42.39 - 39.25 = 3.14 inches
Find out more on wire at https://brainly.com/question/29205033
#SPJ1
If x and x+ 10 are a pair of adjacent angle find them
Answers
Answer:
85° and 95°
Step-by-step explanation:
Given that , x and x + 10 are a pair of angles .
If they will be pair of angles on the same line , then their sum will be 180° .=> x + x + 10° = 180°
=> 2x = 180° -10°
=> 2x = 170°
=> x = 170°/2
=> x = 85° .
Hence the two angles are 85° and 95° .The graph shows the distance, y, that a car traveled in x hours:

Answers
I need help with this math assignment!! Will mark brainliest!

Answers
Answer:
Sorry but i can't help u.
Step-by-step explanation:
A tank contains 60 kg of salt and 1000 L of water. Pure water enters a tank at the rate 6 L/min. The solution is mixed and drains from the tank at the rate 3 L/min. (a) What is the amount of salt in the tank initially? (b) Find the amount of salt in the tank after 4.5 hours. (c) Find the concentration of salt in the solution in the tank as time approaches infinity. (Assume your tank is large enough to hold all the solution.)
Answers
Initially, the tank contains 60 kg of salt, calculated by multiplying the salt concentration (0.06 kg/L) by the water volume (1000 L).
In the given scenario, the tank starts with a known salt concentration and water volume. By multiplying the concentration (0.06 kg/L) with the water volume (1000 L), we find that the initial amount of salt in the tank is 60 kg.
After 4.5 hours, considering the rate of water entering and leaving the tank, the net increase in solution volume is 810 L. Multiplying this by the initial concentration (0.06 kg/L), we determine that the amount of salt in the tank after 4.5 hours is 48.6 kg.
As time approaches infinity, with a constant inflow and outflow of solution, the concentration of salt in the tank stabilizes at the initial concentration of 0.06 kg/L.
Learn more about Multiplying click here :brainly.com/question/25834626
#SPJ11
how many dimes and nickels make 6.10 dollars if there are 79 coins
Answers
I need help with this problem, I don't know how to solve it!!!

Answers
The given figure appears to be a parallelogram.
A parallelogram is a quadrilateral with two pairs of parallel sides. In the given figure, we can see that opposite sides are parallel, so it is a parallelogram.
We can also find that the measure of angle A is 120 degrees because the adjacent angles to angle A are 30 and 30 degrees, and the sum of adjacent angles in a parallelogram is 180 degrees. Similarly, the measure of angle C is 60 degrees.
The formula to find the area of a parallelogram is:
Area = base x height
We can choose any base and its corresponding height to find the area of the parallelogram. In the given figure, we can choose AB as the base and the perpendicular distance from AB to line DC as the height. Let's call this distance h.
From the figure, we can see that triangle ADE is a 30-60-90 triangle. The ratio of the sides in a 30-60-90 triangle is 1:sqrt(3):2. So, if AD is x, then DE is x*sqrt(3) and AE is 2x.
Since AE is parallel to DC, we can say that the height h is the same for triangles ADE and CFB. So, we can use triangle CFB to find the value of h.
Triangle CFB is a right triangle with angles 30, 60, and 90 degrees. The length of CB is 4 units and the length of CF is 2 units. So, the length of FB is 2*sqrt(3) units (using the ratio 1:sqrt(3):2). Therefore, the area of triangle CFB is:
Area = (1/2) x base x height
Area = (1/2) x 4 x 2
Area = 4
Since the area of triangle CFB is equal to the area of triangle ADE, we can say that:
Area of parallelogram ABCD = 2 x area of triangle CFB
Area of parallelogram ABCD = 2 x 4
Area of parallelogram ABCD = 8 square units
So, the area of the parallelogram is 8 square units.
how many cubes, with side measures of 2 cm, will fit inside a right rectangular prism with dimensions of 6 cm by 8 cm by 4 1 2 cm? group of answer choices 24 108 54 27
Answers
Answer:
Using the volume formula we know that (B) 27 cubes can be fitted into the right rectangular prism.
What is the right rectangular prism?
The right rectangular prism has four rectangle-shaped side faces and two parallel end faces that are perpendicular to each of the bases.
Parallelograms make up the sides of an oblique prism, a non-right rectangular prism.
A cuboid is yet another name for a right rectangle prism.
So, the volume of the right rectangular prism:
V = wlh
Insert values:
V = wlh
V = 6*8*4.5
V = 216cm³
Now, the volume of the cube:
V = a³
V = 2³
V = 8cm³
Then, the number of cubes that can be fitted in the right rectangular prism:
216/8 = 27
Therefore, using the volume formula we know that (B) 27 cubes can be fitted into the right rectangular prism.
Know more about the right rectangular prism? here:
brainly.com/question/3317747
#SPJ1
Correct question:
How many cubes, with side measures of 2 cm, will fit inside a right rectangular prism with dimensions of 6 cm by 8 cm by 4 1/2 cm?
Group of answer choices
a. 24
b. 27
c. 108
d. 54
The final answer is 27
To determine how many cubes will fit inside the right rectangular prism, we need to find the volume of the prism and the volume of the cubes, then divide the volume of the prism by the volume of the cubes.
Volume of a cube (V_cube) = side^3
V_cube = 2 cm * 2 cm * 2 cm = 8 cubic cm
Volume of the right rectangular prism (V_prism) = length * width * height
V_prism = 6 cm * 8 cm * 4.5 cm = 216 cubic cm
Now, divide the volume of the prism by the volume of the cubes:
Number of cubes = V_prism / V_cube = 216 cubic cm / 8 cubic cm = 27 cubes
Therefore, 27 cubes with side measures of 2 cm will fit inside the right rectangular prism.
To know more about cubes visit : https://brainly.com/question/28134860
#SPJ11