Answers
Answer:
∠ P = 116°
Step-by-step explanation:
Given:
In Δ NOP
PN = OP
∠ O = 32°
Find:
∠ P
Computation:
PN = OP
So,
∠ O = 32° = ∠ N = 32°
So,
∠ P = 180 - ∠ O - ∠ N
∠ P = 116°
Related Questions
what are all the geometry concepts
Answers
Which of the following lines have the greatest slope? EXAM DUE IN 20 MINUTES!!



Answers
A motel clerk counts his $1 and $10 bills at the end of the day. He finds that he has a total of 55 bills having a combined monetary value of $163. Find the number of bills of each denomination that he has.
Answers
Answer:
43 dollar bills and 12 ten dollar bills
Step-by-step explanation:
let x = number of $1
y = number of $10
x + 10y = 163
x+y = 55
x = 55 - y
x + 10y = 163
(55-y) + 10y =163
55 - y + 10y = 163
9y = 163 - 55
9y = 108
y = 12
x = 55 - 12
x = 43
how many ways are there to select a committee of five members of the department if at least one woman must be on the committee?
Answers
Answer: 16
Step-by-step explanation:
In the past Reagan got paid 214,350 annually. Since switching to a new career she has been making 347,247 annually. What is the percent of increase in Reagan’s pay
Answers
Answer: Reagan's pay was increased by 62%
Step-by-step explanation:
First, lets find the difference between the two pays:
$347,247 - $214,350 = $132,897
Let 214350 = 100%
Dividing 132,897 by 214350 to find the percent increase
\(\bold{\frac{132,897}{214,350} }=0.62\times100=62\)
Therefore, Reagan's pay was increased by 62%
help me pleaseeeeeee

Answers
Step-by-step explanation:
Similar triangles
\( \frac{6}{uv } = \frac{8}{3} \)
uv=18/8
uv= 2.25
91.2727272727 simplify??
Answers
Answer:
91 +3409/12500
Step-by-step explanation:
Did you mean simplify as a fraction?
What is binomial distribution table ?
Answers
Answer:
Step-by-step explanation:
A binomial distribution table is a table that lists the probabilities of obtaining a specific number of success outcomes in a fixed number of independent trials, given a constant probability of success in each trial. The binomial distribution is a discrete probability distribution that describes the number of success outcomes in a fixed number of independent trials with a binary outcome (success or failure).
The table lists the probabilities of obtaining k successes in n trials, where k is a non-negative integer and n is a positive integer. The probabilities are calculated using the binomial formula, which is given by:
P(k successes in n trials) = (n choose k) * p^k * (1 - p)^(n-k)
where (n choose k) is the number of ways to choose k success outcomes from n trials, p is the probability of success in each trial, and (1 - p) is the probability of failure in each trial.
Binomial distribution tables are useful for solving problems related to counting and probability, especially in situations where the number of trials is fixed and the probability of success is constant. They can also be used for hypothesis testing, calculating confidence intervals, and estimating population proportions in statistical applications.
which expression is a possible leading term for the polynomial function graphed below? –18x14 –10x7 17x12 22x9
Answers
Among the given expressions, the one that could be the possible leading term for the polynomial function graphed below is -18x¹⁴.
The leading term of a polynomial function is the term containing the highest power of the variable. Among the given expressions, the one that could be the possible leading term for the polynomial function graphed below is -18x¹⁴.
The degree of a polynomial function is the highest degree of any of its terms.
If a polynomial has only one term, then the degree of that term is the degree of the polynomial and is also called a monomial.
For example, consider the given function:Now, observe the degree of the function, which is 14, as the highest exponent of the function is 14.
Thus, the term containing the highest power of the variable x is -18x¹⁴.
Therefore, among the given expressions, the one that could be the possible leading term for the polynomial function graphed below is -18x¹⁴.
To know more about probability visit :-
https://brainly.com/question/1496352
#SPJ11
people drive an average of 12,000 miles per year with a standard deviation of 2,580 miles per year. what is the probability that a randomly selected sample of 36 drivers will drive, on average, more than 12,500 miles? does the central limit theorem apply? what is the sampling distribution of the mean?
Answers
The probability that a randomly selected sample of 36 drivers will drive, on average, more than 12,500 miles is approximately 0.123
This problem involves the sample mean of a set of data, and we can use the central limit theorem to approximate the distribution of sample means, even if the original distribution is not normal.
Let X be the number of miles driven by a single driver in a year. We know that the population mean µ = 12,000 miles and the population standard deviation σ = 2,580 miles. We also know that the sample size n = 36.
The sample mean X is an estimator of the population mean µ. The distribution of sample means is approximately normal with a mean of µ and a standard deviation of σ/√n, according to the central limit theorem
So, the distribution of sample means can be expressed as
X ~ N(µ, σ/√n)
Substituting the given values, we get
X ~ N(12,000, 2,580/√36) = N(12,000, 430)
Now we need to find the probability that a randomly selected sample of 36 drivers will drive, on average, more than 12,500 miles. This is equivalent to finding the probability that the sample mean is greater than 12,500
P(X > 12,500) = P(Z > (12,500 - 12,000) / 430)
where Z is a standard normal random variable.
P(Z > 1.16) = 1 - P(Z < 1.16) = 1 - 0.877 = 0.123
Learn more about probability here
brainly.com/question/11234923
#SPJ4
The given question is incomplete, the complete question is:
People drive an average of 12,000 miles per year with a standard deviation of 2,580 miles per year. what is the probability that a randomly selected sample of 36 drivers will drive, on average, more than 12,500 miles?
Help me I need this quick

Answers
Answer:
I think 3,9, and 15
Step-by-step explanation:
Evaluating the solution is the last step of the problem solving process.
Answers
It is true that evaluating the solution is the last step of the problem solving process.
What is problem solving?The idea that mathematics is essentially about thinking, not memorizing, leads to the significance of problem-solving in math education. Instead than just recalling and using a set of instructions, problem-solving enables students to comprehend and articulate the steps taken to reach solutions. Math problem-solving has two main objectives: to increase students' motivation to try issues and to increase their persistence when solving problems. Enhance pupils' perceptions of their own problem-solving skills. Make the techniques for solving problems known to the pupils.
Here,
It is true that the final phase in the problem-solving process is assessing the solution.
To know more about problem solving,
https://brainly.com/question/11526758
#SPJ1
Designs of experiments (DOE) is a tool for analyzing potential reliability problems and weaknesses in a product or process a statistical measure used to determine whether there is a statistical, not random, difference in the means of two groups of data a statistical technique used in Six Sigma to collect, analyze, and interpret data a structured, organized method for determining whether there is a statistical correlation between two variables Productivity is broadly defined as output divided by input the quality-productivity ratio quality cost divided by production cost yield divided by total planned units
Answers
DOE is a statistical tool for analyzing reliability issues, while productivity is a measure of efficiency. Designs of experiments (DOE) is a statistical technique used in Six Sigma to collect, analyze, and interpret data.
It is a structured and organized method for determining whether there is a statistical correlation between two variables. DOE helps in identifying potential reliability problems and weaknesses in a product or process by systematically varying the factors and observing their impact on the output.
Productivity is a measure that broadly defines the efficiency of a process or system. It is calculated by dividing the output by the input. On the other hand, the quality-productivity ratio refers to the relationship between the quality and productivity of a product or process. It can be represented by the ratio of quality cost divided by production cost or the ratio of yield divided by total planned units.
In summary, DOE is a statistical tool for analyzing reliability issues, while productivity is a measure of efficiency. The quality-productivity ratio assesses the relationship between quality and productivity by considering factors such as quality cost, production cost, and yield.
Learn more about productivity here
brainly.com/question/31859289
#SPJ11
Rachel has a $10,000, three-year loan with an APR of 7.25%.
a. What is the monthly payment?
b. What is the total amount of the monthly payments?
c. What is the finance charge
Answers
Simple interest analysis reveals that
a) The installment was $321.53 per month.
b) The total amount of the payments is $11,575.
c) Interest was paid of $1,575.
How to find the Calculation?After t years, the amount of simple interest is calculated using:
A(t) = p(1 + rt)
that where:
The initial deposit is known as the primary, or P.
The interest rate in decimal form is r.
In this issue:
Hence, a $10,000 loan.
APR of 5.25%, which equals 3 years, therefore
Item c:
The result is A(3), so:
A(t) = P( 1 + rt)
A(3) = 10000[ 1 + 0.0525(3)]
A(3) = 11575
The total amount of the monthly installments is $11,575.
Item d:
I = A(3) - P = 11575 - 10000 = 1575
When interest is calculated, the principal is removed, so:
Interest was paid of $1,575.
Item b:
36 payments were made over a period of 3 years, or 3 x 12 months.
Item a:
11575/3 = 321.53
The amount due each month was $321.53.
To Learn more About interest analysis refer to:
https://brainly.com/question/28212065
#SPJ1
Question 3 (8 points)
You have $10.00. Each week you
save $2.50. The number of weeks
you save w increases your
savings s.
Write an equation relating your savings s to the number of weeks you save w.
help me make the equation please

Answers
s= 10 + 2.50w
explanation:
the amount of money in your savings is equal to the initial $10 you had before you started saving 2.50 per week. weak week $2.50 is added to the savings. 2.50w can be seen as 2.50 times the amount of weeks. say you were 3 weeks into saving, you would do 10 + 2.50(3) to find the amount of money you have.
y = 4x + 10 y = 3x + 15
Answers
Answer:
-(135/31), 60/31
Step-by-step explanation:
y=60/31
x=-(135/31)
Ms. Carter charges her piano students $40 per lesson plus a semester
recital fee of $55. How many lessons did Tyhir take if he paid a total of $695
for lessons this semester?
1: 14
2: 15
3: 16
4: 17
Answers
Work: 695-55 ( because the question is not asking for recitals). Then you do 640/40. That gives you an answer of 16.
Have a nice day!!!
PLEASE HELP ASAP! WILL MARK BRAINLIEST

Answers
compare the y-intercept and the rate of change of the following items

Answers
Answer:
A) They have the same y intercept and the same rate of change
Step-by-step explanation:
Write the equation for the line passing through the points (2,4) and (12,7)
Slope \(\frac{7-4}{12-2}\) = \(\frac{3}{10}\) = .3
y intercept (b)
y = mx + b
Use 2 fox x, 4 for y and .3 for m
y=mx + b
4 = .3(2) + b
4 = .6 + b Subtract .6 from both sides
3.4 = b
The equation is
y = .3x + 3.4
The two equation are identical so the slope and y intercepts are the same.
compare the y-intercept and the rate of change of the following items. Option (d) The rates of change are the same, but the y-intercepts are different. is correct.
for rates,
we will find the slope of I and II
slope = dy/dx = change in y/change in x
for both of them when we compare the slope, it is 0.3.
for y-intercepts.
equation for II should be formed in order to compare with the y-intercept of I which is 3.4.
y-7=0.3(x-12) .................(i)
so, the y-intercept is 7 for II
thus, the y-intercepts of given eq are not equal.
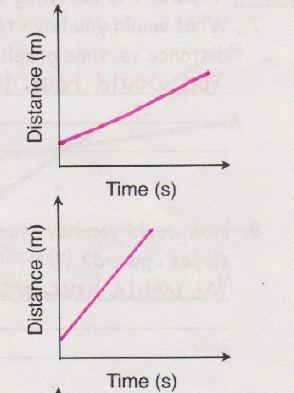
3. Sketch the distance-time graphs for the following scenarios.
a. Stand one meter from the sensor, walk
at a constant (steady) slow pace away
from the sensor.
b. Stand one meter from the sensor, walk
away from the sensor changing your
pace from slow to fast.
Answers
The distance-time graphs for the given scenarios have been attached below. The solution has been obtained using distance-time function.
What is distance-time function?
The distance between a person and an object through time is described by distance-time functions. Depending on the kind of movement, a distance-time function may be linear or non-linear, increasing, decreasing, or constant. We need to tell where the object starts, what direction it moves in, how quickly it moves, and whether it is speeding up, slowing down, or travelling at a constant speed in order to explain a distance-time function.
a. The distance-time graph for this scenario is the first graph wherein the line is less steeper than the other graph because the speed is constant.
b. The distance-time graph for this scenario is the second graph wherein the line is steeper than the first one because the speed is changing from slow to fast.
Hence, the distance-time graphs for the given scenarios have been obtained.
Learn more about distance-time function from the given link
https://brainly.com/question/28753735
#SPJ1
CONSTRUCT ARGUMENTS Consider three points, (3, 7), (−6, 1), and (9, p) on the same line. Find the value of p. Justify your argument.
Answers
Answer:
p=11
Step-by-step explanation:
let A(3,7),B(-6,1),C(9,p) be the points.
or
B(-6,1),A(3,7),C(9,p) are the points in the same line.
let A divides BCin the ratio k:1
\(3=\frac{9k+1(-6)}{k+1} \\3k+3=9k-6\\3k-9k=-6-3\\-6k=-9\\k=\frac{-9}{-6} =\frac{3}{2} \\so~ratio~is~\frac{3}{2} :1\\or\\3:2\\again\\7=\frac{3p+2(1)}{3+2} \\5*7=3p+2\\3p=35-2=33\\p=\frac{33}{3} =11\)
What is the value of the expression below when x=10x=10 and y=4y=4? 8x-7y 8x−7y
Answers
Answer:
X=10x =10
means here value of X is 1
y = 4y = 4
means here value of y is also 1
now substitute the values of X and y in the equation
8×1-7×1×(8×1-7×1)
8-7(8-7)
64-49
15
Which of the following is an example of a quadratic equation?
O A. y = 4x +6
O B. x2 - 64 = 0
O C. X+ 10 = 33
O D. y=1/x2+6
Answers
Answer:
\(x^{2} -64 = 0\\\)
Step-by-step explanation:
According to Wikipedia In algebra, a quadratic equation is any equation that can be rearranged in standard form as where x represents an unknown, and a, b, and c represent known numbers, where a ≠ 0. If a = 0, then the equation is linear, not quadratic, as there is no term.
A submarine drops 1194 ft below sea level in 15 minutes. What is the rate of descent of the submarine
Answers
Answer:
79.6 ft / 1 minute
Step-by-step explanation:
Answer:
79.6ft per minute
Step-by-step explanation:
1194/15=79.6
Can you help me with question 4 step by step

Answers
Answer:
exponetial growth; y-0.25
Answer:
the second option
Step-by-step explanation:
6(0.25)* 1 = 1.5 if x equals 1
6(0.25) * 2= 3 if x equals 2
A sporting goods store manager was selling a ski set for a certain price. The manager offered the markdowns shown, making the one-day sale price of the ski set $323. Find the original selling price of the ski set.

Answers
The original selling price is $520.632
Explanation:
Consider the initial selling price be $x
After marking down 10%,
Final Selling price = Selling price(1-10%)
Selling price = x – 10% of x = x – 0.1x = 0.9x
After marking 30%
Final Selling price = Selling price(1-10%)
Selling price = 0.9x – 0.3*0.9x = 0.9x – 0.27x = 0.63x
According to the question,
0.63x = 328
Therefore, the original selling price is $520.632
Answer:
The original selling price is $520.632
Explanation:
Consider the initial selling price be $x
After marking down 10%,
Final Selling price = Selling price(1-10%)
Selling price = x – 10% of x = x – 0.1x = 0.9x
After marking 30%
Final Selling price = Selling price(1-10%)
Selling price = 0.9x – 0.3*0.9x = 0.9x – 0.27x = 0.63x
According to the question,
0.63x = 328
Therefore, the original selling price is $520.632
Hope It helps!!❤
The results of a paired-difference test are shown below to the right. d = 5.6
a. Construct and interpret a 99% confidence interval estimate for the paired difference Sd =0.25 in mean values.
b. Construct and interpret a 90% confidence interval estimate for the paired difference n=16 in mean values_ (Round to two decimal places as needed:) Choose the correct answer below:
OA This interval will contain the true population mean 90% of the time_
OB. There is a 90% chance that the true population mean is contained in the interval.
Oc: If many random samples of this size were taken and intervals constructed, 90% of them would contain the true population mean: 0
D. Approximately 90% of the differences will be contained in the interval.
Answers
If many random samples of this size were taken and intervals constructed, 90% of them would contain the true population mean. In repeated sampling, about 90% of the constructed confidence intervals will capture the true population mean difference. The correct answer is C.
When we construct a confidence interval, it is important to understand its interpretation. In this case, the correct answer (Oc) states that if we were to take many random samples of the same size and construct confidence intervals for each sample, approximately 90% of these intervals would contain the true population mean difference.
This interpretation is based on the concept of sampling variability. Due to random sampling, different samples from the same population will yield slightly different sample means.
The confidence interval accounts for this variability by providing a range of values within which we can reasonably expect the true population mean difference to fall a certain percentage of the time.
In the given scenario, when constructing a 90% confidence interval for the paired difference, it means that 90% of the intervals we construct from repeated samples will successfully capture the true population mean difference, while 10% of the intervals may not contain the true value.
It's important to note that this interpretation does not imply a probability or chance for an individual interval to capture the true population mean. Once the interval is constructed, it either does or does not contain the true value. The confidence level refers to the long-term behavior of the intervals when repeated sampling is considered.
Learn more about confidence interval at https://brainly.com/question/15576092
#SPJ11
What is the difference in speed between two cars when one traveled 175 km in 4 hours and the other traveled the same distance in 3 hours?
Answers
\( \\ \\ \)
To find:Different between two speeds when distance is same.\( \\ \\ \)
Solution:Part 1 :
In first part we will find speed 1 in which time is 4 hours
we know:-
\( \bigstar \boxed{ \rm speed = \frac{distance}{time} }\)
So:-
\( \dashrightarrow\sf speed_1 = \dfrac{distance}{time_1} \)
\( \\ \\ \)
\( \dashrightarrow\sf speed_1 = \dfrac{175}{4} \)
\( \\ \\ \)
\( \dashrightarrow\bf speed_1 = 43.75 \: km {h}^{ - 1} \)
Part 2
In first part we will find speed 2 in which time is 3 hours
Again:
\( \bigstar \boxed{ \rm speed = \frac{distance}{time} }\)
\( \\ \\ \)
\( \dashrightarrow\sf speed_2 = \dfrac{distance}{time_2} \)
\( \\ \\ \)
\( \dashrightarrow\sf speed_2 = \dfrac{175}{3} \)
\( \\ \\ \)
\( \dashrightarrow\bf speed_2 = 58.33kmh^{-1}\)
\( \\ \\ \)
Part 3
In this part we will find different between speed 1 and speed 2
\( \bigstar \boxed{\rm Difference = speed_2 - speed_1}\)
\( \\ \\ \)
So :
\( \dashrightarrow\sf Difference = speed_2 - speed_1 \\ \)
\( \\ \\ \)
\( \dashrightarrow\sf Difference =58.33 - 43.75\\ \)
\( \\ \\ \)
\( \dashrightarrow\bf Difference =14.58kmh^{-1}\\ \)
\( \\ \\ \)
\(\therefore \underline {\textsf{\textbf{Difference in speed between two cars is \red{14.58km/h}}}}\)
Seven friends shared a two-thirds pound bag of pistachios. If each person had the same amount of nuts, how many pounds did each person eat?.
Answers
Each person ate 21/2 pounds of nut
First of all, we have 7 friends who are going to share 2/3 pound bag of pistachios
This will mean that we will have to divide equally the two-thirds-pound bag of pistachios among the seven people that we have so that we get the share that each person will get
There are 7 friends that share a 2/3-pound bag.
The first step will be: 7÷2/3
7÷0.6666
we will get 21/2 or 10.5
This will imply or mean that each of the friends will get an equal share of 21/2 of the a two-thirds pound bag of pistachios
Learn more about word problems here: https://brainly.com/question/21405634
#SPJ4
Will give brainliest! help asap!

Answers
Answer:
Angle NOR is 127 degrees
Step-by-step explanation:
This is an example of the vertical angles theorem
Answer:
127
Step-by-step explanation:
This is ALSO called vertical angle. They are both congruent.
