Answers
Answer:
Step-by-step explanation:
y - 4 = -2/3(x - 3)
y - 4 = -2/3x + 2
y = -2/3x + 6
Related Questions
find the limit. use l'hospital's rule where appropriate. if there is a more elementary method, consider using it. lim x→0 6x − sin(6x) 6x − tan(6x)
Answers
Answer:
-1/2
Step-by-step explanation:
You want the limit as x→0 of the ratio (6x -sin(6x))/(6x -tan(6x)).
L'Hôpital's ruleThe given expression evaluates at x=0 to (0 -0)/(0 -0) = 0/0, an indeterminate form. Hence, L'Hôpital's rule applies.
The ratio of derivatives of the numerator and denominator is ...
(6 -6cos(6x))/(6 -6sec²(6x))
which still evaluates to 0/0 at x=0.
Applying L'Hôpital's rule a second time gives ...
\(\dfrac{36\sin(6x)}{-72\sec^2(6x)\tan(6x)}=\dfrac{-\cos^3(6x)}{2}\)
At x=0, this evaluates to -1/2.
The limit as x→0 is -1/2.
__
Additional comment
We like to check these limits using a graphing calculator. The graph is in the second attachment.
<95141404393>


(L5) Given: ΔABC with AC>AB;BD¯ is drawn so that AD¯≅AB¯Prove: m∠ABC>m∠C
Answers
Angle ABC is greater than angle C, as required. Given triangle ABC with AC greater than AB, and BD drawn such that AD is congruent to AB, we need to prove that angle ABC is greater than angle C.
To begin with, we can draw a diagram to visualize the situation. In the diagram, we see that BD is an altitude of triangle ABC, as well as a median since it divides the base AC into two equal parts. We also see that triangles ABD and ABC are congruent by the side-side-side (SSS) criterion, which means that angle ABD is equal to angle ABC.
Now, we can use this information to prove our statement. Since triangle ABD and triangle ABC are congruent, their corresponding angles are also equal. Therefore, we know that angle ABD is equal to angle ABC.
Next, we observe that angle ABD is a right angle, since BD is an altitude of triangle ABC. This means that angle ABC is the sum of angles ABD and CBD.
Since AD is congruent to AB, we also know that angles ABD and ADB are congruent. Therefore, angle CBD is greater than angle ADB.
Putting all of this together, we can conclude that angle ABC is greater than angle C, as required.
In summary, we have shown that given triangle ABC with AC greater than AB and BD drawn such that AD is congruent to AB, angle ABC is greater than angle C. This is because angles ABD and CBD add up to angle ABC, and angle CBD is greater than angle ADB.
learn more about congruence here: brainly.com/question/27922830
#SPJ11
The graph below represents the path of a squirrel traveling up and down a tree over time.
The position of the squirrel can be calculated using the function p(t) =at^2 +bt +c

Answers
The values of the variables are a = -1.25, b = 10 and c = 40
How to determine the value of the variables?From the question, we have the following parameters that can be used in our computation:
p(t) =at² +bt +c
When this equation is expressed in vertex form, the equivalent is
p(t) =a(t - h)² + k
Where
Vertex, (h, k) = (4, 20)
So, we have
p(t) = a(t - 4)² + 20
From the graph, we have
Point (0, 0)
This means that
a(0 - 4)² + 20 = 0
So, we have
16a = -20
This gives
a = -1.25
Substitute a = -1.25 in p(t) = a(t - 4)² + 20
p(t) = -1.25(t - 4)² + 20
Expand the bracket
p(t) = -1.25(t² - 8t + 16) + 20
So, we have
p(t) = -1.25t² + 10t + 20 + 20
Evaluate the sum
p(t) = -1.25t² + 10t + 40
This means that a = -1.25, b = 10 and c = 40
Read more about quadratic equations at
https://brainly.com/question/1214333
#SPJ1
suppose you and i play a game where we take turn flipping a coin. the first person to flip heads wins. does it matter who goes first, and if so, would you prefer to go first?
Answers
No, it does not matter who will go first as the probability of getting both head and tail after flipping a coin is equal to ( 1 / 2 ).
As given in the question,
Number of players playing the game = 2
Number of coins to flip = One
Possible outcomes after flipping a coin = { Head , Coin }
Probability of getting a head
= (Number of favourable outcomes) / ( Total number of outcomes)
= 1 / 2
Probability of getting a tail
= 1 / 2
Either you tossed first or second chances are fifty percent.
Therefore, it does not matter who will go first as probability of getting head and tail is ( 1 / 2) after flipping a coin.
Learn more about flipping a coin here
brainly.com/question/13262284
#SPJ4
what is the value of b2-4ac for the following equation? 5x2+7x=6
Answers
Answer:
169
Step-by-step explanation:
Given data
The function is
5x^2+7x=6
rearrange
5x2+7x-6=0
From the above expression, we can see that
a=5
b=7
c=-6
Substitute into the expression b^2-4ac
=7^2-4*5*-6
= 49-(-120)
=49+120
=169
Hence the answer is 169
Andrea reads 20 pages an hour. Which expression below represents how many pages Andrea would read in h hours?
A. 60h
B. 20h
C. 20 + 60 + h
D. 20 + h
Answers
x-5y=2 and 4x+2y=8 using elimination
Answers
Answer:
y=0 x=2
Step-by-step explanation:
Answer:
x=2+5y
and
x=2-y/2
Step-by-step explanation:
How do the areas of the parallelograms compare? On a coordinate plane, parallelograms A B C D and E F G H are shown. Parallelogram A B C D has points (4, 2), (7, 2), (4, 6), (1, 6). Parallelogram E F G H has points (negative 2, 2), (negative 5, 2), (negative 6, 6), and (negative 3, 6).
Answers
The areas of both parallelograms compare in that: They both have the same area
How to find the area of the Parallelogram?The coordinates of Parallelogram ABCD are given as:
A(4, 2), B(7, 2), C(4, 6), D(1, 6).
The coordinates of Parallelogram EFGH are:
E(-2, 2), F(-5, 2), G(-6, 6), and H(-3, 6)
The area of a parallelogram is given by the formula:
Area = base * height
So: To do this, we plot ABCD and EFGH on a grid, then we measure the base and the height of both.
For ABCD:
base = 3
height = 4
Area = 3 * 4 = 12 sq.units
For EFGH, we have same thing:
Area = 3 * 4 = 12 sq.units
Read more about Area of Parallelogram at: https://brainly.com/question/970600
#SPJ1
Complete question is:
On a coordinate plane, parallelograms A B C D and E F G H are shown. Parallelogram A B C D has points (4, 2), (7, 2), (4, 6), (1, 6). Parallelogram E F G H has points (negative 2, 2), (negative 5, 2), (negative 6, 6), and (negative 3, 6). How do the areas of the parallelograms compare? The area of parallelogram ABCD is 4 square units greater than the area of parallelogram EFGH. The area of parallelogram ABCD is 2 square units greater than the area of parallelogram EFGH. The area of parallelogram ABCD is equal to the area of parallelogram EFGH. The area of parallelogram ABCD is 2 square units less than the area of parallelogram EFGH.
write an explicit formula for a n the nth term of of the sequence 8,48,288
Answers
Answer:(n=6n)
Step-by-step explanation:
In the right triangle shown, angle C is 90, cos(B)- 036 and side a side c? A) 3x0.36 B) None of the answers listed C) 3/5 D) 5 E) 3/2
Answers
The answer is A) 3x0.36, which is approximately equal to 4.68
Since cos(B) = 0.36, we can use the Pythagorean identity
To find sin(B):
sin^2(B) + cos^2(B) = 1
sin^2(B) = 1 - cos^2(B)
sin(B) = sqrt(1 - cos^2(B)) = sqrt(1 - 0.36^2) = sqrt(0.8736) ≈ 0.934
Now we can use the sine ratio to find side a:
sin(B) = a/c
a = sin(B) * c = 0.934 * 5 = 4.67
So the answer is A) 3x0.36, which is approximately equal to 4.68 (close to our calculated value for side a).
Click the below link, to learn more about right triangle:
https://brainly.com/question/13324532
#SPJ11
Help me- what number of units and where

Answers
Just pick any point on the blue triangle and count how many you move to get to that same point on the red triangle.
Which expressions are equivalent to 7x - 14? Check all that apply.
O 114 - 7x
O X-2
O -(14-7x)
O 7(x - 2)
O 3x – 14 + 4x
O 2x - 10 + 5x +4
Answers
Answer:
-(14 - 7x); 7(x - 2); 3x - 14 + 4x
Step-by-step explanation:
for -(14 - 7x) when the negative is distributed you get -14 + 7x which is still 7x - 14
for 7(x - 2) when multiplied in you get 7x - 14
for 3x - 14 + 4x when added together you get 7x - 14
Help me pls and thank you

Answers
Answer:
To make a table NOT a function you can make any input value lead to two or more outputs.
Step-by-step explanation:
Ex.
(2,3)
(1,4)
(6,7)
(2,8)
Write a linear equation that represents each situation.
a. An equation for the line below.

Answers
The linear functions in each context are presented as follows:
1. y = -0.5x + 4.
2. y = 2x - 1.
3. y = 0.4x + 1.4.
4. C(t) = 7t + 8.
What is a linear function?A linear function is defined by the equation presented below:
y = mx + b.
In which the meaning of the variables m and b is presented as follows:
m is the slope of the line.b is the y-intercept of the line.For item 1, the line crosses the y-axis at y = 4, hence the intercept is given as follows:
b = 4.
When x increases by 8, y decays by 4, hence the slope is given as follows:
m = -4/8 = -0.5.
Hence the equation is:
y = -0.5x + 4.
When two lines are perpendicular, the multiplication of their slopes is of -1, hence:
-0.5m = -1
m = 2.
Then:
y = 2x + b.
When x = -1, y = -3, hence the intercept is calculated as follows:
-3 = -2 + b
b = -1.
Hence the equation is:
y = 2x - 1.
For item 3, the slope is calculated as the change in y divided by the change in x, hence:
m = (3 - 1)/(4 - (-1)) = 2/5 = 0.4.
Hence:
y = 0.4x + b.
When x = 4, y = 3, hence the intercept is calculated as follows:
3 = 0.4 x 4 + b
b = 3 - 1.6
b = 1.4.
Hence the equation is:
y = 0.4x + 1.4.
For cost functions, we have that:
The fixed cost is the intercept.The variable cost is the slope.Hence, for item 4, the equation is:
C(t) = 15 + 7(t - 1) -> considering that 7 is only charged after the first hour
C(t) = 15 + 7t - 7
C(t) = 7t + 8.
More can be learned about linear functions at https://brainly.com/question/24808124
#SPJ1
12 customers entered a store over the course of 2 minutes. At what rate were the
customers entering the store in minutes per customer?
Answers
Answer:
.16
Step-by-step explanation:
if you divide 2 min into 12 it's going to give you the rate which is .1666666667 and just say .16 each person
i need help in mathematics please i will give branliest
4. Find the value of x so that the mean of the given data : 14, 6,2x,8,10,4 is 8
5.what number should be included in the data 2,8,7,4 and 9 so that the mean is 6
Answers
Answer:
0
Step-by-step explanation:
find mean of given numbers u notice that mean is actually 6 so no number is required to be included
Answer:
4. x = 35. Number 6 should be includedStep-by-step explanation:
Mean is the average
4.
Data given:
14, 6, 2x, 8, 10, 4The mean is
mean = sum of the data / number of data(14 + 6 + 2x + 8 + 10 + 4)/6 = 842 + 2x = 6*82x = 48 - 422x = 6x = 35. Let the added number is x, then the mean is:
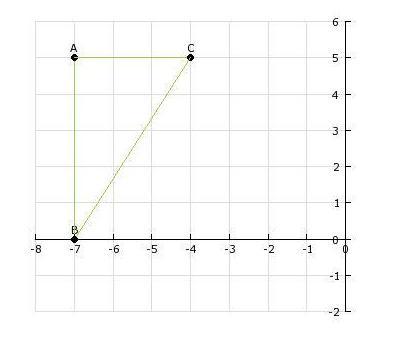
(2 + 8 + 7 + 4 + 9 + x)/ 6 = 6x + 30 = 36x = 36 - 30x = 6Find the distance between points B and C on the graph.
A√5
B18
C5
D√34
E √146
Answers
The distance between points B and C on the graph is equal to: D. √34 units.
What is Pythagorean theorem?In Mathematics, Pythagorean's theorem is represented by the following mathematical expression:
c² = a² + b²
Where:
a, b, and c represent the side length of any right-angled triangle.
How to determine the distance between points B and C?In order to determine the distance between points B and C in right-angled triangle ABC, we would have to apply Pythagorean's theorem.
BC² = AB² + AC²
BC² = 5² + 3²
BC² = 25 + 9
BC² = 34
BC = √34 units.
Read more on Pythagorean theorem here: brainly.com/question/15430861
#SPJ1
Help ASAP I’ll mark you as brainlister

Answers
Answer:
100°
Step-by-step explanation:
Answer:
it is 100
Step-by-step explanation:
Since the two opposite angles in a rhombus are equal
Researchers are interested in determining whether more women than men prefer the beach to the mountains. In a random sample of 200 women, 45% prefer the beach, whereas in a random sample of 300 men, 52% prefer the beach. What is the 99% confidence interval estimate for the difference between the percentages of women and men who prefer the beach over the mountains?
Answers
Answer:
The 99% confidence interval estimate for the difference between the percentage of women and men who prefer the beach over the mountains is -18.72% < (p₁ - p₂) < 4.72%
Step-by-step explanation:
The given parameters are;
Sample size of the women sample, n₁ = 200
Percentage of the women that prefer beach, p₁ = 45%, \(\hat p_1\) = 0.45
Sample size of the men sample n₂ = 300
Percentage of the men that prefer beach, p₂ = 52%, \(\hat p_2\) = 0.52
Confidence level of the confidence interval = 99%
α = 1 - 0.99 = 0.01, therefore, α/2 = 0.005
The equation for the confidence interval for the difference between two proportions is given as follows;
\(\left (\hat{p}_{1} - \hat{p}_{2} \right )\pm z_{\alpha /2}\sqrt{\dfrac{\hat{p}_{1}(1-\hat{p}_{1})}{n_{1}}+\dfrac{\hat{p}_{2}(1-\hat{p}_{2})}{n_{2}}}\)
\(z_{\alpha /2}\) = ±2.57 from the z score tables
Plugging in the vales, we have;
\(\left (0.45 - 0.52 \right )\pm 2.57\sqrt{\dfrac{0.45(1-0.45)}{200}+\dfrac{0.52(1-0.52)}{300}}\)
Which gives;
-0.1872 < \((\hat p_1 - \hat p_2)\) < 0.0472
The 99% confidence interval estimate for the difference between the percentage of women and men who prefer the beach over the mountains = -18.72% < (p₁ - p₂) < 4.72%.
Determine between which consecutive integers the real zeros of f(x)=x²-4x-2 are located.
a. between 3&4 and -1&0
c. between 4&5 and -1&0
b.
between 4&5 and 0&1
d.
between 3&4 and 0&1
Please select the best answer from the choices provided
ΟΑ
OB
Ос
OD
Answers
Answer:
Step-by-step explanation:
To find the real zeros of f(x) = x²-4x-2, we can use the quadratic formula:
x = (-b ± √(b²-4ac)) / 2a
Here, a = 1, b = -4, and c = -2.
x = (4 ± √(16+8)) / 2
x = (4 ± 2√6) / 2
x = 2 ± √6
Therefore, the real zeros are located between 2-√6 and 2+√6, which is approximately between 0.17 and 3.83.
So, the answer is (d) between 3&4 and 0&1.
PLEASE I NEED THIS QUICK!!!!!
Susan wants to make pumpkin bread and zucchini bread for the school bake sale. She has 15 eggs and 16 cups of flour in her pantry. Her recipe for one loaf of pumpkin bread uses 2 eggs and 3 cups of flour. Her recipe for one loaf of zucchini bread uses 3 eggs and 4 cups of flour. She plans to sell pumpkin bread loaves for $5 each and zucchini bread loaves for $4 each. Susan wants to maximize the money raised at the bake sale. Let x represent the number of loaves of pumpkin bread and y represent the number of loaves of zucchini bread Susan bakes.
What is the objective function for the problem?
P = 15x + 16y
P = 5x + 7y
P = 5x + 4y
P = 4x + 5y
Answers
The amount of money raised can be calculated by multiplying the number of pumpkin bread loaves (x) by the selling price of each pumpkin bread loaf ($5), and adding it to the product of the number of zucchini bread loaves (y) and the selling price of each zucchini bread loaf ($4).
Therefore, the objective function for this problem is:
P = 5x + 4y
So, the correct option is:
P = 5x + 4y
determine the equation of the circle graphed below .

Answers
Answer:
(x+1)^2 + (y-7)^2 = 2^2
Step-by-step explanation:
The center of the circle is at (-1,7) and the radius from the center to the edge is 2, so the equation should look like (x-h)^2 + (y-k)^2 = r^2
You would flip the signs of the center so that when you solve the equation it ends up in the correct place.
Pls Pls help asap! no f links pls n will give brainliest n points?
How could you show

Answers
By SAS similarity, ΔKML and ΔRTL are similar triangles. Therefore, option A is the correct answer.
From the given figure, KR=9 units, RL=15 units, MT=7.5 units and TL=12.5 units.
What are similar triangles?Two triangles are similar if the angles are the same size or the corresponding sides are in the same ratio. Either of these conditions will prove two triangles are similar.
Consider ΔKML and ΔRTL, we get
RL/KL =15/(15+9)
= 15/24
= 5/8
TL/ML =12.5/(12.5+7.5)
= 12.5/20
= 5/8
Here, RL/KL=TL/ML
Here, ∠KLM≅∠RLT
By SAS similarity, ΔKML and ΔRTL are similar triangles
So, ∠KMT≅∠RTL
By SAS similarity, ΔKML and ΔRTL are similar triangles. Therefore, option A is the correct answer.
To learn more about the similar triangles visit:
https://brainly.com/question/25882965.
#SPJ1
Answer:
a
Step-by-step explanation:
got it right in edmentum
Investors buy a studio apartment for $200,000 of this amount they have a down payment of $50,000 their down payment is what percent of the purchase price what percent of the purchase price would a $70,000 down payment be their down payment is what percent of the purchase price
Answers
Answer: 35%
Step-by-step explanation:
$70,000 was the down payment for a total price of $200,000.
Down payment percentage = 70,000 / 200,000
= 0.35
= 35%
The average temperature decrease of -6.5 degrees/km celsius is known as ________. group of answer choices dry adiabatic rate normal lapse rate stability environmental lapse rate maximum mixing depth
Answers
The average temperature decrease of -6.5 degrees/km Celsius is known as Normal lapse rate.
Normal lapse rate is defined as the decrease in the temperature while moving upwards in the atmosphere. Normally, the rate of decrease is 1 degree Celsius for every 165 meter rise in altitude or we can say that it is 6.5 degree Celsius for every 1 km rise in altitude.
This lapse rate is known as normal lapse rate or environmental lapse rate.
This is why when we move higher in the atmosphere, we experience low temperatures. On average this decrease is equal to the amount of temperature as discussed in the normal lapse rate.
You can learn more about the normal lapse rate from
https://brainly.in/question/2875341
#SPJ4
Need help asap Please
1. How can you save for your goals faster?
a. choosing needs, not wants
b. choosing wants before needs
c. choosing needs before wants
d. choosing wants, not needs
2. Which of these can help you save money?
a. Budgeting money
b. increasing income
c. reducing expenses
d. all of the above
3. Calculating your expenses helps you budget because ____________.
a. you can figure out how to spend less and save more
b. you can stop spending money and save for your goals
c. you can return things you have bought more easily
d. you can understand how much money you can spend
Answers
Answer:
1. C
2. D
3. probably A
hope this helps.
Help out with this question please!

Answers
Answer:
my answer is A
Step-by-step explanation:
if you work out the equation where you know that at the x intercept y=0 you will find A to be true
What is the value of the expression below when x=5x=5?
8x+9
Answers
The value of the expression below when x=5x=5?
8x+9 is equal to \(7^{2}\) (or) 49.
Substitute \(\left \{ {{x=5x} \atop {5x=5}} \right. into (8x+9)\)
The expression,
y = 8x + 9
If the domain of the function is x = 5, hence the range of the function is gotten by substituting x = 5 into the given function as shown:
So, we can substitute x = 5,
Then, y = 8 * 5 + 9
Calculate the product or quotient,
y = 40 + 9
Calculate the sum or difference,
y = 49
We can write alternative form,
y = \(7^{2}\)
Therefore,
Hence the value of the expressions if x = 5 is \(7^{2}\) (or) 49.
To learn more about value of expression problems visit : brainly.com/question/26170139
#SPJ9
ratio of 3 boys and 4 girls there are now 12 boys
Answers
Answer:
There are 16 girls.
Step-by-step explanation:
3 : 4
12 : x
Now if we cross multiply:
3(x) = 12(4)
3x = 48
x = 16
The price of a tv is rs3000.if the discount is rs1560,find the percent of discount.
Answers
Answer:
price of tv = 3000
discount =1560
discount % = 1560/3000 * 100% = 52%
Step-by-step explanation:
Answer:
52
Step-by-step explanation:
Formula =discount ×100÷original price
1560×100÷3000=52%
