If you invest 5000 Swiss Francs
today in an investment that pays
3.25% per annum, compounded for the investment to double in
value, assuming no additional
withdrawals or deposits are
made?
Answers
Answer:
22 years.
Step-by-step explanation:
(continued), How long will it take the 5000 Swiss Francs to accrue to 10000 = 2 x 5000 Swiss Francs.
Define T as
T = the time to double the investment.
Then, we have that
10000 = 5000(1 + 3.25%)^T
2 = (1 + 0.0325)^T
2 = (1.0325)^T
ln(2) = ln [ (1.0325)^T ]
ln(2) = T ln (1.0325)
T = ln(2) / ln(1.0325)
T = 21.6723317643
T = 22 years, rounded to the nearest year.
Related Questions
A family is going on a 603-mile car trip. So far they have driven 24% of the distance. Which is the best estimate for the number of miles they have driven so far?
Answers
Answer:
the best eatimated answer would be 150 miles.
24% of 603 = 144.72
so you can round it to 150
Answer:
24% of 603 is 144.72, so the best estimate would probably be 150 miles.
what is angle b in this image

Answers
Answer:
Step-by-step explanation:
you have the hypotenuse side and adjacent side, so you would use cosine (adj/hyp). since you are trying to find the angle, you use the inverse operation.
put it in the calculator and you should get 0.98176535657.
What is remainder when x3 2x² X 1 is divided by x 1?
Answers
When x^3+2x^2+x+1 is divided by (x+1) then remainder is 1.
In the given question, we have to find what is remainder when x^3+2x^2+x+1 is divided by (x+1).
To find the remainder there are two ways. First we divide the x^3+2x^2+x+1 by (x+1). Second we find the value of from (x+1) by equating (x+1) equal to zero. The put the value of x in the expression x^3+2x^2+x+1.
In this we ca easily find the remainder.
Now we firstly find the value of x;
(x+1) = 0
Subtract 1 on both side we get;
x= −1
Now put x= -1 in the expression x^3+2x^2+x+1.
x^3+2x^2+x+1 = (−1)^3+2(−1)^2+(−1)+1
x^3+2x^2+x+1 = −1+2−1+1
x^3+2x^2+x+1 = 1
Hence, when x^3+2x^2+x+1 is divided by (x+1) then remainder is 1.
To learn more about division of polynomial link is here
brainly.com/question/29718477
#SPJ4
The right question is:
What is remainder when x^3+2x^2+x+1 is divided by (x+1)?
is 627 divisible by 3
Answers
Answer:
yes.
Step-by-step explanation:
If you divide 627 by 3, you get 209 which is a whole number.
Hope this helped! Have a nice day!
Please give Brainliest when you can!
-Jaron
Answer:
technically speaking, yes. but if you don't want any remainders, (or decimals) then no.
Step-by-step explanation:
7 isnt divisable by anything, unless you have remanders or decimals, fractions ext. so yeah.
A model of the daily profits p of a gas station based on the price per gallon g is p = -15,000g2 + 34,500g – 16,800.
How much money did the company invest in gas?
Find the price that will yield the maximum profit.
What is the maximum profit?
What is the lowest price of gas needed to break even?
Answers
Answer:
Step-by-step explanation:
P = -15000g² + 34,500g - 16800
use quadratic formula to find the 2 solutions for g when P = 0
(a=-15,000, b=34500, c=-16800)
g= 0.7, g=1.6
1) company invested $16,800
2) price that yields max. p = (0.7 + 1.6)/2 = $1.15
3) max. P = -15,000(1.15)² + 34,500(1.15) - 16,800 = $3037.50
4) lowest price to break even = $0.70
A project under consideration has a 10-year projected life. The initial investment for the project is estimated to have a mean of $10,000 and a standard deviation of $1,000. The annual receipts are independent, with each year’s expected return having a mean of $1,800 and a standard deviation of $200. MARR is 12 percent. Assuming that initial investment and annual receipts are independent and normally distributed, estimate the probability that the present worth is negative using NORM.INV function in excel.
Answers
This value represents the present worth below which the probability is 0.5, indicating a negative present worth.
To estimate the probability that the present worth is negative using the NORM.INV function in Excel,
we need to calculate the present worth of the project and then determine the corresponding probability using the normal distribution.
The present worth of the project can be calculated by finding the sum of the present values of the annual receipts over the 10-year period, minus the initial investment. The present value of each annual receipt can be calculated by discounting it back to the present using the minimum attractive rate of return (MARR).
Using the given information, the present value of the initial investment is $10,000. The present value of each annual receipt is calculated by dividing the expected return of $1,800 by \((1+MARR)^t\),
where t is the year. We then sum up these present values for each year.
We can use the NORM.INV function in Excel to estimate the probability of a negative present worth. The function requires the probability value, mean, and standard deviation as inputs.
Since we have a mean and standard deviation for the present worth,
we can calculate the corresponding probability of a negative present worth using NORM.INV.
This value represents the present worth below which the probability is 0.5. By using the NORM.INV function,
we can estimate the probability that the present worth is negative based on the given data and assumptions.
Learn more about probability here:
https://brainly.com/question/31828911
#SPJ4
A national sampling of cookie preferences showed that 75% of people like chocolate chip, 50% like peanut butter, and only 3% like coconut. The use of this information makes sense in which of the following scenarios? A. The school cafeteria decides to make 3 coconut cookies for each 100 students who buy lunch, even though it puts them over budget for desserts. B. A national cookie company is thinking of changing the recipe in their peanut butter cookies. C. A national cookie company decides to spend $5 million in advertising to convince people to eat coconut cookies. D. Tom is about to open a small bakery and is using the result of the sampling to decide what kind of cookies to offer.
Answers
Answer:
D. Tom is about to open a small bakery and is using the result of the sampling to decide what kind of cookies to offer.
Step-by-step explanation:
I believe that is correct....
In the figure below, the segments FG and FH are tangent to the circle centered at O. Given that OG=8 and FH=8.4, find OF.OF=

Answers
We can conclude that they form a right triangle
So GF = FH
OF^2 = GF^2 + OG^2
OF^2 = 8.4^2 + 8^2
OF^2 = 70.56 + 64
OF^2 = 134.56
OF = 11.6
can someone help me with the picture I put above please
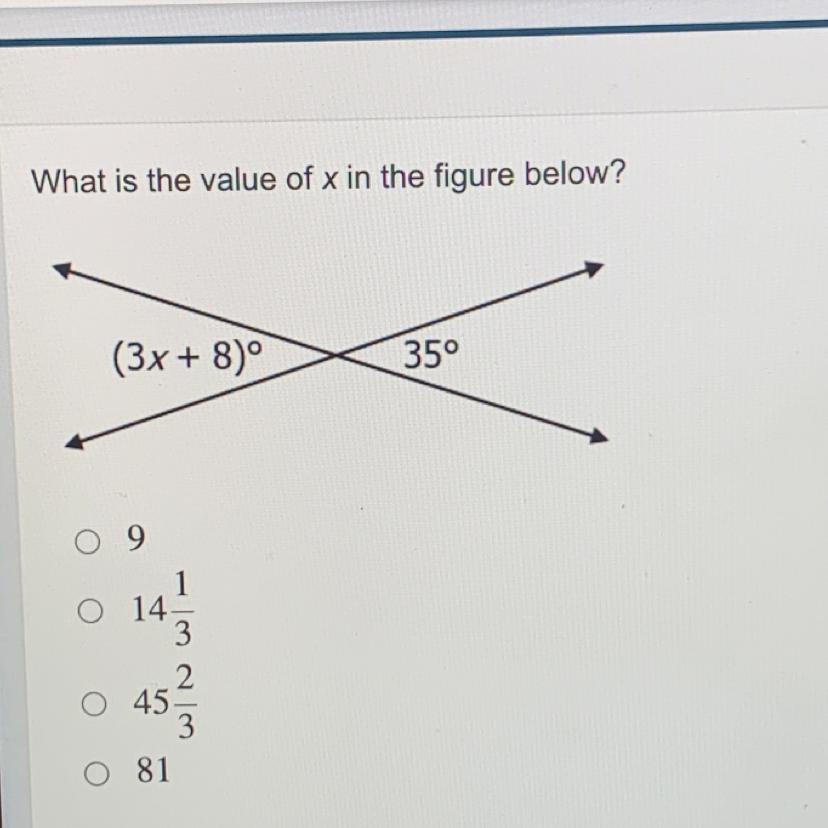
Answers
Answer:
9
Step-by-step explanation:
3x+8=35
3x=27
x=9
Solve for the variable -x=-12
Answers
Answer:
12
Step-by-step explanation:
Using the Euclidean distance between pairwise observations, which pairwise observation is most dissimilar? Observation X1 X2
1 2 2
2 5 5
3 12 1
Group of answer choices a. Observations 1 & 3 b. Observations 2 & 3 and 1 & 3 c. Observations 2 & 3 d. Observations 1 & 2 and 2 & 3
Answers
Using the Euclidean distance between pairwise observations, the pairwise observation is most dissimilar is Observations 1 & 3. The correct answer is A.
In order to determine the most dissimilar pairwise observation using the Euclidean distance, we need to calculate the distance between each pair of observations. The Euclidean distance between two observations is the square root of the sum of the squared differences between their corresponding values in each variable.
For example, for observations 1 and 2:
d(1,2) = √((2-5)² + (22-21)²) = √(9 + 1) = √(10)
We can calculate the distances between all pairs of observations and then identify the pair with the largest distance as the most dissimilar.
d(1,2) = √(10)
d(1,3) = √((2-12)² + (22-1)²) = √(1400)
d(2,3) =√((5-12)² + (53-1)²) = √(1865)
Therefore, the most dissimilar pairwise observation is between observations 1 and 3 with a distance of √(1400). The correct answer is A.
Learn more about euclidian distance at https://brainly.com/question/30288897
#SPJ11
Using 20 boxes of nails a carpenter was able to finish 40 bird houses. Write an
equation that can be used to express the relationship between the total number of
birdhouses completed(t)and the boxes of nails(b) used.
Help ASAP!
Answers
Answer: 20 times 2 equal 40/ 40 divided by 2 is 20
Step-by-step explanation: Both of them should help you find the answer
a summer squash, spinach, and leek frittata recipe requires 6 ounces of spinach. if the ep unit cost of the ingredient is $2.25 per pound, what is the total cost of the ingredient?
Answers
The total cost of the ingredient is $0.84 for 6 ounces.
Define fixed cost.
The cost or expense known as fixed cost is unaffected by a short-term horizon's growth or reduction in the number of units produced or sold. To put it another way, it refers to a cost that is linked to a time period rather than a specific economic activity.
It can be viewed as costs that a business must pay regardless of the volume of business activity, such as the number of units produced or sales made. One of the two main parts of the overall manufacturing cost is the fixed cost.
Solution Explained:
Given,
1 pound = $2.25
Since 1 pound = 16 ounces
So, 16 ounces = 2.25
1 ounce = 2.25/16 = 0.14
Therefore, 6 ounces = 0.14 X 6 = 0.84
To learn more about fixed cost, use the link given
https://brainly.com/question/24868261
#SPJ4
Can y’all help me find the width

Answers
Answer:
Step-by-step explanation:
To find the width, you have to divide the height and length by the volume
Solve eight and three fifths minus two and four ninths.
Answers
Answer:
6.15555555556
Step-by-step explanation:
the 5 is infinite in 6.15 such as 6.155555555 it does not stop
Simplify the following expression. 2x^5+3x^3-5x^2+x^2+7x+1+7x^5-3x^3-4
Answers
Option (A) is the correct option and on simplifying the expression by combining like terms we get,
24 - 3p.
Here, we have,
Given, the expression 6(4 - 2p) + 9p
On simplifying, we get
24 - 12p + 9p
24 - 3p
So, on simplifying the given expression, we get
24 - 3p
Hence, Option (A) is the correct option and on simplifying the expression by combining like terms we get,
24 - 3p
Learn more about Algebraic Expressions here brainly.com/question/24267361
#SPJ1
How do you calculate
√25+√16-√49 in BODMAS
Answers
\( \sqrt{25} = 5 \\ \sqrt{16 } = 4 \\ \sqrt{49} = 7\)
5+4-7 = 9-7 = 2
Our Answer 2
Answer:
The answer is 2
Step-by-step explanation:
√25 = 5
√16 = 4
√49 = 7
Now,
√25 + √16 - √49
5 + 4 - 7
9 - 7 = 2
Thus, The answer is 2
-TheUnknownScientist
2x - 3 = 1 and -3x + 6 = 12 like plz I'm really tired
Answers
The displacement, s metres, of a cari seconds after it starts from a fixed point A is given by 45-50 (a) Find an expression for its velocity (in ms) alteri seconds. (b) Find the acceleration (in ms) at A
Answers
(a) The expression for the velocity after I second is -50i m/s.
(b) Since we want the acceleration at point A, we need to evaluate this expression at t = 0:
a(A) = a(0) = -50 m/s^2
Thus, the acceleration at point A is -50 m/s^2.
To find the expression for the velocity and acceleration of the car, we need to use the given displacement equation:
s(t) = 45 - 50t
(a) To find the velocity (v) after t seconds, we need to differentiate the displacement equation with respect to time (t):
v(t) = ds/dt
Differentiating s(t) with respect to t:
v(t) = -50
So, the velocity of the car after t seconds is -50 m/s.
(b) To find the acceleration (a) at point A, we need to differentiate the velocity equation with respect to time (t):
a(t) = dv/dt
Since the velocity equation is a constant (-50 m/s), its derivative with respect to time is:
a(t) = 0
So, the acceleration of the car at point A is 0 m/s².
Learn more about Second:
brainly.com/question/29020369
#SPJ11
What is 4/7−2/5 ??????????????????????????
Answers
Answer:
6/35
Step-by-step explanation:
20/35 - 14/35= 6/35
Answer:
0.1214 or 6/35
Step-by-step explanation:
didn't know if they were whole numbers with division signs or fractions
A hiker walks 15.3 miles to the east in 4.8 hours then turns around and walks 6.2 miles to the west in 2.7 hours. What was her average velocity during the trip
Answers
Velocity is defined as the rate at which an object changes position. It is a vector quantity that specifies the speed and direction of the motion of an object.
Step 1: Find the total displacement Total displacement = displacement to the east - displacement to the west Displacement to the east = 15.3 miles Displacement to the west = 6.2 miles Total displacement = 15.3 - 6.2 = 9.1 miles The hiker had a total displacement of 9.1 miles.
Step 2: Find the total time taken Total time taken = time taken to walk to the east + time taken to walk to the west Time taken to walk to the east = 4.8 hours Time taken to walk to the west = 2.7 hours Total time taken = 4.8 + 2.7 = 7.5 hours The hiker took a total time of 7.5 hours.
Step 3: Find the average velocity Average velocity = total displacement / total time taken Average velocity = 9.1 miles / 7.5 hours Average velocity = 1.213 miles per hour Therefore, the average velocity of the hiker during the trip was 1.213 miles per hour.
To know more about Velocity visit:
https://brainly.com/question/18084516
#SPJ11
Determine whether the series converges or diverges. (n+4)! a) 4!n!4" b) 1 \n(n+1)(n+2) =
Answers
We have to determine whether the given series converges or diverges. The given series is as follows: `(n+4)! / 4!(n!)` Let's use the ratio test to find out if this series converges or diverges.
The Ratio Test: It is one of the tests that can be used to determine whether a series is convergent or divergent. It compares each term in the series to the term before it. We can use the ratio test to determine the convergence or divergence of series that have positive terms only. Here, a series `Σan` is convergent if and only if the limit of the ratio test is less than one, and it is divergent if and only if the limit of the ratio test is greater than one or infinity. The ratio test is inconclusive if the limit is equal to one. The limit of the ratio test is `lim n→∞ |(an+1)/(an)|` Let's apply the Ratio test to the given series.
`lim n→∞ [(n+5)! / 4!(n+1)!] * [n!(n+1)] / (n+4)!` `lim n→∞ [(n+5)/4] * [1/(n+1)]` `lim n→∞ [(n^2 + 9n + 20) / 4(n^2 + 5n + 4)]` `lim n→∞ (n^2 + 9n + 20) / (4n^2 + 20n + 16)`
As we can see, the limit exists and is equal to 1/4. We can say that the given series converges. The series converges. To determine the convergence of the given series, we use the ratio test. The ratio test is a convergence test for infinite series. It works by computing the limit of the ratio of consecutive terms of a series. A series converges if the limit of this ratio is less than one, and it diverges if the limit is greater than one or does not exist. In the given series `(n+4)! / 4!(n!)`, the ratio test can be applied. Using the ratio test, we get: `
lim n→∞ |(an+1)/(an)| = lim n→∞ [(n+5)! / 4!(n+1)!] * [n!(n+1)] / (n+4)!` `= lim n→∞ [(n+5)/4] * [1/(n+1)]` `= lim n→∞ [(n^2 + 9n + 20) / 4(n^2 + 5n + 4)]` `= 1/4`
Since the limit of the ratio test is less than one, the given series converges.
The series converges to some finite value, which means that it has a sum that can be calculated. Therefore, the answer is a).
To learn more about ratio test visit:
brainly.com/question/31856271
#SPJ11
Eli needs pieces of ribbon cut into strips that are 2 1/2 feet long. The roll of ribbon is 7 1/2 feet long. Which expression could be one of the steps in determining how many strips can be cut?
Answers
Hi there! 2 ½ is equivalent to 2.5 and 7 ½ is equivalent to 7.5. An expression that can be used to find out how many strips can be cut is this:
2.5x = 7.5
This is because the length of the ribbon needed to be cut is 2.5 ft long and we are looking for how many of that length could be cut out of a roll of ribbon that is 7.5ft long. All you have to do for this is divide both sides by 2.5 to isolate the variable. 7.5/2.5 is 3. x = 3. Eli can cut 3 strips of 2½ ft long ribbon.
2 + x 1. Let f(x) = 1- x (a) (2 marks) Use the definition of derivative to find the derivative of f(x) at x = 2. (b) (2 marks) Hence or otherwise, determine the equation of the tangent line to f(x) at x = 2.
Answers
The derivative of the function f(x) = 1 - x at x = 2 is -1. Therefore, the equation of the tangent line to f(x) at x = 2 is y = -1(x - 2) + f(2).
(a) To find the derivative of f(x) at x = 2 using the definition of the derivative, we start by calculating the difference quotient:
f'(2) = lim(h→0) [f(2 + h) - f(2)] / h.
Substituting the given function f(x) = 1 - x into the difference quotient, we have:
f'(2) = lim(h→0) [1 - (2 + h)] / h.
Simplifying this expression, we get:
f'(2) = lim(h→0) (1 - 2 - h) / h
= lim(h→0) (-1 - h) / h.
Next, we can apply algebraic manipulation to simplify the expression further:
f'(2) = lim(h→0) (-1/h - 1).
Taking the limit as h approaches 0, the first term (-1/h) approaches negative infinity, and the second term (-1) remains constant. Therefore, the derivative of f(x) at x = 2 is -1.
(b) The equation of the tangent line to f(x) at x = 2 can be determined using the point-slope form of a line. We know that the point (2, f(2)) lies on the tangent line. Substituting x = 2 into the given function, we find f(2) = 1 - 2 = -1.
Using the point-slope form, y - y1 = m(x - x1), where (x1, y1) is the given point (2, -1) and m is the slope of the tangent line (-1), we can write the equation of the tangent line as:
y - (-1) = -1(x - 2).
Simplifying this equation, we get:
y + 1 = -x + 2
y = -x + 1.
Therefore, the equation of the tangent line to f(x) at x = 2 is y = -x + 1.
Learn more about equation of the tangent line:
https://brainly.com/question/6617153
#SPJ11
19. Sketch the graph of x² + y² + 4x - 2y - 31 = 0.
Write the center and radius under the graph. (show all work)

Answers
For given equation of circle radius is 6 unit and center is at (-2,1).
What is a circle?
A circle is a special kind of ellipse in which the eccentricity is zero and the two foci are coincident. A circle is also termed as the locus of the points drawn at an equidistant from the center. The distance from the center of the circle to the outer line is its radius. Diameter is the line which divides the circle into two equal parts and is also equal to twice of the radius.
The equation of circle in the plane is given as:
(x-h)^2 + (y-k)^2 = r^2
where (x, y) are the coordinate points
(h, k) is the coordinate of the center of a circle
and r is the radius of a circle.
Now,
As shown in graph the graph by taking two points on graph
(-2,7) and (-2,-5)
Center will be at (-2-2)/2, (7-5)/2
i.e. (-2,1)
And for radius take magnitude of points from y-coordinate
(7+5)/2=6 unit
To know more about Circles visit the link
https://brainly.com/question/11833983?referrer=searchResults
#SPJ1

In the figure there are 5 equal rectangles and each of its sides is marked with a number as indicated in the drawing. Rectangles are placed without rotating or flipping in positions I, II, III, IV, and V in such a way that the sides that stick together in two rectangles have the same number. Which of the rectangles should go in position I?

Answers
The rectangle which should go in position I is rectangle A.
We are given that;
The rectangles A,B,C and D with numbers
Now,
To take the same the number of side
If we take A on 1 place
F will be on second place
And B will be on 4th place
Therefore, by algebra the answer will be rectangle A.
More about the Algebra link is given below.
brainly.com/question/953809
#SPJ1
A gallon of milk cost $2.90 in 2002. In 2018, it cost
$3.80. what is the percent change pf the cost of a gallon
of milk from 2002 to 2008?
Answers
Answer:
The cost of a gallon of milk changed 31%
Step-by-step explanation:
Percent Change
Is the difference between two values of the same variable in different times or conditions, expressed as a percentage of the original value.
The formula is:
\(\displaystyle Pc=\left |\frac{v1-v2}{v1} \right |\cdot 100\%\)
Where
v1 = original value
v2 = final value
The gallon of milk cost v1=$2.90 in 2002 and v2=$3.80 in 2018. The percent change is:
\(\displaystyle Pc=\left |\frac{2.90-3.80}{2.90} \right |\cdot 100\%\)
\(\displaystyle Pc=\left |\frac{-0.9}{2.90} \right |\cdot 100\%\)
Pc = 31%
The cost of a gallon of milk changed 31%
a study of the career paths of hotel general managers sent questionnaires to a simple random sample of 290 hotels belonging to major u.s. hotel chains. there were 181 responses. the average time these 181 general managers had spent with their current company was 10.43 years. (take it as known that the standard deviation of time with the company for all general managers is 4.5 years.) (a) what is the needed z -critical value (to two decimal places) for a 85% confidence interval to estimate the mean time a general manager had spent with their current company? (b) find the margin of error for an 85% confidence interval to estimate the mean time a general manager had spent with their current company: years (c) find the margin of error for a 99% confidence interval to estimate the mean time a general manager had spent with their current company: years
Answers
The margin of error for a 99% confidence interval to estimate the mean time a general manager had spent with their current company is approximately 2.80 years.
(a) The z-critical value for an 85% confidence interval is approximately 1.44.
(b) The margin of error for an 85% confidence interval is approximately 1.19 years.
(c) The margin of error for a 99% confidence interval is approximately 2.80 years.
To find the needed values for the confidence intervals, we can use the formula: Margin of Error = z * (standard deviation / square root of sample size)
(a) To find the z-critical value for an 85% confidence interval, we need to find the z-score corresponding to an area of 0.85 in the standard normal distribution table. The z-critical value for an 85% confidence interval is approximately 1.44.
(b) For an 85% confidence interval, we can calculate the margin of error using the given standard deviation of 4.5 years and the sample size of 181: Margin of Error = 1.44 * (4.5 / sqrt(181)) ≈ 1.19 years
Therefore, the margin of error for an 85% confidence interval to estimate the mean time a general manager had spent with their current company is approximately 1.19 years.
(c) Similarly, for a 99% confidence interval, we can calculate the margin of error using the same standard deviation and sample size: Margin of Error = 2.58 * (4.5 / sqrt(181)) ≈ 2.80 years
Hence, the margin of error for a 99% confidence interval to estimate the mean time a general manager had spent with their current company is approximately 2.80 years.
Learn more about Margin of Error here:
brainly.com/question/29100795
#SPJ11
what is an coefficient? I bet you guys on here could explain it better and how do I find out what the coefficients are?

Answers
Answer: 3 and 19
Whoops, I'm a bit rusty, but numbers are also terms. Hope that also helps.

Caleb an investment banker sold his shares for $18,189.27 when there was a boom in the stock market. Calculate the amount he paid for the shares if his selling price was 130% of the amount he paid for the shares.
Answers
Therefore, Caleb paid approximately $14,067.90 for the shares.
Let's assume the amount Caleb paid for the shares is represented by the variable "x". According to the given information, his selling price was 130% of the amount he paid.
Selling price = 130% of the amount paid
$18,189.27 = 1.3 * x
To find the amount he paid for the shares, we can solve the equation for "x" by dividing both sides by 1.3:
x = $18,189.27 / 1.3
Calculating this, we find:
x ≈ $14,067.90
To know more about paid,
https://brainly.com/question/14549389
#SPJ11