If one of the roots of the equation x² – 4x + k = 0 exceeds the other by 2, then find the roots and determine the value of k.
Answers
Step-by-step explanation:
the solutions for a quadratic equation is
x = (-b ± sqrt(b² - 4ac))/(2a)
in our case
a = 1
b = -4
c = k
x = (4 ± sqrt(16 - 4k))/2 = 2 ± sqrt(4 - k)
x1 = 2 + sqrt(4 - k)
x2 = 2 - sqrt (4 - k)
x1 = 2 + x2
2 + sqrt(4 - k) = 2 + 2 - sqrt(4 - k)
2×sqrt(4 - k) = 2
sqrt(4 - k) = 1
4 - k = 1
k = 4 - 1 = 3
x1 = 3
x2 = 1
Answer:
The roots are 1 and 3.
k = 3.
Step- by-step explanation:
We use the facts that if α and β are the roots of ax^2 + bx + c = o then α+ β = -b/a and α β = c/a.
The roots are written as α and α+2, then:
α + α + 2 = -(-4)
2α + 2 = 4
α + 1 = 2
α = 1
also
α (α + 2) = k
Substituting for α:
1(1 + 2) = k
k = 3.
The roots are 1 and 1 + 2 = 3.
Related Questions
Write a rule to describe this translation. (photo attached)

Answers
The rule for given translation is (x,y)→(x + 3, y - 4).
What is Transformation?In mathematics, the phrases translation, rotation, and reflection are all used to describe the change of forms. This refers to a form moving beyond a mirror line or a fixed point. While being translated, rotated, or reflected, the shape doesn't change. The constant rotation of a shape around a fixed point or across the mirror line is known as translation. Rotation defines the way a form moves about a central axis. Shapes can be rotated by a specific number of degrees either clockwise or counterclockwise.
The rule for translation of given figure will be,
We need to move x for 3 units to left and y for 4 units downwards to get the translation, so the rule is:
(x,y)→(x + 3, y - 4).
Therefore, the rule for given translation is (x,y)→(x + 3, y - 4).
To learn more about Translation and Transformation visit here:
https://brainly.com/question/12861087
#SPJ1
Let n=3. Suppose K=Z 2
3
, and let B k
:Z 2
3
→Z 2
3
such that B k
(x)=k+x. (a) Suppose the block cipher is Output Fecdback Mode. Encrypt 111000101 when the key is 101 and the initial state is s=000. (b) Suppose the block cipher is Block Chaining. Encrypt 111000101 when the key is 101 and the priming value ius y 0
=000
Answers
The ciphertext for 111000101 when the key is 101 and the initial state is s = 000, using Output Feedback Mode is 101100001 and using Block Chaining is 111011111 is the answer.
Given n = 3. Let K = Z23, and Bk: Z23 → Z23 such that Bk(x) = k + x. Now, we have to encrypt 111000101 using the key 101 and the initial state s = 000, using Output Feedback Mode.
Let us discuss it: Output Feedback Mode Encryption: At first, convert the initial state s to a 3-bit integer. Then, compute the first ciphertext bit c1 as the first bit of the state s XOR with the first bit of the plaintext m1.
Now, compute s1 as (s/2) ⊕ c1 and then compute the second ciphertext bit c2 as the second bit of s1 XOR the second bit of the plaintext m2.
Similarly, compute s2 as (s1/2) ⊕ c2 and then compute the third ciphertext bit c3 as the third bit of s2 XOR the third bit of the plaintext m3.
Continuing in this way, we get the ciphertext, which is 101100001. So, the ciphertext for 111000101 when the key is 101 and the initial state is s = 000, using Output Feedback Mode is 101100001.
Block Chaining Encryption:
In Block Chaining, we use the priming value to generate the ciphertext.
For the given plaintext, we have to start with y0 = 000, the priming value.
Then, compute z1 = Bk(y0) XOR m1.
Similarly, we get the next ciphertext as zi = Bk(yi-1) XOR mi and yi = zi-1, where i = 2,3,...,9.
So, we get the ciphertext as 111011111 for 111000101 when the key is 101 and the priming value is y0 = 000, using Block Chaining.
Hence, the ciphertext for 111000101 when the key is 101 and the initial state is s = 000, using Output Feedback Mode is 101100001 and using Block Chaining is 111011111.
know more about Block Chaining
https://brainly.com/question/32790194
#SPJ11
A homeowner is building a circular fire pit in his backyard. He plans to outline the pit with bricks and cover the space inside the pit with sand. The homeowner has decided to build the pit with a diameter of 3 feet.
In order to know how many bricks to buy, the homeowner must know the distance around the outside of the pit. Calculate both the exact distance and the approximate distance.
In order to know how much sand to buy, the homeowner must know how much space needs to be covered inside the pit. Calculate both the exact area and the approximate area.
Answers
Answer:
To calculate the distance around the outside of the circular fire pit, also known as the circumference:
Exact distance:
Circumference = pi x diameter
C = pi x 3 feet
C = 3.14 x 3 feet
C = 9.42 feet
Approximate distance:
Circumference = 2 x radius x pi
Radius = diameter / 2 = 1.5 feet
C = 2 x 1.5 feet x 3.14
C = 9.42 feet
Therefore, the exact distance around the outside of the circular fire pit is 9.42 feet, and the approximate distance is also 9.42 feet.
To calculate the area inside the circular fire pit:
Exact area:
Area = pi x (radius)^2
Area = 3.14 x (1.5 feet)^2
Area = 7.07 square feet
Approximate area:
Area = (diameter / 2)^2 x pi
Area = (1.5 feet)^2 x 3.14
Area = 7.065 square feet
Therefore, the exact area inside the circular fire pit is 7.07 square feet, and the approximate area is 7.065 square feet.
ratio of triangle 9:8:7, permimeter is 144 , find the measure of each side of the triangle
Answers
Step-by-step explanation:
There are (9+8+7) = 24 parts that add up to 144
each part is then 144/24 = 6
then each side is 9 parts = 9*6 = 54
8 parts = 8 * 6 = 48
7 parts 7 * 6 = 42
Situation: a 40 gram sample of a substance that’s used for drug research has a k-value of 0.1472. N=N0e(-kt)
Find the substance’s half-life, in days. Round your answer to the nearest tenth
Answers
Rounding to the nearest tenth, the substance's half-life is approximately 4.7 days.
To find the substance's half-life, we can use the formula N = N0 * e^(-kt), where:
N is the final amount of the substance,
N0 is the initial amount of the substance,
k is the decay constant,
t is the time in days.
In this case, the half-life represents the time it takes for the substance to decay to half of its initial amount. So, we have N = N0/2.
Substituting these values into the formula, we get:
N0/2 = N0 * e^(-k * t)
Dividing both sides by N0 and simplifying, we have:
1/2 = e^(-k * t)
To isolate t, we can take the natural logarithm (ln) of both sides:
ln(1/2) = -k * t
Since ln(1/2) is the natural logarithm of 1/2 (approximately -0.6931), we can rewrite the equation as:
-0.6931 = -k * t
Dividing both sides by -k, we find:
t = -0.6931 / k
Substituting k = 0.1472 (given), we have:
t = -0.6931 / 0.1472 ≈ -4.7121
Since time cannot be negative, we take the absolute value:
t ≈ 4.7121
Rounding to the nearest tenth, the substance's half-life is approximately 4.7 days.
For more questions on Rounding
https://brainly.com/question/28128444
#SPJ8
Suppose 52% of the doctors in America are dentists. If a random sample of size 775 is selected, what is the probability that the proportion of doctors who are dentists will differ from the population proportion by less than 5%? Round your answer to four decimal places.
Answers
Answer:
0.134%
Step-by-step explanation:
We have to
sd * P = [p * (1 - p) / n] ^ (1/2)
where p = 0.52 and n = 775, replacing:
sd * P = [0.52 * (1 - 0.52) / 775] ^ (1/2) = 0.0179
Now the probability would be:
P = 1 - P [(- 0.05) /0.0179 <z <(0.05) / 0.0179]
P = 1 - P (-2.79 <z <2.79)
P = 1 - P (z <2.79) - P (z <-2.79)
P = 1 - 0.9974 - 0.00126
P = 1 - 0.99614
P = 0.00134
Therefore the probability is 0.134%

Together a chair, a table, and a lamp cost $572. The chair costs 4 times as much as the lamp, and the table costs $23 less than the chair. Calculate the cost of the table
Answers
Answer:
241.44 maybe the number 572 is wrong? is strange you get decimals for such a simple problem
Step-by-step explanation:
chair=c
lamp=l
table=a
we know everything costs 572 dollars
a+l+c=572
c=4*l so the next equations is a+l+4l=572 or a+5l=572
but a=4*l-23
next equation will be
4l-23+5l=572
9l=572+23
9l=595
lamp=66.11 chair=264.44
table=264.44-23=241.44
if a cubic box (all sides the same length) has a volume of 1.0 l, what is the length of each side of the box in cm?
Answers
The length of each side of the box is 10 centimeter.
Volume is the amount of space occupied by a three-dimensional figure as measured in cubic units.
Given,
The volume of the cubic box = 1 liter
We know 1 liter= 1000 cubic centimeter
Volume of the cubic box= \(x^3}\)
Then,
\(x^{3}=1000\\ x=\sqrt[3]{1000}\)
x=10 centimeter
Hence, the length of each side of the box is 10 centimeter.
Learn more about volume here
brainly.com/question/1578538
#SPJ4
A. A population of values has a normal distribution with μ=208.5 and σ=35.4. You intend to draw a random sample of size n=236.
Find the probability that a single randomly selected value is greater than 203.4.
P(X > 203.4) = Round to 4 decimal places.
Find the probability that the sample mean is greater than 203.4.
P(X¯¯¯ > 203.4) = Round to 4 decimal places.
B. A population of values has a normal distribution with μ=223.7 and σ=56.9. You intend to draw a random sample of size n=244.
Find the probability that a single randomly selected value is between 217.5 and 234.6.
P(217.5 < X < 234.6) = Round to 4 decimal places.
Find the probability that the sample mean is between 217.5 and 234.6.
P(217.5 < X¯¯¯ < 234.6) = Round to 4 decimal places.
Answers
A. Using the given information, we can standardize the value 203.4 using the formula \(z = (X - μ) / σ\), where X is the value of interest, μ is the mean, and σ is the standard deviation.
Thus, we get: \(z = (203.4 - 208.5) / 35.4 = -0.14407\)
Using a standard normal distribution table or calculator, we can find the probability that a randomly selected value is greater than \(203.4\):
\(P(X > 203.4)\) = \(P(Z > -0.14407)\) = \(0.5563\) (rounded to 4 decimal places)
To find the probability that the sample mean is greater than 203.4, we need to use the central limit theorem, which states that the sample mean of a large enough sample size (n >= 30) from a population with any distribution has a normal distribution with mean μ and standard deviation σ / sqrt(n). Thus, we get:
\(z = (203.4 - 208.5) / (35.4 / sqrt(236))\)\(= -1.3573\)
Using a standard normal distribution table or calculator, we can find the probability that the sample mean is greater than 203.4:
\(P(X¯¯¯ > 203.4) = P(Z > -1.3573)\)= \(0.0867\) (rounded to 4 decimal places)
B. Using the given information, we can standardize the values \(217.5\) and \(234.6\) using the same formula as before. Thus, we get:
\(z1 = (217.5 - 223.7) / 56.9\) \(= -0.10915\)
\(z2 = (234.6 - 223.7) / 56.9 = 0.19235\)
Using a standard normal distribution table or calculator, we can find the probability that a randomly selected value is between 217.5 and 234.6:
\(P(217.5 < X < 234.6) = P(-0.10915 < Z < 0.19235) = 0.2397\) (rounded to 4 decimal places)
To find the probability that the sample mean is between 217.5 and 234.6, we can use the same formula as before, but with the sample size and population parameters given in part B. Thus, we get:
\(z1 = (217.5 - 223.7) / (56.9 / sqrt(244)) = -1.0784\)
\(z2 = (234.6 - 223.7) / (56.9 / sqrt(244)) = 1.7256\)
Using a standard normal distribution table or calculator, we can find the probability that the sample mean is between 217.5 and 234.6:
\(P(217.5 < X¯¯¯ < 234.6) = P(-1.0784 < Z < 1.7256)\)= \(0.8414\)(rounded to 4 decimal places)
To learn more about standard normal distribution, visit here
https://brainly.com/question/29509087
#SPJ4
A group of hikers begins a trip with 30 pounds of food per hiker. They will eat a total of 12 pounds of food a day. If there are 7 hikers, which equation can be used to find y, the amount of food remaining after x days? F) y = 12x + 30 G) y = -7x + 84 H) y = 12x + 210 J) y = 30x-12
Answers
Given:
Food = 30 pounds of food per hiker.
Food consumption = 12 pounds of food a day.
No. of hikers = 7
To find:
The equation for the given situation.
Solution:
Let x be the number of days and y be the amount of remaining food.
Total amount is the product of number of hikers and food per hiker.
\(\text{Total food}=7\times 30\)
\(\text{Total food}=210\)
Food consumption of one day = 12 pounds
Food consumption of x days = 12x pounds
Now,
Remaining food = Total food - Food consumption of x days
\(y=210-12x\)
\(y=-12x+210\)
Therefore, the required equation is \(y=-12x+210\).
Note: Option C is correct if there is -12 instead of 12.
please help hghjhghjjgfhjjjfhgfhbfhgkfgh

Answers
Answer:
Hello there! I would say the answer is C,
Step-by-step explanation:
I hope this helps!!!
what is the distance of LM

Answers
Answer
(-4,3)
Step-by-step explanation:
2. if a sample has a mean of 100 and standard deviation of 6, what value would correspond the the z-score of 2?
Answers
The z-score of 2 for a sample has a mean of 100 and a standard deviation of 6 is 112.
The value that would correspond to the z-score of 2 for a sample with a mean of 100 and standard deviation of 6 can be calculated using the formula for z-score:
z = (x - mean) / standard deviation
In this case, we want to find the value of x that corresponds to a z-score of 2. We can rearrange the formula to solve for x:
x = (z * standard deviation) + mean
Substituting the values given in the question:
x = (2 * 6) + 100
x = 12 + 100
x = 112
Therefore, the value that corresponds to the z-score of 2 for this sample is 112.
For more information about standard deviation, visit:
https://brainly.com/question/475676
#SPJ11
WHAT DOES C=???
QUESTION BELOW

Answers
================================================
Work Shown:
M = number of miles
L = cost to rent a luxury car = 1.15M + 16.40
E = cost to rent an economy car = 0.80M+10.95
C = difference in price
C = L - E
C = (1.15M + 16.40) - (0.80M+10.95)
C = 1.15M + 16.40 - 0.80M-10.95
C = 0.35M + 5.45
Don't forget to distribute the negative to each term in the second parenthesis.
-------------------
Optional Section:
Let's say you drove M = 100 miles as an example.
The luxury car would cost L = 1.15M+16.40 = 1.15*100+16.40 = 131.40 dollars to rent.
The economy car would cost E = 0.80M+10.95 = 0.80*100+10.95 = 90.95 dollars to rent.
The difference in rental costs is L-E = 131.40-90.95 = 40.45 dollars.
Or you could say C = 0.35M+5.45 = 0.35*100+5.45 = 40.45
This means you pay $40.45 more if you go for a luxury car instead of an economy car.
A 10% increase in cigarette prices has been found to result in a _____ decrease of smokers. a. 2% b. 4% c. 10% d. 20% please select the best answer from the choices provided. a b c d
Answers
A 10% increase in cigarette prices has been found to result in a 4% decrease of smokers.
What is the effect of price increase in the demand for cigarettes?An increase in cigarette price will result in a decrease in the demand for cigarettes.
Since more money needs to be spent to but the same product, less consumers can be able to afford it.
Therefore, a 10% increase in cigarette prices has been found to result in a 4% decrease of smokers.
Learn more about increase in price at: https://brainly.com/question/26526671
#SPJ4
Please help!!! It's for a timed quiz on conditional probabilities!!!
A researcher randomly surveyed 122 college professors to determine what types of courses they teach and their sleeping habits. The two-way table displays the data.
Suppose a survey respondent is randomly selected. Let M = professor teaches math and B = professor is an early bird. What is the value of P(B|M)?
a. 17/63
b. 16/59
c. 16/33
d. 21/34

Answers
Answer:
A
Step-by-step explanation:
A
8x2y + qxy2 + 41XY2 - 570X2Y
+
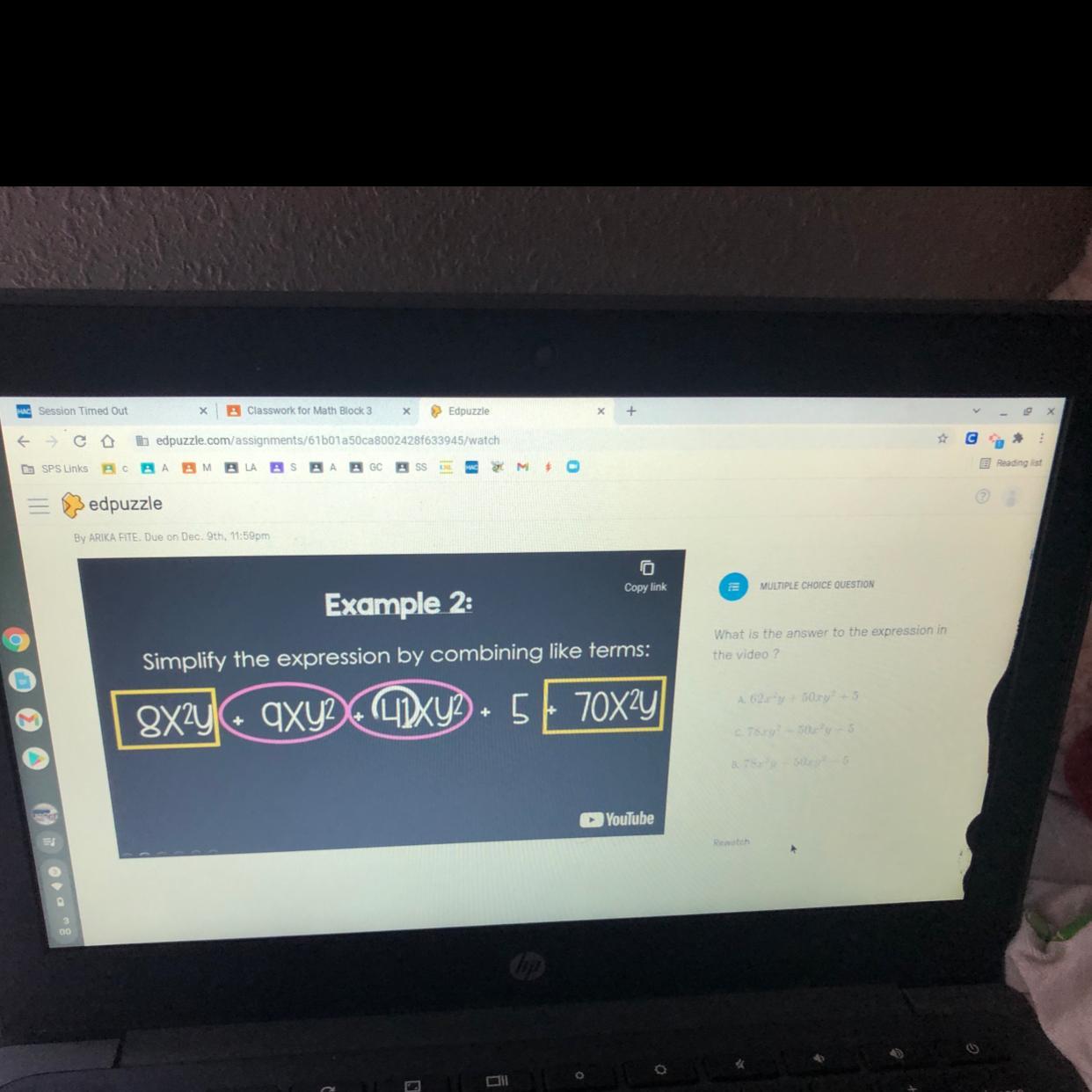
Answers
Answer:
16y+qy
2
+41Y
2
−1140Y
Step-by-step explanation:
Pasos de la solución
8×2y+q×y2+41×Y2−570×2Y
Multiplica 8 y 2 para obtener 16.
16y+qy
2
+41Y
2
−570×2Y
Multiplica 570 y 2 para obtener 1140.
16y+qy
2
+41Y
2
−1140Y
Which polynomial functions are written in standard form? Select all that apply.
f(x) = 8 – x5
f(x) = –3x5 + 5x – 2
f(x) = 2x5 + 2x + x3
f(x) = x3 – 8x2
Answers
The correct options are (b) f(x)=-3x⁵+5x-2
(d) f(x)=x³-8x²
How the polynomial functions are written in standard form?The function are written in standard form by writing the highest degree term at first and then writting other term according to decreasing order of degree of the variable.
for example f(x)=x⁴+x³+x²+x+1
So according to the question
the function should be written in standard form
so by checking every option,
(a) f(x)=8-x⁵ here highest power is 5 but that term is present at second term. so option A is incorrect.
(b) f(x)=-3x⁵+5x-2 here highest power is 5 and that term is present at first term. then all the terms are arranged in decrreasing order of power.
(c) f(x)=2x⁵+2x+x³ here highest power is 5 and that term is present at first term. then the second highest powered term is 3 and that term is present at third position which should be present at second position.
(d) f(x)=x³-8x² here here highest power is 3 and that term is present at first term. then all the terms are arranged in decrreasing order of power.
Therefore, The correct options are
(b) f(x)=-3x⁵+5x-2
(d) f(x)=x³-8x²
Learn more about the polynomial in standard form
here: https://brainly.com/question/194303
#SPJ2
Answer: The first answers are B. and D.
The second answer is 5
Step-by-step explanation: The third answer is -3
The area of castles roof is 985 square feet.If shingles cost $12.50 per square foot, how much money would a cost to completely cover the roof?
Answers
Answer:
Step-by-step explanation:
Since there are 985 square feet to cover, and it costs 12.50 per square foot, you multiply 12.50 by 985 to get 12,312.50, the total cost and answer.
please answer asap!:)

Answers
Maggie's total benefit of consuming n scoops of ice-cream is TB(n)=84n−n ^2
, in which n can only be integers. Suppose the price of ice-cream is $27.5 per scoop. We would expect Maggie to consume [ Answer04 ] scoops of ice-cream.
Answers
Thus, we would expect Maggie to consume 0 scoops of ice cream.
To determine how many scoops of ice cream Maggie is expected to consume, we need to find the value of n that maximizes Maggie's total benefit function, TB(n) = 84n - n^2.
Since the price of ice cream is $27.5 per scoop,
we can equate Maggie's total benefit to the cost of the ice cream: 84n - n^2 = 27.5n.
Simplifying the equation, we have: n^2 - 56.5n = 0.
Factoring out an n, we get: n(n - 56.5) = 0.
Therefore, n = 0 or n = 56.5.
However, since n can only be an integer, Maggie cannot consume 56.5 scoops of ice cream.
Know more about the benefit function,
https://brainly.com/question/29418750
#SPJ11
What is 12x³ – 9x² – 4x + 3 in factored form? hurry...
Answers
Answer:
Combine Like Terms:
=1728+−9x^2+−4x+3
=(−9x^2)+(−4x)+(1728+3)
= −9x^2+−4x+1731
At a zoo, youth ticket cot five dollar an adult ticket cot nine dollar a group purchaed ex youth ticket NY adult ticket pent $90 on ticket what i the domain of the relationhip?
Answers
The domain of this relationship would be all possible combinations of values for x and y that satisfy the equation 5x + 9y = 90.
To find the equation:
The domain of the relationship in this scenario is the number of youth and adult tickets that were purchased by the group.
It is given that the cost of a youth ticket is $5 and the cost of an adult ticket is $9. Therefore, the number of youth and adult tickets can be represented by the variables x and y respectively.
We also know that the group spent a total of $90 on tickets, so the equation representing the relationship between the number of youth and adult tickets purchased would be:
5x + 9y = 90
So the domain of this relationship would be all possible combinations of values for x and y that satisfy the equation 5x + 9y = 90.
It's important to note that the domain would be all non-negative integers of x and y as the number of youth and adult tickets cannot be negative numbers.
To learn more about the variables, visit:
brainly.com/question/29583350
#SPJ4
A turtle ambles leisurely, as turtles tend to do, when it moves from a location with position vector r
1,x
=1.07 m and r
1,y
=−2.69 m in a lettuce garden to another location, with position vector r
2,x
=3.35 m and r
2,y
=−4.79 m, where the lettuce appears to be tastier (but really is not; this turtle cannot see very well). The excursion takes 323 s to complete. What are the components and the magnitude of the turtle's average velocity in meters per second? v
av,x
= m/s v
av,y
= ∫m/s ∣
v
av
∣=
Answers
The turtle's average velocity in the x-direction is approximately 0.00707 m/s, while in the y-direction it is approximately -0.00650 m/s. The magnitude of its average velocity, which represents the overall speed, is approximately 0.00955 m/s.
To find the components and magnitude of the turtle's average velocity, we can use the following formulas:
Average velocity components:
\(v_{av,x}\) = Δx / Δt
\(v_{av,y}\) = Δy / Δt
Magnitude of average velocity:
|\(v_{av}\)| = √(\((v_{av,x})^2\) + \((v_{av,y})^2\))
where:
Δx is the change in the x-coordinate (r2,x - r1,x)
Δy is the change in the y-coordinate (r2,y - r1,y)
Δt is the time taken for the excursion (323 s)
Given the position vectors:
r1,x = 1.07 m
r1,y = -2.69 m
r2,x = 3.35 m
r2,y = -4.79 m
Δt = 323 s
We can calculate the average velocity components and magnitude as follows:
Δx = r2,x - r1,x = 3.35 m - 1.07 m = 2.28 m
Δy = r2,y - r1,y = -4.79 m - (-2.69 m) = -2.10 m
\(v_{av,x}\) = Δx / Δt = 2.28 m / 323 s ≈ 0.00707 m/s
\(v_{av,y}\) = Δy / Δt = -2.10 m / 323 s ≈ -0.00650 m/s
|\(v_{av}\)| = √(\((v_{av,x})^2\) + \((v_{av,y})^2\)) = √\(((0.00707 m/s)^2 + (-0.00650 m/s)^2\)) ≈ 0.00955 m/s
Therefore, the components of the turtle's average velocity are approximately:
\(v_{av,x}\)≈ 0.00707 m/s
\(v_{av,y}\) ≈ -0.00650 m/s
And the magnitude of the turtle's average velocity is approximately:
|\(v_{av}\)| ≈ 0.00955 m/s
Learn more about magnitude here:
https://brainly.com/question/24256726
#SPJ11
find the circumference using the formula calculate your answer to the nearest hundredth
Answers
The circumference of the circle to the nearest hundredth is 21.98 inches.
How to find the circumference of a circle?The circumference of a circle is the measure of the boundary or the length of the complete arc of a circle.
The circumference of a circle is the perimeter of the circle. It's the wholes boundary of the circle.
The circumference of the circle can be found as follows:
circumference of a circle = 2πr
where
r = radiusTherefore,
diameter of the circle = 7 inches
radius of the circle = 7 / 2 = 3.5 inches
Hence,
circumference of a circle = 2 × 3.14 × 3.5
circumference of a circle = 21.98 inches
learn more on circumference here: https://brainly.com/question/20007490
#SPJ1

Write an equation is point slope form of a line given that the slope is 2/3 and that the line goes through the point (5,-2)
Answers
Answer:
\((y+2)=\frac{2}{3} (x-5)\)
Step-by-step explanation:
Point slope form is shown below:
\((y-y_1)=m(x-x_1)\)
We are given the slope m = 2/3 and the point x1 = 5, y1 = -2
Simply plug in these numbers into the above equation.
\((y--2)=\frac{2}{3} (x-5)\)
Simplify:
\((y+2)=\frac{2}{3} (x-5)\)
The problem is 52÷(−4/5)
Answers
Answer: either -65 or -2.6
Hope this helps
It depends on the way you do the math, but Its one of those.
11 term of 7 -28 112
Answers
The 11th term of this geometric sequence 7 -28, 112, .... include the following: 7,340,032.
How to calculate the nth term of a geometric sequence?In Mathematics, the nth term of a geometric sequence can be calculated by using this mathematical equation (formula):
aₙ = a₁rⁿ⁻¹
Where:
aₙ represents the nth term of a geometric sequence.r represents the common ratio.a₁ represents the first term of a geometric sequence.Next, we would determine the common ratio as follows;
Common ratio, r = a₂/a₁
Common ratio, r = -28/7
Common ratio, r = -4
For the 11th term, we have:
a₁₁ = 7(-4)¹¹⁻¹
a₁₁ = 7,340,032.
Read more on geometric sequence here: brainly.com/question/16423479
#SPJ1
In the triangle below, m∠K=55° and m∠N=75°. KN⎯⎯⎯⎯⎯⎯⎯⎯⎯ is ___________ of △KNT.
a) the shortest side
b) the longest side
c) congruent to one other side
d) the middle-length side
e) congruent to two other sides
f) None of the above
Expert Answer
Answers
In the triangle below, m∠K=55° and m∠N=75°. KN is the longest side of △KNT.
We are given a triangle with angles K, N, and T as shown below. We have been given that ∠K = 55° and ∠N = 75°.
We are to find out the side which is the longest among KN, NT, and KT.
Now, we know that in any triangle, the longest side is opposite to the largest angle and the smallest side is opposite to the smallest angle.
We can see that ∠N is the largest angle in this triangle and KN is opposite to it. So, KN is the longest side.
Hence, KN is the longest side of △KNT. Therefore, option (b) is correct.
To know more about triangles,
https://brainly.com/question/1058720
#SPJ11
Please can someone help me

Answers
Answer:
Matt is a lawyer who used to charge his clients $330 per hour. Matt recently reconsidered his rates and ultimately decided to charge $231 per hour. What was the percent of decrease in the billing rate?
Step-by-step explanation:
c is the ansswer