Answers
Answer:
Angle ZXY = 41 degrees.
Step-by-step explanation:
If WX is parallel to ZY then angle XZY = 71 degrees.
Angle ZXY = 180 - 68 - 71 = 41 degrees.
Related Questions
You can draw 2 cards from a deck of 52. Each card is numbered 1 number from the set {4,4,2,2,1,1,1,3}. What is the conditional probability that you will draw 1 first and 4 second?
Answers
Answer:
Step-by-step explanation:
Are the outcomes on the two cards independent? Why?
Yes. The events can occur together. No. The probability of drawing a specific second card depends on the identity of the first card. No. The events cannot occur together. Yes. The probability of drawing a specific second card is the same regardless of the identity of the first drawn card.
ANSWER RIGHT AWAY PLZ 30 POINTS
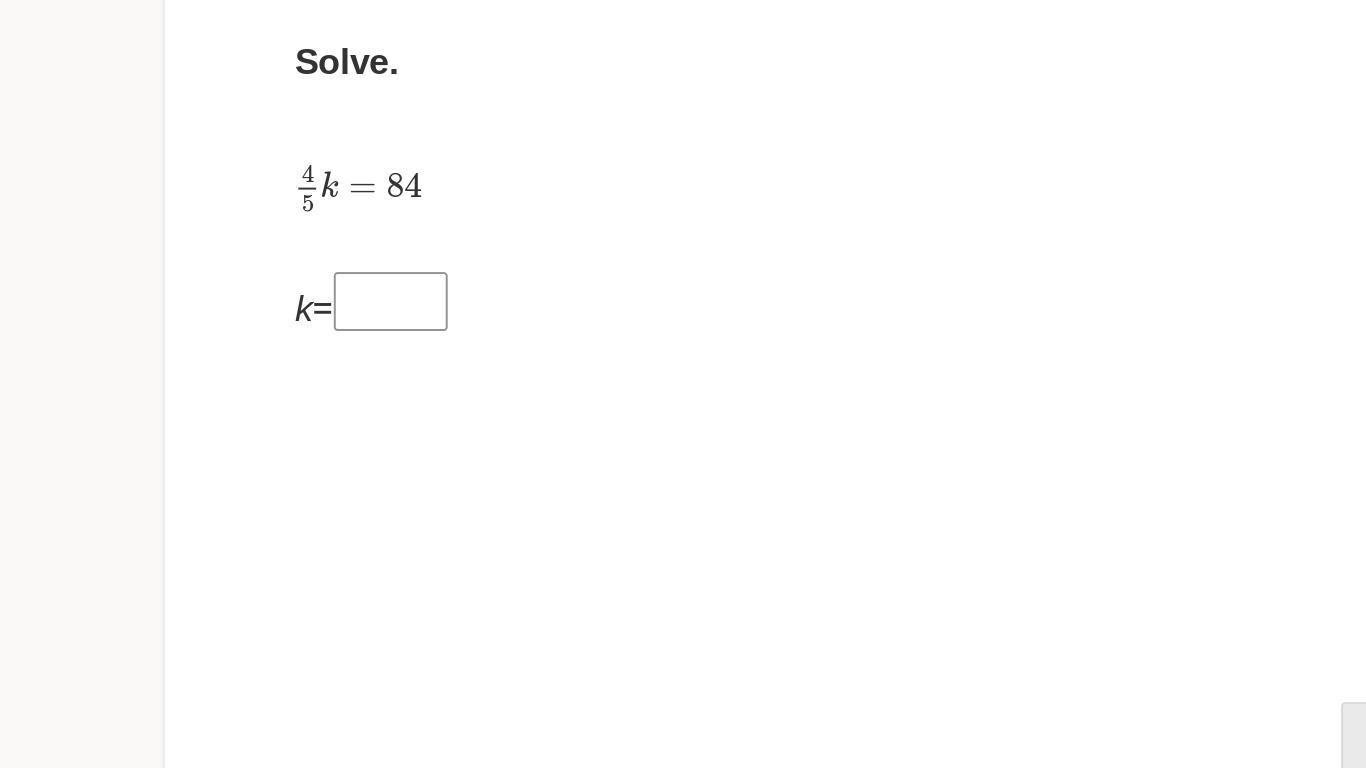
Answers
Answer:
105
Step-by-step explanation:
Answer: 105
Step-by-step explanation: 105 times 4 divided by 5 equals 84
twenty-five automobiles have been brought in for service. fifteen of them need tuneups and ten of them need new brakes. nine cars are chosen at random to be worked on. what is the probability that three of them need new brakes?
Answers
The probability that three of out nine cars need new brakes is 0.294
Combination:
The combination is the process of selecting items from a group of objects where the order of objects does not matter. In simple words, we can say that it is a combination of n things in r times without repetition.
The formula for the combination of 'a' things from the set of n things without replacement is given
ⁿCₐ = n!/a!(n - a)!Here from the given problem,
Number of automobiles = 25
Number of automobiles that need new tune-ups = 15
Number of automobiles that need new brakes = 10
Number of automobiles chosen at time = 9
From above formula
The number of ways of selecting 9 cars from 25 cars
²⁵C₉ = 25!/9!(25 - 9)!
= 25!/9!(16)!
= 2,042,975
The number of ways that can be chosen as 3 out of 9 cars that need breaks
Choosing 3 cars that need breaks from 10 cars = ¹⁰C₃
= 10!/3!(10 - 3)!
= 10!/3!(7)!
= 120
Choosing the remaining 6 cars from the 15 groups of cars that need new tune-ups = ¹⁵C₆
= 15!/6!(15 - 6)!
= 15!/6!(6)!
= 5,005
From above calculations
The number of ways that can be chosen as 3 out of 9 cars that need new breaks = 120 × 5,005
= 600600
As we know that
Probability of event = No of favorable outcomes / total outcomes
The probability that three of them need new brakes
= 600600/2042975
= 0.294
Learn more about Combinations at
brainly.com/question/28720645
#SPJ4
Draw the next pattern in sequence:1;3;10...
Answers
Answer:
1, 3, 10, 34....
Step-by-step explanation:
3x each number and add the previous numbers:
(1 x 3) + 0= 3
(3 x 3) + 1 = 10
(3 x 10) +3 + 1 = 34
There are six counties listed in the table. Each county is generally in the shape of a rectangle. County Length Width Population Johnson 16 miles 19 miles 7,600 Greene 17 miles 24 miles 12,240 Robin 28 miles 30 miles 23,520 Clark 15 miles 21 miles 9,450 Wade 22 miles 23 miles 15,180 Beaver 20 miles 20 miles 11,600 Which towns have the same population density?
Answers
The towns in Greene, Clark, and Wade counties have the same Population density.
The same population density, we need to compare the population densities of each county. Population density is calculated by dividing the population of a county by its area. In this case, since each county is in the shape of a rectangle, we can calculate the area by multiplying the length and width.
Let's calculate the population density for each county:
County: Johnson
Area: 16 miles * 19 miles = 304 square miles
Population Density: 7,600 / 304 ≈ 25 people per square mile
County: Greene
Area: 17 miles * 24 miles = 408 square miles
Population Density: 12,240 / 408 ≈ 30 people per square mile
County: Robin
Area: 28 miles * 30 miles = 840 square miles
Population Density: 23,520 / 840 ≈ 28 people per square mile
County: Clark
Area: 15 miles * 21 miles = 315 square miles
Population Density: 9,450 / 315 ≈ 30 people per square mile
County: Wade
Area: 22 miles * 23 miles = 506 square miles
Population Density: 15,180 / 506 ≈ 30 people per square mile
County: Beaver
Area: 20 miles * 20 miles = 400 square miles
Population Density: 11,600 / 400 ≈ 29 people per square mile
From the calculations, we can see that Greene, Clark, and Wade have the same population density, approximately 30 people per square mile.
Therefore, the towns in Greene, Clark, and Wade counties have the same population density.
For more questions on Population .
https://brainly.com/question/31101410
#SPJ8
I need help with this practice problem solving the subject is trig I have an additional picture that is the answer options, I will send this to you

Answers
Given
\(f(x)=\tan x\)To fill the blanks.
Now,
The grpah of f(x)=tanx is given as,
Therefore, from the graph,
\(\tan x=\tan (x+\pi)=\tan (x+2\pi)\)Then,
\(\pi\text{ is the fundamental period of tanx.}\)Also,
For x=0, tan(0)=0.
Therefore, the y-intercept is (0,0).
The points for the function f(x)=tanx is,
\(\begin{gathered} x=\frac{\pi}{3},\text{ y=tan(}\frac{\pi}{3})=\sqrt[]{3} \\ x=\frac{\pi}{4},y=\tan \frac{\pi}{4}=1 \end{gathered}\)Since
\(\tan (-A)=-\tan A\)Then, the points of the graph y=tanx is,
\((-\frac{\pi}{3},-\sqrt[]{3}),(-\frac{\pi}{4},-1),(0,0),(\frac{\pi}{3},\sqrt[]{3}),(\frac{\pi}{4},1)\)Hence, the answer should be in the order,
i)
\(\pi\)ii)
\((0,0)\)iii)
\((-\frac{\pi}{3},-\sqrt[]{3}),(-\frac{\pi}{4},-1),(0,0),(\frac{\pi}{3},\sqrt[]{3}),(\frac{\pi}{4},1)\)
ASAP please!!! Subject: 6th grade Math
A coach needs to fill a rectangular container with water to have available for karate practice. If the dimensions of the container are 30 inches by 22 inches by 20.8 inches, what is the maximum amount of water that the rectangular container will hold?
1,741.6 in3
3,483.2 in3
6,864 in3
13,728 in3
Answers
Answer:
The answer is D, did the test.
Step-by-step explanation:
Answer:
13,728 in.²
Step-by-step explanation:
volume = length × width × height
V = 30 in. × 22 in. × 20.8 in.
V = 13,728 in.²
find the ordered pair that corresponds to the given pair of parametric equations and value of t. x=4t 3, y=-3t 1; t=2
Answers
The ordered pair that corresponds to the given pair of parametric equations x = 4\(t^{3}\) and y = -3t + 1 when t = 2 is (32, -5).
In the given parametric equation, the variable t represents a parameter that ranges over a certain interval. By substituting the specific value of t = 2 into the equations, we can determine the corresponding values of x and y. In this case, when t = 2, the x-coordinate is calculated as 32 using the equation x = 4\(t^{3}\), and the y-coordinate is calculated as -5 using the equation y = -3t + 1. Therefore, the ordered pair that corresponds to the given equations and t = 2 is (32, -5).
learn more about parametric equation here:
https://brainly.com/question/30748687
#SPJ11
Colette earned a total of $15 for 3 hours of yard work. How many hours in all will it take Colette to earn $20? Assume the relationship is directly proportional.
Answers
Answer:
Step-by-step explanation:
It would take four hours because you divide fifteen dollars by three hours which would be five per hour. If each hour is five dollars, then four hours would be twenty dollars.
To determine the effectiveness of a diet to reduce cholesterol, 100 people are put on the diet. After a certain length of time their cholesterol level is taken. The diet is deemed a success if at least 55% have lowered their levels.
a) What is the probability the diet is a success, if, in fact, it has no effect on cholesterol levels? Use the normal approximation with a continuity correction. Round to 4 decimal places.
b) Calculate the answer using the binomial distribution and software (R, Excel or anything else).
Answers
a) The probability that the diet is a success, assuming no effect on cholesterol levels, is approximately 0.9441, using the normal distribution with a continuity correction.
b) Using the binomial distribution, the probability is approximately 0.9447, which closely aligns with the result obtained from the normal distribution approximation.
a) To determine the probability that the diet is a success, we will use the normal distribution with a continuity correction because the number of observations n = 100 is large enough to justify this approximation.
We have:
P(X ≥ 55)
To convert to the standard normal distribution, we calculate the z-score:
z = (55 - np) / sqrt(npq) = (55 - 100(0.55)) / sqrt(100(0.55)(0.45)) = -1.59
Using the standard normal distribution table, we obtain:
P(X ≥ 55) = P(Z ≥ -1.59) = 0.9441 (rounded to four decimal places)
Therefore, the probability that the diet is a success, given that it has no effect on cholesterol levels, is approximately 0.9441. This means that we would expect 94.41% of the sample to have cholesterol levels lowered if the diet had no effect.
b) Using the binomial distribution, we have:
P(X ≥ 55) = 1 - P(X ≤ 54) = 1 - binom.dist(54, 100, 0.55, TRUE) ≈ 0.9447 (rounded to four decimal places)
Therefore, the probability that the diet is a success, given that it has no effect on cholesterol levels, is approximately 0.9447. This is very close to the value obtained using the normal distribution, which suggests that the normal approximation is valid.
Learn more about probability
https://brainly.com/question/31828911
#SPJ11
daria bought a lemonade cup for $9 . each refill cost $2 . last month daria spent $31 on mug and refills . How many refills did she buy?
Answers
Answer:
Daria bought 11 refills of lemonade last month
Step-by-step explanation:
The equation is:
y = 2x + 9
The cup costs 9 dollars and is 2 dollars per refill
She spent 31 dollars so:
31 = 2x +9
Now we can solve!
2x + 9 = 31
Subtract 9 from both sides!
2x = 22
Divide both sides by 2!
x = 11
We know x is the amount of refills so,
Daria bought 11 refills of lemonade last month!
A relation is defined by the points (1, 4), (3, -1), (-1, -2), and (1, -3).
This relation ( is , is not ) a function because there ( is no x-value,is one x-value,are two x-values) corresponding to multiple y-values.
Answers
Answer: is not / is one x-value
Step-by-step explanation:
The relation is not a function because there is one x-value corresponding to multiple y-values.
Why it is not a function?
For a function, each point on the domain is mapped into only one point on the range.
In this relation we can see the points:
(1, 4), (3, -1), (-1, -2), and (1, -3).
Then we can see that the element of the domain "1" is being mapped into two different elements of the range (4, and -3).
Then the relation is not a function because there is one x-value corresponding to multiple y-values.
If you want to learn more about functions:
https://brainly.com/question/4025726
#SPJ2
Two teaching methods and their effects on science test scores are being reviewed. A random sample of 11 students, taught in traditional lab Sessions, had a mean test score of 77.4 with a standard deviation of 3.5. A random sample of 15 students, taught using interactive simulation software, had a mean test score of 84.8 with a standard deviation of 5.1. Do these results support the claim that the mean science test score is lower for students taught in traditional lab sessions than it is for students taught using interactive simulation software? Let me be the mean test score for the students taught in traditional lab sessions and 2 be the mean test score for students taught using interactive simulation software Use a significance level of a = 0.05 for the test. Assume that the population variances are equal and that the two populations are normally distributed
Answers
The mean science test score is lower for students taught in traditional lab sessions than it is for students taught using interactive simulation software at the α = 0.05 level of significance.
How mean science test score is lower for students?we will conduct a two-sample t-test with equal variances.
The null hypothesis is that there is no difference in the mean test scores between the two groups:
H₀: μ₁ = μ₂
The alternative hypothesis is that the mean test score for traditional lab sessions is lower than that of interactive simulation software:
Hₐ: μ₁ < μ₂
Here, n₁ = 11, n₂ = 15, x₁= 77.4, x₂ = 84.8, s₁ = 3.5, s₂ = 5.1, and the significance level α = 0.05.
First, we need to calculate the pooled sample standard deviation:
sp = \(\sqrt\)(((n₁ - 1)s₁² + (n₂ - 1)s₂²)/(n₁ + n₂ - 2))
sp = \(\sqrt\)(((10)(3.5)² + (14)(5.1)²)/(25))
sp = 4.446
Next, we calculate the test statistic t:
t = (x₁ - x₂)/(sp * \(\sqrt\)(1/n₁ + 1/n₂))
t = (77.4 - 84.8)/(4.446 * \(\sqrt(1/11 + 1/15)\))
t = -2.636
The degrees of freedom for the test is (n₁ + n₂ - 2) = 24.
Using a t-table with 24 degrees of freedom and a one-tailed test at the α = 0.05 level of significance, we find that the critical value is -1.711.
Since the calculated t-value (-2.636) is less than the critical value (-1.711), we reject the null hypothesis.
Therefore, we can conclude that the results support the claim that the mean science test score is lower for students taught in traditional lab sessions than it is for students taught using interactive simulation software at the α = 0.05 level of significance.
Learn more about Null hypothesis
brainly.com/question/28920252
#SPJ11
If you order 12 pizzas, you will get 96 slices of pizza. How many slices are there in one
pizza?
Answers
Answer:
8 slices per pizza.
Step-by-step explanation:
To find the number of slices in one pizza, we can use division.
If you order 12 pizzas, you will get 96 slices of pizza. To find the number of slices in one pizza, we can divide the total number of slices by the number of pizzas:
96 ÷ 12 = 8
Therefore, there are 8 slices in one pizza.
Answer:
8
Step-by-step explanation:
12 pizzas have 96 slices
So one pizza must have 96/12 = 8 slices of pizza
4. Eva saves $60 each week. Since she needs to save at least $2,400 to go on a trip to Europe, she will need to
save for at least 40 weeks.
Answers
Answer:
where is the question so that I can solve it
Answer:
54
Step-by-step explanation:
tsfadgas sadjkasbdaskbj as dasdas
In a sample of 20 items, you found six defective. In constructing a confidence interval for the proportion of defectives, you should use: the plus four method. the large-sample interval. neither of these two methods.
Answers
To construct a confidence interval for the proportion of defectives, we should use the plus four method.
Since the sample size is 20, which is not very large, the large-sample interval is not appropriate. Instep, we ought to utilize a strategy that's suitable for little test sizes.
The plus four method is one such method that is commonly used when the sample size is small. Therefore, to construct a confidence interval for the proportion of defectives, we should use the plus four method.
The plus four strategies may be a strategy for building a certainty interim for an extent when the test estimate is little.
To utilize this strategy, we to begin with include four fanciful perceptions to our test, two of which are flawed and two of which are not imperfect.
This increments the test estimate to 24, which permits us to utilize the typical guess to the binomial dispersion to build the certainty interim.
The equation for the certainty interim utilizing the also four strategies is:
p ± zα/2 √((p + 2) (1 - p + 2) / n + 4)
where:
p is the test extent (number of defectives/test estimate)
zα/2 is the basic esteem from the standard typical dissemination at the level of importance α/2
n is the test estimate (counting the included four nonexistent perceptions)
Therefore, to construct a confidence interval for the proportion of defectives, we should use the plus four method.
learn more about the plus four method
brainly.com/question/31504643
#SPJ4
Mason Bought 18 fish to fill up his new 30 gallon aquarium. If fish cost 1 .89 each how much did he spend all togetherA 17.01B 19.89C 34.02D 56.70
Answers
We have the following:
In this case, to know the value of the total expense, we must multiply the price of the fish by the amount of fish that he bought like this:
\(18\cdot1.89=34.02\)Therefore, the answer is the option C. 34.02
Help me please !! i need the answer
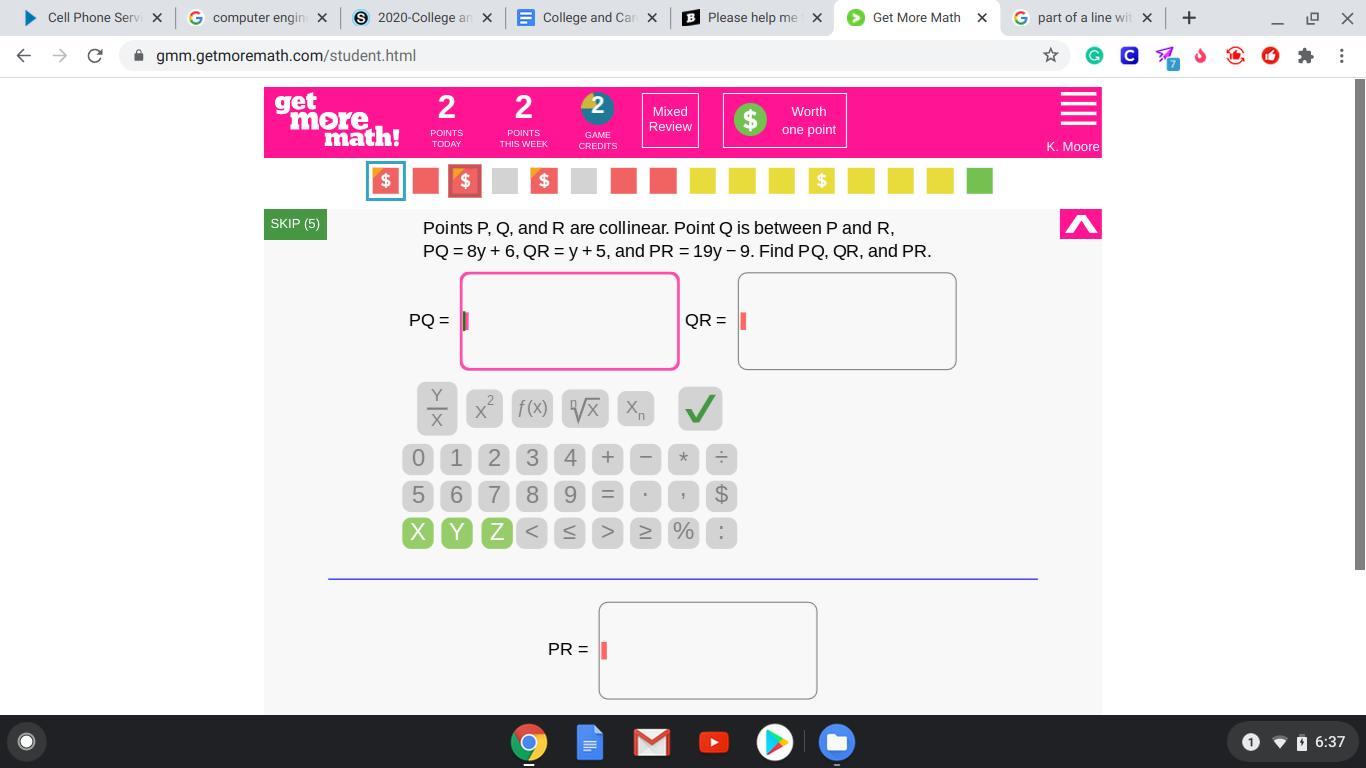
Answers
Answer:
\(PQ=22\\\\QR=7\\\\PR=29\)
Step-by-step explanation:
To find the value of y, you can create an equation where two parts of the line are equal to the whole line:
\(PQ+QR=PR\)
Substitute given values:
\((8y+6)+(y+5)=(19y-9)\)
Solve for y. Simplify parentheses:
\(8y+6+y+5=19y-9\)
Combine like terms to simplify the equation:
\(8y+y+6+5=19y-9\\\\9y+11=19y-9\)
Get the variable on one side of the equation and the constants on the other. Add 9 to both sides:
\(9y+11+9=19y-9+9\\\\9y+20=19y\)
Subtract 9y from both sides:
\(9y-9y+20=19y-9y\\\\20=10y\)
Isolate the variable. Divide both sides by 10:
\(\frac{20}{10} =\frac{10y}{10} \\\\y=2\)
The value of y is 2. Insert this value into each line segment value and solve:
\(PQ=8(2)+6\\\\PQ=16+6\\\\PQ=22\)
\(QR=(2)+5\\\\QR=7\)
\(PR=19(2)-9\\\\PR=38-9\\\\PR=29\)
:Done
You can check by using:
\(PQ+QR=PR\\\\22+7=29\)
This statement is true, so the values are correct.
What is the length of 7.2 ft & 9.6ft
Answers
Answer:
16.8ft !
7.2 + 9.6 = 16.8 ft .
Answer:
12
Step-by-step explanation:
Using Pythagorean Theorem we know that
a² + b² = c²
7.2² + 9.6² = c²
51.84 + 92.16 = 144
Then find the square root of your c and you should get 12
__________________________
Or: 7.2 + 9.6 = 16.8 ft if that's what you mean
Given that a = 6i- 3j, find:
a the unit vector in the same direction of a
b the angle that a makes with the positive x-axis.
Answers
The unit vector in the same direction will be equal to (6 i/5.1 - 3 j/5.1).
What is a Vector?A vector is a quantity with both magnitude and direction in physics. It is often depicted by an arrow with the same direction as the amount and a length proportionate to the size of the quantity.
A vector lacks position, but has magnitude and direction. A vector is therefore unaffected by displacement parallel to itself as far as its length is unaltered.
As per the given data in the question,
The unit vector is calculated as:
\(\vec u_a=\frac{\vec a}{||\vec a||}\)
\(\vec a= x \vec i+y \vec j\)
Now, calculate the magnitude of the vector,
\(\vec a\) = √(x² + y²)
\(|\vec a|\) = √6² - 3²
= √36 - 9
= 5.1
So, the unit vector will be,
\(\vec u_a\) = (6 i - 3 j)/5.1
To know more about vector:
https://brainly.com/question/29740341
#SPJ1
7m+2=7n-5 solve so m is an independent variable
Answers
Here is part of a recipe for different-size cakes, showing the ratio of eggs to flour.
Make a table that represents the same situation.
eggs : 0 2 4 6
flour (cups) : 0 1.5 3 4.5
Answers
question, we have to calculate the ratio by dividing a by b we will get the ratio as \(\frac{4}{3}\).
What is a ratio formula?Using the ratio formula, ratios can be represented as a fraction. The ratio formula for any two quantities says a and b, is a:b = a/b. Because a and b are individual amounts for two portions, the total quantity is given as (a + b).
How do you calculate the ratios of a recipe?Known Total Amount
Determine the total quantity to be produced.
Determine the total number of parts in the ratio.
Divide the total quantity made by the total number of parts to get the amount per part.
Calculate the amount of each ingredient by multiplying each component by the amount per part.
Given:
Here is the ratio of eggs to flour
Eggs(a) 0 2 4 6
Flour(b) 0 1.5 3 4.5
For the table look at the image.
as per the question, we have to calculate the ratio by dividing a by b we will get the ratios as \(\frac{4}{3}\).
To know more about Ratios visit:
https://brainly.com/question/13419413
#SPJ4

Whenever he visits Dayton, Quincy has to drive 12 miles due north from home. Whenever he
visits Belleville, he has to drive 16 miles due east from home. How far apart are Dayton and
Belleville, measured in a straight line?
Answers
Answer: 20 miles
Step-by-step explanation:
Hi, since the situation forms a right triangle (see attachment) we have to apply the Pythagorean Theorem:
x^2 = a^2 + b^2
Where x is the hypotenuse of the triangle (in this case the distance between Dayton and Belleville) and a and b are the other sides.
Replacing with the values given:
x^2 = 16^2 + 12^2
x^2 = 256+144
x^2 = 400
x = √400
x = 20 miles
Feel free to ask for more if needed or if you did not understand something.

need help with exam study sheet
Answers
The rate of change is (-1)
To solve this, we need to look at graphic to know the values of the function f(x) when x is equal to 1 and 3.
Then we have two points of the graph: (1,6) and (3,4).
The rate of change between two points is:
\(m=\frac{\text{change in f(x)}}{change\text{ in x}}\)In this case,
\(m=\frac{4-6}{3-1}=\frac{-2}{2}=-1\)Note that its the same whatever coordinate put first:
\(\frac{6-4}{1-3}=\frac{4-6}{3-1}=-1\)But when you do the difference between the coordinates, make sure to respect the order: if you use the y coordinate of the point P minus the y coordinate of point Q, you must to do the same for the x coodinate; Px-Qx
Don’t really get these very much. Can anyone help ?

Answers
Answer:
23) \(g\) has an amplitude greater than the period of \(f\)/Both lines have the same period/There are no shifts of any kind between both lines.
24) Both lines have the same amplitude/\(g\) has a period less than period of \(f\)/There are no shifts of any kind between both lines.
25) Both lines have the same amplitude/Both lines have the same period/There is an horizontal shift between \(f\) and \(g\).
26) Both lines have the same amplitude/Both lines have the same period/There is a vertical shift between \(f\) and \(g\).
Step-by-step explanation:
We proceed to describe succintly all differences and simmilarities between \(f\) and \(g\) in each case:
23) \(g\) has an amplitude greater than the period of \(f\)/Both lines have the same period/There are no shifts of any kind between both lines.
24) Both lines have the same amplitude/\(g\) has a period less than period of \(f\)/There are no shifts of any kind between both lines.
25) Both lines have the same amplitude/Both lines have the same period/There is an horizontal shift between \(f\) and \(g\).
26) Both lines have the same amplitude/Both lines have the same period/There is a vertical shift between \(f\) and \(g\).
A small tool-and-die shop manufactures kneuter valves. A shipment of 15 valves to a Swedish automobile assembly plant contains three defective values. Suppose the assembly plant randomly selects four valves from the shipment.
a. What is the probability that all four valves will be defect-free?
b. What is the probability that the plant will select all three defectives?
c. What is the probability that the plant will select at least one defective?
Answers
To solve these probability problems, we need to calculate the probabilities using the concept of combinations.
a. To find the probability that all four valves will be defect-free, we need to select four valves from the 12 non-defective valves out of a total of 15 valves. The probability can be calculated as follows:
P(all four defect-free) = (Number of ways to choose 4 defect-free valves) / (Number of ways to choose 4 valves from the total)
P(all four defect-free) = (C(12, 4)) / (C(15, 4))
C(n, r) represents the combination formula, which calculates the number of ways to choose r items from a set of n items.
b. To find the probability that the plant will select all three defectives, we need to select three valves from the three defective valves out of a total of 15 valves. The probability can be calculated as follows:
P(all three defectives) = (Number of ways to choose 3 defective valves) / (Number of ways to choose 4 valves from the total)
P(all three defectives) = (C(3, 3)) / (C(15, 4))
c. To find the probability that the plant will select at least one defective valve, we can subtract the probability of selecting all four defect-free valves from 1. In other words:
P(at least one defective) = 1 - P(all four defect-free)
Now, let's calculate the probabilities using the given information:
a. P(all four defect-free) = (C(12, 4)) / (C(15, 4))
b. P(all three defectives) = (C(3, 3)) / (C(15, 4))
c. P(at least one defective) = 1 - P(all four defect-free)
Learn more about probability here
https://brainly.com/question/25839839
#SPJ11
consider the quadratic function y equals short dash x squared plus 6 x minus 5. what do we know about the graph of this quadratic equation, based on its formula?
Answers
Based on the formula of the quadratic function y=-x^2+6x-5, we know that its graph is a downward-facing parabola that opens wide, with a vertex at (3,-14), and an axis of symmetry at x=3.
Based on the formula of the quadratic function y=-x^2+6x-5, we can determine several properties of its graph, including its shape, vertex, and axis of symmetry.
First, the negative coefficient of the x-squared term (-1) tells us that the graph will be a downward-facing parabola. The leading coefficient also tells us whether the parabola is narrow or wide. Since the coefficient is -1, the parabola will be wide.
Next, we can find the vertex using the formula:
Vertex = (-b/2a, f(-b/2a))
where a is the coefficient of the x-squared term, b is the coefficient of the x term, and f(x) is the quadratic function. Plugging in the values for our function, we get:
Vertex = (-b/2a, f(-b/2a))
= (-6/(2*-1), f(6/(2*-1)))
= (3, -14)
So the vertex of the parabola is at the point (3,-14).
Finally, we know that the axis of symmetry is a vertical line passing through the vertex. In this case, it is the line x=3.
Know more about quadratic function here;
https://brainly.com/question/18958913
#SPJ11
2. Bucky walked 85 feet. His friend in Canada wanted to
know how many meters he walked.
Given that 1 foot = .3048 meter, how many meters
did Bucky walk?
Answers
The distance that Bucky walked is 25.908 meters
What is distance ?
Distance is a numerical measurement of how far apart two objects or points are in space. It is a scalar quantity, which means that it is defined only by its magnitude and not by its direction. Distance can be measured using various units, such as meters, feet, kilometers, miles, and so on, depending on the system of measurement being used.
Distance is an important concept in mathematics, physics, and other fields that deal with spatial relationships. It is often used to describe the length of a path traveled by an object or the separation between two objects in space.
According to the question:
To convert feet to meters, we can use the conversion factor 1 foot = 0.3048 meter.
The distance that Bucky walked is given as 85 feet. To find the distance in meters, we can multiply the number of feet by the conversion factor:
Distance in meters = 85 feet x 0.3048 meter/foot
Simplifying this expression, we get:
Distance in meters = 25.908 meters
Therefore, Bucky walked 25.908 meters.
To know more about distance visit:
https://brainly.com/question/15256256
#SPJ1
Find the degree measure of the complement of an angle whose degree measure is 2c.
Answers
Derrick is outside playing basketball with his friend Bryce. Derrick is óft tall and at this time of day is casting a 13ft shadow. The basketball hoop is 10ft tall. How long is the basketball hoop's shadow? * Your answer
Answers
Answer:
21ft
Step-by-step explanation:
