Answers
Answer:
34.64 feet.
Step-by-step explanation:
A wire from the top of a telephone pole to a point on the ground 20m from the pole is 40m long.
Step-by-step explanation:
by using Pythagoras law
let x=?
x²=20²+5²
x²=425
x=√425=20.61ft
21 ft long the wire from the top of the telephone pole to the ground.
Related Questions
find the value of x
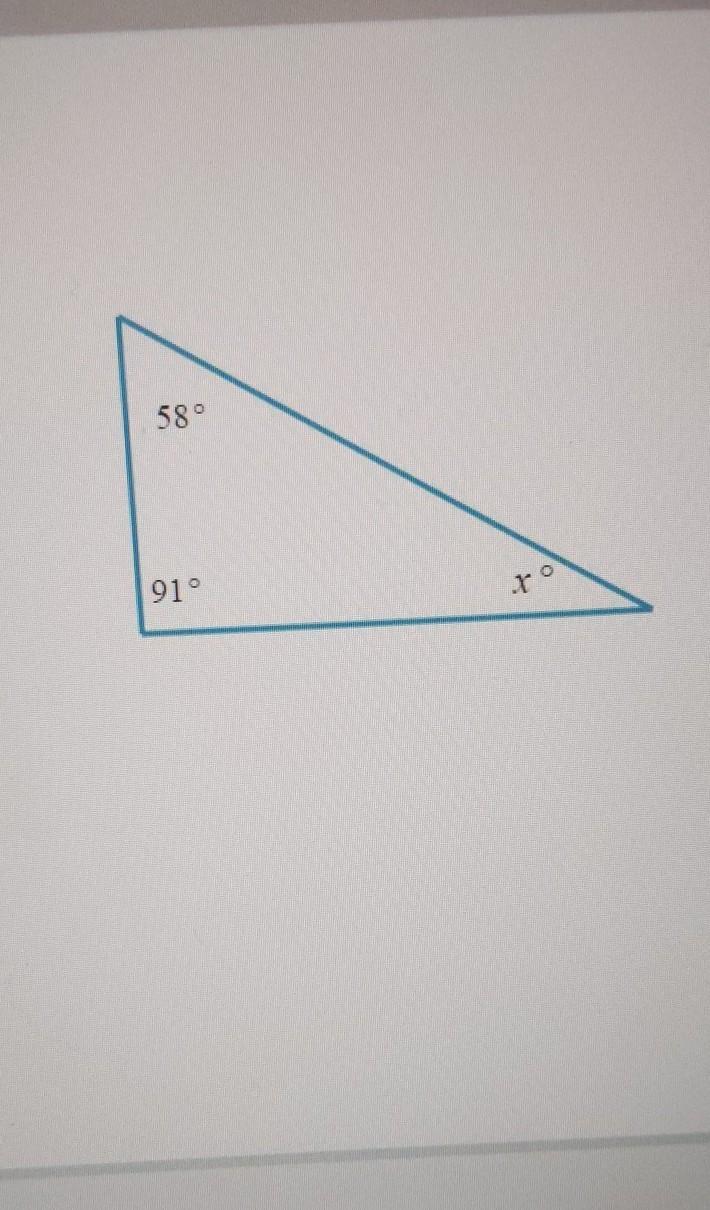
Answers
The sum of 1/2 and 2/5 is greater than one yes or no
Answers
Sherelle and Venita find two bags of coins in their grandmother’s attic. Each girl selects a bag, takes a random sample of 20 coins from that bag, and lists the dates of those coins as the number of years since 1900. Here are the results.
Sherelle: 72, 56, 81, 84, 75, 26, 39, 62, 65, 66, 85, 71, 74, 68, 73, 66, 83, 60, 58, 72
Venita: 45, 65, 73, 57, 79, 66, 69, 75, 51, 44, 53, 69, 58, 77, 51, 55, 53, 78, 70, 62
1. Find the five-number summary of each data set. Then construct box-and-whisker plots of the data sets. Draw both plots above the same number line. Label the plots with the girls’ names.
Answers
The five-number summary comprehends the minimum and maximum values, the 1st and 3rd quartiles and the median.
1st step: is to arrange the data sets from least to greatest.
Sherelle:
26 39 56 58 60 62 65 66 66 68 71 72 72 73 74 75 81 83 84 85
Venita:
44 45 51 51 53 53 55 57 58 62 65 66 69 69 70 73 75 77 78 79
2nd step: To calculate each quartile you have to determine their position and then identify which observation sits in that position:
Quartile 1:
PosQ₁: n/4= 20/4= 5
Quartile 2 (Median)
PosMe: n/2= 20/2= 10
Quartile 3
PosQ₃: n*(3/4)= 20*(3/4)= 15
Since both samples have the same size, the 1st quartile will be the fifth observation of each sample, the median will be the tenth observation and the 3rd quartile will be the fifteenth.
Sherelle:
Q₁: 60
Me: 68
Q₃: 74
Minimum: 26
Maximum: 85
Venita:
Q₁: 53
Me: 62
Q₃: 70
Minimum: 44
Maximum: 79
An experiment calls for each student to use 3.50 g of sodium hydroxide. There is only
78.2 g of sodium hydroxide remaining. How many students can complete the experiment?
Answers
Answer:
22 students
Step-by-step explanation:
In a bag of gum balls, 30% of the gum balls are blue. There are 12 blue gum balls in the bag. How many total gum balls are in the bag?
Answers
Answer:
64
Step-by-step explanation:
Answer:
40
Step-by-step explanation:
Since 30% of the bag is blue, you can setup the equation, \(\frac{3}{10}x=12\) where x is the total number of gumballs in the bag because that is what you are looking for. Simplify and you get \(x=40\). Checking this you get \(12=12\) which is true.
pleaseeee helppp i’m running out of time

Answers
Answer:
Square Root of 162 ~ 12.727
Step-by-step explanation:
Simply do the Pythagorean Theorem!
a^2 + b^2 = c^2
This case we're solving for the long side which is the hypothenuse.
9^2+9^2= c^
81+81=c^
162=c^
Square root of 162
Which is 12.727
Pythagorean Theorem

Answers
Step-by-step explanation:
........................


Answer:
root of 162
Step-by-step explanation:
i hope this helps please put brainliest im only 9
LIt cost Sophia $530 to make wind chimes. How many
chimes must she sell at $12 apiece to make a profit?
Answers
Answer:
c = number of chimes
12c = amount gotten from the sale of the chimes.
To make a profit what she gets from the sales must be greater than her expenses.
12c > 530
c > 44.166666
She must sell each chime for more than 44 an one-sixth cent.
Step-by-step explanation:
I need help with this problem

Answers
Answer:
X=13
Step-by-step explanation:
So if ΔABC ≅ ΔDEC, then that means that ∠B is ≅ ∠E. Therefore you would write the equation 3x=6x-39 and solve for X to get 13.
what does it mean to say that two variables are negatively correlated
A.)When one variable increases, the other decreases.
B.)When variable increases, the other also
C.)When one variable decreases, the other also decfreases
D.)Both variables changes at the same rate
Answers
To say that two variables are negatively correlatted means that when one variable increases, the other variable decreases.
Negative correlation refers to a relationship between two variables where they tend to move in opposite directions. When one variable increases, the other variable tends to decrease, and vice versa. This negative relationship indicates an inverse association between the two variables.
Option A, "When one variable increases, the other decreases," accurately describes negative correlation. As one variable increases, the other variable decreases, reflecting the negative relationship between them.
Negative correlation does not imply that both variables change at the same rate (Option D). In negative correlation, the relationship is characterized by the opposite direction of change, not necessarily the same magnitude or rate of change.
Option B, "When variable increases, the other also," suggests a positive correlation, where both variables move in the same direction. This is not the case in negative correlation.
Option C, "When one variable decreases, the other also decreases," is a misinterpretation of negative correlation. In negative correlation, when one variable decreases, the other variable tends to increase, not decrease.
To know more about variable click here
brainly.com/question/2466865
#SPJ11
Is 7 to the 8th power times 7 equivalent to 7 to the the third power times 7 to third power equivalent?
Answers
Answer:
They are not equivalent.
Step-by-step explanation:
Before we solve the question, we need to know that from the laws of indices:
A^x x A^y = A^(x+y).
7 to the power of 8th power means 7^8
7^8 times 7 means 7^8 x 7. From the laws of indices we have 7^8 x 7 = 7^9.
7 to the power of 3rd power means 7^3
7^3 times 7 to the power of 3rd power means 7^3 x 7^3. From the laws of indices we have 7^3 x 7^3 = 7^6.
Obviously, 7^9 ≠ 7^3 therefore, they are not equivalent.
rita offers to pay for 8 of her friends to play lazer tag, but 3 of them dont want to pay
Answers
Answer:
only 5 of her friends will play
Step-by-step explanation:
8-3=5
Answer:
The answer 5
Step-by-step explanation:
8 friends minus the three that dont want to play
Natalie invests $3,686 in a retirement account with a fixed annual interest rate of 7% compounded 4 times per year. what will the account balance be after 18 years?
Answers
Answer:
12,853.85568
Step-by-step explanation:
\(A=P(1+\frac{r}{n})^{nt}\)
A= final amount
P= inital principal balance
r= interest rate
n= number of times interest applied per time period
t= numbers of period
\(A=3,686(1+\frac{.07}{4})^{(4)(18)}\)
Carlos graphed the system of equations that can be used to solve x cubed minus 2 x squared 5 x minus 6 = negative 4 x squared 14 x 12.
Answers
The system of equations for x^3 - 2x^2 + 5x - 6 = -4x^2 + 14x + 12.
Carlos graphed the system of equations that can be used to solve the equation x^3 - 2x^2 + 5x - 6 = -4x^2 + 14x + 12.
To graph this system of equations, we need to set the equation equal to zero. So, the equation becomes:
x^3 - 2x^2 + 5x - 6 + 4x^2 - 14x - 12 = 0
Combining like terms, we get:
x^3 + 2x^2 - 9x - 18 = 0
To graph this equation, you can use a graphing calculator or plot points manually. Here are the steps to manually plot the graph:
1. Choose a range for x, for example, from -5 to 5.
2. Pick a few values for x within the chosen range and substitute them into the equation to find the corresponding y-values.
3. Plot the points (x, y) on a coordinate plane.
4. Connect the plotted points to create a smooth curve.
By following these steps, you can graph the system of equations for x^3 - 2x^2 + 5x - 6 = -4x^2 + 14x + 12.
To learn more about graph
https://brainly.com/question/31838491
#SPJ11
811 decreased by 354
Answers
811-354
you are taking away 354 from 811
answer: 547
please consider giving be brainliest, i need it to level up
The product of two positive numbers is 9. The reciprocal of
one of these numbers is 4 times the reciprocal of the other number. What is the sum of the two numbers?
Answers
Step-by-step explanation:
Let x and y be the two positive numbers.
Now according to the given condition we have:
x.y=9........(1)
1/x=4.1/y......(2)
Now from (1) we have,
1/x=y/9
Substituting 1/x=y/9 in (2) we have,
y/9=4/y
Solving this we have
y^2=36
y=+6 and y=-6
But neglecting the negative value as we are given in the question that the numbers are positive. So
y=6
Substituting y=6 in (1) we have,
x.6=9
Solving which gives
x=3/2
So the numbers are 3/2 and 6
what is the area???!?!?!

Answers
9514 1404 393
Answer:
ac
Step-by-step explanation:
The length of the base is the x-distance between E and H, so is ...
base = a - 0 = a
The height is the y-distance between E and F, so is ...
height = c - 0 = c
The area of the parallelogram is the product of base and height.
A = base×height
A = a×c
The area of the parallelogram is ac.
How would I solve the equation’s by cross multiplying number 4,5,9,10

Answers
Answer:
4) a = 6
5) m = 1
Step-by-step explanation:
4) use FOIL, First Inside Outside Last, when you cross multiply.
\(\frac{a+4}{a-1} =\frac{a-2}{a-4}\)
\((a+4)(a-4) = (a-1)(a-2)\)
I'm going to do the left side first.
\((a+4)(a-4)\\a^2-4a+4a-16\\a^2-16\)
Now I'm going to do the right side.
\((a-1)(a-2)\\a^2-2a-1a+2\\a^2-3a+2\)
Next I'm going to rewrite back as the equation and solve for a.
\(a^2 - 16 = a^2 -3a +2\\a^2 - a^2 -16 = a^2-a^2-3a+2\\-16-2=-3a+2-2\\-18=-3a\\-18/-3=-3a/-3\\a=6\)
5) I'm going to use distributive propoerty when I cross multiply. Then I will setup an equation like number 4 and solve for m.
\(\frac{5m-2}{3}=\frac{8-3m}{5} \\\)
\(5(5m-2)=3(8-3m)\\25m-10=24-9m\\25m+9m-10=24-9m+9m\\34m-10=24\\34m-10+10=24+10\\34m=34\\34m/34=34/34\\m=1\)
Hello can someone please help me with this

Answers
I don't understand how to solve this, please help!

Answers
Answer:
x+1 , x<-1
x²-bx-4 , -1≤x≤4
1/2 x +a
substitute x=-1 , continuous everywhere
x+1=x²-bx-4 when x=-1
-1+1=-1²-b-4
1+b-4=0 ⇒ +b-3 ⇒ b=3then for :
x²-bx-4=1/2 x +a for x=4
4²-4b-4=1/2x+a
16-4(3)-4=4/2+a
16-12-4-2=a
a=-2the equations will be :
x+1
x²-3x-4
1/2x-2
I hope this help
Given that the polynomial f(x) has 3 intercepts, which of the following most accurately describes the degree of f(x)?
Answers
Answer:
the degree of f(x) is 3
Step-by-step explanation:
fundamental theorem of algebra
The degree of function f(x) is at least 3.
How to determine the number of root from the number of intercepts of the function?The function has n intercepts i.e. it touches the x-axis n times, then the function will have at least n roots.
And as we know if the degree of the function is n then the number of roots of the function is n.
So, if the function has n intercepts, then the function has at least n degrees.
Here given that the polynomial function f(x) has 3 intercepts.
Then from above, it is clear that the function will have at least 3 roots.
And the function will have at least 3 degrees.
Therefore If the polynomial f(x) has 3 intercepts, then The degree of function f(x) is at least 3.
Learn more about the degree of function
here: https://brainly.com/question/1600696
#SPJ2
1. Let the distribution of X be the normal distribution N (μ, σ2) and let Y = aX + b. Prove that Y is distributed as N (aμ + b, a2σ2).
2. Let X and Y be two independent random variables with E|X| < [infinity], E|Y| < [infinity] and E|XY| < [infinity]. Prove that E[XY] = E[X]E[Y].
Answers
1 Y is distributed as N(aμ + b, a^2σ^2), as desired.
2 We have shown that under these conditions, E[XY] = E[X]E[Y].
To prove that Y is distributed as N(aμ + b, a^2σ^2), we need to show that the mean and variance of Y match those of a normal distribution with parameters aμ + b and a^2σ^2, respectively.
First, let's find the mean of Y:
E(Y) = E(aX + b) = aE(X) + b = aμ + b
Next, let's find the variance of Y:
Var(Y) = Var(aX + b) = a^2Var(X) = a^2σ^2
Therefore, Y is distributed as N(aμ + b, a^2σ^2), as desired.
We can use the definition of covariance to prove that E[XY] = E[X]E[Y]. By the properties of expected value, we know that:
E[XY] = ∫∫ xy f(x,y) dxdy
where f(x,y) is the joint probability density function of X and Y.
Then, we can use the fact that X and Y are independent to simplify the expression:
E[XY] = ∫∫ xy f(x) f(y) dxdy
= ∫ x f(x) dx ∫ y f(y) dy
= E[X]E[Y]
where f(x) and f(y) are the marginal probability density functions of X and Y, respectively.
Therefore, we have shown that under these conditions, E[XY] = E[X]E[Y].
Learn more about distributed here:
https://brainly.com/question/29664127
#SPJ11
Write the following numbers
product of their prime factors.
numbers as a
120; 180
300
Answers
Answer:
120 = 2 * 2 * 2 * 3 * 5 = 2^3 * 3 * 5
180 = 2 * 2 * 3 * 3 * 5 = 2^2 * 3^2 * 5
300 = 2 * 2 * 3 * 5 * 5 = 2^2 * 3 * 5^2
Step-by-step explanation:
Here, we want to write the given numbers as the product of their prime numbers
Prime numbers are numbers divisible by 1 and itself
We have the prime factorization as follows;
120 = 2 * 2 * 2 * 3 * 5 = 2^3 * 3 * 5
180 = 2 * 2 * 3 * 3 * 5 = 2^2 * 3^2 * 5
300 = 2 * 2 * 3 * 5 * 5 = 2^2 * 3 * 5^2
Naomi's car used \frac{2}{5} 5 2 of a gallon to travel 15\tfrac{1}{2}15 2 1 miles. how many miles can the car go on one gallon of gas?
Answers
Answer:
To determine how many miles Naomi's car can go on one gallon of gas, we first need to determine how many gallons of gas the car used to travel 15\tfrac{1}{2}15 2 1 miles. We are told that the car used \frac{2}{5} 5 2 of a gallon to travel this distance, so we can multiply this value by 15\tfrac{1}{2}15 2 1 to get the total number of gallons used:
\frac{2}{5} \cdot 15\tfrac{1}{2} = 9
Therefore, the car used 9 gallons of gas to travel 15\tfrac{1}{2}15 2 1 miles. To determine how many miles the car can go on one gallon of gas, we need to divide the total number of miles traveled by the number of gallons used:
15\tfrac{1}{2} \div 9 = \frac{31}{18}
This means that the car can go 31/18 miles on one gallon of gas. Since this fraction is not in simplest form, we can further simplify it by dividing the numerator and denominator by the greatest common factor, which is 3:
\frac{31}{18} \div \frac{3}{3} = \frac{31}{18} \cdot \frac{3}{3} = \frac{31 \cdot 3}{18 \cdot 3} = \frac{93}{54}
Therefore, the car can go 93/54 miles on one gallon of gas. This fraction can be further simplified by dividing the numerator and denominator by the greatest common factor, which is 1:
\frac{93}{54} \div \frac{1}{1} = \frac{93}{54} \cdot \frac{1}{1} = \frac{93 \cdot 1}{54 \cdot 1} = \frac{93}{54}
Therefore, the final answer is that the car can go 93/54 miles on one gallon of gas.
In triangle ABC show find BC..
Need help ASAP!!!

Answers
Answer: BC=22cm
Step-by-step explanation:
5d-3=3d+7
2d=10
d=5
plug 5 into the equation for BC, and you get 22
Answer:
Segment BC is (3d + 7)cm, where d=5. So, BC= 22cm.
Step-by-step explanation:
Segment BC is (3d + 7)cm, where d=5. You find this through these steps:
(5d-3)=(3d+7) Subtract 3d from 5d, which equals 2d.
2d-3=7 Now, add 3 to 7.
2d=10 Divide 10 by 2.
d=5 The solution is 5.
Plug 5 into the equation of Segment BC:
3(5) + 7
BC= 22
The diameter of a cone's circular base measures 10 inches, and the slant height of the cone is 8 inches.
What is the approximate surface area of the cone?
Responses
Answers
The approximate surface area of a cone is 204 inches².
What is a cone?It is a shape of a Christmas tree where there is a base of radius r and a top point called the apex.
We have,
The surface area of a cone is πr (r + √(h² + r²) )
Now,
Diameter = 10 inches
Radius = 5 inches
Slant height = 8 inches
Now,
The surface area of a cone is πr (r + √(h² + r²) )
Slant height = √(h² + r²) = 8 inches
So,
= πr (r + √(h² + r²) )
= π x 5 (5 + 8)
= 3.14 x 5 x 13
= 204 inches²
Thus,
The surface area of a cone is 204 inches².
Learn more about cones here:
https://brainly.com/question/13798146
#SPJ1
The approximate surface area of the cone is 227 square inches.
What is Three dimensional shape?a three dimensional shape can be defined as a solid figure or an object or shape that has three dimensions—length, width, and height.
The diameter of a cone's circular base measures 10 inches
Radius of cone is 5 inches.
Slant height = 8 inches
A=πr(r+√h²+r²)
A=3.14×5(5+√64+25)
A=3.14×5(5+√89)
A=3.14×5(5+9.43)
A=3.14×5(14.43)
A=226.61
Hence, the approximate surface area of the cone is 227 square inches.
To learn more on Three dimensional figure click:
https://brainly.com/question/2400003
#SPJ1
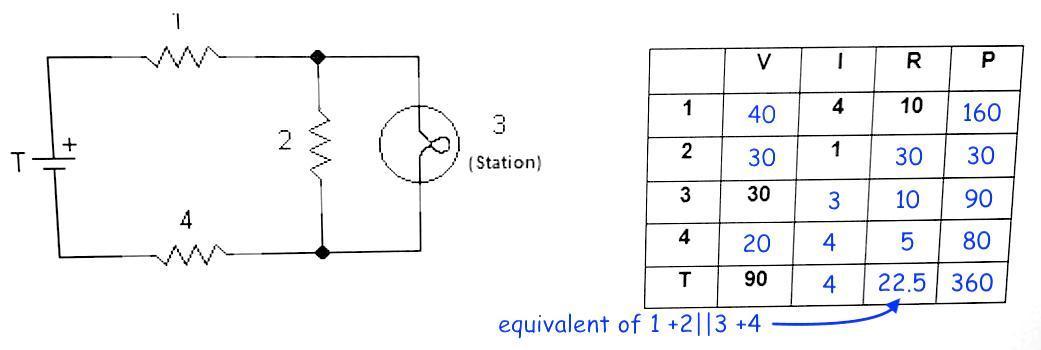
please solve :( i can’t figure it out whatsoever

Answers
Answer:
a) see attached
b) 15015 meters
Step-by-step explanation:
You want the voltage, current, resistance, and power for each component of the circuit shown in the diagram.
Voltage and current lawsThe relevant circuit relations are ...
Kirchoff's voltage law: the sum of voltages around a loop is zeroKirchoff's current law: the sum of currents into a node is zeroOhm's law: voltage is the product of current and resistanceSeries: elements in series have the same currentParallel: elements in parallel have the same voltageVoltageGiven current and resistance for element 1, we immediately know its voltage is ...
V = IR = (4)(10) = 40 . . . . volts
Given the voltage on element 3, we know that parallel element 2 has the same voltage: 30 volts.
Given the voltage at T is 90 volts, the sum of voltages on elements 1, 2, and 4 must be 90 volts. That means the voltage on element 4 is ...
90 -(40 +30) = 20
CurrentThe current in elements 1, 4, and T are all the same, because these elements are in series. They are all 4 amperes.
That 4 ampere current is split between elements 2 and 3. The table tells us that element 2 has a current of 1 ampere, so element 3 must have a current of ...
4 - 1 = 3 . . . . amperes
ResistanceThe resistance of each element is the ratio of voltage to current:
R = V/I
Dividing the V column by the I column gives the values in the R column.
Note that power source T does not have a resistance of 22.5 ohms. Rather, it is supplying power to a circuit with an equivalent resistance of 22.5 ohms.
PowerPower is the product of voltage and current. Multiplying the V and I columns gives the value in the P column.
Note that the power supplied by the source T is the sum of the powers in the load elements.
b) WavelengthWe found that the transmitter is receiving a power of 90 watts, so its operating frequency is ...
(90 W)×(222 Hz/W) = 19980 Hz
Then the wavelength is ...
λ = c/f
λ = (3×10⁸ m/s)/(19980 cycles/s) ≈ 15015 m/cycle
The wavelength of the broadcast is about 15015 meters.
__
Additional comment
The voltage and current relations are "real" and used by circuit analysts everywhere. The relationship of frequency and power is "made up" specifically for this problem. You will likely never see such a relationship again, and certainly not in "real life."
Kirchoff's voltage law (KVL) means the sum of voltage rises (as at T) will be the sum of voltage drops (across elements 1, 2, 4).
Kirchoff's current law (KCL) means the sum of currents into a node is equal to the sum of currents out of the node. At the node between elements 1 and 2, this means the 4 amps from element 1 into the node is equal to the sum of the currents out of the node: 1 amp into element 2 and the 3 amps into element 3.
As with much of math and physics, there are a number of relations that can come into play in any given problem. You are expected to remember them all (or have a ready reference).
<95141404393>

do any of y'all know how to do this I'm so confused and I need help

Answers
Answer:
x=28 bottom left angle is 60, bottom right is 70
Step-by-step explanation:
the angles add up to 180
lets set up an equation
x+32+x+42+50=180
2x+124=180
2x=56
x=28
For the following three vectors, what is 3⋅
C
⋅(2
A
×
B
)?
A
=4.00
i
^
+3.00
j
^
−3.00
k
^
B
=−3.00
i
^
+2.00
j
^
+4.00
k
^
C
=8.00
i
^
−9.00
j
^
Number Units
Answers
So the value of the vector product 3C . (2A × B) = 1242.
Given that the vectors are,
A = 4 i + 3 j - 3 k
B = - 3 i + 2 j + 4 k
C = 8 i - 9 j
So, now calculating the required we get,
2A = 2 (4 i + 3 j - 3 k) = 8 i + 6 j - 6 k
3C = 3 (8 i - 9 j) = 24 i - 27 j
So, cross product is given by,
2A × B
= \(\left[\begin{array}{ccc}i&j&k\\8&6&-6\\-3&2&4\end{array}\right]\)
= [(6) (4) - (2) (- 6)] i - [(8) (4) - (- 3) (- 6)] j + [(8) (2) - (- 3) (6)] k
= [24 + 12] i - [32 - 18] j + [16 + 18] k
= 36 i - 14 j + 34 k
So the dot product is given by
3C . (2A × B) = (24 i - 27 j) . (36 i - 14 j + 34 k ) = 24 * 36 + (- 27) * (- 14) = 1242
To know more about vector product here
https://brainly.com/question/2005068
#SPJ4
The question is not clear. The clear question will be -
"For the following three vectors, what is 3⋅C⋅(2A × B)?
A=4.00i+3.00j−3.00k; B=−3.00i+2.00j+4.00k; C=8.00i−9.00 j"
anyone question 8 pleaseeeeeeeeeee

Answers
Answer:
$200 and $450
Step-by-step explanation:
We can start by assigning variables to our unknowns.
Our unknowns are the price for a DVD player and the price for a television.
x= price of a television
y= price of a DVD player
Next, let's translate the given information into a system of equations.
two televisions and three DVD players for $1750
2x+3y=1750
four televisions and one DVD player for $1250
4x+y=1250
Since the instructions did not specify, I will use substitution to solve, however you can also use elimination.
First, from the second equation, we subtract 4x from both sides.
4x+y-4x=1250-4x
y=1250-4x
Now we plug 1250-4x for y in the first equation.
2x+3y=1750
2x+3(1250-4x)=1750
Distribute.
2x+3*1250-3*4x=1750
Simplify.
2x+3750-12x=1750
Combine like terms.
-10x+3750=1750
Subtract 3750 from both sides
-10x+3750-3750=1750-3750
-10x=-2000
Divide both sides by -10
x=200
A television costs $200.
Now using that, we can plug that into one of the original equations. I'll use the second one.
4x+y=1250
4(200)+y=1250
800+y=1250
Subtract 800 from both sides.
y=450
A DVD player costs $450
You can plug those values back into the other equation (the first one) to double-check if you'd like as well.
