How do I solve -6-(-2)-1
Answers
Answer:
-5
Step-by-step explanation:
(-6) - (-2) - 1
(-6) - (-2) = -4
-4 - 1 = -5
Mark Brainleiest if this helped! <3
Related Questions
29.4.3 Quiz: Parabolas with Vertices at the Origin
Question 5 of 10
The equation below describes a parabola. If a is negative, which way does the
parabola open?
y=ax²2²
O A. Right
B. Down
OC. Up
OD. Left
SUBMIT
Answers
The equation of a parabola with its vertex at the origin includes a negative coefficient 'a', the parabola opens downward. option B.
The equation y = ax² represents a parabola with its vertex at the origin. In this case, if the coefficient 'a' is negative, it determines the direction in which the parabola opens.
When 'a' is negative, the parabola opens downward. This means that the vertex, which is at the origin (0, 0), represents the highest point on the graph, and the parabola curves downward on both sides.
To understand this concept, let's consider the basic equation y = x², which represents a standard upward-opening parabola. As 'a' increases, the parabola becomes narrower. Conversely, when 'a' becomes negative, it flips the parabola upside down, resulting in a downward-opening parabola.
For example, if we have the equation y = -x², the negative coefficient causes the parabola to open downward. The vertex remains at the origin, but the shape of the parabola is now inverted.
In summary, when the equation of a parabola with its vertex at the origin includes a negative coefficient 'a', the parabola opens downward. This can be visually represented as a U-shape curving downward from the origin. So Optyion B is correct.
For more question on parabola visit:
https://brainly.com/question/29635857
#SPJ8
(Impossible) Twelve men are on a desert island. They all have identical weights except for one of them, who is either slightly lighter or slightly heavier than the others.
The only other thing on the island is a seesaw. There are no scales or means to measure weight otherwise. Can you determine which man has the different weight? You only get to use the seesaw three times.
Answers
Answer:
Yes, it is possible to determine which man has the different weight by using the seesaw three times. Here is one possible method
Divide the 12 men into three groups of four: A, B, and C.
Weigh group A against group B on the seesaw. (First use)
If the seesaw balances, then the odd man is in group C. Otherwise, he is in the heavier or lighter group (A or B).
Take two men from group C and weigh them against two men from group A or B that were balanced. (Second use)
If the seesaw balances again, then the odd man is one of the remaining two men from group C. Otherwise, he is one of the two men from group C that were weighed.
Weigh one of the suspected men against any other man. (Third use)
If the seesaw balances, then the odd man is the other one. Otherwise, he is the one that was weighed.
This method works because it eliminates half of the possible candidates at each step and identifies whether the odd man is heavier or lighter by comparing him with known balanced men.
Step-by-step explanation:
I need help on the Math HW. I don’t get how to get the answers.
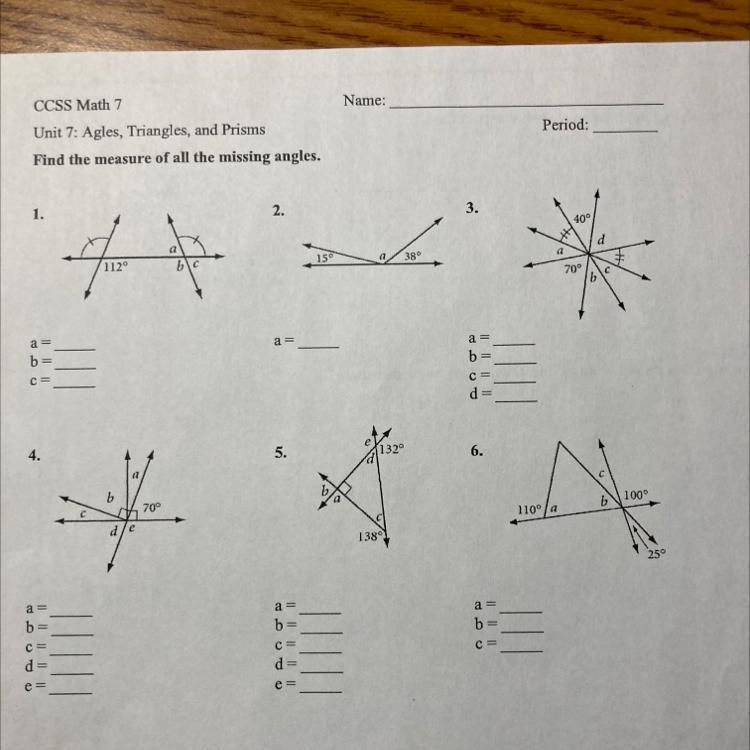
Answers
Answer:
1
A= 34
B= 112
C= 34
Step-by-step explanation:
A and C are vertical angles so would have the same angle degree. The 112 and B are same side interior angles so have the same degree
A and 112 are opposite interior angles so they have to add up to 180.
So 180-112=68
then 68 divided by 2 to get both A and C
hope that made sense
Solve the question below
-9 = x/8

Answers
Answer:
-72
Step-by-step explanation:
x= -9×8
Answer: x = -72
Step-by-step explanation:
We know that since the answer is negative, x must be negative.
So now we need to multiply -9 and 8.
-9 times 8 = -72.
The answer is -72, and this is because when you plug -72 into the numerator, you must divide it by 8.
-72 divided by 8 = -9.
What is the solution for x in the equation?
-2x + 14 + 10x = 34
A.
B.
C.
D.
Answers
Answer: 2.5
Step-by-step explanation:
-2x + 14 + 10x = 34
8x + 14 = 34
8x = 34 - 14
8x = 20
x = 20/8
x = 5/2
x = 2.5
14 +8x =34
Subtract 14 both sides
8x = 20
Divide both sides by 8
x = 20/8
Reduce
x= 5/2
x = 2 1/2 or 2.5
Landon and Maria are meeting at the library to work on their history project. Maria walks 9 blocks east and 3 blocks north to get to the library from her house. Landon walks 5 blocks south and 7 blocks west to get to the library from his house. The map below shows the location of the library and Landon's and Maria's houses. To the nearest block, how far is Landon's house from Maria's house if Maria could walk in a straight line?
Answers
To the nearest block, Landon's house is at distance of 12 blocks away from Maria's house if Maria could walk in a straight line.
What is Pythagoras theorem?A basic mathematical theorem relating to the sides of a right-angled triangle is known as Pythagoras' theorem. The square of the length of the hypotenuse, the side that faces the right angle, is said to be equal to the sum of the squares of the lengths of the other two sides, known as the legs, in a right triangle.
This can be written in mathematical notation as:
c² = a² + b²
where c is the length of the hypotenuse, and a and b are the lengths of the legs of the right triangle.
In this case, we can consider the straight line between Maria's house and Landon's house as the hypotenuse of a right triangle, with the distances they walked as the other two sides. We can use the distance formula to find the lengths of those sides:
Distance walked by Maria = √(9² + 3²) = √90 ≈ 9.49 blocks
Distance walked by Landon = √(5² + 7²) = √74 ≈ 8.60 blocks
Now we can use the Pythagorean theorem to find the distance between their houses:
Distance between houses = √(9.49² + 8.60²) ≈ 12.46 blocks
To know more about Pythagoras' theorem, visit:
https://brainly.com/question/21926466
#SPJ1
What is the equation of the line that is perpendicular to y = 3x + 3 and passes through the point (-6, 9)?
y=-1/3x+3
y=-1/3x+7
y=3x-33
y = 3x + 27
Answers
Answer:
y = - \(\frac{1}{3}\) x + 7
Step-by-step explanation:
the equation of a line in slope- intercept form is
y = mx + c ( m is the slope and c the y- intercept )
y = 3x + 3 ← is in slope- intercept form
with slope m = 3
given a line with slope m then the slope of a line perpendicular to it is
\(m_{perpendicular}\) = - \(\frac{1}{m}\) = - \(\frac{1}{3}\) , then
y = - \(\frac{1}{3}\) x + c ← is the partial equation
to find c substitute (- 6, 9 ) into the partial equation
9 = - \(\frac{1}{3}\) (-6) + c = 2 + c ( subtract 2 from both sides )
7 = c
y = - \(\frac{1}{3}\) x + 7 ← equation of perpendicular line
2 Write 386 in expanded form
Answers
Answer:
300+ 80+6
Step-by-step explanation:
i think i not sure
(3546 x 5671) + (5829 - 587)
You will get 15 points to answer this.
Answers
Answer:
201,098,906
Step-by-step explanation:
Help me please, the answer choices are on the pic

Answers
Answer:
104
Step-by-step explanation:
Let's say that the numbers are x and y. So, we know that....
x+y=186
y-x=22
Now we can use the substitution method to find the numbers. y-x=22 turns into y=22+x. And now we can substitute to the second equation into the first equation.
x+22+x=186
2x=164
x=82
y=22+82
y=104
Our numbers are 82 and 104 and the larger integer is 104, so that's the answer.
Answer:
I believe the answer is 104.
Step-by-step explanation:
Because 186 - 104 = 82 and 104 - 22 = 82.
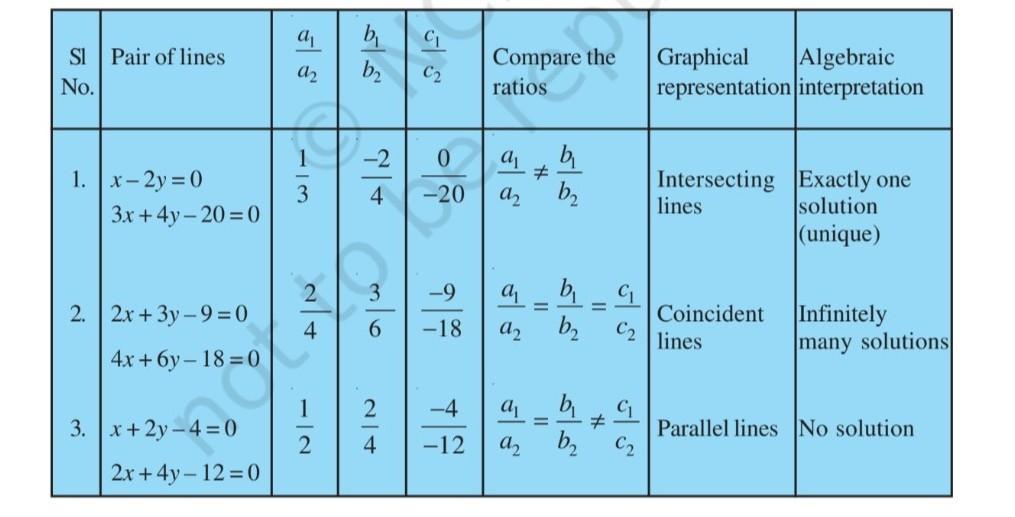
Explain how you know when an equation has only one so-
lution, no solution, or infinitely many solutions.
Answer in complete sentences.
Plsss helpppp
Answers
Answer:
i hope you can understand from given table
Q2) A company is evaluating the extension of credit to a new group of customers.
Although these customers will provide $180,000 in additional credit sales, 12% are likely
to be uncollectible. The company will also incur $16,200 in additional collection
expense. Production and marketing costs represent 72% of sales. The firm is in a 34% tax
bracket and has a receivables turnover of four times. No other asset buildup will be
required to service the new customers. The firm has a 10% desired return.
has a
a. Calculate the incremental income after taxes and the return on incremental
investment. Should the company extend credit to these customers?
b. Based on the income level determined in (a), should credit be extended if an
additional investment in inventory is considered? Assume an inventory turnover
of 1.6 times.
Answers
Answer:
Step-by-step explanation:
a. To calculate the incremental income after taxes, we need to determine the incremental costs and revenues associated with extending credit to the new customers. The additional credit sales will generate $180,000 in revenue, but we need to subtract the expected uncollectible amount of 12% * $180,000 = $21,600. The net additional credit sales will be $180,000 - $21,600 = $158,400.
The production and marketing costs will be 72% * $180,000 = $129,600. The total incremental costs will be $129,600 + $16,200 (collection expense) = $145,800.
The incremental income before taxes will be $158,400 - $145,800 = $12,600. The income tax will be 34% * $12,600 = $4,284. The incremental income after taxes will be $12,600 - $4,284 = $8,316.
To calculate the return on incremental investment, we need to determine the incremental investment required to extend credit to the new customers. Since no additional investment in assets is required, the incremental investment will be equal to the incremental costs of $145,800. The return on incremental investment will be $8,316 / $145,800 = 5.7%.
Based on the return on incremental investment of 5.7%, the company should not extend credit to the new customers, as it is below the desired return of 10%.
b. If an additional investment in inventory is required to extend credit to the new customers, we will need to recalculate the incremental income after taking into account the additional investment. If the additional investment in inventory is $x, the incremental investment will be $145,800 + $x. The incremental income before taxes will be $158,400 - ($129,600 + $16,200 + $x) = $12,600 - $x. The income tax will be 34% * ($12,600 - $x) = $4,284 - $x * 0.34. The incremental income after taxes will be ($12,600 - $x) - ($4,284 - $x * 0.34) = $8,316 - $x * 0.34.
To determine whether credit should be extended, we need to compare the return on incremental investment to the desired return of 10%. The return on incremental investment will be ($8,316 - $x * 0.34) / ($145,800 + $x). Setting this equal to the desired return of 10% and solving for x, we find that the additional investment in inventory cannot exceed $24,764 for the company to meet its desired return. If the additional investment in inventory is less than or equal to $24,764, the company should extend credit to the new customers. If it is greater than $24,764, the company should not extend credit to the new customers.
7 5/7 as a decimal please help
Answers
Answer:
the answer was 7.71
Step-by-step explanation:
hope this helps
A fraction is usually split into two parts: the first part is the number on top, called the numerator; and the second part is the number on the bottom, called the denominator. These are both separated by a line called the “divisor line”. We can use the division method help to solve this question: to get a decimal, simply divide the numerator 54 by the denominator 7 (which you can enter in any calculator):
54 (numerator) ÷ 7 (denominator) = 7.71
And finally, you get 7.71 as your answer when you convert 7 5/7 (or 54/7) to a decimal.
Answer:
7.71
Step-by-step explanation:
Find the slope of the line through the point (2,2) and (-4,2) and then graph
Answers
Given:
points (2,2) and (-4,2)
Recall the formula for determining the slope of a line given two points
\(\begin{gathered} m=\dfrac{y_2 - y_1}{x_2 - x_1} \\ \text{where} \\ (x_1,y_1)\text{ and }(x_2,y_2)\text{ are two points in the line} \end{gathered}\)Given the point (2,2) and (-4,2), the slope of the line is
\(\begin{gathered} (x_1,y_1)=(2,2) \\ (x_2,y_2)=(-4,2) \\ \\ m=\dfrac{y_2 - y_1}{x_2 - x_1} \\ m=\dfrac{2 - 2}{-4 - 2} \\ m=\dfrac{0}{-6} \\ m=0 \end{gathered}\)The slope of the line is zero.
Graphing the two point, connect the two points (2,2) and (-4,2), then draw a solid line connecting the two points and we get

Find m∠ABD and m∠CBD given m∠ABC = 77°. m∠ABD= ° and m∠CBD= °.
Answers
The value of m∠ABD is 49° and m∠CBD is 28°.
(3x + 22) + (5x - 17) = 77°
3x + 22° + 5x - 17° = 77°
Collect like terms
3x + 5x = 77° - 22° + 17°
8x = 72°
Divide both side by 8
8x/8 = 72/8
x = 9°
Since we have gotten the value of x, we put it back into the angles given.
Therefore,
m∠ABD = 3x + 22
= 3(9) + 22 = 27° + 22° = 49°
m∠CBD = 5x - 17
= 5(9) - 17
= 45° - 17°
= 28°
Read related link on:
https://brainly.com/question/24583602

simplify the expression (b+3)+(2b-2)
Answers
Answer:
if you meant (b+3)+(2^b-2), the answer is b+1+2^b
Step-by-step explanation:
but if you meant (b+3)+(2b-2), the aanswer is 3b+1
Answer:
3b+1
Step-by-step explanation:
(b+3)+(2b-2)
b+3+2b-2
3-2
b+1+2b
b+2b
3b+1
Prove the identity with rules
(1-cos(-x)) / (sec(-x)-1) =cosx

Answers
By using the rules:
\(cos(-x) = cos(x)\\\\sec(x) = \frac{1}{cos(x)}\)
We have proven the identity. Below you can follow the demonstration.
How to prove the identity?Here you need to remember two things:
\(cos(-x) = cos(x)\\\\sec(x) = \frac{1}{cos(x)}\)
Here we have the expression:
\(\frac{1 - cos(-x)}{sec(-x) - 1}\)
By using the first rule, we can rewrite:
\(\frac{1 - cos(-x)}{sec(-x) - 1} = \frac{1 - cos(x)}{sec(x) - 1}\)
By using the second rule, we can rewrite:
\(\frac{1 - cos(x)}{sec(x) - 1} = \frac{1 - cos(x)}{\frac{1}{cos(x)} - 1}\)
Now if we multiply and divide by cos(x), we get:
\(\frac{1 - cos(x)}{\frac{1}{cos(x)} - 1} = \frac{1 - cos(x)}{\frac{1}{cos(x)} - 1} *\frac{cos(x)}{cos(x) } = \frac{(1- cos(x))*cos(x)}{1 - cos(x)} = cos(x)\)
In this way, the identity was proven.
If you want to learn more about trigonometric identities:
https://brainly.com/question/7331447
#SPJ1
what is the y intercept of this graph
Answers
Answer:
The y-intercept is the point at which the graph crosses the y-axis. At this point, the x-coordinate is zero.
What is the value of the expression 30 X 5 + 6 X 4
will give brainliest
Answers
Answer:
174
Step-by-step explanation:
Answer:
30 × 5 + 6 × 4= 150 + 24= 174Step-by-step explanation:
I hope it helps ❤❤What is the area of this polygon?
16 cm2
24 cm2
40 cm2
18 cm2
Answers
Answer:
Where's the question...
sketch the curve with the given vector equation. indicate with an arrow the direction in which t increases. r(t)
Answers
Answer:Sketch the curve with the given vector equation. indicate with anarrow the direction in which t increases
r(t) = t2i +t4j +t6k
I have no idea how to go about drawing the vector. I knowthat
x=t2
y=t4
z=t6
and that a possible subsititution can be y=x2and z=x3
Step-by-step explanation:that is your answer
:)
Twelve cards are numbered from 1 to 12 and placed in a box. One card is selected at random and not replaced. Another is randomly selected. What is the probability of selecting two even numbers?
Answers
In this case, we are to determine the probability of selecting two even numbers. This can be solved as follows:There are six even numbers: {2, 4, 6, 8, 10, 12}.We can use the concept of conditional probability since the first event affects the probability of the second event. This can be expressed as follows:
In this case, P(A) represents the probability of selecting an even number, and P(B|A) represents the probability of selecting an even number given that the first card selected was even. P(A) = 6/12 = 1/2 (there are six even numbers and twelve cards in total)P(B|A) = 5/11 (there are five even numbers left in the box after the first even number is selected, and eleven cards are left in total).
The probability of selecting two even numbers can be found by multiplying these probabilities: P(A) x P(B|A) = (1/2) x (5/11) = 5/22Therefore, the probability of selecting two even numbers is 5/22.Answer: 5/22
For more questions on: probability
https://brainly.com/question/23417919
#SPJ8
Mike went to the store to get his favorite fruit grapes. One bunch of grape cost $5.20. How many bunches can he buy for $26 ?
Answers
Answer:
He can buy 4 bunches of his grapes.
Step-by-step explanation:
PLEASE PLEASE HELP ME IVE BEEN STRUGGLING FOR THE PAST 12 HOURS IF CORRECT ILL CASHAPP 5$ I PROMISE

Answers
Answer:
First Bed: 15 white 20 pink
Second Bed: 30 white and 60 pink= 90 hydrangeas
Total Flowers: 45 white and 80 pink
Step-by-step explanation:
Questions like these are normally jsut trial and error. If the numbers that are given to you end with a 5 or 0, you should experiment with numbers that end in a 5 or 0.
En la final de un concurso escolar de
matemática participan 6 alumnos de los
cuales 3 son alumnos del colegio A. Si se
premia a los dos primeros con regalos
diferentes, entonces la probabilidad de que
los alumnos del colegio A obtengan los dos
premios (no hay empates), es:
Answers
Usando la distribución hipergeométrica, la probabilidad de que los alumnos del colegio A obtengan los dos premios (no hay empates), es: 0.2 = 20%.
¿Qué es la fórmula de distribución hipergeométrica?La fórmula es:
\(P(X = x) = h(x,N,n,k) = \frac{C_{k,x}C_{N-k,n-x}}{C_{N,n}}\)
\(C_{n,x} = \frac{n!}{x!(n-x)!}\)
Los parámetros son:
x es el número de éxitos.N es el tamaño de la población.n es el tamaño de la muestra.k es el número total de resultados deseados.Los valores de los parámetros son:
N = 6, k = 3, n = 2.
La probabilidad de que los alumnos del colegio A obtengan los dos premios (no hay empates), es P(X = 2), por eso:
\(P(X = x) = h(x,N,n,k) = \frac{C_{k,x}C_{N-k,n-x}}{C_{N,n}}\)
\(P(X = 2) = h(2,6,2,3) = \frac{C_{3,2}C_{3,0}}{C_{6,2}} = 0.2\)
Puede-se aprender más a cerca de la distribución hipergeométrica en https://brainly.com/question/24826394
#SPJ1
If each of these runners travels the indicated number of spaces in the same amount of time, at which numbered spot will all of the runners be next to one another?

Answers
The numbered spot at which all the runners will be next to one another is spot 19.
What is the LCM?Least Common Multiple is the meaning of the acronym LCM. The lowest number that may be divided by both numbers is known as the least common multiple (LCM) of two numbers. It may also be computed using two or more real numbers.
Starting with the runner on the outside track, the provided parameters are;
The runner covered n₁ = 5 places on the outside track, which is the number of spaces.
Next, the inner runner will traverse n₂ spaces, which equals one space.
The following inner runner will cover n₃ = 3 spaces.
The subsequent runner will traverse n₄ = 2 spaces.
The Lowest Common Multiple, or LCM, of all the runners' speeds or the total number of spaces they cover in the same amount of time, determines where all the runners will be placed next to one another.
LCM(5, 1, 3, 2) = 30 is the LCM of 5, 1, 3, and 2.
Time = 30/ = 6
Consequently, when the first runner has covered 30 places, we have;
Six time units have been expended.
The runner comes to a stop at position 30- (30 -19) = Position 19.
First runner's new destination is Spot 19.
The distance covered simultaneously by runner 2 is 6 x 1 = 6.
The distance covered by two runners running simultaneously equals six spaces.
Second runner's new position: 6 spaces plus spot 13 equals spot 19.
The combined distance covered by the three runners is 6 x 3 = 18.
The distance runner 3 covers 18 spaces simultaneously.
Third runner's new position: 18 spaces + Spot 1 = 19 spaces
Runner 4 covers a distance of 6 x 2 = 12 at the same time.
Distance runner 4 journeys equals 12 spaces
Runner 4's new position is now 12 spaces Plus Spot 7 = Spot 19.
Therefore, all the runners will be next to one another is spot 19.
To learn more about the LCM;
https://brainly.com/question/20739723
#SPJ1
50 Points! Multiple choice algebra question. Find the domain and range of the function whose graph is shown. Photo attached. Thank you!

Answers
Answer:
"B". Domain is all real numbers, and the Range is all positive real numbers
Step-by-step explanation:
It is important to recognize that this function is an exponential function, either by the graph (observe a horizontal asymptote on the x-axis, and increasing exponentially), or more importantly by the equation which is in exponential form \(y=a*b^x\) where "a" is a non-zero real number, and "b" is a positive real number not equal to 1.
Observe that for the given function, a=4 (a real number not equal to zero), and b=2 (a positive real number that is not 1).
The domain for all exponential functions is all real numbers, so this function's domain is all real numbers.
The Range for exponential functions depends on "a", where if "a" is a positive number the Range is positive numbers only, and if "a" is a negative number, the Range is negative numbers only.
Since "a" is positive, the Range is positive numbers only.
Writing the Domain in "set-builder notation" (since all of the choices are given using that notation), the Domain is "all real numbers" put into curly brackets, so {all real numbers}.
Writing the Range in "set-builder notation", recall that the Range is the outputs of the function, so the Range is "y values such that y is greater than zero". There is some shorthand used, where the phrase "such that" is symbolized using a short vertical line (common in set-builder notation), and the phrase "y is greater than zero" is shortened using inequality symbols "y>0". So, the Range is written as { y | y>0 }.
Further, the "Domain" and "Range" are abbreviated with "D" and "R" respectively.
Therefore, the final answer would be D = {all real numbers}; R = { y | y>0 }, which is answer "B"
The supplement of an angle is 42 degrees less than 3 times the complement of the angle. What is the measure of the angle?
Answers
Answer: 24°
Step-by-step explanation:
Given the following :
supplement of an angle is 42 degrees less than 3 times the complement of the angle
Let the angle = θ
Supplement of angle = 180° - θ
Complement of angle = 90° - θ
Supplement of angle is also said to be:
(3 × complement) - 42° = 3(90° - θ) - 42°
= 270° - 3θ - 42° = 228° - 3θ
Hence,
180° - θ = 228° - 3θ
Solve for θ
-θ + 3θ = 228° - 180°
2θ = 48°
θ = 48°/2
θ = 24°
what is 1/4 divided by 2
Answers
Answer:
1/8 because pretend that you have 1/4 of a pie you divide that quarter by two you have 1/8.
Answer:
1/4 ÷ 2 = 1/8 in fraction form. 1/4 ÷ 2 = 0.125 in decimal form.
Step-by-step explanation:
Hope it helps :)
Please answer this correctly

Answers
Answer:
50 km
Step-by-step explanation:
As the figures are given similar ,
56/v = 84/75
v = 56 × 75/84
v = 50 km
Answer:
v = 50
Step-by-step explanation:
The trapezoids are similar, so set up a proportion like so:
\(\frac{56}{v} =\frac{84}{75}\)
→Cross multiply:
\(\frac{4200}{84v}\)
→Divide 4200 by 84:
v = 50