Answers
Related Questions
The graphs of the functions f and g are shown in the figure.
The x y coordinate plane is given. There are 2 functions on the graph.
The function labeled f consists of 3 line segments. Function f begins at the point (−2, 0.5), goes linearly down and right to the origin where it sharply changes direction, goes linearly up and right, passes through the point (1, 2), sharply changes direction at the point (2, 4), goes linearly down and right, passes through the point (5, 3), and ends approximately at the point (7, 2.3).
The function labeled g consists of 2 line segments. Function g begins at the point (−2, 4), goes linearly down and right, passes through the point (-1, 3), crosses the y-axis at y = 2, passes through the point (1, 1), sharply changes direction at the point (2,0), goes linearly up and right, passes through the point (5, 2), and ends approximately at the point (7, 3.2).
Let u(x) = f(x)g(x) and
v(x) =
f(x)
g(x)
.
Answers
The output of each function include the following:
u'(1) = 0.
v'(5) = -2/3
How to determine the output of each function?By critically observing the graph of the functions f and g, we can logically deduce the following parameters;
f(1) = 2 f(5) = 3
g(1) = 1 g(5) = 2
f'(1) = 2 f'(5) = -1/3
g'(1) = -1 g'(5) = 2/3
Next, we would take the first derivative of u with respect to x and then, substitute the x-value into the composite function, and then evaluate as follows;
u(x) = f(x)g(x)
u'(x) = f'(x)g(x) + g'(x)f(x)
u'(1) = f'(1)g(1) + g'(1)f(1)
u'(1) = 2(1) + (-1)2
u'(1) = 2 - 2
u'(1) = 0.
For v'(5), we have the following function by applying quotient rule:
\(v'(x) = \frac{f'(x)g(x)\;-\;f(x)g'(x)}{g^2(x)} \\\\v'(5) = \frac{f'(5)g(5)\;-\;f(5)g'(5)}{g^2(5)} \\\\v'(5) = \frac{\frac{-1}{3} \times 2 - (3 \times \frac{2}{3}) }{2^2}\)
v'(5) = -8/3 × 1/4
v'(5) = -2/3
Read more on function here: https://brainly.com/question/31048166
#SPJ1
Missing information:
The question is incomplete and the complete question is shown in the attached picture.

the leftmost end point of a line segment must be specified as the start point of the line. true false
Answers
The statement "the leftmost end point of a line segment must be specified as the start point of the line." is true because it extends infinitely in both directions and does not have a starting or ending point.
To specify a line segment, we must indicate the points that define its endpoints. The leftmost endpoint of a line segment is significant because it establishes the relative position of the segment in space.
Furthermore, it is essential to remember that the order of the endpoints of a line segment matters. Reversing the order of the endpoints changes the direction of the line segment. For instance, the line segment AB is not the same as the line segment BA.
Therefore, specifying the leftmost endpoint is critical because it establishes the direction of the line segment.
To know more about line segment here
https://brainly.com/question/30072605
#SPJ4
find the equations of the osculating circles of the ellipse 25x2 4y2 = 100 at the points (2, 0) and (0, 5). (2, 0)
Answers
To find the equations of the osculating circles of the ellipse 25x^2 + 4y^2 = 100 at the points (2,0) and (0,5),
we need to find the radius of curvature at these points and use the formula for the equation of the osculating circle.
We start by finding the second derivatives of the ellipse with respect to x and y:
d^2x/dy^2 = -25x/(2y)^3
d^2y/dx^2 = -4y/(25x)^3
At the point (2,0), we have x = 2 and y = 0, so:
d^2x/dy^2 = 0
d^2y/dx^2 = -4/(25*2^3) = -1/50
The radius of curvature at this point is given by:
R = ((1 + (dy/dx)^2)^(3/2))/|d^2y/dx^2| = ((1 + 1/2500)^(3/2))/(1/50) = 50√2501/2500
Therefore, the equation of the osculating circle at (2,0) is given by:
(x - 2)^2 + y^2 = (50√2501/2500)^-1
Simplifying, we get:
(x - 2)^2 + y^2 = 100/2501
Similarly, at the point (0,5), we have x = 0 and y = 5, so:
d^2x/dy^2 = -25/(2*5)^3 = -1/200
d^2y/dx^2 = 0
The radius of curvature at this point is given by:
R = ((1 + (dy/dx)^2)^(3/2))/|d^2x/dy^2| = ((1 + 1/400)^(3/2))/(1/200) = 100√401/401
Therefore, the equation of the osculating circle at (0,5) is given by:
x^2 + (y - 5)^2 = (100√401/401)^-1
Simplifying, we get:
x^2 + (y - 5)^2 = 400/401
Hence, the equations of the osculating circles at the points (2,0) and (0,5) are (x - 2)^2 + y^2 = 100/2501 and x^2 + (y - 5)^2 = 400/401, respectively
Learn more about radius here:brainly.com/question/12923242
#SPJ11
see image for question. HELP PLEASE

Answers
Answer: the last question at the very bottom
Step-by-step explanation:
the graph is not a scatter plot, so its a line plot .
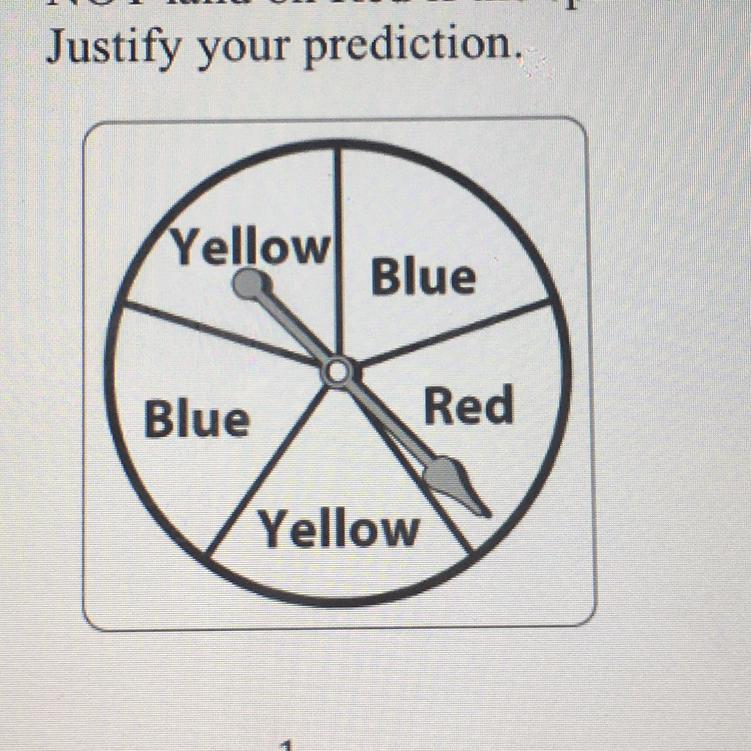
PLSS HELP ASAP!!! WILL GIVE YOU BRAINLYEST!!
How many times would you expect the spinner to
NOT land on Red if the spinner is spun 40 times?
Justify your prediction.
A. 8 times; 1/5 x 40 is 8
B. 16 times; 2/5 x 40 is 16
C. 24 time; 3/5 x 40 is 24
D. 32 times; 4/5 x 40 is 32
Answers
Answer:
8
Step-by-step explanation:
im not sure how to justify but...
there are 5 'slices'
if it spins 40 times that would be 40 divided by 5 (i think?)
40/5 = 8
please help me with this

Answers
Answer:
Because when you divide 100 by 4 and 50 by 2, you will get the same value
Help me pleasee... thank you sm in advance.

Answers
Please help! I’ll mark you brainlist
Question: a relation is not a function when
A: an output is paired with input of zero
B: an input is paired with more than one output
C: an input is paired with an output of zero
D: an output is paired with more than one input
Answers
Answer:
B
Step-by-step explanation:
a relation is not a function when input value leads to two or more outputs
Find the directional derivative of the function at the given point in the direction of the vector v.
f(x, y) = 7 e^(x) sin y, (0, π/3), v = <-5,12>
Duf(0, π/3) = ??
Answers
The directional derivative of the function at the given point in the direction of the vector v are as follows :
\(\[D_{\mathbf{u}} f(\mathbf{a}) = \nabla f(\mathbf{a}) \cdot \mathbf{u}\]\)
Where:
- \(\(D_{\mathbf{u}} f(\mathbf{a})\) represents the directional derivative of the function \(f\) at the point \(\mathbf{a}\) in the direction of the vector \(\mathbf{u}\).\)
- \(\(\nabla f(\mathbf{a})\) represents the gradient of \(f\) at the point \(\mathbf{a}\).\)
- \(\(\cdot\) represents the dot product between the gradient and the vector \(\mathbf{u}\).\)
Now, let's substitute the values into the formula:
Given function: \(\(f(x, y) = 7e^x \sin y\)\)
Point: \(\((0, \frac{\pi}{3})\)\)
Vector: \(\(\mathbf{v} = \begin{bmatrix} -5 \\ 12 \end{bmatrix}\)\)
Gradient of \(\(f\)\) at the point \(\((0, \frac{\pi}{3})\):\)
\(\(\nabla f(0, \frac{\pi}{3}) = \begin{bmatrix} \frac{\partial f}{\partial x} (0, \frac{\pi}{3}) \\ \frac{\partial f}{\partial y} (0, \frac{\pi}{3}) \end{bmatrix}\)\)
To find the partial derivatives, we differentiate \(\(f\)\) with respect to \(\(x\)\) and \(\(y\)\) separately:
\(\(\frac{\partial f}{\partial x} = 7e^x \sin y\)\)
\(\(\frac{\partial f}{\partial y} = 7e^x \cos y\)\)
Substituting the values \(\((0, \frac{\pi}{3})\)\) into the partial derivatives:
\(\(\frac{\partial f}{\partial x} (0, \frac{\pi}{3}) = 7e^0 \sin \frac{\pi}{3} = \frac{7\sqrt{3}}{2}\)\)
\(\(\frac{\partial f}{\partial y} (0, \frac{\pi}{3}) = 7e^0 \cos \frac{\pi}{3} = \frac{7}{2}\)\)
Now, calculating the dot product between the gradient and the vector \(\(\mathbf{v}\)):
\(\(\nabla f(0, \frac{\pi}{3}) \cdot \mathbf{v} = \begin{bmatrix} \frac{7\sqrt{3}}{2} \\ \frac{7}{2} \end{bmatrix} \cdot \begin{bmatrix} -5 \\ 12 \end{bmatrix}\)\)
Using the dot product formula:
\(\(\nabla f(0, \frac{\pi}{3}) \cdot \mathbf{v} = \left(\frac{7\sqrt{3}}{2} \cdot -5\right) + \left(\frac{7}{2} \cdot 12\right)\)\)
Simplifying:
\(\(\nabla f(0, \frac{\pi}{3}) \cdot \mathbf{v} = -\frac{35\sqrt{3}}{2} + \frac{84}{2} = -\frac{35\sqrt{3}}{2} + 42\)\)
So, the directional derivative \(\(D_{\mathbf{u}} f(0 \frac{\pi}{3})\) in the direction of the vector \(\mathbf{v} = \begin{bmatrix} -5 \\ 12 \end{bmatrix}\) is \(-\frac{35\sqrt{3}}{2} + 42\).\)
To know more about derivative visit-
brainly.com/question/31422048
#SPJ11
LetX1, ..., Xn denote a random sample from a distribution with density f(x; 1) = 3.x2 150-23/13 = { x > 0 else 0 and cumulative distribution function e-23/13 -e F(0:1) = { : : x > 0 else 0 (a) Find the distribution of e-X°/13. Explain your reasoning. (b) Find the distribution of Q i į X3. Explain your reasoning. Is Q a pivot? = 20 = (C) Suppose we observe the statistic x = 480. Use this observation to construct a 96% confidence interval for 1. i=1
Answers
(a) The distribution of e^(-X/13) is an exponential distribution with parameter λ = 1/13.
To see this, note that if Y = e^(-X/13), then the cumulative distribution function of Y is given by F_Y(y) = P(Y ≤ y) = P(e^(-X/13) ≤ y) = P(-X/13 ≤ ln(y)) = F_X(-13 ln(y)), where F_X is the cumulative distribution function of X.
Since X has a density function f_X(x) = 3x^2/150 e^(-23x/13)I_{x>0}, we have F_X(x) = (1 - e^(-23x/13))(I_{x>0}), and so F_Y(y) = (1 - e^(23 ln(y)/13))(I_{y>0}) = (1 - y^(23/13))(I_{y>0}), which is the cumulative distribution function of an exponential distribution with parameter λ = 1/13.
(b) The distribution of Q = X_1 + X_2 + X_3 is a gamma distribution with parameters α = 3 and β = 150/23.
To see this, note that the joint density function of X_1, X_2, and X_3 is given by f(x_1, x_2, x_3) = (3/150)^3 x_1^2 x_2^2 x_3^2 e^(-23/13(x_1 + x_2 + x_3))I_{x_1>0, x_2>0, x_3>0}.
Integrating out x_1 and x_2 gives the marginal density function of X_3, which is f_X3(x_3) = (3/150)^3 x_3^2 e^(-23/13 x_3)I_{x_3>0}, which is the density function of a gamma distribution with parameters α = 3 and β = 150/23. Therefore, Q = X_1 + X_2 + X_3 has a gamma distribution with parameters α = 3 and β = 150/23.
(c) Using the given observation x = 480, we can construct a 96% confidence interval for the parameter θ using the formula (x ± z_{α/2} σ /sqrt(n)), where z_{α/2} is the 96/2 = 48th percentile of the standard normal distribution, σ^2 = Var(X_1) = 150/23^2, and n = 3 is the sample size.
Using a table of the standard normal distribution, we find z_{α/2} = 1.75. Therefore, the 96% confidence interval for θ is (480 - 1.75(150/23)/sqrt(3), 480 + 1.75(150/23)/sqrt(3)) = (368.7, 591.3).
For more questions like Function click the link below:
https://brainly.com/question/16008229
#SPJ11
An elementary school has 1,134 seeds. The seeds will be planted in 27 rows.
Each row will have the same number of seeds. How many seeds will be planted
in each row?
Answers
You divide 1,134 by 27 and get 42 as your answer
Answer:
42 seeds
Step-by-step explanation:
You would first divide 1,134 seeds by 27 rows. You would get 42 seeds per row, and that would be your answer.
Using vertex form write an equation for the parabola that passes through the point ( 2 , 5 ) and has a vertex ( 1 , 3 ).
Answers
The equation of the parabola is y = 2(x - 1)² + 3
How to determine the equation of the parabola?From the question, we have the following parameters that can be used in our computation:
Vertex = (1. 3)
Point = (2, 5)
These parameters can be expressed as
(h, k) = (1, 3)
(x, y) = (2. 5)
A parabola can be represented as
y = a(x - h)² + k
Substitute the known values in the above equation, so, we have the following representation
y = a(x - 1)² + 3
Next, we have
5 = a(2 - 1)² + 3
Evaluate the difference and the exponent
5 = a + 3
So, we have
a = 2
Substitute a = 2 in y = a(x - 1)² + 3
y = 2(x - 1)² + 3
Hence, the equation is y = 2(x - 1)² + 3
Read more about parabola at
https://brainly.com/question/1480401
#SPJ1
In triangle ABC, angle ABC = 90º, and point D lies on segment BC such that AD is an angle bisector. If AB = 12 and BD = 4, then find AC
Answers
In triangle ABC, AB = 12, BD = 4, BC = 8 Then the length of AC = 14.42 units.
In triangle ABC, angle ABC = 90º, which means it's a right triangle. Point D lies on segment BC, with AD as an angle bisector. Given AB = 12 and BD = 4, we need to find AC.
Since AD is an angle bisector, it divides angle BAC into two congruent angles. In right triangles, angle bisectors also divide the opposite side (BC) proportionally to the adjacent sides (AB and AC). Let CD = x. Thus:
BD/AB = CD/AC
4/12 = x/AC
x = (4 * AC)/12
Now, apply the Pythagorean theorem for right triangle ABC:
AB² + BC² = AC²
12² + (4 + x)² = AC²
Substitute x from the proportion:
12² + (4 + (4 × AC)/12)² = AC²
144 + (4 + (4 × AC)/12)² = AC²
Solve for AC:
12² + 8² = AC²
144 + 64 = AC²
208 = AC²
Taking square root on both the sides
√208 = AC
14.42 units = AC
So, the length of side AC in triangle ABC is approximately 14.42 units.
To learn more about triangle click here
brainly.com/question/2773823
#SPJ11
Evaluate the expression

Answers
Answer:
x = - 3
Step-by-step explanation:
note that y = f(x)
locate y = 3 on the y- axis, go horizontally across to meet f(x) at (- 3, 3 ) , then
x = - 3 when f(x) = 3
How do you find the derivative of the function using the definition of derivative f(x)=10?
Answers
The derivative of of f(x) = 10 is 0
The derivative of a function is the measure of the rate of change of the function. They are fundamental to the solution of problems in calculus and differential equations.
Here the function is not changing as 10 is a constant. So, if f(x) = c where c is a constant then f'(x) = 0 as the derivative of a constant is always zero.
Mathematically:
d(f(x))/dx = \(\lim_{h \to 0}\) (f(x+h) - f(x))/h
There is no x to substitute for x + h so no difference is noticed:
\(\lim_{h \to 0}\) (f(x+h) - f(x))/h = \(\lim_{h \to 0}\) (10 - 10)/h = 0
Hence, the derivative of f(x) = 10 is 0
To know more about Derivatives here:
brainly.com/question/25324584
#SPJ4
The box plot below represents some data set. What percentage of the data values are between 50 and 75?

Answers
Answer:
25
Step-by-step explanation:
For box plot, the box contains 50percent and the line is the 50percent for the whole plot
25% of the data values are between 50 and 75.
Important information:
A box plot that represents some data set.We need to find, what percentage of the data values are between 50 and 75.
Box plot:From the given box plot it is clear that 50 is the median and 75 is the third quartile.
We know that 25% of data values are between the median and the third quartile.
Thus, 25% of the data values are between 50 and 75.
Find out more about 'box plot' here:
https://brainly.com/question/1523909
Juan walks up a hill to 30 3/4 ft above sea level.He kicks a rock that falls to 18.5 feet below sea level.what is the vertical distance the rock traveled.
Answers
Answer: -49.25 feet below sea level
Explanation: 30.75 - 30.75 - 18.5 = -49.5
Hope this helps!
What is the approximate volume of the cylinder? Use 3.14 for pie
Answers
Answer:
2×pi×radius²
thats it hope this help
If g( 2x + 1) = 4x-3 then find f(x) and f(-2).
Answers
Answer:
Dont know if this is correct but:
f(x) = 4x-3
f(-2) = 4(-2)-3
f(-2) = -11
Step-by-step explanation:
what is the answer to this

Answers
Answer:
96
Step-by-step explanation:
Can anyone help me it is urgent
I just need help with (c) and the other green part I think is what is going to help answer

Answers
Answer:
Yes
See explanation below
Step-by-step explanation:
Solving (c)
We have the following scenario:
Step Number of squares
1 1² + 1 = 2
2 2² + 2 = 6
3 3² + 3 = 12
The relationship between step number and squares is
Step n = n² + n squares
This is a quadratic expression since the highest power is 2(degree of the expression)
YES is the answer
cual es la expresión equivalente a 6(12+8)
Answers
Answer: 20(6)
Step-by-step explanation: 20 times 6 because 12+8 equals 20 and you would multiply it by 6.
Jasmine has a budget of 600 to spend on home renovations. She will spend 175 on a new sink and the remainder on countertops. The countertops cost $35 per square foot. What is the maximum number of square feet jasmine can purchase
Answers
Answer:
The maximum number of square feet Jasmine can purchase is 12 square feet
Step-by-step explanation:
Given:
Jasmine has a budget of $600.00 to spend on home renovations.
She will spend $175.00 on a new sink and the remainder on countertops.
The countertops cost $35 per square foot.
Now, to get the maximum number of square feet Jasmine can purchase.
Now, we find the remainder amount to be spend on countertops:
$600 - $175 = $425.
Remainder amount = $425.
Cost of per square foot countertops = $35.
Now, to get the number of square feet which can purchase with $425:
Estimating value = 12 square feet.
Therefore, the maximum number of square feet Jasmine can purchase is 12 square feet.
Emma pays $2,600 for a washer/dryer combo. Each year, the appliance loses 15% of its resale value. How much will it be worth in 4 years?
If necessary, round your answer to the nearest cent.
Answers
The value for the washer/dryer combo after 4 years is 1,357.2 dollars.
How much will it be worth in 4 years?We know that Emma pays $2,600 for a washer/dryer combo. Each year, the appliance loses 15% of its resale value, then the value after x years is modeled by the exponential decay.
V(x) = 2,600*(1 - 0.15)ˣ
The value after 4 years is what we get when we evaluate this in x = 4, we will get:
V(4) = 2,600*(1 - 0.15)⁴
Simplify that:
V(4) = 1,357.2
The value is after 4 years of the washer/dryer is 1,357.2 dollars.
Learn more about exponential decays at:
https://brainly.com/question/27822382
#SPJ1
when building a house, the number of days required to build is inversely proportional with the number of workers. one house was built in 133 days by 4 workers. how many days would it take yo build a similar house with 28 workers?
Answers
Answer:
28 workers will build the house in 19 days.
Step-by-step explanation:
Inverse proportion:x*y = k
Where x is the number of workers and y is the number of days
Number of workers Number of days
4 133
28 ? d
When x = 4 and y = 133
k = 4 * 133
Let the number of days to build the house by 28 workers be 'd'.
\(d = \dfrac{k}{28}\\\\\\d =\dfrac{133*4}{28}\\\\\\d = 19 \ days\)
You deposit $4600 in an account that earns 3% per year simple interest. The
equation that represents this situation is:
A(n) = 4600+ (n-1)(0.03 4600)
How much will you have in the account at the beginning of the 9th year?
Round your answer to the nearest dollar.
OA. $5704
OB. $5842
OC. $4738
OD. $4710
Answers
at the beginning of the 9th year, meaning at the end of the 8th year, or we can say 8 years later.
\(~~~~~~ \textit{Simple Interest Earned Amount} \\\\ A=P(1+rt)\qquad \begin{cases} A=\textit{accumulated amount}\\ P=\textit{original amount deposited}\dotfill & \$4600\\ r=rate\to 3\%\to \frac{3}{100}\dotfill &0.03\\ t=years\dotfill &8 \end{cases} \\\\\\ A = 4600[1+(0.03)(8)] \implies A = 4600(1.24) \implies A = 5704\)
suppose the null hypothesis, h0, is a surgical procedure is successful at least 80% of the time. and the alternative hypothesis, ha, states the doctors' claim, which is a surgical procedure is successful less than 80% of the time. what is the type ii error in this scenario?
Answers
In this scenario, the null hypothesis states that a surgical procedure is successful at least 80% of the time, while the alternative hypothesis claims that it is less than 80% successful.
In hypothesis testing, Type II error occurs when the null hypothesis is not rejected even though it is false. The Type II error, denoted by β, would occur if we fail to reject the null hypothesis even though it is false, i.e., when the actual success rate of the surgical procedure is less than 80%.
Therefore, β represents the probability of accepting the null hypothesis when the alternative hypothesis is true. It is also known as the false negative rate, as it occurs when we fail to detect a significant difference between the sample and population due to random chance or other factors.
The value of β depends on various factors, such as the sample size, significance level, and effect size. To calculate β, we need to specify these values and use statistical software or tables to find the probability of Type II error.
To learn more about error click on,
https://brainly.com/question/13138172
#SPJ1
Kara compared the number of text messages 100 students sent in one day and the grade point average (GPA) of each student. The correlation coefficient among the
data is -0.9 rounded to the nearest tenth. Based on this information, which statement is MOST likely true?
OA. There is correlation but not causation between GPA and the number of text messages.
B. There is causation but not correlation between GPA and the number of text messages.
OC There is both correlation and causation between GPA and the number of text messages.
D. There is neither correlation nor causation between GPA and the number of text messages.
Answers
There is correlation but not causation between GPA and the number of text messages. that is option A is correct.
The correlation coefficient is defined.How one variable changes in relation to another is indicated by the correlation coefficient. A score of 1 shows a complete positive correlation, with a positive correlation being one where both variables move in the same direction. While 0 indicates there is no linear connection, a number of -1 indicates a complete negative correlation.
Given to us is that The data have a -0.9 correlation coefficient.
That is, there is a significant inverse relationship between the quantity of texts and each student's grade point average (GPA).
Hence, choice A is right.
There is correlation but not causation between GPA and the number of text messages.
option B,C,D are incorrect because we know that correlation does not imply causation that is correlation does not imply a cause and effect between the two variable. correlation between variables means that relationship between two variables not the change in one variable , is the cause of the change in the variable. causation indicates that one event is the result of the occurrence of the other event.
To know more about correlation coefficient click:
brainly.com/question/27948939
#SPJ1
ch02 04 given wins = a0 a1 x population e1 . what is the regression term that describes a0 in the equation?
Answers
a0 is the regression term that describes the constant or intercept in the linear regression equation.
In a simple linear regression model, the equation takes the form of y = a0 + a1x + e1, where y is the dependent variable (or response variable), x is the independent variable (or predictor variable), a0 is the intercept or constant term, a1 is the coefficient of the independent variable, and e1 is the error term.
The intercept term, a0, represents the value of the dependent variable when the independent variable is zero. For example, in a linear regression model that predicts salary based on years of experience, the intercept would represent the starting salary for someone with zero years of experience. The intercept is an important component of the regression equation because it allows us to make predictions for values of x that are outside the range of our observed data.
The coefficient, a1, represents the change in the dependent variable for each one-unit increase in the independent variable. In the salary example, the coefficient would represent the average increase in salary for each additional year of experience.
Both the intercept and coefficient are estimated from the data using methods such as least squares regression. Once these values are estimated, we can use them to make predictions for new values of x.
Learn more about equation from
https://brainly.com/question/29174899
#SPJ11
g every time the system transitions it is equally likely to choose any of the three modes. what is the expected time taken for the system to failin
Answers
Therefore, the expected time for the system to fail is the weighted average of the failure times of the three modes, with equal weights assigned to each mode.
the expected time for a system to fail, given that it can transition between three modes with equal probability. To calculate the expected time, we'll use the concept of expected value.
Let's assume the failure times for the three modes are T1, T2, and T3, and the probability of choosing each mode is 1/3, since it's equally likely.
The expected time taken for the system to fail can be calculated by multiplying the failure time of each mode with its respective probability and then adding the products together:
Expected Time = (T1 * 1/3) + (T2 * 1/3) + (T3 * 1/3)
Therefore, the expected time for the system to fail is the weighted average of the failure times of the three modes, with equal weights assigned to each mode.
To learn more about the average visit:
https://brainly.com/question/20118982
#SPJ11
