HELP
9 •8 +16 / 4
Rewrite the expression so that addition is performed first.
9 •8 +16 / 4
Rewrite the expression so that division is performed first.
Answers

Related Questions
An equation is given.
2
x
=
26
2x=26
Which calculation would need to be done to solve the equation?
A
divide both sides by 2626
B
multiply both sides by 2626
C
divide both sides by 22
D
multiply both sides by 2
Answers
D because 2 has a x next to it so you divide by 2 on both sides
Find the accumulated amount of P3,500 invested 6.25% compound
monthly at for a period of 5 years.
Answers
The accumulated amount of P3,500 invested at a compound interest rate of 6.25% compounded monthly for a period of 5 years is approximately P4,579.79.
To calculate the accumulated amount, we can use the formula for compound interest:
\(A = P(1 + r/n)^{nt}\)
Where:
A is the accumulated amount
P is the principal amount (initial investment)
r is the annual interest rate (in decimal form)
n is the number of times interest is compounded per year
t is the number of years
In this case, the principal amount (P) is P3,500, the annual interest rate (r) is 6.25% (or 0.0625 in decimal form), the interest is compounded monthly (n = 12), and the period of investment (t) is 5 years.
Plugging in the values, we have:
\(A = 3500(1 + 0.0625/12)^{12*5}\)
Calculating this expression, we find that the accumulated amount (A) is approximately P4,579.79.
Therefore, after 5 years of investing P3,500 at a compound interest rate of 6.25% compounded monthly, the accumulated amount is approximately P4,579.79.
Learn more about interest rate visit:
brainly.com/question/29294869
#SPJ11
In the​ U.S., shoe sizes are defined differently for men and​ women, but in​ Europe, both sexes use the same shoe size scale. The accompanying histogram shows the European shoe sizes of 269 male and female college​ students, converted from their reported U.S. shoe sizes. What might be the problem with either the mean or the median as a measure of​ center?
Answers
To accurately represent men's and women's shoe sizes separately, it is recommended to calculate and compare the average or median shoe size for each group separately.
The problem with the central measure, mean or median, in this case is that the data is not gender-specific and there is a possibility that male and female shoe size distributions are different. Therefore, calculating the average or median shoe size for all students may not accurately reflect the typical male and female shoe sizes, respectively.
For example, if the males in the sample have, on average, a larger shoe size than the females, then the average shoe size of all students might be biased towards the larger size, even if most of the students are female. On the other hand, if you have several boys with very large shoe sizes, the median shoe size of all students may be biased toward the larger size, even if most of the students are women with small shoe sizes.
To accurately represent men's and women's shoe sizes separately, it is recommended to calculate and compare the average or median shoe size for each group separately. Alternatively, a fashion report representing the most common shoe sizes in the data might be a better measure of the centroid.
Learn more about Median:
https://brainly.com/question/28060453
#SPJ4
Solve x^2 -24 = -80 by completing the square.
What is the solution set of the equation?
A.(2,40)
B.(4,20)
C.(5,16)
D.(8,10)
Answers
Answer:
B.(4,20)
Step-by-step explanation:
Given: \(x^2 -24x = -80\)
To solve the quadratic equation by completing the square, we follow these steps.
Step 1: Divide the coefficient of x by 2
\(-\dfrac{24}{2}=-12\)
Step 2: Square your result fro Step 1
\((-12)^2\)
Step 3: Add the result form step 2 to both sides of the equation
\(x^2 -24x+(-12)^2 = -80+(-12)^2\)
Step 4: Rewrite the Left hand side in the form \((x+k)^2\)
\((x-12)^2=-80+144\\(x-12)^2=64\)
Step 5: Take square roots of both sides
\(x-12=\pm \sqrt{64}\)
Step 6: Solve for x
\(x=12\pm \sqrt{64}\\=12\pm8\\x=12+8$ or x=12-8\\x=20 or x=4.\)
Therefore, the solution set of the equation is (4,20).
Find p and q if 3(p 5// 4 8)-(-2 7//0 2)=2(-5 4//6 q). Please help me solve the matrix
Answers
Answer:2[6-2-34]
Step-by-step explanation: cuz i jus know everything
How can you find the product of a fraction and a whole number without using a model? I WILL GIVE BRAINLEST!
Answers
Answer:
Process:
Convert all whole numbers to fractionsMultiply acrossSimplify (is possible)Step-by-step explanation:
Ex. Question: \(\frac{1}{2} \cdot 5\)
Process:
Convert all whole numbers to fractionsMultiply acrossSimplify (is possible)Work:
- \(\frac12 \cdot5\)
- \(\frac12 \cdot\frac51\)
- \(\frac{1\cdot5}{2\cdot1}\)
- \(\frac52\)
-Chetan K
Mark has four and one-fifth yards of rope and gives one-third of it to his friend.
How many yards of rope did Mark give his friend?
one and six-fifteenths
two and five-eighths
four and one-fifteenth
four and two-eighths
Answers
The yards of rope that Mark give his friend is A. one and six-fifteenths
What is fraction?A fraction is simply a piece of a whole. The number is represented mathematically as a quotient where the numerator and denominator are split. In a simple fraction, the numerator as well as the denominator are both integers.
In this case, Mark has four and one-fifth yards of rope and gives one-third of it to his friend.
The fraction given out will be:
= Fraction given × Total yards
= 1/3 × 4 1/5
= 1/3 × 21/5
= 7/5
= 1 2/5
The correct option is A.
Learn more about fractions on:
brainly.com/question/17220365
#SPJ1
f(x)=x^4-8x^2+10x+22 divied by x+3
Answers

What is the first step to solving this algebraic equation?
-5c + 6 = -4
Answers
Answer: I am pretty sure it is add -4 to both sides
Step-by-step explanation:
Answer:
Step-by-step explanation:
-5c+6=4
-6 -6 to get -5c by itself
-5c=4-6
-5c=-2
Divide -5c to -2
c=0.4
11. three cards are dealt from a well-shuffled deck. (a) find the chance that all of the cards are diamonds. (b) find the chance that none of the cards are diamonds. (c) find the chance that the cards are not all diamonds
Answers
The probability that the chosen card is a diamond is 3/13. According to the definition of probability, which is "the degree to which something is probable; the likelihood of something happening or being the case," there is a 1/13 chance that none of the cards will be diamonds.
What is probability?Probability is simply the likelihood that something will happen. The likelihood or likelihood of various outcomes can be discussed when we don't know how an event will turn out. The study of events that fit into a probability distribution is known as statistics. The full range of potential outcomes is known as the sample space, or individual space, of a random experiment. The likelihood of any event occurring ranges from 0 to 1. Probability is the study of random events in mathematics, and it can be classified into four main categories: axiomatic, classical, empirical, and subjective. You could say that probability is the likelihood that a particular event will occur because probability and possibility are synonyms.
Here,
a. Given that there are 13 diamond cards, the probability that all the cards are diamonds is 3/13.
b. the likelihood that none of the 52 cards, with 13 diamond cards, are diamonds.
unused card = 52-13 = 39 = 3/39
There is a 3/13 chance that the selected card will be a diamond. There is a 1/13 chance that no cards will be diamonds, according to the definition of probability, which is "the degree to which something is probable; the likelihood of something happening or being the case."
To know more about probability,
https://brainly.com/question/11234923?referrer=searchResults
#SPJ4
what is the probability of serving a student that is in math Spanish and chemistry round to the three decimal places

Answers
In this problem the total number of students are: 260 students
so the probablility is:
\(p=\frac{15}{260}=0.058\)both methods requires two initial guesses x1 and x2, and it is necessary that f(x1)*f(x2) < 0
Answers
The requirement f(x1) * f(x2) < 0 for choosing initial guesses x1 and x2 with opposite signs ensures the validity and convergence of certain numerical root-finding methods such as the bisection method or the regula falsi method.
The statement you provided is true for certain numerical methods used to find roots of equations, such as the bisection method or the regula falsi method. These methods are iterative and require an initial interval or range where the root is expected to be found. To ensure convergence and a valid solution, it is necessary to choose initial guesses x1 and x2 such that the function f(x) evaluated at those points have opposite signs, i.e., f(x1) * f(x2) < 0.
The rationale behind this requirement is based on the Intermediate Value Theorem, which states that if a continuous function f(x) changes sign over an interval [x1, x2], then there exists at least one root within that interval. By ensuring that f(x1) and f(x2) have opposite signs, we guarantee the existence of a root within the interval [x1, x2].
The bisection method works by repeatedly bisecting the interval and selecting a new subinterval that contains the root. At each iteration, the method narrows down the interval by halving it, based on the sign change observed in the function evaluations.
Similarly, the regula falsi (or false position) method also operates by iteratively refining the interval based on the linear interpolation between the function values at the endpoints. The method adjusts the interval based on the sign change of the function, converging to the root.
Both methods rely on the property of opposite signs to guarantee convergence and avoid getting stuck in a non-converging or incorrect solution. If the initial guesses do not satisfy the condition f(x1) * f(x2) < 0, it is possible that the method fails to converge or converges to a different root or solution.
Learn more about bisection method at: brainly.com/question/30320227
#SPJ11
* You have a dog that can run 5 km/hr. How fast can she run in mi/hr? (i.e. convert the rate to miles per hour) (1.6 km=1mi) DO NOT JUST TYPE THIS INTO A CONVERTER ONLINE. YOU WILL NOT GET THE ANSWER RIGHT. Express your answer as decimal, rounded to the nearest thousandth (three decimal places) in mi/hr - no spaces EXAMPLE: 78.345mi/hr
Answers
The dog's running speed of 5 km/hr can be converted to approximately 3.125 mi/hr by multiplying it by the conversion factor of 1 mi/1.6 km. Rounding to the nearest thousandth, the dog can run at about 3.125 mi/hr.
To convert the dog's running speed from kilometers per hour (km/hr) to miles per hour (mi/hr), we need to use the conversion factor of 1.6 km = 1 mi.First, we can convert the dog's speed from km/hr to mi/hr by multiplying it by the conversion factor: 5 km/hr * (1 mi/1.6 km) = 3.125 mi/hr.
However, we need to round the answer to the nearest thousandth (three decimal places). Since the digit after the thousandth place is 5, we round up the thousandth place to obtain the final answer.
Therefore, the dog can run at approximately 3.125 mi/hr.
To learn more about factor click here
brainly.com/question/26923098
#SPJ11
ill give brainliest!! please help with algebra!! Find the factors of these trinomials
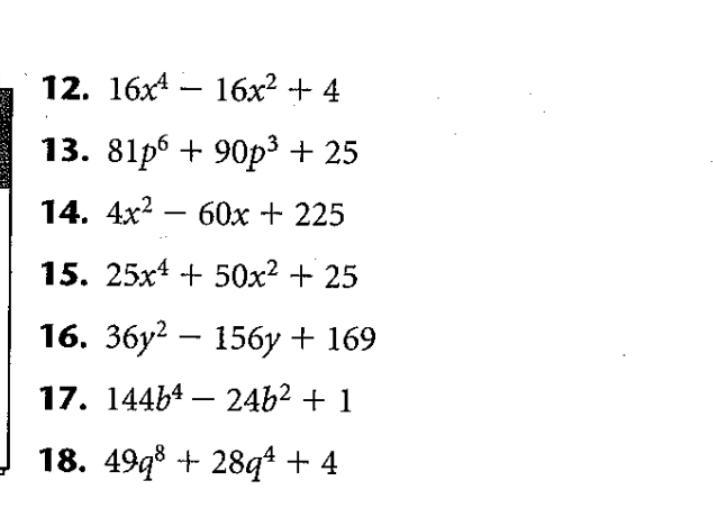
Answers
Step-by-step explanation:
Use formula:
(a + b)^2 = a^2 + 2ab + b^2Q12
16x^4 - 16x^2 + 4 =(4x^2)^2 - 2*4x^2*2 + 2^2 =(4x^2 - 2)^2 = 4(2x^2 - 1)^2Q13
81p^6 + 90p^3 + 25 = (9p^3)^2 + 2*9p^3*5 + 5^2 = (9p^3 + 5)^2Q14
4x^2 - 60x + 225 =(2x)^2 - 2*2x*15 + 15^2 =(2x^2 - 15)^2Q15
25x^4 + 50x^2 + 25 = (5x^2)^2 + 2*5x^2*5 + 5^2 = (5x^2 + 5)^2 =25(x^2 + 1)^2Q16
36y^2 - 156y + 169 = (6y)^2 - 2*6y*13 + 13^2 = (6y - 13)^2Q17
144b^4 - 24b^2 + 1 =(12b^2)^2 - 2*12b^2*1 + 1^2 = (12b^2 - 1)^2Q18
49q^8 + 28q^4 + 4 = (7q^4)^2 + 2*7q^4*2 + 2^2 = (7q^4 + 2)^2Find nd the 10th term of the series attached.

Answers
Answer:
\(C) \ -2(2/3)^8\)--------------------------
The nth term is visible in the sum:
\(t_n=3(-2/3)^{n-1}\)Apply n = 10 to find the 10th term:
\(t_{10}=3(-2/3)^{10-1}\)\(t_{10}=3(-2/3)^9\)\(t_{10}=3(-2/3)^{8+1}\)\(t_{10}=3(-2/3)^8*(-2/3)\)\(t_{10}=-2(-2/3)^8\)\(t_{10}=-2(2/3)^8,\ since\ the\ power\ is\ even\ number\)The matching choice is C.
37​% of adults say cashews are their favorite kind of nut. You randomly select 12 adults and ask each to name his or her favorite nut. Find the probability that the number who say cashews are their favorite nut is​ (a) exactly​ three, (b) at least​ four, and​ (c) at most two. If​ convenient, use technology to find the probabilities.
Answers
Solution :
It is given that about 37% of the adults say cashew nuts are their favorite nut. And a sample of 12 adults are taken to name their favorite nut.
We note that probability of \($x$\) successes out of \($n$\) trial is given by :
\($P(n,x)=^nC_x p^x (1-p)^{(n-x)}$\)
Here, number of trails, n = 12
probability of success, p = 0.37
number of successes, x = 3
a). Therefore the probability of the adults to say cashew nut as their favorite of exactly three is given by :
\($P(3)=^{12}C_3 (0.37)^3 (1-0.37)^{(12-3)}$\)
= 0.174217909
b). We know that :
P(at least x) = 1 - P(at most x - 1)
So we use the cumulative binomial distribution table.
i.e. \($P(x \geq 4) = 1 -P(x \leq3)$\)
\($= -1[P(x=0)+P(x=1)+P(x=2)+P(x=3)]$\)
\($= 1-[^{12}C_0 (0.37)^0 (0.63)^{12}+^{12}C_1 (0.37)^1(0.63)^{11}+^{12}C_2 (0.37)^2(0.63)^{10}+^{12}C_3(0.37)^3(0.63)^9]$\)= 0.70533
Therefore, P(at least 4) = 0.70533
c). \($P(x \leq 2) = P(x=0) + P(x=1)+P(x=2)$\)
\($=^{12}C_0(0.37)^0(0.63)^{12}+^{12}C_1(0.37)^1(0.63)^{11}+^{12}C_2(0.37)^2(0.63)^{10}$\)
= 0.12045205
Therefore, P(at most 2) = 0.12045205
How are the properties of exponents used when dividing a polynomial by a monomial?
Answers
Answer:
a couple different obes
Step-by-step explanation:
there are five
Study the road plan shown in the figure. A service station will be built on the highway, and a road will connect it with Cray. How long will the new road be? A.54.2 mi B. 312 mi C. 46.2 mi D.400 mi
Answers
The length of the road that connects the service station with Cray is 46.2 mi.
How long will the new road be?
We need to apply the principle of similar triangles to obtain the length of the road that will connect the service station with Cray as shown in the image attached.
Hence;
The distance between Alba and Blare = 130 mi
The distance between Alba and Cray = 120 mi
Road connecting the service station with Cray =x
And;
120/130 = x/50
x = 46.2 mi
Learn more about similar triangles:https://brainly.com/question/12100952
#SPJ1

The graph of f(x) and g(x) are shown below. How many solutions does the system of equations have?
Click pic to see whole problem

Answers
Answer:
Step-by-step explanation:
Solving systems of equations gives the points of intersection when the equations are graphed.
The answer is 3.
Suppose a survey of women in Thunder Bay with full-time jobs indicated that they spent on average 11 hours doing housework per week with a standard deviation of 1.5 hours. If the number of hours doing housework is normally distributed, what is the probability of randomly selecting a woman from this population who will have spent more than 15 hours doing housework over a one-week period? Multiple Choice
a. 0.9962
b. 0.4962
c. 0.5038
d. 0.0038
Answers
The probability of randomly selecting a woman from the population in Thunder Bay who spent more than 15 hours doing housework per week will be calculated. The answer will be chosen from the provided multiple-choice options.
To calculate the probability, we need to find the area under the normal distribution curve that corresponds to the event of spending more than 15 hours doing housework. We can use the properties of the normal distribution to determine this probability.
Given that the average hours of housework is 11 hours per week with a standard deviation of 1.5 hours, we can standardize the value of 15 hours using the z-score formula: z = (x - μ) / σ, where x is the value, μ is the mean, and σ is the standard deviation.
Using the z-score, we can then find the corresponding area under the standard normal distribution curve using a z-table or a statistical calculator. The area to the right of the z-score represents the probability of spending more than 15 hours on housework.
Comparing the calculated probability to the provided multiple-choice options, we can determine the correct answer.
In conclusion, by calculating the z-score and finding the corresponding area under the normal distribution curve, we can determine the probability of randomly selecting a woman from the population who spent more than 15 hours on housework.
Learn more about normal distribution curve here:
https://brainly.com/question/30783928
#SPJ11
Fid the missing terms of each arithmetic sequence. (Hint: The arithmetic mean of the first and fifth terms is the third term.)... a+1,a₃, a₄, a₅, a+17, \ldots
Answers
Let's use the given hint to find the missing terms of the arithmetic sequence.
The arithmetic mean of the first and fifth terms is the third term. In other words, the average of the first term (a + 1) and the fifth term (a + 17) is equal to the third term (a₃).
We can set up an equation based on this information:
(a + 1 + a + 17) / 2 = a₃
Simplifying the equation:
(2a + 18) / 2 = a₃
(a + 9) = a₃
So, we have found that the third term of the sequence is (a + 9).
To find the missing terms, we can use the common difference between consecutive terms. The common difference is the difference between any two consecutive terms in the arithmetic sequence.
In this case, we can find the common difference by subtracting the second term (a₃) from the first term (a + 1):
Common difference = (a + 1) - (a + 9) = -8
Now, we can determine the missing terms:
a₄ = a₃ + Common difference = (a + 9) - 8 = (a + 1)
a₅ = a₄ + Common difference = (a + 1) - 8 = (a - 7)
Therefore, the missing terms of the arithmetic sequence are (a + 1) and (a - 7).
To know more about arithmetic sequence visit:
https://brainly.com/question/12952623
#SPJ11
A store has a 4% restocking fee. if you join the stores membership program, all items over $199 will have a flat rate restocking fee of $7. how much money will a member save if they return an item costing $270?
Answers
A store has a 4% restocking fee. if you join the stores membership program, all items over $199 will have a flat rate restocking fee of $7. A member will save $3.80 if they return an item costing $270.
If a non-member returns an item costing $270, they will be charged a restocking fee of 4% which would amount to $10.80. However, if a member returns the same item, they will only be charged a flat rate restocking fee of $7. Therefore, the member will save $3.80 on the restocking fee for the returned item.
Joining the store's membership program can be beneficial for customers who frequently return items that cost over $199. They can save money on restocking fees by paying a lower flat rate fee rather than a percentage of the item's cost. However, it's important to consider the cost of the membership program and whether the benefits outweigh the cost.
In this particular scenario, the member saved $3.80 on the restocking fee for the $270 item. If the membership program costs less than this amount and the customer anticipates returning items frequently, then joining the program may be a good choice. However, if the customer does not anticipate returning items frequently or the cost of the membership program is more than the potential savings, it may not be worth it.
To learn more about restocking fee, refer:-
https://brainly.com/question/23524616
#SPJ11
what is the probability that the light bulb she purchases from this manufacturer will last less than or equal to 14,500 hours? use the z score formula and the portion of the standard normal table below to help answer the question.
Answers
To answer this question, we need to use the z-score formula:
z = (x - μ) / σ
where x is the value we are interested in (in this case, 14,500 hours), μ is the mean (average) lifespan of the bulbs produced by the manufacturer, and σ is the standard deviation (how much the lifespans vary around the mean).
Let's assume that the mean lifespan of the bulbs is 15,000 hours, and the standard deviation is 500 hours.
Plugging these values into the formula, we get:
z = (14,500 - 15,000) / 500 = -1
Now, we need to find the probability that a bulb will last less than or equal to 14,500 hours, given that the mean lifespan is 15,000 hours and the standard deviation is 500 hours.
We can use a standard normal table to find this probability. The portion of the table we need shows the area under the curve to the left of a given z-score.
Looking at the table, we can see that the area to the left of z = -1 is 0.1587. This means that there is a 15.87% chance that a randomly selected bulb will last less than or equal to 14,500 hours.
So the probability that the light bulb she purchases from this manufacturer will last less than or equal to 14,500 hours is approximately 0.1587, or 15.87%.
Learn more about standard deviation here: brainly.com/question/23907081
#SPJ11
Add 1039 g and 36.7 kg and express your answer in milligrams
(mg) to the correct number of significant figures.
Answers
The sum of 1039 g and 36.7 kg expressed in milligrams (mg) to the correct number of significant figures is 37,739,000 mg.
To perform the addition, we need to convert 36.7 kg to grams before adding it to 1039 g. There are 1000 grams in 1 kilogram, so we multiply 36.7 kg by 1000:
36.7 kg * 1000 g/kg = 36,700 g
Now, we can add 1039 g and 36,700 g:
1039 g + 36,700 g = 37,739 g
To convert grams to milligrams, we multiply by 1000 because there are 1000 milligrams in 1 gram:
37,739 g * 1000 mg/g = 37,739,000 mg
The final result, expressed in milligrams with the correct number of significant figures, is 37,739,000 mg.
The sum of 1039 g and 36.7 kg, expressed in milligrams (mg) with the correct number of significant figures, is 37,739,000 mg. Remember to consider unit conversions and maintain the appropriate number of significant figures throughout the calculation.
To know more about significant figures visit:
https://brainly.com/question/24491627
#SPJ11
help pls!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!!

Answers
Reason: Replace m with 160 to go from 8m+150 to 8(160)+150
When using PEMDAS or a calculator, that expression simplifies to 1430.
1280 + 150
1430
Find the equation of a line perpendicular to y=3x+3 that passes through the point (3,2)
Answers
keeping in mind that perpendicular lines have negative reciprocal slopes, let's check for the slope of the equation above
\(y = \stackrel{\stackrel{m}{\downarrow }}{3} x + 3\qquad \impliedby \qquad \begin{array}{|c|ll} \cline{1-1} slope-intercept~form\\ \cline{1-1} \\ y=\underset{y-intercept}{\stackrel{slope\qquad }{\stackrel{\downarrow }{m}x+\underset{\uparrow }{b}}} \\\\ \cline{1-1} \end{array} \\\\[-0.35em] ~\dotfill\)
\(\stackrel{~\hspace{5em}\textit{perpendicular lines have \underline{negative reciprocal} slopes}~\hspace{5em}} {\stackrel{slope}{3\implies\cfrac{3}{1}} ~\hfill \stackrel{reciprocal}{\cfrac{1}{3}} ~\hfill \stackrel{negative~reciprocal}{-\cfrac{1}{3}}}\)
so we're really looking for the equation of a line whose slope is -1/3 and that is passes through (3 , 2)
\((\stackrel{x_1}{3}~,~\stackrel{y_1}{2})\hspace{10em} \stackrel{slope}{m} ~=~ - \cfrac{1}{3} \\\\\\ \begin{array}{|c|ll} \cline{1-1} \textit{point-slope form}\\ \cline{1-1} \\ y-y_1=m(x-x_1) \\\\ \cline{1-1} \end{array}\implies y-\stackrel{y_1}{2}=\stackrel{m}{- \cfrac{1}{3}}(x-\stackrel{x_1}{3}) \\\\\\ y-2=- \cfrac{1}{3}x+1\implies {\Large \begin{array}{llll} y=- \cfrac{1}{3}x+3 \end{array}}\)
Consider the two vectors A=4.00i^+5.00j^−3.00k^ and B=2.00i^−3.00j^−5.00k^ (a) Calculate A−B using the components of the two vectors. (b) Calculate the magnitude of the vector difference A−B. (c) Is it possible to calculate a direction for this vector? Explain. (d) Calculate the vector product between the two vectors, A×B.
Answers
(a) The components of the two vectors are A - B = 2.00i + 8.00j + 2.00k.
(b) The magnitude of the vector difference A - B is approximately 11.49.
(c) The vector product A × B is -16.00i + 14.00j - 22.00k.
(a) To calculate A - B, we subtract the corresponding components of the two vectors:
A - B = (4.00i + 5.00j - 3.00k) - (2.00i - 3.00j - 5.00k)
Simplifying:
A - B = 4.00i + 5.00j - 3.00k - 2.00i + 3.00j + 5.00k
Combining like terms:
A - B = (4.00i - 2.00i) + (5.00j + 3.00j) + (-3.00k + 5.00k)
A - B = 2.00i + 8.00j + 2.00k
Therefore, A - B = 2.00i + 8.00j + 2.00k.
(b) To calculate the magnitude of the vector difference A - B, we use the formula:
|A - B| = sqrt((A - B) · (A - B))
where (A - B) · (A - B) represents the dot product of A - B with itself.
|A - B| = sqrt((2.00i + 8.00j + 2.00k) · (2.00i + 8.00j + 2.00k))
Expanding and calculating the dot product:
|A - B| = sqrt((2.00i · 2.00i) + (2.00i · 8.00j) + (2.00i · 2.00k) + (8.00j · 2.00i) + (8.00j · 8.00j) + (8.00j · 2.00k) + (2.00k · 2.00i) + (2.00k · 8.00j) + (2.00k · 2.00k))
|A - B| = sqrt(4.00 + 16.00 + 4.00 + 16.00 + 64.00 + 4.00 + 4.00 + 16.00 + 4.00)
|A - B| = sqrt(132.00)
|A - B| ≈ 11.49
Therefore, the magnitude of the vector difference A - B is approximately 11.49.
(c) To calculate the vector product (cross product) between vectors A and B, we use the formula:
A × B = (A_yB_z - A_zB_y)i + (A_zB_x - A_xB_z)j + (A_xB_y - A_yB_x)k
Plugging in the values:
A × B = ((5.00)(-5.00) - (-3.00)(-3.00))i + ((-3.00)(2.00) - (4.00)(-5.00))j + ((4.00)(-3.00) - (5.00)(2.00))k
Simplifying:
A × B = (-25.00 + 9.00)i + (-6.00 + 20.00)j + (-12.00 - 10.00)k
A × B = -16.00i + 14.
00j - 22.00k
Therefore, the vector product A × B is -16.00i + 14.00j - 22.00k.
Learn more about like terms here:
https://brainly.com/question/29169167
#SPJ11
Consider the two vectors A=4.00i^+5.00j^−3.00k^ and B=2.00i^−3.00j^−5.00k^ (a) Calculate A−B using the components of the two vectors. (b) Calculate the magnitude of the vector difference A−B. (c) Calculate the vector product between the two vectors, A×B.
These figures are congruent. What is NO?

Answers
Answer:
50mm
Hope that helps!
Step-by-step explanation:
Factor ab + bc + b 2 + ac.
Answers
The factor of the terms ab + bc + b² + ac is ( a + b ) ( b + c ).
Given ab + bc + b² + ac
A factorization is a mathematical object of multiplying several factors usually smaller or simpler objects of the same kind.
To factor the terms we need to write like terms on one side as below
= ab + ac + bc + b²
Separating the common terms as
= a ( b + c ) + b (c + b )
= a ( b + c ) + b ( b + c )
= ( a + b ) ( b + c )
Therefore, the factor of the given terms ab + bc + b² + ac is ( a + b ) ( b + c ).
To know more about factorization refer below
https://brainly.com/question/20293447
#SPJ9
Use logarithmic differentiation to find the derivative of the function. y=(ln(x+4)) x
Answers
the derivative of the function y = (ln(x + 4))x using logarithmic differentiation is given by y' = (ln(x + 4))x * [ln(ln(x + 4)) + (1/ln(x + 4)) * (1/(x + 4))].
To find the derivative of the function y = (ln(x + 4))x using logarithmic differentiation, we can follow these steps:
Step 1: Take the natural logarithm of both sides of the equation:
ln(y) = ln((ln(x + 4))x)
Step 2: Use the logarithmic property ln(a^b) = b ln(a) to simplify the right-hand side of the equation:
ln(y) = x ln(ln(x + 4))
Step 3: Differentiate both sides of the equation implicitly with respect to x:
(1/y) * y' = ln(ln(x + 4)) + x * (1/ln(x + 4)) * (1/(x + 4))
Step 4: Simplify the expression on the right-hand side:
y' = y * [ln(ln(x + 4)) + (1/ln(x + 4)) * (1/(x + 4))]
Step 5: Substitute the original expression of y = (ln(x + 4))x back into the equation:
y' = (ln(x + 4))x * [ln(ln(x + 4)) + (1/ln(x + 4)) * (1/(x + 4))]
Therefore, the derivative of the function y = (ln(x + 4))x using logarithmic differentiation is given by y' = (ln(x + 4))x * [ln(ln(x + 4)) + (1/ln(x + 4)) * (1/(x + 4))].
To know more about Equation related question visit:
https://brainly.com/question/29538993
#SPJ11