Hank paid $16,780 for a used car 5 years ago. Because he did not properly maintain the car, he sold it for $4,280. What was his average annual depreciation?
Answers
The average annual depreciation of the car over the 5 years span is $2500/year.
It is given that Hank paid $16,780 for a used car 5 years ago. Because he did not properly maintain the car, he sold it for $4,280.
We can write the average annual depreciation as -
Annual depreciation = (4280 - 16780)/5
Annual depreciation = (4280 - 16780)/5
Annual depreciation = - 2500
Therefore, the average annual depreciation of the car over the 5 years span is $2500/year.
To solve more questions on depreciation, visit the link-
https://brainly.com/question/15381486
#SPJ1
Related Questions
b. in general, when dealing with inferences for two population proportions, which two of the following are equivalent: confidence interval method; p-value method; critical value method?
Answers
The confidence interval and critical value methods are equivalent in providing an interval estimate, the p-value method is used for hypothesis testing and evaluates the strength of evidence against the null hypothesis.
What is the confidence interval?
A confidence interval is a range of values that is likely to contain the true value of an unknown population parameter, such as the population mean or population proportion. It is based on a sample from the population and the level of confidence chosen by the researcher.
In general, when dealing with inferences for two population proportions, the confidence interval method and the critical value method are equivalent. These two methods provide a range of plausible values (confidence interval) for the difference between two population proportions and involve the calculation of critical values to determine the margin of error.
On the other hand, the p-value method is not equivalent to the confidence interval and critical value methods. The p-value method involves calculating the probability of observing a test statistic as extreme as, or more extreme than, the one obtained from the sample data, assuming the null hypothesis is true. It is used in hypothesis testing to determine the statistical significance of the difference between two population proportions.
To summarize:
- Confidence interval method: Provides a range of plausible values for the difference between two population proportions.
- Critical value method: Uses critical values to determine the margin of error in estimating the difference between two population proportions.
- P-value method: Determines the statistical significance of the observed difference between two population proportions based on the calculated p-value.
Hence, the confidence interval and critical value methods are equivalent in providing an interval estimate, the p-value method is used for hypothesis testing and evaluates the strength of evidence against the null hypothesis.
Learn more about the confidence interval here:
https://brainly.com/question/20309162
#SPJ4
A palindrome is an integer that reads the same forwards and backwards. How many positive 3-digit palindromes are multiples of $3$
Answers
There are total 30 positive 3-digit palindromes are multiples of 3.
The numbers are as follows,
111, 141, 171, 222, 252, 282, 303, 333, 363, 393, 414, 444, 474, 525, 555, 585, 606, 636, 666, 696, 717, 747, 777, 828, 858, 888, 909, 939, 969, 999.
Palindrome numbers
A palindromic number is a number that remains the same when its digits are reversed. In other words, it has reflectional symmetry across a vertical axis.
A palindrome number is a number that is same after reverse.
For example - 121, 34543, 343, 131, 48984 are the palindrome numbers.
Learn more about palindrome number here :-
https://brainly.com/question/19052372
#SPJ4
Muffins are sold by the dozen at a bakery. The table shows the cats for oferent mens of muffins sold at the bakery, Cost of Muffins Domen of Cost (dollars) Muffins 2 17 4 6 51 Explain your thin with equations 34 What is the cost in dollars for 24 dozen muffins? A $408 B $204 Wha С $85 Not here
Answers
hello
from the table given, we can establish a relationship bewteen the number of dozens to the cost in dollars
if 2 dozens costs $17
8 dozens costs x
cross multiply and solve for x
\(\begin{gathered} 2\text{ =17} \\ 8=x \\ 2x=8\times17 \\ 2x=136 \\ x=68 \end{gathered}\)what i did above was test if the relationship is correct
now let's find for 24 dozens
if 2 dozens = $17
24 dozen = ?
\(\begin{gathered} 2dz=17 \\ 24dz=x \\ 2x=17\times24 \\ 2x=408 \\ x=\frac{408}{2} \\ x=204 \end{gathered}\)24 dozens of muffins would cost $204
Let n be a positive integer. Show that in any set of n consecutive integers there is exactly one divisible by n. (Using The Pigeon-Hole Principle)
Answers
Using the Pigeonhole Principle, we can prove that in any set of n consecutive integers, there is exactly one integer that is divisible by n.
The Pigeonhole Principle states that if you have more pigeons than pigeonholes, at least one pigeonhole must contain more than one pigeon. I
n this case, the pigeons represent the consecutive integers and the pigeonholes represent the possible remainders when dividing the integers by n.
Consider a set of n consecutive integers, starting from some integer k. The n integers can be written as k, k+1, k+2, ..., k+n-1. To show that there is exactly one integer divisible by n, we will assign each integer to a pigeonhole based on its remainder when divided by n.
Since there are n possible remainders when dividing an integer by n (0, 1, 2, ..., n-1), and we have n consecutive integers, by the Pigeonhole Principle, there must be at least two integers that have the same remainder when divided by n.
Let's say two integers, k+i and k+j, have the same remainder r when divided by n, where i < j.
Then we have (k+i) ≡ r (mod n) and (k+j) ≡ r (mod n).
Subtracting these two congruences, we get (k+j) - (k+i) ≡ 0 (mod n), which simplifies to j - i ≡ 0 (mod n). This implies that n divides (j - i).
Since j > i, we have j - i > 0, and since n divides (j - i), we conclude that n must divide (j - i), which means that one of the integers between k+i and k+j is divisible by n.
Therefore, in any set of n consecutive integers, there is exactly one integer that is divisible by n.
To learn more about Pigeonhole Principle visit:
brainly.com/question/31253051
#SPJ11
Which BEST describes the difference between the medians of Plot A and Plot B as a multiple of the interquartile range of Plot A?
A) 3/4
B) 1
C) 3/2
D) 2

Answers
Answer:
B) 1
Plot A range is 4 and plot b is 5
Answer:
1
Step-by-step explanation:
96 newspapers in 12 piles = 8 newspapers in piles
Answers
Answer:
8*12=96
Step-by-step explanation:
Answer:
8 newspapers in one pile
Step-by-step explanation:
\(\frac{96}{12} = \frac{8}{x} \\\\96x=8*12\\\\96x=96\\x=1\)
Suppose 1 cast member will be picked at random from the 20 cast members who sold tickets to receive a prize. What is the probability of picking a cast member who sold more than 30 tickets
Answers
The probability of picking a cast member who sold more than 30 tickets is 2/5
Given that one cast member will be picked at random from the 20 cast members who sold tickets to receive a prize. We have to determine the probability of picking a cast member who sold more than 30 tickets.
To find the probability of picking a cast member who sold more than 30 tickets, we need to count the number of cast members who sold more than 30 tickets. Let A be the event of picking a cast member who sold more than 30 tickets. The number of cast members who sold more than 30 tickets is 8. Therefore, P(A) = 8/20 = 2/5.
The probability of picking a cast member who sold more than 30 tickets is 2/5.Answer: 2/5.
Know more about probability here:
https://brainly.com/question/31828911
#SPJ11
Pet training classes cost $191.10 for 13 sessions. What is the fee for one session?
Answers
Answer:
$14.70
Step-by-step explanation:
191.10/13= 14.70
Answer: 14.70
Step-by-step explanation: you're supposed to divide 191.10 by 13. you get 14.7 so that's the answer.
Solve equation y/9=-8
Answers
Answer:
y = - 72
Step-by-step explanation:
Given
\(\frac{y}{9}\) = - 8 ( multiply both sides by 9 to clear the fraction )
y = - 72
Answer:
y/9=-8
y=-8*[multiply]9
y=-72
answer is negative 72
write the first six cube if natural number
Answers
Answer:
1³ = 1
2³ = 8
3³ = 27
4³ = 64
5³ = 125
6³ = 216
Step-by-step explanation:
Answer:
The cube of first six natural numbers are
1, 8, 27, 65, 125, 216
Step-by-step explanation:
Natural Numbers are known as 1, 2, 3, 4, ..., ∞
Now,
For Cube of first six natural numbers
1³ = 1
2³ = 8
3³ = 27
4³ = 64
5³ = 125
6³ = 216
Thus, The cube of first six natural numbers are
1, 8, 27, 65, 125, 216
-TheUnknownScientist
Find the values of a and b that make f continuous everywhere.
f(x) =
(x2 − 4)/(x − 2) if x < 2
ax2 − bx + 3 if 2 ≤ x < 3
2x − a + b if x ≥ 3
Answers
The values of a and b that make f(x) continuous everywhere are a = 2x and b = 2a - 3/2 = 2(2x) - 3/2 = 4x - 3/2.
What is the limit?The limit is a concept in mathematics that describes the behavior of a function near a particular value, called the limit point. The limit of a function gives the value that the function approaches as the input (variable) approaches the limit point.
For f(x) to be continuous everywhere, the function must have the same value and the same limit as x approaches 2 from the left and the right. In other words, f(2-) = f(2+) and the limit of f(x) as x approaches 2 from the left and the right must be equal.
Let's start by finding f(2-), which is the value of f(x) as x approaches 2 from the left. In this case, f(x) = (x2 - 4)/(x - 2) for x < 2, so as x approaches 2 from the left, f(x) approaches (2^2 - 4)/(2 - 2) = 0.
Next, let's find f(2+), which is the value of f(x) as x approaches 2 from the right. In this case, f(x) = ax^2 - bx + 3 for 2 <= x < 3, so as x approaches 2 from the right, f(x) approaches a(2^2) - b(2) + 3 = 4a - 2b + 3.
Since f(x) must be continuous at x = 2, we need to have f(2-) = f(2+), so we can set f(2-) = f(2+) and solve for a and b:
0 = 4a - 2b + 3
2b = 4a - 3
b = 2a - 3/2
Now that we have an expression for b in terms of a, we can substitute b = 2a - 3/2 into the expression for f(x) for x >= 3 to find the value of a that makes f(x) continuous everywhere:
f(x) = 2x - a + b for x >= 3
f(x) = 2x - a + (2a - 3/2) for x >= 3
f(x) = 2x + 3/2 - a for x >= 3
Since f(x) must be continuous at x = 2, we need to have f(2+) = f(2+), so we can set f(2+) = f(2+) and solve for a:
4a - 2b + 3 = 2x + 3/2 - a for x >= 3
4a - 2(2a - 3/2) + 3 = 2x + 3/2 - a
4a - 4a + 3 + 3/2 = 2x + 3/2 - a
3/2 = 2x + 3/2 - a
a = 2x
So, the values of a and b that make f(x) continuous everywhere are a = 2x and b = 2a - 3/2 = 2(2x) - 3/2 = 4x - 3/2.
To learn more about Limit, Visit
brainly.com/question/282767
#SPJ4
06. Find the value of (a + b + c)², ifa = 5,6 = 4 and c = 3.
Answers
Answer:
\( {(a + b + c)}^{2} = {(5 + 4 + 3)}^{2} \\ = {12}^{2} \\ = \boxed{144}\)
144 is the right answer.can someone please help with this

Answers
All correct proportions include the following:
A. \(\frac{AC}{CE} =\frac{BD}{DF}\)
D. \(\frac{CE}{DF} =\frac{AE}{BF}\)
What are the properties of similar geometric figures?In Mathematics and Geometry, two geometric figures are said to be similar when the ratio of their corresponding side lengths are equal and their corresponding angles are congruent.
Hence, the lengths of the pairs of corresponding sides or corresponding side lengths are proportional to one another when two (2) geometric figures are similar.
Since line segment AB is parallel to line segment CD and parallel to line segment EF, we can logically deduce that they are congruent because they can undergo rigid motions. Therefore, we have the following proportional side lengths;
\(\frac{AC}{CE} =\frac{BD}{DF}\)
\(\frac{CE}{DF} =\frac{AE}{BF}\)
Read more on scale factor here: brainly.com/question/29967135
#SPJ1
compute the surface area of revolution of y=4x 3y=4x 3 about the x-axis over the interval [4,5][4,5].
Answers
The surface area of revolution of y = 4\(x^3\) about the x-axis over the interval [4, 5] is approximately 806.259 square units.
To find the surface area of revolution of the curve y = 4\(x^3\) about the x-axis over the interval [4, 5], we can use the formula:
S = 2π ∫ [a,b] y √(1 + \((dy/dx)^2\)) dx
where a = 4, b = 5, and dy/dx = 12\(x^2\).
Substituting these values, we get:
S = 2π ∫[4,5] 4x \(\sqrt{(1 + (12x^2)^2)}\) dx
Simplifying the expression inside the square root:
1 + \((12x^2)^2\) = 1 + 144\(x^4\)
= 144\(x^4\) + 1
The integral becomes:
S = 2π ∫[4,5] 4x √(144\(x^4\) + 1) dx
To evaluate this integral, we can make the substitution u = 144\(x^4\) + 1. Then, du/dx = 576\(x^3\), and dx = du/576\(x^3\).
Substituting these values, we get:
S = 2π ∫[577, 11521] 4x √u du / (576x^3)
Simplifying:
S = π/36 ∫[577, 11521] √u du
S = π/36 x (2/3) x \((11521^{(3/2)} - 577^{(3/2)})\)
S = π/54 x \((11521^{(3/2)} - 577^{(3/2)})\)
Using a calculator, we can approximate this value to be:
S ≈ 806.259
For similar question on surface area
https://brainly.com/question/26403859
#SPJ11
Gustavo is in a contest where he will win one of four possible prizes. he creates a four-part spinner to represent the four prizes that he has an equal chance of winning: a video game system, a bicycle, a watch, and a gift card. he spins the spinner several times to demonstrate the likelihood of winning a certain prize. what is true about gustavo’s method of data collection and his data on the possible prizes?
Answers
Answer:
Gustavo used a simulation where the data is quantitative’
solve the given initial-value problem. y'' 49y = 0, y(0) = 4, y'(0) = −5
Answers
Particular solution with the determined constants is:
y(t) = 4*cos(7t) - (5/7)*sin(7t)
To solve the given initial-value problem y'' + 49y = 0 with initial conditions y(0) = 4 and y'(0) = -5, follow these steps:
Step 1: Identify the type of problem.
This is a second-order linear homogeneous differential equation with constant coefficients.
Step 2: Write down the characteristic equation.
The characteristic equation for this problem is \(r^2\) + 49 = 0.
Step 3: Solve the characteristic equation.
\(r^2\) = -49
r = ±√(-49) = ±7i
Since the roots are complex conjugates, the general solution of the differential equation is in the form:
y(t) = C1*cos(7t) + C2*sin(7t)
Step 4: Apply the initial conditions to find the constants.
Apply the initial condition y(0) = 4:
4 = C1*cos(0) + C2*sin(0)
4 = C1
So, C1 = 4
Next, find the first derivative of y(t):
y'(t) = -7*C1*sin(7t) + 7*C2*cos(7t)
Apply the initial condition y'(0) = -5:
-5 = -7*4*sin(0) + 7*C2*cos(0)
-5 = 7*C2
So, C2 = -5/7
Step 5: Write the particular solution.
The particular solution with the determined constants is:
y(t) = 4*cos(7t) - (5/7)*sin(7t)
Learn more about complex conjugates.
brainly.com/question/29960316
#SPJ11
Complete the pattern:
9 + 6 =
9,000,000 +
+ 600 = 1,500
15,000,000
Answers
Answer:
6.00150024e+14
Step-by-step explanation:
i am sorry if im wrong
estimate the error that is made by approximating the sum of the given series by the series the fitst 5 terms 1/k^3
Answers
The error involved in approximating the sum of the given series by the sum of the first five terms of the series is `R5 = 1/6³ + 1/7³ + ...`.
To estimate the error that is made by approximating the sum of the given series by the series the first 5 terms 1/k³, we can use the remainder term of a convergent series.
The given series is ∑ 1/k³ from k = 1 to infinity.We have to find the error involved in approximating the sum of the given series by the sum of the first five terms of the series.
That is, we need to find the difference between the actual sum of the series and the sum of the first five terms of the series.
The sum of the series is given by: `S = 1/1³ + 1/2³ + 1/3³ + 1/4³ + ... + 1/n³ + ...` We can use the remainder term of the series to find the error in approximation.
The remainder term `Rn` is given by: `Rn = Sn - S` where `Sn` is the sum of the first `n` terms of the series. Thus, we have to find the remainder term for `n = 5`.
The remainder term `Rn` is given by: `Rn = S - Sn = 1/6³ + 1/7³ + ...` Since the given series is convergent, the remainder term `Rn` tends to zero as `n` tends to infinity.
So, if we take the sum of the first five terms of the series, the error involved in approximation is given by the remainder term `R5`.
The error involved in this approximation is very small and can be neglected.
To learn more about : terms
https://brainly.com/question/30643700
#SPJ8
If a = (x-3) and b = (x+3), show that 2ab = 2x square - 18
Answers
Step-by-step explanation:
ab = (x - 3)(x + 3)
multiply
ab = x² + 3x - 3x - 9
Simplify
ab = x² - 9
---------------------------
2ab = 2(x² - 9)
Distribute
2ab = 2x² - 18
Which of the following relation graphs represents a function?
A.


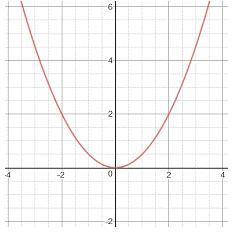

Answers
Answer:
The third one (parabola)
Step-by-step explanation:
Do a vertical line test.
Basically draw vertical lines over the function. If the x-value has more than one y-value then it is a relation and not a function
The diameter of a circle is 32 millimeters. What is the circle's area?
Answers
Answer:
803.84 bc radius is half of diameter which is 16
Area of circle is (pi)r^2 = (3.14)16^2= 3.14 x 256 = 803.84
Step-by-step explanation:
Answer:
The answer is 804.25
Step-by-step explanation:
\(\frac{32}{2}=16\)
\(Area = 3.14 * 16^2\)
A=3.141592654×16×16
804.2477193mm^2
Area 804.248
Two fair dice, one yellow and one blue, are rolled. The value of the blue die is subtracted from the value of the yellow die. Which of the following best describes the theoretical probability distribution?
constant
symmetric
positively skewed
negatively skewed
Answers
Answer:
B. Symmetric
Step-by-step explanation:
PLS HELP ME ASAP I DONT HAVE TIME IT ALSO DETECTS IF ITS RIGHT OR WRONG

Answers
Answer:
5 hours
Step-by-step explanation:
Let x represent the amount of hours that ralph can do.
Let y represent the money he earned.
Let m represent how much he earns per hour.
Let b represent the initial fee
y=mx+b
y=33x + 24
189=33x+24
Solve for x:
189=33x+24
165 = 33x
x=5
Determine the quadratic form (vertex, standard, intercept, or not a quadratic) for the function below.
y=-5(x+2)(x-3)
Answers
Quadratic Form : \(y = 5x^2 - 5x - 30\)
What is quadratic form?
Any equation in the form \(ax^2+bx+c = 0\) is said to be in quadratic form. This equation then can be solved by using the quadratic formula, by completing the square, or by factoring if it is factorable.
Given,
⇒ \(y = 5 (x+2) (x-3)\\ \\y = 5 (x^2-x-6)\\\\y = 5x^2 - 5x - 30\)
Hence,
Quadratic Form : \(y = 5x^2 - 5x - 30\)
Learn more about Quadratic Form from the link
https://brainly.com/question/1214333
#SPJ1
Can you solve it?
find h. (system of equations)

Answers
Ally rents a car for one day. It costs her $35 plus $0.35 per mile. Ally only has $134.75. How many miles can she drive?
Answers
Ally will be able to cover 285 miles for the trip
How to calculate the number of miles ?Ally wants to rent the car for one day
The rents costs $35 and $0.35 per mile
Ally has $134.75 to spend for the trip
The number of miles Ally can drive for the trip can be calculated as follows
134.75 - 35
= 99.75
99.75/0.35
= 285
Hence Ally will be able to drive 285 miles for the trip
Read more on miles here
https://brainly.com/question/7044088
#SPJ1
simplify the following expression

Answers
The simplified form of the expression 2x^2 - 3x - 2 remains as 2x^2 - 3x - 2.
To simplify the expression 8x - 2x - x^2, we can combine like terms by adding or subtracting coefficients.
8x - 2x - x^2
First, let's combine the x terms:
(8x - 2x) - x^2
This simplifies to:
6x - x^2
Therefore, the simplified form of the expression 8x - 2x - x^2 is 6x - x^2.
Now, let's simplify the expression 2x^2 - 3x - 2:
The expression is already in simplified form, and no further simplification is possible.
Therefore, the simplified form of the expression 2x^2 - 3x - 2 remains as 2x^2 - 3x - 2.
for such more question on simplified form
https://brainly.com/question/11680269
#SPJ8
customers arrive at a travel agency at a mean rate of 11 per hour. assuming that the number of arrivals per hour has a poisson distribution, give the probability that strictly more than 5 customers arrive in a given hour.
Answers
To find the probability that strictly more than 5 customers arrive in a given hour, we can use the Poisson distribution with a mean rate of 11 customers per hour.
The probability mass function of the Poisson distribution is given by:
P(X = k) = (e^(-λ) * λ^k) / k!
Where X is the random variable representing the number of arrivals, λ is the mean rate, and k is the desired number of arrivals.
In this case, λ = 11 (mean rate of 11 customers per hour). We want to find the probability that strictly more than 5 customers arrive, which is equivalent to finding the probability that 6, 7, 8, 9, 10, 11, and so on, customers arrive.
Let's calculate the probability using the complement rule, which states that the probability of an event happening is equal to 1 minus the probability of the event not happening.
P(X > 5) = 1 - P(X ≤ 5)
To find P(X ≤ 5), we sum the probabilities for k = 0, 1, 2, 3, 4, and 5:
P(X ≤ 5) = P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3) + P(X = 4) + P(X = 5)
Using the Poisson distribution formula, we can calculate each term:
P(X = k) = (e^(-λ) * λ^k) / k!
P(X = 0) = (e^(-11) * 11^0) / 0! = e^(-11)
P(X = 1) = (e^(-11) * 11^1) / 1! = 11e^(-11)
P(X = 2) = (e^(-11) * 11^2) / 2!
P(X = 3) = (e^(-11) * 11^3) / 3!
P(X = 4) = (e^(-11) * 11^4) / 4!
P(X = 5) = (e^(-11) * 11^5) / 5!
Now we can calculate P(X ≤ 5):
P(X ≤ 5) = e^(-11) + 11e^(-11) + (11^2 * e^(-11)) / 2! + (11^3 * e^(-11)) / 3! + (11^4 * e^(-11)) / 4! + (11^5 * e^(-11)) / 5!
Finally, we can find P(X > 5) by subtracting P(X ≤ 5) from 1:
P(X > 5) = 1 - P(X ≤ 5)
You can calculate this value using a calculator or software that supports mathematical functions.
Visit here to learn more about probability brainly.com/question/31828911
#SPJ11
CAN SOMEONE ANSWER THIS PLEASE AND RHANK YOU!

Answers
Answer:
1/9?
Step-by-step explanation:
15)The demand equation for a certain product is given by the formulap = 32-10.0001x+1where x is the number of units sold in a month and p is the price perunit. If the price is set at $14.75 for the month, how many units will be sold?

Answers
The demand equation is given to be:
\(p=32-\sqrt{0.0001x+1}\)where p is the price and x is the number of units sold.
If the price per unit is $14.75, the number of units will be calculated as follows:
\(\begin{gathered} p=14.75 \\ \therefore \\ 14.75=32-\sqrt{0.0001x+1} \end{gathered}\)Subtracting 32 from both sides, we have:
\(\begin{gathered} -\sqrt{0.0001x+1}=14.75-32 \\ -\sqrt{0.0001x+1}=-17.25 \end{gathered}\)Multiply both sides by -1:
\(\sqrt{0.0001x+1}=17.25\)Square both sides:
\(\begin{gathered} 0.0001x+1=17.25^2 \\ 0.0001x+1=297.5625 \end{gathered}\)Subtract 1 from both sides:
\(\begin{gathered} 0.0001x=297.5625-1 \\ 0.0001x=296.5626 \end{gathered}\)Divide both sides by 0.0001:
\(\begin{gathered} x=\frac{296.5625}{0.0001} \\ x=2965625 \end{gathered}\)The number of units sold will be 2,965,625 units.