given vectors: p= 3i, q= j
find: 1. p+q, 2. p-q, 3. 2p-3q
Answers
Answer:
See below
Step-by-step explanation:
\(p=3i\)
\(q=j\)
\(p+q=3i+j\)
\(p-q=3i-j\)
\(2p-3q=2(3i)-3(j)=6i-3j\)
Related Questions
A car that cost 700,000.00 depreciates 15% in the value each year for the first five years. What is the cost after 5 years?
Answers
Answer:
310559.29375
Step-by-step explanation:
i multiplied the original 700,000 times 0.15 to see what that would equal which was 105,000 then took that away from 700k which was 595,000 then multiplied that by 0.15 which was 89,250 i did this process 5 times and came up with 310,559. 29375
Please help me answer this quickly!!!! Give a thorough explanation and answer every part! Thank youuuuu :) (will give brainliest)

Answers
The features of the sine function are given as follows:
Midline: y = 1.Maximum value: y = 4.Minimum value: y = 2.Amplitude: 3 units.Period: 8 units.Frequency: 1/8 units. (one divided by the period).The equation is given as follows:
y = 3sin(0.25πx) + 1.
How to define the sine function?The standard definition of a sine function is given as follows:
y = Asin(Bx) + C.
The parameters are given as follows:
A: amplitude.B: the period is 2π/B.C: vertical shift.The minimum value is of -2, while the maximum value is of 4, hence the midline is given as follows:
y = (-2 + 4)/2
y = 1.
The difference between the minimum and the maximum value is of 6 units, hence the amplitude is obtained as follows:
2A = 6
A = 3.
The function with no vertical shift would oscillate between -A = -3 and A = 3, while this function oscillates between -2 and 4, hence the vertical shift is given as follows:
C = 1.
The shortest distance between repetitions of the function is of 8 units, hence the period is of 8, the frequency is of 1/8, and the coefficient B is given as follows:
2π/B = 8
8B = 2π
B = π/4.
B = 0.25π.
Hence the function is defined as follows:
y = 3sin(0.25πx) + 1.
More can be learned about trigonometric functions at brainly.com/question/21558626
#SPJ1
Help, Help, Please
\( \sf find \: the \: value \: of \: \theta \: when \: \theta \: is \: acute\: angle\)
\( \sf \cos^{2}(\theta) - \sin^{2}(\theta) =2-5 \cos(\theta) \)
\(\text{note:explanation is a must}\)
Answers
Answer:
θ = \(\frac{\pi }{3}\) (60° )
Step-by-step explanation:
Using the identity
sin²x + cos²x = 1 ⇒ sin²x = 1 - cos²x
Given
cos²θ - sin²θ = 2 - 5cosθ
cos²θ - (1 - cos²θ) = 2 - 5cosθ
cos²θ - 1 + cos²θ = 2 - 5cosθ
2cos²θ - 1 = 2 - 5cosθ ( subtract 2 - 5cosθ from both sides )
2cos²θ + 5cosθ - 3 = 0 ← in standard form
(cosθ + 3)(2cosθ - 1) = 0 ← in factored form
Equate each factor to zero and solve for θ
cosθ + 3 = 0
cosθ = - 3 ← not possible as - 1 ≤ cosθ ≤ 1
2cosθ - 1 = 0
cosθ = \(\frac{1}{2}\) , so
θ = \(cos^{-1}\) (\(\frac{1}{2}\) ) = \(\frac{\pi }{3}\) ( or 60° )
Answer:
\( \huge \boxed{ \boxed{\blue{ { \theta = 60}^{ \circ} }}}\)
Step-by-step explanation:
to understand thisyou need to know about:trigonometryPEMDASlet's solve:\( \sf \: rewrite \: \sin ^{2} ( \theta) \: as \: 1 - \cos ^{2} ( \theta) : \\ \sf \implies \: \cos ^{2} ( \theta) - (1 - \cos ^{2} ( \theta)) = 2 - 5 \cos( \theta) \)\( \sf \: remove \: parentheses \: and \: change \: its \: sign : \\ \sf \implies \: \cos ^{2} ( \theta) - 1 + \cos ^{2} ( \theta)) = 2 - 5 \cos( \theta) \)\( \sf \: add : \\ \sf \implies \: 2\cos ^{2} ( \theta) - 1 = 2 - 5 \cos( \theta) \)\( \sf \: move \: left \: hand \: sides \: expression \: to \: right \: hand \: sides \: : \\ \sf \implies \: 2\cos ^{2} ( \theta) + 5 \cos( \theta) - 1 -2 = 0\)\( \sf \: rewrite \: 5\cos( \theta) \: as \: 6 \cos( \theta) - \cos( \theta) : \\ \sf \implies \: 2\cos ^{2} ( \theta) + 6 \cos( \theta) - \cos( \theta) - 3 = 0\)\( \sf \:factor \: out \: 2 \cos( \theta) \: and \: - 1 : \\ \sf \implies \: 2\cos ( \theta)( \cos( \theta) + 3 ) -1( \cos( \theta) + 3) = 0\)\( \sf \: group: \\ \sf \implies \: (2\cos( \theta) - 1) ( \cos( \theta) + 3 ) = 0\)\( \sf \: rewrite \: as \: two \: seperate \: equation: \\ \sf \implies \: \begin{cases}2\cos( \theta) - 1 = 0\\ \cos( \theta) + 3 = 0 \end{cases} \)\(\sf add \: 1 \: to \: the\: first \: equation \: and \: substract \: 3 \: from \: the \: second \: equation: \\ \sf \implies \: \begin{cases}2\cos( \theta) = 1\\ \cos( \theta) = - 3 \end{cases} \)\( \sf the \: second \: eqution \: is \: false \: \\ \sf because \: - 1 \leqslant \cos( \theta) \leqslant 1 \: \\ \sf but \: we \: can \: still \: work \: with \: the \: second \: equation\)
\( \sf substract \: both \: sides \: by \: 2 : \\ \implies\frac{ 2\cos( \theta) }{2} = \frac{1}{2} \\ \implies\cos( \theta) = \frac{1}{2} \\ \therefore \: \theta \: = {60}^{ \circ} \)what units do scientist use to measure force
Answers
Answer: newtons
Step-by-step explanation:
Answer:
I believe it is Newtons :)
Step-by-step explanation:
Given l || m
n, find the value of x.
m

Answers
The value of 'x' is highlighted in the box

In the input/output rule: y = -3x - 7 What
would be the output of an input of 5? Write
only the number.
Answers
Answer:
-22
Step-by-step explanation:
Plug in 5 for x.
y = -3(5) - 7
y = -15 - 7
y = -22
An online music store charges $1.25 for each song after you pay a $25 subscription fee. write a function that represents the arithmetic sequence. how many songs
can you buy if you have $57 to spend?
Answers
Step by step
Using slope intercept formula for inequality
y ≥ mx +b
y = value
m = rate or slope, here it is price per song
x = variable, here it is how many songs
b = set cost or starting point, here it’s the set subscription fee
57 ≥ 1.25x + 25
Subtract 25 from both sides to isolate x
57 - 25 ≥ 1.25x + 25 - 25
32 ≥ 1.25x
Now divide both sides by 1.25 to solve for x
32/1.25 ≥ 1.25/1.25x
25.6 ≥ x
This tells us x is less than or equal to 25.6
Since we cannot buy a percent of a song, we round down to 25 songs
Check your work, substitute 25 for x
57 ≥ 1.25 (25) + 25
57 ≥ 31.25 + 25
57 ≥ 56.25
Statement is true
57 is more than or equal to 56.25
plz hurry!! A person standing close to the edge on top of a 108-foot building throws a ball vertically upward. The quadratic function h ( t ) = − 16 t 2 + 132 t + 108 h ( t ) = - 16 t 2 + 132 t + 108 models the ball's height above the ground, h ( t ) h ( t ) , in feet, t t seconds after it was thrown. a) What is the maximum height of the ball?
Answers
Answer:
The maximum height of the ball is 380.25 feet in the air.
Step-by-step explanation:
The quadratic function:
\(h(t)=-16t^2+132t+108\)
Models the ball's height h(t), in feet, above the ground t seconds after it was thrown.
We want to determine the maximum height of the ball.
Note that this is a quadratic function. Therefore, the maximum or minimum value will always occur at its vertex point.
Since our leading coefficient is leading, we have a maximum point. So to find the maximum height, we will find the vertex. The vertex of a quadratic equation is given by:
\(\displaystyle \left(-\frac{b}{2a},f\left(\frac{b}{2a}\right)\right)\)
In this case, a = -16, b = 132, and c = 108. Find the t-coordinate of the vertex:
\(\displaystyle t=-\frac{132}{2(-16)}=-\frac{132}{-32}=\frac{33}{8}=4.125\)
So, the maximum height occurs after 4.125 seconds of the ball being thrown.
To find the maximum height, substitute this value back into the equation. Thus:
\(h(4.125)=-16(4.125)^2+132(4.125)+108=380.25\text{ feet}\)
The maximum height of the ball is 380.25 feet in the air.
E probability of observing the experiment result, a sample mean, for example, or something more unusual just by chance if the null hypothesis is true is the definition of _____________.
Answers
The probability of observing the experiment result, a sample mean, for example, or something more unusual just by chance if the null hypothesis is true is the definition of "P-Value."
What is null hypothesis?A null hypothesis is a sort of statistical hypothesis which asserts that there is no statistical significance in a particular set of observations.
Using sample data, hypothesis testing is performed to determine the believability of a theory. It really is expressed as H0 and is also known to simply as "null."
Some key features regarding null hypothesis are-
A null hypothesis is a statistical conjecture that claims there is no variation between particular qualities of a population and data-generating process.The alternate hypothesis asserts the existence of a distinction.Hypothesis testing allows you to reject the null hypothesis with a particular level of confidence.If the null hypothesis can be rejected, it lends support to the alternative hypothesis.The notion of falsity in science is founded on null hypothesis testing.To know more about null hypothesis, here
https://brainly.com/question/14016208
#SPJ4
aa please help thanks if you do (:

Answers
Answer:
12.5
Step-by-step explanation:
A=HbB/2=5*5/2=12.5
Define T in L(C2) by T(w,z)=(−z,w).
Find the generalized eigenspaces corresponding to the distinct eigenvalues of T.
I believe that once I have the eigenvalues, I know how to find the eigenspaces, but I'm not sure I'm looking for the eigenvalues correctly.
I know that if the eigenvalues are a,b corresponding to (w,0) and (0,z) respectively, then (ab)=1, since a(w)=−z implies ab(−z)=−z. But I think my whole approach to this is wrong and that I'm missing some very elementary idea.
Answers
T is the linear transformation described by T(w,z) in L(C2). This indicates that the linear transformation T changes two-dimensional vectors of the type (w,z) to (-z,w).
To get T's eigenvalues, solve the equation T(v) = v, where is the eigenvalue and v is the eigenvector. By solving this equation, we discover that T's eigenvalues are λ1 = -1 and λ2 = 1.
For each eigenvalue, the associated eigenspaces are the subspaces of C2 that are spanned by the eigenvectors of T. The eigenspace is the subspace covered by the eigenvector λ1= -1 is (1,0). The eigenspace is the subspace spanned by the eigenvector λ2= 1 is (0,1).
As a result, the subspaces covered by (1,0) and (1,1) are the generalized eigenspaces corresponding to the different eigenvalues of T. (0,1).
Learn more about eigenvalues here:
https://brainly.com/question/15423383
#SPJ4
A line that includes the points (6, 8) and
(f, -7) has a slope of 5. What is the value
of f?
Answers
Answer:
f = 3
Step-by-step explanation:
Slope m = (y2-y1)/(x2-x1)
Using (6,8) and (f,-7) then
m = (-7 - 8)/(f - 6) = 5
- 15 = 5(f - 6)
-15 = 5f - 30
5f = 30 - 15
5f = 15
f = 3
100 Points!!!
Polynomial Identities
Part 1. Pick a two-digit number greater than 25. Rewrite your two-digit number as a difference of two numbers. Show how to use the identity (x − y)2 = x2 − 2xy + y2 to square your number without using a calculator.
Part 2. Choose two values, a and b, each between 8 and 15. Show how to use the identity a3 + b3 = (a + b)(a2 − ab + b2) to calculate the sum of the cubes of your numbers without using a calculator.
Answers
Let's see
#a
Take 28
(28)²(30-2)²30²-2(30)(2)+2²900-120+4780+4784#2
Take 9,10
9³+10³(9+10)(9²-9×10+10²)(19)(81-90+100)19(181-90)19(91)1729Answer:
Two-digit number greater than 25: 32
Rewrite 32 as the difference of 2 numbers: 40 - 8
Therefore, x = 40 and y = 8
\(\begin{aligned}\implies (40-8)^2 & =40^2-2(40)(8)+8^2\\ & = (4 \cdot 10)^2-(80)(8)+64\\ & = 4^2 \cdot 10^2-640+64\\ & = 16 \cdot 100-640+64\\ & = 1600-640+64\\ & = 960+64\\ & = 1024\end{aligned}\)
Let a = 10
Let b = 11
\(\begin{aligned}\implies 10^3+11^3 & =(10+11)(10^2-10 \cdot 11+11^2)\\& = 21(100-110+121)\\ & = 21(-10+121)\\ & = 21(111)\\& = 21 (100 + 10 + 1)\\ & = (21 \cdot 100)+(21 \cdot 10)+(21 \cdot 1)\\ & = 2100 +210+21\\ & = 2310 + 21\\ & = 2331\end{aligned}\)
At a coffee shop, the amount of tax due is calculated based on the cost of the customer's
order.
t = the amount of tax due
c = the cost of the order
Which of the variables is independent and which is dependent?
Answers
In this context, the dependent variable is t (amount of tax due) and the independent variable is c (cost of the order).In this scenario, the variables are t (the amount of tax due) and c (the cost of the order).
To determine which variable is independent and which is dependent, we need to understand their relationship.In this case, the amount of tax due, t, is calculated based on the cost of the customer's order, c. The tax amount is dependent on the cost of the order because it is directly influenced by the value of c. As the cost of the order changes, the amount of tax due will also change accordingly.
On the other hand, the cost of the order, c, is independent. It is not influenced or determined by the amount of tax due. The customer can choose the cost of their order, and the tax will be calculated based on that chosen amount.
For more such questions on variable
https://brainly.com/question/27894163
#SPJ8
Initially, there were equal amounts of bananas and kiwis on the table. Sarah
put 6 bananas and 8 kiwis in each basket. After the baskets were ready there were 16
bananas and 8 kiwis left on the table. How many baskets were made?
Answers
The number of baskets that were made is 4.
How many baskets were made?The first step is to form a pair of simultaneous equations using the information provided in the question:
x - 6y = 16 equation 1
x - 8y = 8 equation 2
Where:
x = the initial number of bananas and kiwisy = the number of the basketsThe elimination method would be used to solve for the number of baskets :
Subtract equation 2 from equation 1:
2y = 8
Divide both sides of the equation by 2L
y = 8 / 2
y = 4
To learn more about simultaneous equations, please check: https://brainly.com/question/25875552
#SPJ1
For the month of June in a certain city, 87% of the days are cloudy. Also in the month of June in the same city, 73% of the days are cloudy and foggy. What is the probability that a randomly selected day in June will be foggy if it is cloudy
Answers
The probability that a randomly selected day in June will be foggy if it is cloudy can be determined by calculating the conditional probability of foggy days given cloudy days.
Let's denote the events as follows: C represents a cloudy day, and F represents a foggy day. We are given that the probability of a day being cloudy is 0.87 (87%) and the probability of a day being both cloudy and foggy is 0.73 (73%).
To find the probability of a day being foggy given that it is cloudy, we use the formula for conditional probability:
P(F | C) = P(F and C) / P(C)
In this case, P(F and C) represents the probability of a day being both foggy and cloudy, which is given as 0.73 (73%), and P(C) represents the probability of a day being cloudy, which is given as 0.87 (87%).
Therefore, the probability of a randomly selected day in June being foggy if it is cloudy can be calculated as:
P(F | C) = 0.73 / 0.87 ≈ 0.839
Learn more about probability here:
https://brainly.com/question/31828911
#SPJ11
help me with math, its 8th grade 100 points look at attachment
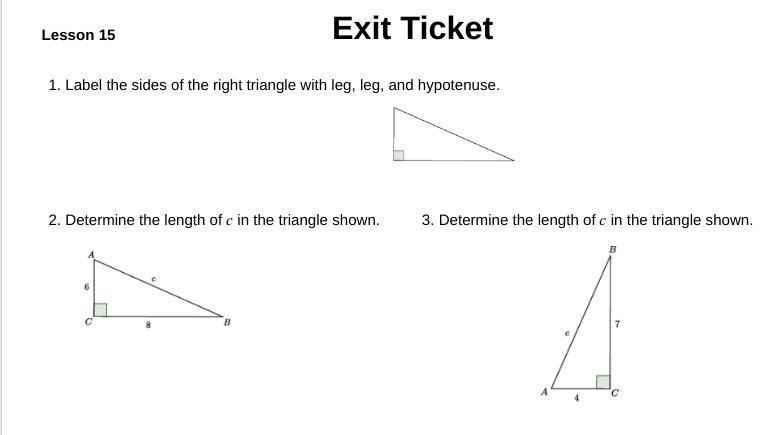
Answers
Answer:
1)
The hypotenuse is the longest side of a triangle. It is ALWAYS the one across from the right angle (depicted as the box in the corner at 90 degrees). Therefore the 2 sides that intersect the 90-degree angle are "leg" and "leg" and the longest side is the"hypotenuse"
2)
The Pythagorean theorem states: \(a^{2} + b^{2} = c^{2}\) where a and b are sides and c is the hypotenuse.
\(a^{2} + b^{2} = c^{2} \\8^{2} + 6^{2} = c^{2} \\64 + 36 = c^{2} \\100 = c^{2} \\\sqrt{100} = c\\10 = c\)
For your purposes, a and b do not matter which number you choose as long as you make sure that they are sides and not the hypotenuse.
3)
The Pythagorean theorem states: \(a^{2} + b^{2} = c^{2}\) where a and b are sides and c is the hypotenuse.
\(a^{2} + b^{2} = c^{2} \\4^{2} + 7^{2} = c^{2} \\16 + 49= c^{2} \\65 = c^{2} \\\sqrt{65} = c\\8.0622 = c\)
Hope that helps!
Probability of dependent events

Answers
Answer:
1/6
Step-by-step explanation:
4/9 live in Wells, so the probability of ONE winner being from Wells is 4/9. Now there are 8 people left, and 3 live in Wells. The odds of another person being chosen from Wells is 3/8.
4/9x3/8=12/72
12/72=1/6
Write an equation in slope-intercept form of the line that is a segment bisector of both

Answers
Building the Cartesian Plane with the proper scale we can conclude that
Y = - 8X + 10


Sorry another question!

Answers
Answer:
y =5
Step-by-step explanation:
2y + 4y = 30
6y = 30
Divide both sides by six
y = 30/6
y = 5
Answer:
= 5
Explain: Combine like terms
3 0 = 2 + 4
30=6y
Divide both sides of the equation by the same term
3 0 = 6
30/6 = 6y/6
Simplify:
1)Divide the numbers
2)Cancel terms that are in both the numerator and denominator
3)Move the variable to the left
= 5
Please answer this is due soon! (There's a screenshot and the last box says "check")

Answers
inverse operations = x=.5
simplify = x=1/2
inverse operations = x=.5
solution = x=1/2
check:
Let x = 3/2
10×3/2-5=10
use this rule = a×b/c=ab/c
10×3/2-5=10
Simplify 10×3 to 30.
30/2-5=10
Simplify 30/2 to 15
15-5=10
Simplify 15-5=10
10=10
Checked✅
HELPPP ME WITH THIS I DONT GET IT PLEASE

Answers
The value of x for the given triangle will be 4√3.
What is a triangle?A triangle is a 3-sided shape that is occasionally referred to as a triangle. There are three sides and three angles in every triangle, some of which may be the same.
The sum of all three angles inside a triangle will be 180° and the area of a triangle is given as (1/2) × base × height.
As per the given triangle,
The unknown third angle will be 180° - 60° - 30° = 90°.
Thus, it is a right-angle triangle so a trigonometric ratio will apply.
By sin60° = x/8
x = 8(√3/2) = 4√3
Hence "The value of x for the given triangle will be 4√3".
For more about triangles,
https://brainly.com/question/2773823
#SPJ1
Peyton has a number cube and a
coin. What is the probabiltiy that he
will roll a prime number and flip tails?
(ANSWER IN PERCENT
Answers
Answer:
Hello Darling! Answer: 14%
Step-by-step explanation:
Now I'm not always accurate, I can re-check myself if you think it isn't right! c;
sarah is playing a game in which she rolls a number cube 20 times the results are recorded in the chart below. what is the experimental probability of rolling a 1 or a 2? answers 0.3, 0.45, 0.65, 1.25.
Answers
The experimental probability of rolling a 1 or a 2 is 0.2.
Hence, Option A is correct.
We know that,
The experimental probability of an event is defined as the number of times the event occurred divided by the total number of trials.
In this case,
The event is rolling a 1 or a 3,
Which occurred ⇒ 3 + 1
= 4 times.
Given that there are total number of trials = 20.
Therefore,
The experimental probability of rolling a 1 or a 3 = 4/20,
= 1/5
= 0.2
Hence, the required probability is 0.2.
Learn more about the probability visit:
https://brainly.com/question/13604758
#SPJ1
The complete question is:
Sarah is playing a game in which she rolls a number cube 20 times. The results are recorded in the chart below. What is the experimental probability of rolling a 1 or a 3?
Number on cube:1,2,3,4,5,6
Number of times event occurs:3,6,1,5,3,2
A.0.2
B.0.3
C.0.6
D.0.83
What is the answer to this?
-72 = 8d
Answers
Answer:
-9 = d
Step-by-step explanation:
Answer:
d= -9
Step-by-step explanation:
Lucas has a 15 ft ladder that he placed against a wall.
How far will the wall ladder reach when the base of the
ladder is 9 ft from the wall?
Answers
Answer:
The ladder will go 6 feet up.
10x⁵+18
Factor the expression completely
Answers
2(5x^5 + 9)
Solve for x.
1/2x = -9
x = -18
x = -4
x = 18
Answers
Answer:
x = -18
Step-by-step explanation:
1/2x = -9
(21)1/2x = -9(2/1)
x = -9/1(2/1)
x = -18/1
x = -18
Answer:
x = -18
Step-by-step explanation:
1/2x = -9
Multiply each side by 2
1/2x * 2 = -9*2
x = -18
Bruce has been offered a position selling bungee seat belts to sports car manufacturers. He was given a choice of receiving a monthly salary of $500 plus a bonus of 25% of his monthly sales or a $2,000 monthly salary with a 5% bonus on sales. How much would Bruce have to sell to make the same amount of money from each?
Answers
Answer:
$7500
Step-by-step explanation:
Let x = amount of monthly sales.
Choice A:
"monthly salary of $500 plus a bonus of 25% of his monthly sales"
monthly salary = 0.25x + 500
Choice B:
"$2,000 monthly salary with a 5% bonus on sales"
monthly salary = 0.05x + 2000
We set the total monthly salaries equal and solve for x.
0.25x + 500 = 0.05x + 2000
0.2x = 1500
x = 1500/0.2
x = 7500
Answer: $7500
4. Write an equation in the form of y = kx for the following situation. A person can burn 750
calories in 60 minutes.
Answers
Answer:
k750×60x=Ohio
Step-by-step explanation:
Swag like Ohio down in Ohio